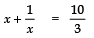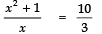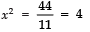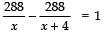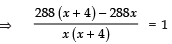Class 10 Maths Chapter 4 Question Answers - Quadratic Equations
Q1: A two-digit number is 5 times the sum of its digits and is also equal to 5 more than twice the product of its digits. Find the number.
Let the tens digit = x
And the ones digit = y
∴ The number = 10x + y
According to the conditions,
10x + y = 5 (x + y) ...(1)
10x + y = 2xy + 5 ...(2)
From (1), we have
10x + y = 5x + 5y
⇒ 10x + y − 5x − 5y =0
⇒ 5x − 4y = 0⇒ 5x = 4y or y = 54 x
Substituting y = 54 x in (2), we get
10x + 5x4 = 5x22 + 5
⇒ 40x + 5x = 10x2 + 20 [Multiplying both sides by 4]
⇒ 45x = 10x2 + 20
⇒ 10x2 − 45x − 20 = 0
⇒ 2x2 − 9x − 4= 0
⇒ 2x2 − 8x − x + 4 = 0
⇒2x (x − 4) − 1 (x − 4) = 0
⇒ (x − 4) (2x − 1) = 0
Either x − 4 = 0 ⇒ x = 4
or 2x − 1 = 0 ⇒ x = 1/2
But a digit cannot be a fraction,
∴ x = 4
⇒ The tens digit = 4
∴ x = 4 and y = 5
∴ The required number = 10 × 4 + 5
= 40 + 5 = 45
Q2: The denominator is one more than twice the numerator. If the sum of the fraction and its reciprocal is find the fraction.
Let the numerator be x
∴ Denominator = (2x + 1)
∴ Fraction = x/(2x +1)
Reciprocal of the fraction = 2x +1/x
According to the condition,x2x + 1 + 2x + 1x = 216 = 5821
⇒ x × x + (2x + 1)(2x + 1)x(2x + 1) = 5821
⇒ x2 + (2x + 1)22x2 + x = 5821
⇒ x2 + 4x2 + 4x + 12x2 + x = 5821
⇒ 5x2 + 4x + 12x2 + x = 5821
⇒ 21 (5x2 + 4x + 1) = 58 (2x2 + x)
⇒ 105x2 + 84x + 21 = 116x2+ 58x
⇒ 105x2 − 116x2 + 84x − 58x + 21 = 0
⇒ − 11x2 + 26x + 21 = 0
⇒ 11x2 − 26x − 21 = 0 ...(1)Comparing (1) with ax2 + bx + c = 0, we have:
a =11
b = − 26
c = − 21
∴ b2 − 4ac =(− 26)2 − 4 × 11 (− 21) = 676 + 924 = 1600Since, x = -b ± √(b2 - 4ac)2a
∴ x = -(-26) ± √16002(11)
⇒ x = 26 ± 4022
Taking the +ve sign,
x = 26 + 4022 = 6622 = 3
Taking the -ve sign,
x = 26 - 4022 = -1422 = -711
But the numerator cannot be -7/11
∴ x = 3 ⇒ The numerator = 3
∴ Denominator = 2 (3) + 1 = 7
Thus, the required fraction = 3/7
Q3: The sum of a number and its reciprocal is 10/3. Find the number.
Let the required number = x
∴ Its reciprocal = 1/x
According to the condition, we have:
⇒
⇒⇒ 3 (x2 + 1) = 10x
⇒ 3x2 + 3 − 10x = 0
⇒ 3x2 − 10x + 3 = 0
⇒ 3x2 − 9x − x + 3 = 0
⇒ 3x (x − 3) − 1 (x − 3) = 0
⇒ (x − 3) (3x − 1) = 0Either x −3 = 0 ⇒ x = 3
or 3x − 1 = 0 ⇒ x = 1/3
Thus, the required number is 3 or 1/3.
Q4: The hypotenuse of a right triangle is cm. If the smaller side is tripled and the longer side doubled, the new hypotenuse will be
cm. How long are the sides of the triangle?
Let the smaller side = x
∴ Longer side = √((Hypotenuse)2 - (Smaller side)2)
= √( 310 )2 - x2
= √(9 × 10 - x2)
= √(90 - x2)
According to the condition, we have [3 (Smaller side)]2 + [2 (Longer side)]2 = [New Hypotenuse]2
⇒ [3(x)]2 + [2 √(90 - x2)]2 = [9√5]2
⇒ 9x2 + 4(90 - x2) = 81 × 5
⇒ 9x2 + 360 - 4x2 = 405
⇒ 5x2 = 405 - 360
⇒ 5x2 = 45
⇒ x2 = 455 = 9
⇒ x = ±√9 = ±3
But x = -3 is not required, because the side of a triangle cannot be negative.
∴ x = 3 ⇒ Smaller side = 3 cm
Longer side = √(90 - 32)
= √(90 - 9)
= √81 = 9 cm
Thus, the required sides of the triangle are 3 cm and 9 cm.
Q5: A motorboat goes 10 km upstream and returns back to the starting point in 55 minutes. If the speed of the motorboat in still water is 22 km/hr, find the speed of the current.
Let the speed of the current = x km/hr
∴ The speed downstream = (22 + x) km/hr
The speed upstream= (22 − x) km/hrSince, Time = Distance/Speed
∴ Time for going 10 km downstream
= 1022 + x hrs
Time for returning back 10 km upstream = 1022 - x hours
According to the condition,
1022 + x + 1022 - x = 5560
⇒ 10 × 122 + x + 122 - x = 1112
[∵ 55 minutes = 5560 hours]
⇒ 10 × 12 (22 + x + 22 - x)(22 + x)(22 - x) = 11
⇒ 120 × 44484 - x2 = 11
⇒ 11 (484 − x2) = 120 × 44
⇒ 5324 − 11x2 = 5280
⇒ 11x2 = 5324 − 5280 = 44
⇒
⇒ x = ± 2
But speed of the current cannot be negative,∴ x = 2
⇒ Speed of current = 2 km/hr
Q6: A motorboat whose speed in still water is 5 km/hr, takes 1 hour more to go 12 km upstream than to return downstream to the same spot. Find the speed of the stream.
Let the speed of the stream be x km/hr ∴ Downstream speed of the motor boat = (x + 5) km/hr
⇒ Time taken to go 12 km upstream= 12/5 − x hours
Time taken to return 12 km downstream
= 12/5 + x hours
According to the condition
∴12 (5 + x) − 12 (5 − x) = 1 (5 − x) (5 + x)
⇒ 60 + 12x − 60 + 12x = 25 − x2
⇒ 24x = 25 − x2
⇒ x2 + 24x − 25 = 0
⇒ x2 + 25x − x − 25 = 0
⇒ x (x + 25) − 1 (x + 25) = 0
⇒ (x − 1) (x − 25) = 0Either x − 1 = 0 ⇒ x = 1
or x + 25 = 0 ⇒ x = − 25
But x = − 25 is not admissible, because the speed of the stream cannot be negative∴ x = 1
⇒ speed of the stream = 1 km/hr.
Q7: The sum of the areas of two squares is 260 m2. If the difference of their perimeters is 24 m, then find the sides of the two squares.
Let the side of one of the squares be ‘x’ metres
∴ Perimeter of square-I = 4 × x metres = 4x metres
∴ Perimeter of square-II = (24 + 4x) metres
∴ Side of the square-IImetres
= (6 + x) metresNow, according to the condition, we have:
x2 + (6 + x)2 = 260
⇒x2 + 36 + x2 + 12x − 260 = 0
⇒ 2x2 + 12x − 224 = 0
⇒ x2 + 6x − 112 = 0 ...(1)Comparing (1) with ax2 + bx + c = 0, we get,
a = 1
b = 6
c = − 112∴ b2 − 4ac = (6)2 − 4 (1) (− 112)
= 36 + 448 = 484
∴ x = -b ± √(b2 - 4ac)2a
⇒ x = -6 ± √4842(1)
⇒ x = -6 ± 222
Taking the +ve sign, we have
x = -6 + 222 = 162 = 8
Taking the -ve sign, we have
x = -6 - 222 = -282 = -14
But x = − 14 is not required, as the length of a side cannot be negative.
∴ x = 8
⇒ Side of square-I = 8 m
⇒ Side of square-II = 6 + 8 m = 14 m.
Q8: The age of a father is twice the square of the age of his son. Eight years hence, the age of the father will be 4 years more than three times the age of his son. Find their present ages.
Let the present of son be ‘x’ years.
∴ Father’s present age = 2x2 years 8 years hence:Age of son = (x + 8) years
Age of father = (2x2 + 8) yearsAccording to the condition:
(2x2 + 8) = 3 (x + 8) + 4
⇒ 2x2 + 8 − 3x − 24 − 4= 0
⇒ 2x2 − 3x + 8 − 28 = 0
⇒ 2x2 − 3x − 20 = 0 ...(1)Comparing (1) with ax2 + bx + c = 0, we get
a = 2
b = −3
c = −20∴ b2 − 4ac =(− 3)2 − 4 (2) (− 20)
= 9 + 160 = 169Now, x = -b ± √(b2 - 4ac)2a
∴ x = -(-3) ± √1692(2) = 3 ± 134
Taking the +ve sign,
x = 3 + 134 = 164 = 4
Taking the -ve sign,
x = 3 - 134 = -104 = -52
But x = -15/2 is not required, as the agecannot be negative.
∴ x = 4
⇒ Present age of son = 4 years
Present age of father = 2 × 42 = 32 years.
Q9: A motorboat whose speed in still water is 16 km/h, takes 2 hours more to go 60 km upstream than to return to the same spot. Find the speed of the stream.
Let the speed of the stream = x km/hr For the motor boat, we have:
∴Downstream speed = (16 + x) km/hr
Upstream speed = (16 − x) km/hr
For going 60 km:
According to the condition:
⇒ 60 (16 + x) − 60 (16 − x) = 2 (16 − x) (16 + x)
⇒ 960 + 60x − 960 + 60x = 2 (256 − x2)
⇒ 2x2 + 120x = 2 × 256 −2x2
⇒ x2 + 60x = 256
⇒ x2 + 60x − 250 = 0 ...(1)Comparing (1) with ax2 + bx + c = 0,
a = 1
b = 60
c = − 256∴ b2 − 4ac = (60)2 − 4 (1) (− 256)
1 = 3600 + 1024 = 4624∴ x = -b ± √(b2 - 4ac)2a
⇒ x = -60 ± √46242(1)
⇒ x = -60 ± 682
Taking the +ve sign,
x = -60 + 682 = 82 = 4
Taking the -ve sign,
x = -60 - 682 = -1282 = -64
Since, the speed of a stream cannot be negative,
∴x = − 64 is not admissible
∴ x = 4
⇒ speed of the stream = 4 km/hr.
Q10: A train travels 288 km at a uniform speed. If the speed had been 4 km/hr more, it would have taken 1 hour less for the same journey. Find the speed of the train.
Let the speed of the train be x km/hr
∵ Total distance travelled = 288 km
In the other case, Speed of the train = (x + 4) km/hr
According to the condition,⇒ 288x + 1152 − 288x = 1 (x) (x + 4)
⇒ 288x + 1152 − 288x = x2 + 4x
⇒ 1152 = x2 + 4x
⇒ x2 + 4x − 1152 = 0 ...(1)Comparing (1) with ax2 + bx + c = 0,
a = 1
b = 4
c = − 1152∴ b2 − 4ac = (4)2 − 4 (1) (− 1152) = 16 + 4608
⇒ x = -b ± √(b2 - 4ac)2a
⇒ x = -(-4) ± √46082(1) = -4 ± 682
Taking the +ve sign,
x = -4 + 682 = 642 = 32
Taking the -ve sign,
x = -4 - 682 = -722 = -36
∵ speed cannot be negative,
∴ x ≠− 36
∴ x = 32
⇒ speed of the train = 32 km/hr.
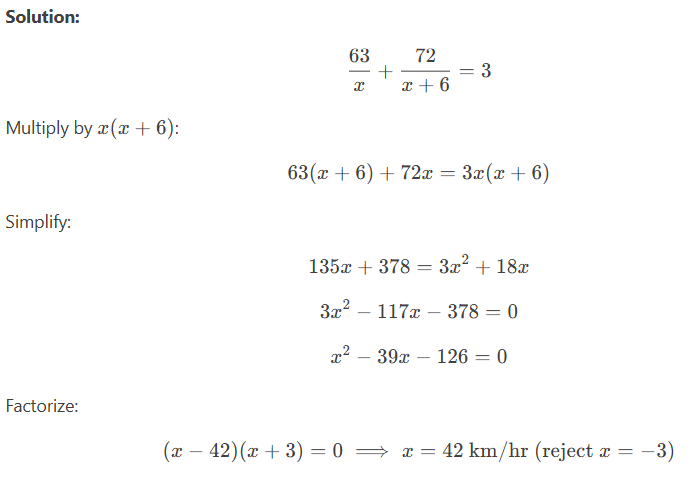
Q11: A train travels at a certain average speed for a distance of 63 km and then travels a distance of 72 km at an average speed of 6 km/hr more than its original speed. If takes 3 hours to complete the total journey, what is its original average speed?
Q12: In a class test, the sum of Gagan’s marks in Mathematics and English is 45. If he had 1 more mark in Mathematics and 1 less in English, the product of marks would have been 500. Find the original marks obtained by Gagan in Mathematics and English separately.
Let Gagan’s marks in maths = x and Marks in English = (45 − x)
∴ According to the condition, (x + 1) × (45 − x + 1) = 500
⇒ (x + 1) × (44 − x) = 500
⇒ 44x − x2 + 44 − x = 500
⇒− x2 + 44x − 456 − x =0
⇒ x2 − 43x + 456 = 0
⇒ x2 − 19x − 24x + 456 = 0⇒ x (x − 19) − 24 (x − 19) = 0
⇒ (x − 19) (x − 24) = 0Either x − 19 = 0 ⇒ x = 19
or x − 24 = 0 ⇒ x = 24
When x = 19, then 45 − 19 = 26
When x = 24, then 45 − 24 = 21
∴ Gagan’s marks in Maths = 19 and in English = 26Or
Gagan’s marks in Maths = 24 and in English = 21.
Q13: In a class test, the sum of Kamal’s marks in Mathematics and English is 40. Had he got 3 marks more in Mathematics and 4 marks less in English, the product of his marks would have been 360. Find his marks in two subjects separately.
Let Kamal’s marks in Maths = x
∴ His marks in English = (40 − x) According to the condition,(x + 3) [40 − x − 4] = 360
⇒ (x + 3) (36 − x) = 360
⇒ 36x − x2 + 108 − 3x − 360 = 0
⇒ − x2 + 33x − 252 = 0
⇒ x2 − 33x + 252 = 0
⇒ x2 − 21x − 12x + 252 = 0⇒ x (x − 21) − 12 (x − 21) = 0
⇒(x − 21) (x − 12) = 0
Either (x − 21) = 0
⇒ x = 21 = 0
or (x − 12) = 0
⇒ x = 12
For x = 21, Marks of Kamalin Maths = 21
in English = 40 − 21 = 19For x = 12, Marks of Kamal
in Maths = 12
in English = 40 − 12 = 28.
|
127 videos|584 docs|79 tests
|
FAQs on Class 10 Maths Chapter 4 Question Answers - Quadratic Equations
| 1. What is a quadratic equation? |  |
| 2. How do you solve a quadratic equation using the quadratic formula? |  |
| 3. What are the different methods to solve quadratic equations? |  |
| 4. How can quadratic equations be applied in real-life situations? |  |
| 5. What is the discriminant of a quadratic equation? How does it determine the nature of solutions? |  |