Class 10 Mathematics: CBSE Sample Question Paper (2019-20) - 9 | Mathematics (Maths) Class 10 PDF Download
Class X
Mathematics – Standard (041)
Sample Question Paper 2019-20
Max. Marks: 80
Duration : 3 hrs
General Instructions:
(i) All the questions are compulsory.
(ii) The question paper consists of 40 questions divided into 4 sections A, B, C, and D.
(iii) Section A comprises of 20 questions of 1 mark each. Section B comprises of 6 questions of 2 marks each. Section C comprises of 8 questions of 3 marks each. Section D comprises of 6 questions of 4 marks each.
(iv) There is no overall choice. However, an internal choice has been provided in two questions of 1 mark each, two questions of 2 marks each, three questions of 3 marks each, and three questions of 4 marks each. You have to attempt only one of the alternatives in all such questions.
(v) Use of calculators is not permitted.
Section A
Q.1. For some integer q, every odd integer is of the form : (1 Mark)
(a) q
(b) q + 1
(c) 2q
(d) 2q + 1
Ans. (d)
According to Euclid's division
lemma, a = bq + r, where o < r < b.
If b = 2, then a = 2q or a = 2q + 1
Since a = 2q + 1 is not divisible by 2, then 2q + 1 is an odd integer.
Q.2. The product of the HCF and LCM of the smallest prime number and the smallest composite number is : (1 Mark)
(a) 1
(b) 2
(c) 4
(d) 8
Ans. (d)
[Smallest prime number = 2 Smallest composite number = 4 Product of their HCF and LCM = 2x4 = 8]
Q.3. In right angled ΔABC at ∠B, sin A is equal to (1 Mark)
(a) -cos A
(b) cos C
(c) sin C
(d) None of these
Ans. (b)
In a right angled ΔABC, sin A = sin(90°- C)= cosC [∵ A + C = 90°]
Q.4. The value of (1 + cot2 2θ) sin2 2θ is (1 Mark)
(a) 1
(b) 0
(e) - 1
(d) 2
Ans. (a)
We have,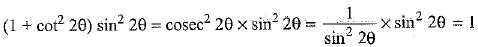
Q.5. In the figure given below, ∠BAC = 90° and AD ⊥ BC. Then: (1 Mark)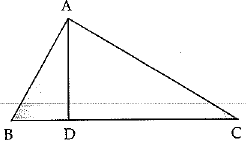
(a) BD x CD = BC2
(b) AB x AC = BC2
(c) BD x CD = AD2
(d) AB x AC = AD2
Ans. (c)
In ΔABD and ΔCAD, ∠ADB = ∠ADC = 90° and ∠ABD = ∠CAD = θ. By AA Similarity, we get, ΔABD ~ ΔCAD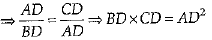
Q.6. Given that sin α = 1/2 and cos β = 1/√2, then the value of a + β is : (1 Mark)
(a) 75°
(b) 30°
(c) 45°
(d) 90°
Ans. (a)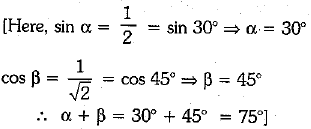
Q.7. For an acute angle θ, sin θ + cos θ takes the greatest value when θ is (1 Mark)
(a) 30°
(b) 45°
(c)60°
(d) 90°
Ans. For θ = 45°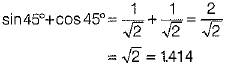
Q.8. If sec A = cosec B, then A + B = _____. (1 Mark)
Ans. 90°
We have,
sec A = cosec B
⇒ sec A = sec (90° - B)
⇒ A = 99° - B
⇒ A+B = 90°
Q.9. A cylindrical pencil sharpened at one edge is the combination of : (1 Mark)
(a) a cone and a cylinder
(b) frustum of a cone and a cylinder
(c) a hemisphere and a cylinder
(d) two cylinders
Ans. (a)
The sharpened part of the pencil is cone and unsharpened part is cylinder.
Q.10.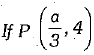 is the mid-point of the line segment joining the points Q (- 6, 5) and R (-2, 3), then the value of a is: (1 Mark)
is the mid-point of the line segment joining the points Q (- 6, 5) and R (-2, 3), then the value of a is: (1 Mark)
(a) - 12
(b) 12
(c) 4
(d) - 6
Ans. (a)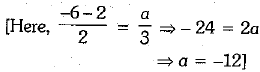
Q.11. If point P lies inside the circle, then number of tangent(s) drawn from point P is .... (1 Mark)
Ans. (No tangent) If point P lies inside the circle, then no tangent will be drawn.
Q.12. The value of tan 48°.tan 13°.tan 42°.tan 77° is 1. (1 Mark)
Ans. True.
We have, tan 48° tan 13° tan 42° tan 77°
= tan (90° - 42°) tan 13° tan 42° tan (90° - 13°)
= cot 42° tan 13° tan 42° cot 13° [∵ tan (90° - A) = cot A]
= (tan 42° cot 42°)(tan 13° cot 13°)
= 1 x 1 = 1 [∵ tan A cot A = 1]
Q.13. What is the perimeter of the sector with radius 10.5 cm and sector angle 60º ? (1 Mark)
Or
If the length of the ladder placed against a wall is twice the distance between the foot of the ladder and the wall. Find the angle made by the ladder with the horizontal.
Ans.
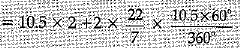
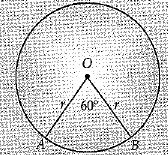
= 21 + 11
= 32 cm
OR
Let the distance between the foot of the ladder and the wall, AB be x.
then AC, the length of the ladder = 2x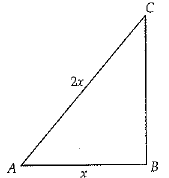

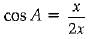
⇒ 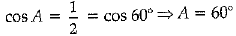
Q.14. In the following AP ’s, fill the missing terms. 53,.........., 23, 8,.......... (1 Mark)
Ans. 38, - 7
[Here, a = 53 and a3 = a + 2d = 23
⇒ 2d = 23 - 53 ⇒ d = - 15
Now, a2 = a + d = 53 - 15 = 38
and a5 = a + 4d = 53 + 4(-15)
= 53 - 60 = - 7]
Q.15. The value of sin 18° - cos 72° is ........
Or
The value of sin θ lies between .......... (1 Mark)
< sin θ < ..........
Ans. (0) As, sin 18° - cos72°
Putting in above equation.
∴ cos72° - cos72° = 0
Or
-1 < sinθ < 1
Q.16. Find the value(s) of x, if the distance between the points 4(0, 0) and B(x, - 4) is 5 units. (1 Mark)
Ans. It is given that distance between the points 4(0, 0) and 5(x, - 4) is 5 units. 45 = 5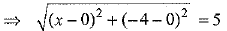
⇒ x2 + 16 = 25
⇒ x2 = 25 - 16
⇒ x2 = 9
⇒ 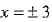
⇒ Hence, the values of x are 3 and - 3.
Q.17. What is the sum of first five positive integers divisible by 6. (1 Mark)
Ans. Here, a = 6, d = 6 and n = 5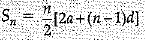
∴ 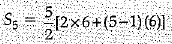
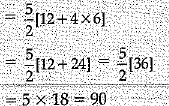
Q.18. In figure, the sides AB, BC and CA of a triangle ABC, touch a circle at P,Q and R respectively.
If PA = 4 cm, BP = 3 cm and AC = 11 cm, then find the length of BC (in cm) (1 Mark)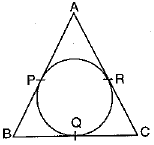
Or
If the radii of two concentric circles are 5 cm and 13 cm, then find the length of the chord of one circle which is tangent to the other circle.
Ans. Since, the tangents drawn from the external point to the circle are equal.
∴ AP = AR = 4 cm
BP = BQ = 3 cm
CQ = CR
CR = AC - AR
= 11 - 4
= 7 cm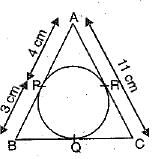
Hence, BC = BQ + CQ = 3 + 7 = 10 cm
Or
Chord AB of larger circle is tangent to smaller circle.
∴ OP is perpendicular bisector of AB.
∴ In right ΔOBP, we have
OB2 = OP2 + BP2
132 = 52 + BP2
⇒ BP2 = 169 - 25
= 144
⇒ BP = 12 cm
Now, AB = 2 x BP
= 2 x 12 = 24 cm
Q.19. For what value of k, 3 is a zero of the polynomial 2x2 1 + x + k? (1 Mark)
Ans. Let f(x) = 2x2 + x + k ... (i)
Given, 3 is a zero of the polynomial f(x). Therefore, put x = 3 in Eq. (i), and consider f(3) = 0
∴ f(3) = 2(3)2 + 3 + k = 0
⇒ 2 x 9 + 3 + k = 0
⇒ 18 + 3 + k = 0
⇒ k = - 21
Q.20. For what value of k, - 4 is a zero of the polynomial x2 - x- (3k + 2) ? (1 Mark)
Ans. The given polynomial is f(x) = x2 - x - (3k + 2)
Since - 4 is a zero of the polynomial f(x) = x2 - x - (3k + 2).
∴ f(-4) = 0
⇒ (- 4)2 - (-4) - (3k + 2) = 0
⇒ 16 + 4 - 3k - 2 = 0
⇒ 18 = 3k
k = 6
Hence, for k = 6, - 4 is a zero of the polynomial x2 - x - (3k + 2)
Section B
Q.21. Using Euclid's algorithm, find the HCF of 240 and 228. (2 Mark)
Ans. Since, 240 = 228 x 1 + 12
and 228 = 12 x 19 + 0
Hence, HCF of 240 and 228 = 12
Q.22. Prove that the centre of a circle touching two intersecting lines lies on the angle bisector of the lines. (2 Mark)
Ans. Let l1 and l2, two intersecting lines, intersect at P, be the tangents from an external point P to a circle with centre O, at Q and R respectively.
Join OQ and OR
Now, in ΔPQO and ΔPRO, we have
PO = PQ [common]
OQ = OR [radii of same circle]
PQ = PR
[tangents from an external point]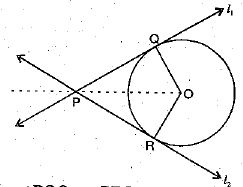
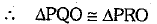
[by SSS congruence rule]
⇒ ∠OPQ = ∠OPR
⇒ O lies on the bisector of the lines l1 and l2.
Q.23. The following data gives the distribution of total household expenditure (in Rs) of manual workers in a city. (2 Mark)
Find the mode from the above data.
Ans. We observe that the class 1500-2000 has the maximum frequency 40.
So, it is the modal class such that
l = 1500, h = 500, f1 = 40, f0 = 24 and f2 = 33 (1/2)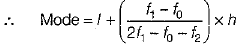
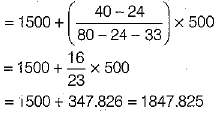
Q.24. A die is thrown once. Find the probability of getting (i) a composite number, (ii) a prime number. (2 Mark)
Ans. When a die is thrown once, the possible outcomes are 1, 2, 3, 4, 5 and 6.
∴ Total number of all possible outcomes = 6
Let A and B be events of getting a composite number and a prime number respectively.
(i) The composite numbers from 1 to 6 are 4 and 6,
∴ Number of cases in favour of event A = 2
Probability of getting a composite number 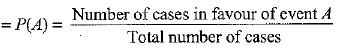
= 2/6 = 1/3.
(ii) Prime numbers are 2, 3 and 5, so number of cases in favour of event B = 3
Probability of getting a prime number 
= 3/6 = 1/2
Q.25.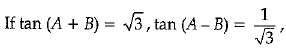 0° < A + B < 90°, A > B, then find A and B.
0° < A + B < 90°, A > B, then find A and B.
Or
Find the number of plates. 1.5 cm in diameter and 0.2 cm thick, that can be fitted completely inside a right circular cylinder of height 10 cm and diameter 4.5 cm. (2 Mark)
Ans.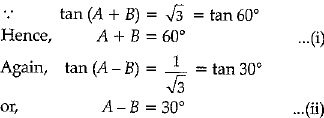
Adding equations (i) and (ii),
2A = 90º
∴ A = 90º/2 = 45
Putting this value of A in equation (i),
B = 60°- A = 60°- 45° = 15°
Hence, A = 45° and B = 15°.
OR
Each one of the circular plate is also a cylinder. Its volume is,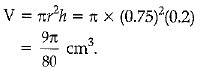
The volume of right circular cylinder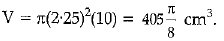
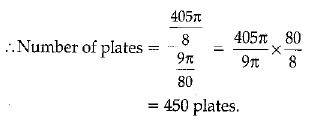
Q.26. A teacher brings clay in the classroom to teach the topic mensuration. He forms cylinder of radius 6 cm and height 8 cm with the clay, then he moulds the cylinder into a sphere. Find the radius and volume of the sphere so formed. [Use π = 3.14] (2 Mark)
Ans. Here, volume of sphere = volume of cylinder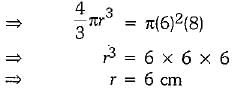
Also, volume of sphere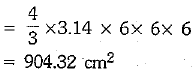
Section C
Q.27. Three different coins are tossed together. Find the probability of getting (3 Mark)
(i) exactly two heads.
(ii) at least two heads
(iii) at least two tails.
Ans. Possible outcomes are
{HHH, HHT, HTH, THH, HTT, THT, TTH, TTT}
⇒ n(S) = 8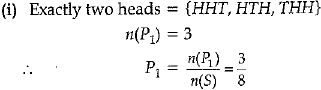
(ii) At least two heads {HHT, HTH, THH, HHH}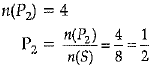
(iii) At least two tails {TTH, THT, HTT, TTT}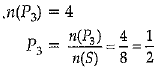
Q.28. A sum of Rs. 700 is to be used to give seven cash prizes to students of a school for their overall academic performance. If each prize is Rs. 20 less than its preceding prize, find the value of each of the prizes. (3 Mark)
Ans. Total amount of seven prizes = Rs. 700
Let the value of first prize be Rs. x
By the given condition, we have
x, x - 20, x - 40, ...
Now, a2 - a1 = x - 20 - x = - 20
a3 - a2 = x - 40 - x + 20 = - 20
Since a2 - a1 = a3 - a2 = - 20, which is constant.
Therefore, it is an A.P. with common difference (d) = - 20
Let S = x + (x - 20) + (x - 40) + ... upto 7 terms
Here, a = x, d = - 20 and n = 7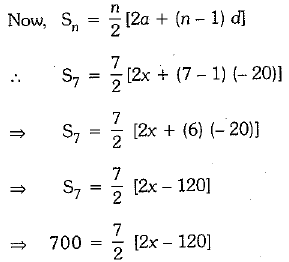
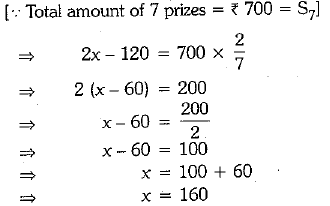
Hence, amount of each prize (in Rs.) respectively is
160, 160 - 20, 160 - 40, 160 - 60, 160 - 80, 160 - 100, 160 - 120 i.e., Rs. 160, Rs. 140, Rs. 120, Rs. 100, Rs. 80, Rs. 60 and Rs. 40.
Q.29. Read the following passage and answer the question that follows:
"The 6th term of an AP is zero. The first term of the AP is a and com m on difference is d. (3 Mark)
(i) Write a relation between ‘a and ‘d’.
(ii) Prove that the 30th term of the AP is three times its 14th term.
Ans. We have, a be the first term and d be the common difference of given AR Then, its general term, i.e. nth term, is given by an - a + (n - 1) d
Clearly, a6 = a + 5d ......(i)
a30 = a + 29d ...........(ii)
and a14 = a + 13d
(i) ∵ a6 = 0
∴ a + 5d = 0
⇒ a = - 5d [using Eq. (i)]
(ii) On substituting a = - 5 d in Eqs, (ii) and (iii), we get
a30 = - 5d + 29d = 24d ...(iv)
and a14 = - 5d + 13d = 8d ...(v)
From Eqs. (iv) and (v), we get
a30 = 3a14 Hence proved.
Q.30. Prove that √3 is an irrational number.
Or
Find the largest number which on dividing 1251,9377 and 15628 leaves remainders 1,2 and 3 respectively. (3 Mark)
Ans. Let us assume, to the contrary, that √3 is rational number.
Then √3 = a/b, where a and b are co -prime i.e., their HCF = 1 and b ≠ 0.
∴ 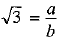 ⇒
⇒ 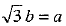
Squaring both sides, we get
3b2 = a2 ... (1)
⇒ a2 is divisible by 3.
⇒ a is divisible by 3. [If r(prime) divides a2, then r divides a]
Let a = 3m, where m is an integer.
Substituting a = 3m in (1) , we get
3b2 = 9m2 ⇒ b2 = 3 m2
This means that b2 is divisible by 3, and so b is also divisible by 3. Therefore, a and b have at least 3 as a common factor. But this contradicts the fact that a and b are co-prime. This contradiction has arisen because of our incorrect assumption that √3 is a rational number.
So, we conclude that √3 is an irrational number.
Or
The required number is the HCF of the numbers
1251 - 1 = 1250, 9377 - 2 = 9375 and 15628 - 3 = 15625
First we find HCF of 1250 and 9375 by Euclid's algorithm as given below :
9375 = 1250 x 7 + 625
1250 = 625 x 2 + 0
625 is the HCF of 1250 and 9375.
Now, we find the HCF of 625 and 15625 by Euclid's algorithm.
15625 = 625 x 25 + 0
Therefore, the HCF of 1250, 9375 and 15625 is 625.
Hence, the largest number that divides 1251, 9377 and 15628 leaving remainders 1, 2 and 3 respectively is 625.
Q.31. If ΔABC ~ ΔPQR and AD and PS are bisectors of corresponding angles A and P, then prove that 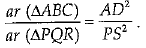
Or
If A(- 5, 7), B(- 4, - 5), C(- 1, - 6) and D(4, 5) are the vertices of a quadrilateral, find the area of the quadrilateral ABCD. (3 Mark)
Ans.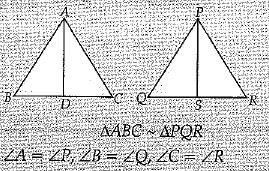
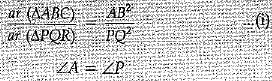


By eqs. (i) and (ii),
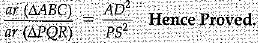
OR
Area of quad. ABGD = arΔABD | arΔBCD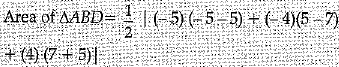
= 53. sq. units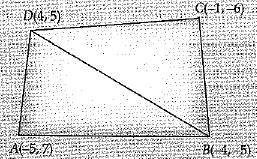
Area of ar ABCD = | (-4) (- 6 - 5) + (-1) (5 + 5) + 4 (- 5 + 6)|
19 sq. units.
Hence, area of quad. ABCD = 53 + 19 = 72 sq. units
Q.32. If tan A = n tan B and sin A = m sin B. (3 Mark)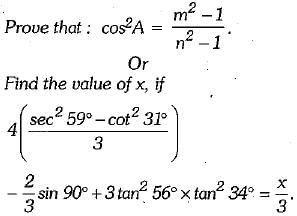
Ans. tan A = n tan B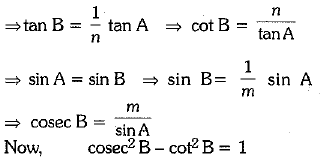
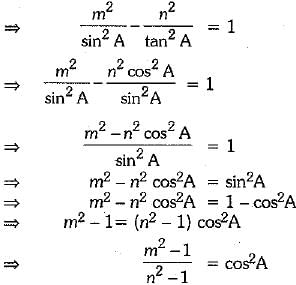
Or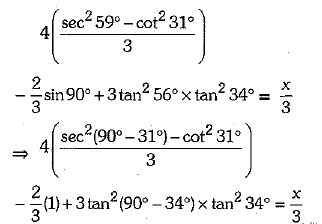
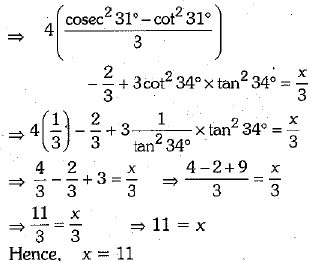
Q.33. Two pillars of equal height are on either sides of a road, which is 100 m wide. The angles of the top of the pillars are 60° and 30° at a point on the road between the pillars. Find the position of the point between the pillars. Also, find the height of each pillar. (3 Mark)
Ans. Let AB and CD be two pillars of equal height h m and distance between them be BD = 100 m.
Let E be a point on the road such that BE = xm,
DE = (100 - x) m, ∠AEB = 60° and ∠CED = 30°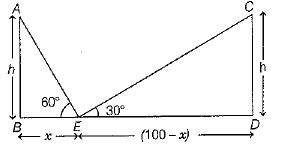
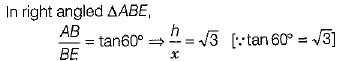

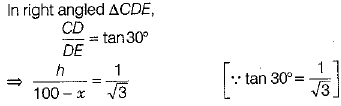
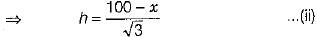
From Eqs. (i) and (ii), we get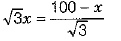
⇒ 3x = 100 - x ⇒ 4x = 100
Q.34. A car has two wipers which do not overlap. Each wiper has a blade of length 21 cm sweeping through an angle 120°. Find the total area cleaned at each sweep of the blades. [Take π = 22/7] (3 Mark)
Ans. Each wiper has a blade of length 21 cm and sweeping through an angle of 120°.
∴ Area cleaned at each sweep of a blade = Area of sector with r - 21 cm and θ = 120°.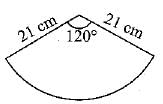
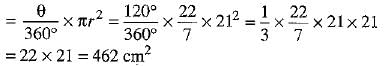
Since there are two wipers, therefore, total area cleaned at each sweep of the blades
= (2 x 462) cm2 = 924 cm2
Section D
Q.35. Solve the following pair of linear equations graphically:
x + 3y = 6 and 2x - 3y = 12.
Also, shade the region bounded by the line 2x - 3y = 12 and both the co-ordinate axes.
OR
The denominator of a fraction is one more than twice its numerator. If the sum of the fraction and its reciprocal is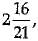 find the fraction. (4 Mark)
find the fraction. (4 Mark)
Ans.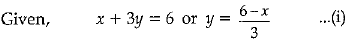
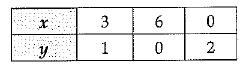
and 2x - 3y = 12 .....(ii)
⇒ 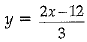

Plotting the above points and drawing lines joining them, we get the graphs of the equations x + 3y = 6 and 2x - 3y = 12.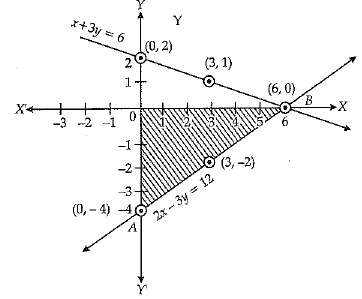
The two lines intersect each other at point B (6, 0).
Hence, x - 6 and y = 0.
Again, ΔOAB is the region bounded by the line 2x - 3y = 12 and both the co-ordinate axes.
OR
Let numerator be x.
Then, die fraction = 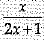
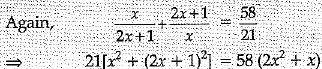
⇒ 11x2 - 26x - 21 = 0
11x2 + 33x - 7x - 21 = 0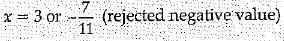
Hence, fraction = 3/7
Q.36. In the given figure, triangle ABC is similar to triangle PQR. AM and PN are altitudes whereas AX and PY are medians. (4 Mark)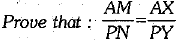
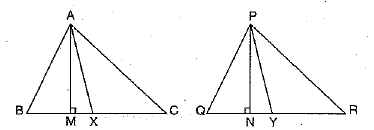
Ans. Since the ratio of the areas of two similar triangles is equal to the ratio of the squares of any two corresponding sides.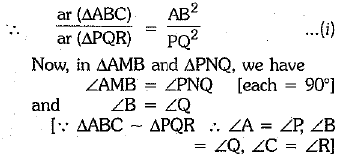
So, by AA criterion of similarity, we have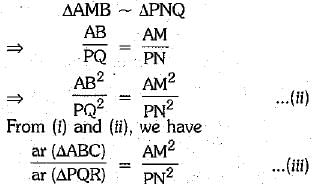
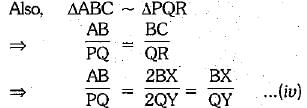
Thus, in ΔAXB and ΔPYQ, we have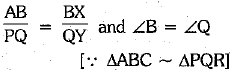
So, by SAS similarity, we have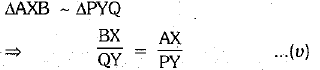
From (iv) and (v), we have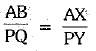
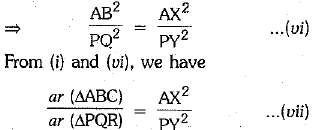
From (iii) and (vii), we have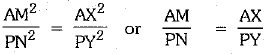
Q.37. Diagonals AC and BD of a trapezium ABCD with AB║DC intersect each other at the point O. Using similarity criterion for two triangles, show that 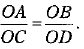 (4 Mark)
(4 Mark)
Ans. Given ABCD is a trapezium in which AB║DC,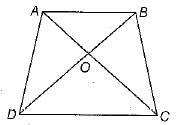
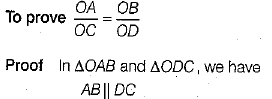
Then, ∠SDAB = ∠OCD [alternate interior angles]
∠AOB = ∠DOC
[vertically opposite angles]
and ∠ABO = ∠CDO [alternate interior angles]
∴ ΔOAB ~ ΔOCD [by AAA similarity criterion]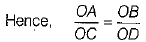
[if two triangles are similar, then their corresponding sides are proportional] Hence proved.
Q.38. In figure, a decorative block is shown which is made of two solids, a cube and a hemisphere. The base of the block is a cube with edge 6 cm and the hemisphere fixed on the top has a diameter of 4.2 cm. Find
(a) the total surface area of the block.
(b) the volume of the block formed. [Take π = 22/7]
Or
A solid iron pole consists of a cylinder of height 220 cm and base diameter 24 cm, which is surmounted by the another cylinder of height 60 cm and radius 8 cm. Find mass of the pole, given that 1 cm3 of iron has approximately 8 g mass. [Use π = 3.14] (4 Mark)
Ans. (a) Side of the block of cube = 6 cm
Total surface area of the cube (S1) = 6 x (side)2
= 6 x (6)2 cm2
= 216 cm2
Diameter of the hemisphere = 4.2 cm
∴ Radius of the hemisphere = 2,1 cm
Area of the base of the hemisphere (S2) = πr2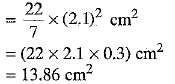
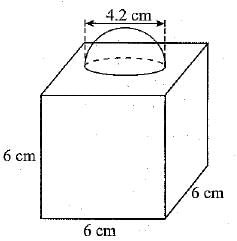
Curved surface area of the hemisphere (S3) = 2πr2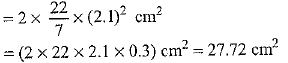
∴ Total surface area of the block (S) = (S1) - (S2) + (S3)
= (216 - 13.86 + 27.72) cm2
= 229.86 cm2
Volume of the cube (V1) = (Side)3 = (6)3 cm3 = 216 cm3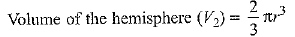
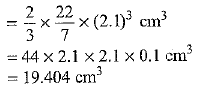
∴ Volume of the block (V) = V1 + V2
= (216 + 19.404) cm3
= 235.404 cm3
Or
Let R and H denote radius of base and height of the bigger cylinder and r and h denote radius of base and height of the smaller cylinder surmounted on bigger cylinder; V be the volume of the solid iron pole.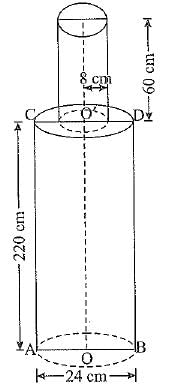
Diameter of bigger cylinder = 24 cm; radius of the bigger cylinder, R - 12 cm and height of the bigger cylinder, H = 220 cm and radius of the smaller cylinder, r = 8 cm and height of the smaller cylinder, h = 60 cm.
Volume of the solid iron pole formed of two cylinders,
V = πR2H + πr2h
⇒ V = π(122 x 220 + 82 x 60) cm3
⇒ V = π(144 x 220 + 64 x 60) cm3
⇒ V = π(31680 + 3840) cm3
⇒ V = 35520π cm3
= 35520 x 3.14 cm3
= 111632.8 cm3
It is given that mass of 1 cm3 of iron is 8 g.
Q.39. A moving boat observed from the top of a 150 m high cliff, moving away from the cliff. The angle of depression of the boat changes from 60° to 45° in 2 minutes. Find the speed of the boat. (4 Mark)
Ans.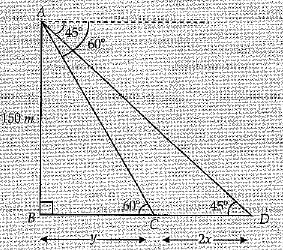
Let the speed of the boat be x m/min.
∴ Distance covered in 2 minutes = 2x
∴ CD = 2x
Let BC be y m.
In ΔABC, AB/BC = tan 60º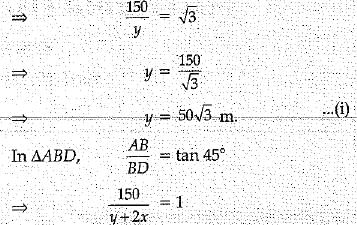
⇒ y + 2x = 150 ...(ii)
Substituting the value of y from (i) in (ii),
Speed of the boat = 25(3-√3) m/min.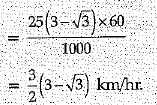
Q.40. Find the mean, mode and median for the following data : (4 Mark)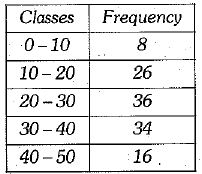
Ans.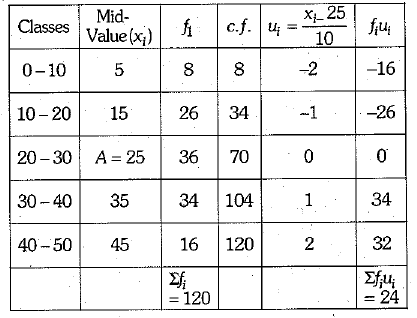
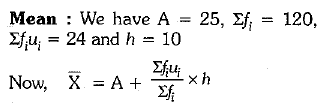
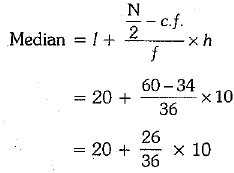
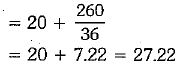
Mode : We have Modal class = 22 - 30
[∵ Max. frequency 36 belongs to 20 - 30]
l = 20, h = 10, f1 = 36, f0 = 26 and f2 = 34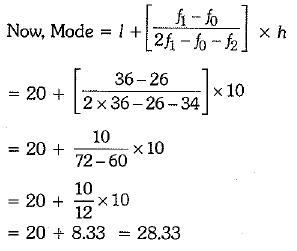
|
127 videos|584 docs|79 tests
|
FAQs on Class 10 Mathematics: CBSE Sample Question Paper (2019-20) - 9 - Mathematics (Maths) Class 10
| 1. What is the format of the CBSE Sample Question Paper for Class 10 Mathematics? |  |
| 2. How many marks are allotted to each section in the CBSE Sample Question Paper for Class 10 Mathematics? |  |
| 3. Can I expect questions from previous years' question papers in the CBSE Sample Question Paper for Class 10 Mathematics? |  |
| 4. Is it necessary to solve the CBSE Sample Question Paper for Class 10 Mathematics before the actual exam? |  |
| 5. Where can I find the CBSE Sample Question Paper for Class 10 Mathematics (2019-20)? |  |
















