Class 10 Mathematics: CBSE Sample Question Paper (2019-20) - 7 | Mathematics (Maths) Class 10 PDF Download
Class X
Mathematics – Standard (041)
Sample Question Paper 2019-20
Max. Marks: 80
Duration : 3 hrs
General Instructions:
(i) All the questions are compulsory.
(ii) The question paper consists of 40 questions divided into 4 sections A, B, C, and D.
(iii) Section A comprises of 20 questions of 1 mark each. Section B comprises of 6 questions of 2 marks each. Section C comprises of 8 questions of 3 marks each. Section D comprises of 6 questions of 4 marks each.
(iv) There is no overall choice. However, an internal choice has been provided in two questions of 1 mark each, two questions of 2 marks each, three questions of 3 marks each, and three questions of 4 marks each. You have to attempt only one of the alternatives in all such questions.
(v) Use of calculators is not permitted.
Section A
Q 1. A number is selected at random from the numbers 1 to 30. The probability that it is a prime number is : (1 Mark)
(a) 2/3
(b) 1/6
(c) 1/3
(d) 11/30
Ans: (c)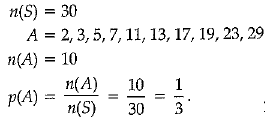
Q 2. If the least prime factor of ‘a’ is 3, the least prime factor of ‘b’ is 7, then the least prime factor of (a + b) is : (1 Mark)
(a) 2
(b) 3
(c) 5
(d) 11
Ans: (a) 2
[Here, least prime factor of 'a’ is 3.
The other factors are more than or equal to 3, but not divisible by 2.
∴ a must be an odd number
Similarly, b is also an odd number.
∴ (a + b) is an even number.
⇒ Least prime factor of (a + b) is 2]
Q 3. If the lines given by 4x + ky = X and 6x - 10y = 14 has unique solution, then value of k is (1 Mark)
(a) -10/3
(b) -20/3
(c) -25/3
(d) -15/4
Ans: (b) The given equations can be rewritten as 4x + ky - 1 = 0 and 6x - 10y - 14 = 0
On comparing with a1x + b1y+C1 = 0 and a2x + b2y+c2 = 0, we get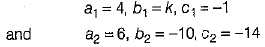
For unique solution,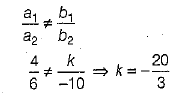
Thus, given lines have a unique solution for all real values of k, except -20/3
Q 4. The coordinates of point P lying on v-axis which is equidistant from the points A(-2, 0) and 5(6, 0) are (1 Mark)
(a) (4, 0)
(b) (2, 0)
(c) (-8, 0)
(d) (-2, 0)
Ans: (b)
The given points are A(- 2, 0) and 5(6, 0).
Let the required point lying on x-axis be P(x, 0).
Since point P is equidistant from A and B.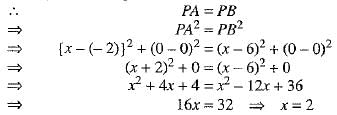
Hence, the required point is P(2, 0).
Q 5. From a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm. The radius of the circle is : (1 Mark)
(a) 7 cm
(b) 12 cm
(c) 15 cm
(d) 24.5 cm
OR
If in triangles ABC and DEF, 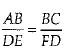 then they will be similar , when:
then they will be similar , when:
(a) ∠B = ∠E
(b) ∠A = ∠D
(c) ∠B =∠D
(d) ∠A = ∠F
Ans: (a)
Let O be the centre of the circle.
Given that, OQ — 25 cm and PQ = 24 cm
We know that the radius is perpendicular to the tangent at the point of contact.
∴ OP ⊥ PQ
In ΔOPQ, we have By Pythagoras theorem,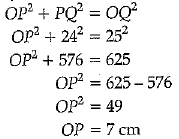
Therefore, the radius of the circle is 7 cm.
OR
(c) In AABC and ADEF, we have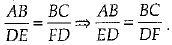
To be similar of ΔABC and ΔDEF, we must have ∠B - ∠D.
Q 6. sin (45° + θ) - cos (45° - θ) is equal to : (1 Mark)
(a) 2 cos 0
(b) 0
(c) 2 sin θ
(d) 1
Ans: (b) 0
[sin (45° + θ) - cos (45° - θ)
= sin (45° + θ) - sin (90° - (45° - θ))
= sin (45° + θ) - sin (90° - 45° + θ)
= sin (45° + θ) - sin (45° + θ) = θ]
Q 7. Sides of two similar triangles are in the ratios 5:7. Areas of these triangles are in the ratio (1 Mark)
(a) 25 : 49
(b) 125 : 49
(c) 49 : 25
(d) 343 : 125
Ans: (a)
Since, the ratio of the areas of two similar triangles is equal to the squares of the ratio of any two corresponding sides.
The ratio of the areas of two similar triangles, whose sides are in the ratio
5 :7 is 52:72 i.e. 25:49
Q 8. The value of cos 80° cos 10° - sin 80° sin 10° = ___________ . (1 Mark)
Ans: θ
We have, cos 80° cos 10° - sin 80° sin 10°
= cos (90° - 10°) cos 10° - sin (90° - 10°) sin 10°
= sin 10° cos 10° - cos 10° sin 10° [∴ sin (90°-θ )= cos θ; cos (90°-θ ) - sin θ] = θ
Q 9. Graphically, the pair of equations (1 Mark)
6x - 3y + 10 = 0
2x - y + 9 = 0
represents two lines which are
(a) intersecting at exactly one point
(b) intersecting at exactly two points
(c) coincident
(d) parallel
Ans: (d)
Here,
So, the system of linear equations is inconsistent (no solution) and graph will be a pair of parallel lines.
Q 10. If the centroid of the triangle formed bp the points (3, - 5), (- 7, 4), (10, - k) is at the point (k, - 1), then k = (1 Mark)
(a) 3
(b) 1
(c) 2
(d) 4
Ans: (c)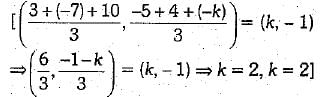
Q 11. A sector is cut from a circle of diameter 21 cm. If the angle of the sector is 150°, then its area is _____ . (1 Mark)
Ans: = 144.38cm2
Given, radius of the cirle, r = 21/2 cm
and angle of sector, θ = 1500
∴ Area of the sector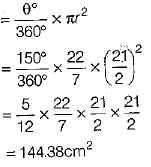
Q 12. If the radii of the bases of a cylinder and cone are in the ratio 3 : 4 and their heights are in the ratio 2 : 3 then the ratio of their volumes is 8 : 9. (1 Mark)
Ans: False.
Let r1, r2 and h1, h2 be radii and heights of cylinder and cone respectively.
∴ 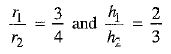
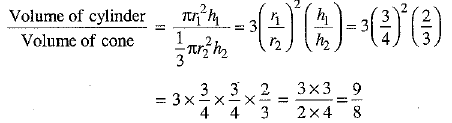
Hence, Volume of cylinder : Volume of cone = 9:8.
Q 13. Two lines are given to be parallel. The equation of one of the lines is 4x + 3y = 14, then find the equation of a second line. (1 Mark)
Ans:
The equation of one line is 4x + 3y — 14. We know that if two lines a1x + b1y + c1 = 0 and a2x + b2y + c2 = 0 are parallel, then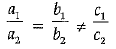
or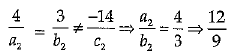
Hence, one of the possible, second parallel line is 12x + 9y = 5.
Q 14. For an AP, a4 + a8 - 24 and a6 + a10 = 34, then a =_________. (1 Mark)
Ans: - 1/2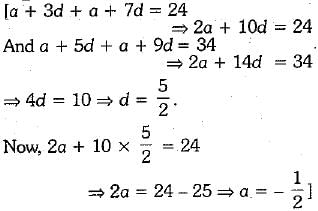
Q 15. ΔABC and ΔBDE are two equilateral triangles such that D is the mid-point of BC. The ratio of th areas of ΔABC and ΔBDE is ______. (1 Mark)
Ans: 4/1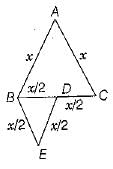
Since, ΔABC and ΔBDE are two equilateral triangles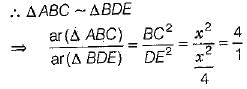
Q 16. Assertion (A) : If x - a cos θ, y = a sin θ, then x2 + y2 = a2
Reason (R) : cos2 θ + sin2 θ=1 (1 Mark)
(a) Both A and R are true and R is the correct explanation for A.
(b) Both A and R are true and R is not the correct explanation for A.
(c) A is true but R is false.
(d) A is false but R is true.
Ans: (a)
We have,
x2 + y2 - (a cos θ)2 + (a sin θ)2 = a2 cos2 θ + a1 sin2 θ
= a2 (cos2 θ + sin2 θ)
= a2(1)
= a2
Q 17. Which of the following equations has the sum of its roots as 3? (1 Mark)
(a) 2x2 - 3x + 6 = 0
(b) - x3 + 3x - 3 = 0
(c) 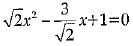
(d) 3x2 - 3x + 3 = 0
Ans:
-x2 + 3x - 3 = 0
On comparing with ax2 +bx +c =
a = -1, b= 3, c = - 3
∴ sum of roots 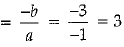
Q 18. If the points A(4, 3) and B(x, 5) are on the circle with centre O( 2 , 3 ) , find the value of x.
OR
If two tangents inclined at an angle 60° are drawn to a c irc le of radius 3 cm , then find the length of each tangent. (1 Mark)
Ans. Here, |OA| = |OB|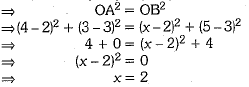
Or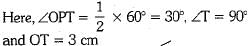
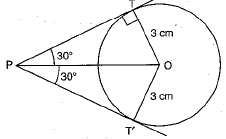
Now, in rt. ∠edΔPTO, we have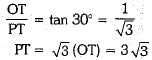
Hence, the length of each tangent is 3√3 cm.
Q.19. If the perimeter of a semi-circular protractor is 72 cm. Its radius is (1 Mark)
Ans. Let the radius of the protractor be r cm.
Then, perimeter of protractor = (πr + 2r) = 72
⇒ (π + 2)r = 72
⇒ 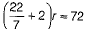
⇒ 36/7 r = 72
⇒ 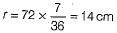
Q.20. Is the equation √2x - 6x + 1 = 0 a quadratic? (1 Mark)
Ans. The given equation is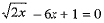
⇒ 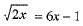
Squaring both sides, we get
2x = (6x-1)2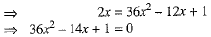
which is a quadratic equation.
SECTION B
Q.21. Find the value of k, if -1 is a zero of the polynomial p(x) = kx2 - 4x + k.
OR
The incomes of two persons A and B are in the ratio 8 : 7 and the ratio of their expenditures is 19 : 16 If their savings are ₹ 2550 per month, find their monthly income. (2 Mark)
Ans. Since, - 1 is a zero of the polynomial
and p(x) = kx2 - 4x + k,
then p (-1) = 0
∴ k (-1)2 - 4 (-1) + k = 0.
⇒ k + 4 + k = 0
⇒ 2k + 4 = 0
⇒ 2k = - 4
Hence, k = -2
OR
Let income of A = 8x and income of B = 7x.
Also their expenditures be 19y and 16y.
⇒ 8x - 19y = 2550 ...(i)
and 7x - 16y = 2550 ...(ii)
Solving the equations
x = 1530 and y = 510
∴ Salary of A = 12240
Salary of B = 10710
Q.22. In two concentric circles, prove that all chords of the outer circle which touch the inner are of equal length. (2 Mark)
Ans. Let AB and CD be two chords of the circle which touch the inner circle in M and N respectively. Since AB and CD are tangents to the smaller circle.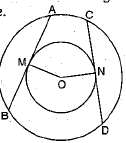
∴ OM = ON = radius of smaller circle. Thus, AB and CD are two chords of the larger circle such that they are equidistant from the centre.
Hence, AB = CD.
Q.23. The Class X students of a secondary school in Krishinagar have been alloted a rectangular plot of land for this gardening activity. (2 Mark)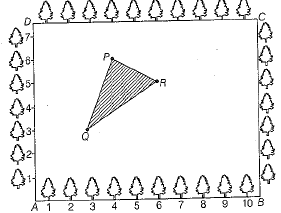
Sapling of Gulmohor are planted on tf boundary at a distance of 1 m from eac other. There is a triangular gracy lawn in the plot as shown in above figure.
The students are to sow seeds of flowerin plants on the remaining area of the plot.
Then, taking A as origin, find the area of the triangle in this case.
Ans. When A is taken as origin, AD and AB as coordinate axes. i.e. X and Y-axes, respectively. Hence coordinates of P, Q and R are respectively, (4, 6), (3, 2) and (6, 5).
In this case, AD and AB taken as coordinate axes.
Then, area of ΔPQR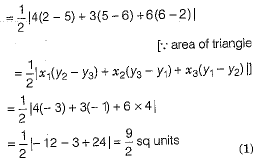
Q.24. Find the value(s) of k so that the pair of equations x + 2y = 5 and 3x + ky + 15 = 0 has a unique solution. (2 Mark)
Ans. We know that the linear equations a1x + b1y + c1 = 0 and a2x + b2y + c2 = 0 has a unique solution if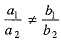
The given system of linear equations is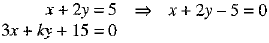
This system of equations is of the form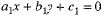
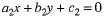
where, 
The given system of linear equations will have a unique solution if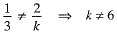
Hence, the given system has a unique solution if k ≠ 6.
Q.25. Prove that: 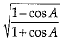 = cosec A - cot A (2 Mark)
= cosec A - cot A (2 Mark)
Ans.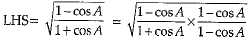
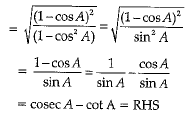
Q.26. Onkar and his sisier Niharika playing chess. There are 64 equal squares and the area of each square is 6.25 cm2. A border round the board is 2 cm wide. Find the length of the side of the chess board. (2 Mark)
Ans. Let the length of the side of the chess board be x cm.
∴ Area of 64 squares = (x-4)2
64 x 6.25 = x2 - 8x +16
400 =x2 - 8x + 16
SECTION C
Q.27. In a single throw of a pair of different dice, what is the probability of getting (i) a prime number on each dice? (ii) a total of 9 or 11? (3 Mark)
Ans. (i) Favourable outcomes are (2, 2) (2, 3) (2, 5) (3, 2) (3, 3) (3, 5) (5, 2) (5, 3) (5, 5) ie. 9 outcomes.
P (a prime number on each die) = 9/36 or 1/4
(ii) Favourable outcomes are (3, 6) (4, 5) (5, 4) (6, 3) (5, 6) (6, 5) i.e. 6 outcomes
P (a total of 9 or 11) = 6/36 or 1/6
Q.28. If the sum of first m terms of an AP is n and the sum of first n terms is m, then show that the sum of its first (m + n) terms is-(m +n). (3 Mark)
Ans. Let a be the first term and d be the common difference
∴ Sm = n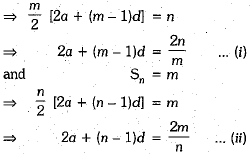
Subtracting (ii) from (i), we have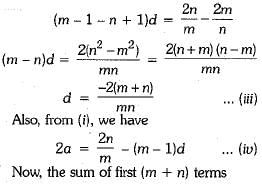

Q.29. Show that the points (12, 8), (-2, 6) and (6, 0) are the vertices of a isosceles right angled triangle. (3 Mark)
Ans. Let P (12, 8), Q (-2, 6) and R (6, 0) be the given points.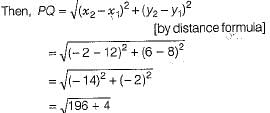
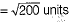
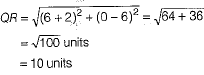
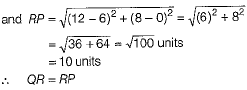
So, ΔPQR is isosceles.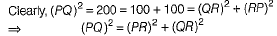
Thus, ΔPQR is a right angled triangle, right angled at R. [by convers of Pythogoras theorem]
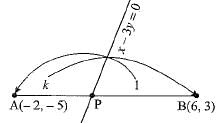
Q.30. Find the ratio in which the line x - 3y = 0 divides the line segment joining the points (- 2, - 5) and (6, 3). Find the coordinates of the point of intersection. (3 Mark)
Ans. Let the given points be A(- 2, - 5) and 5(6, 3).
The equation of the given line is x - 3y = 0 ...(1)
Let given line divides the join of A and B in the ratio k : 1 at P(x, y).
Using section formula, the coordinates of point P are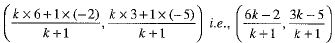
But the point P lies on the line (1), so its coordinates satisfy equation (1).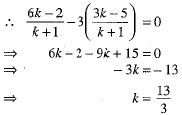
∴ 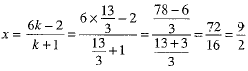
and 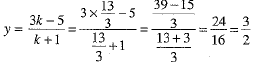
and ratio is k : 1 i.e., 13/3 : 1 i.e., 13 : 3.
Hence, the given line divides the join of pooints A(-2, -5) and B(6, 3) in the ratio 13 : 3 and point of intersection is 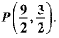
Q.31. Two tangents TP and TQ are drawn to a circle with centre O from an external point T. Prove that ∠PTO = 2∠OPQ
OR
The perpendicular AD on the base BC of a ΔABC intersects BC at D so that DB = 3CD. Prove that 2(AB)2 = 2(AC)2 + BC2. (3 Mark)
Ans. 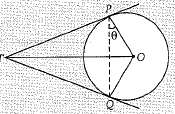
Let ∠OPQ be θ.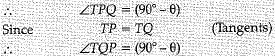
(Opposite angles of equal sides)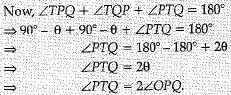
OR
Given, in ΔADB,AB2 = AD2 + BD2 ...(i) (Pythagoras Theorem)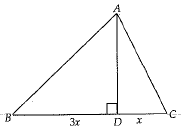
In ΔADC, AC2 = AD2 + CD2 ...(ii) (Pythagoras theorem)
Subtracting eqn. (ii) from eqn. (i),
AB2 - AC2 = BD2 - CD2
Or, 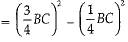
or, 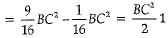
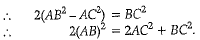
Q.32. If x = sec A + sin A and y = sec A - sin A, prove that 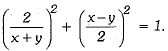
Or
If tan2θ = 1 - a2, prove that secθ + tan3θ cosecθ = (2 - a2)3/2. (3 Mark)
Ans. Here, x = sec A + sin A ...(i)
y = sec A - sin A ...(ii)
Adding (i) and (ii), we obtain
x + y = 2 sec A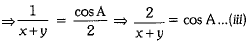
Subtracting (ii) from (i), we obtain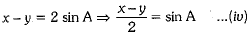
Squaring and adding (iii) and (iv), we obtain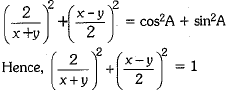
Or
Here, we have tan2θ = 1 - a2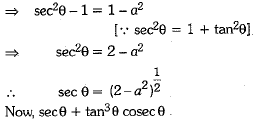
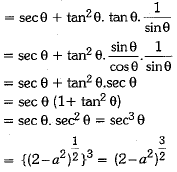
Q.33. On a square handkerchief, 9 circular designs each of radius 7 cm are made (see the figure). Find the area of the remaining portion of the handkerchief. (3 Mark)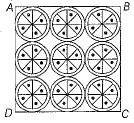
Or
A car has wheels which are 80 cm in diameter. How many complete revolutions does each wheel make in 10 min when the car is travelling at a speed of 66 km/h?
Ans. Given, radius of each circle r = 7 cm
∴ Diameter of each circle = 7 x 2 = 14 cm
Clearly, length of side of the square
= 3 x ( Diameter of a circle) = 3 x 14 = 42 cm
∴ Area of the square = (Side)2 = (42)2 = 1764 cm2
Now, area of one circle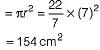
So, area of 9 circles = 9 x 154 = 1386 cm2
∴ Area of remaining portion
= Area of the square - Area of 9 circles
= 1764 - 1386 = 378 cm2
Hence, the area of the remaining portion is 378 cm2.
Or
Given, diameter of a wheel = 80 cm
Clearly, radius of car wheel = 40 cm
Now, distance covered by the car when its wheel take one complete revolution = circumference of wheel 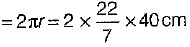
∵ Speed of car = 66 km/h
∴ Distance covered by the car in 1 h i.e. 60 min
= 66 km
⇒ Distance covered by the car in 10 min
= 11 x 1000 m [∵ 1 km = 1000 m]
= 11 x 1000 x 100 cm [∵ 1 m = 100 cm]
Clearly, number of revolutions made by the wheels in 10 min
= Distance covered by the car in 10 min/Distance covered1 by the car when its wheel take one complete revolution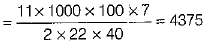
Hence, each wheel makes 4375 revolutions in 10 min.
Q.34. For what value of k, is the polynomial
f(x) = 3x4 - 9x3 + x2 + 15x + k
completely divisible by 3x2 - 5?
OR
Find the zeroes of the quadratic polynomial 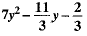 and verify the relationship between the zeroes and the coefficients. (3 Mark)
and verify the relationship between the zeroes and the coefficients. (3 Mark)
Ans. The given polynomial is
f(x) = 3x4 - 9x3 + x2 + 15x + k
and g(x) = 3x2 - 5
Dividing f(x) = 3x4 - 9x3 + x2 + 15x + k by g(x) = 3x2 - 5 using long division, we get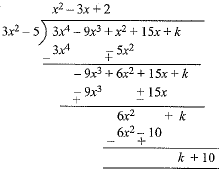
Since, f(x) is divisible by g(x), remainder must be zero.
∴ k + 10 = 0
⇒ k = -10
Or
The given polynomial is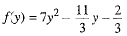
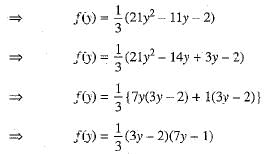
Therefore, the value of f (y)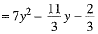 is zero when 3y - 2 = 0 or 7y + 1 = 0 i.e., when y = 2/3 or y = -1/7.
is zero when 3y - 2 = 0 or 7y + 1 = 0 i.e., when y = 2/3 or y = -1/7.
∴ The zeroes of the given polynomial are 2/3 and -1/7.
Sum of zeroes 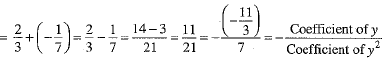
and Product of zeroes = 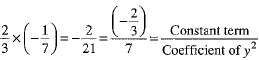
SECTION D
Q.35. Draw the graphs of the pair of linear equations : x + 2y = 5 and 2x - 3y = -4.
Also, find the points where the lines meet the x-axis.
OR
Sum of the areas of two squares is 400 cm2. If the difference of their perimeters is 16 cm, find the sides of the two squares. (4 Mark)
Ans. Given, x + 2y = 5
⇒ 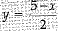
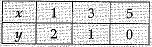
and 2x - 3y = - 4
⇒ 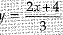
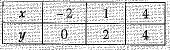
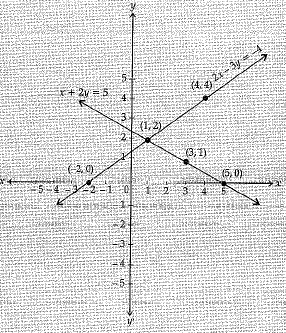
Thus, the lines meet x-axis at (5, 0) and (-2, 0) respectively.
OR
Let the sides of two squares be a and b,
then a2 + b2 = 400 ...(i)
and 4 (a - b) =16
⇒ a - b = 4
⇒ a = 4 + b
From equations (i) and (ii), we get
(4 + b)2 + b2 = 400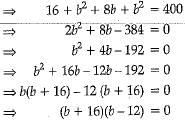
b = - 16 or, b = 12
(Rejecting the negative value)
So, b = 12 cm
and a = 16cm.
Q.36. In a triangle, if square of one side is equal to sum of squares of other two sides, then prove that the angle opposite to the first side is right angle. (4 Mark)
Ans. ΔABC such that AB2 + BC2 = AC2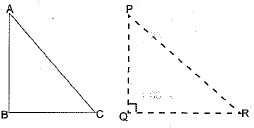
To Prove: ∠B = 90°
Const. : Construct a right triangle PQR, right-angled at Q, such that
PQ = AB
and QR = BC.
Proof : From right ΔPQR, we have
PR2 = PQ2 + QR2
[using Pythagoras Theorem]
⇒ PR2 = AB2 + BC2 ...(i)
[∵ PQ = AB and QR = BC]
Also, we have
AC2 = AB2 + BC2 ... (ii) [given]
From (i) and (ii), we have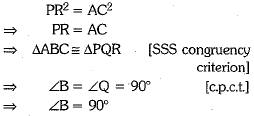
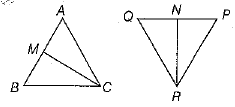
Q.37. In the following figure, CM and RN are respectively the medians of ΔABC and 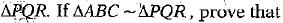 (4 Mark)
(4 Mark)
(i) ΔAMC - ΔPNR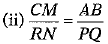
Ans. ΔABC and ΔPQR
CM is the median of ΔABC and RN is the median of ΔPQR.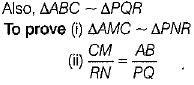
Proof
(i) Given ΔABC ~ ΔPQR
∴ 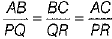 ...(i)
...(i)
[∴ in similar triangle, corresponding sides are proportional]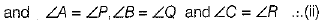
[∴ in similar triangles, corresponding angles are equal]
We know that the median bisects the opposite side.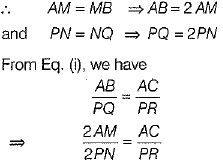
⇒ 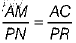 ...(iii)
...(iii)
In ΔAMC and ΔPNR, we have
∠A = ∠P [from Eq. (ii)]
and 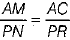 [from Eq. (iii)]
[from Eq. (iii)]
So, ΔAMC ~ ΔPNR
[by SAS similarity criterion]
(ii) We have, ΔAMC ~ ΔPNR
⇒ 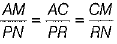
[∵ triangles are similar, so corresponding sides will be proportional]
∴ 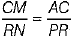
⇒ 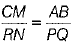 [from Eq. (i)]
[from Eq. (i)]
Q.38. Prove that: (4 Mark)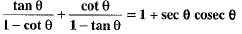
Or
Prove that:
Ans. We have,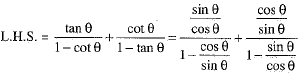
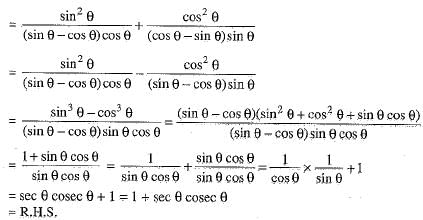
OR
We want to prove that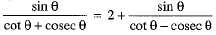
Now, 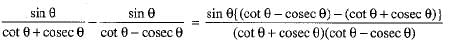
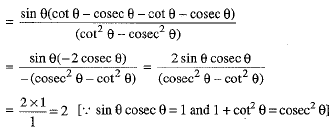
∴ 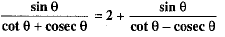
Q.39. Find the area of the shaded region in figure,  are semi-circles of diameter 14 cm, 3.5 cm, 7 cm and 3.5 cm respectively.
are semi-circles of diameter 14 cm, 3.5 cm, 7 cm and 3.5 cm respectively.  (4 Mark)
(4 Mark)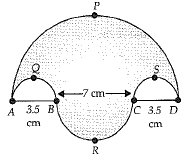
Or
The internal and external diameters of a hollow hemispherical vessel are 16 cm and 12 cm respectively. If the cost of painting 1 cm2 of the surface area is ₹ 5.00, find the total cost of painting the vessel all over. (Use π = 3.14)
Ans. Area of shaded Region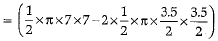
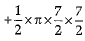
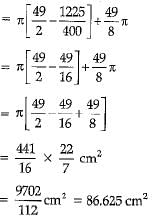
Or
R = 8 cm, r = 6 cm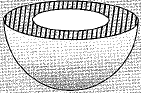
Total Surface area = 2πR2 + 2πr2 + π(R2 - r2)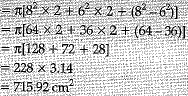
∴ Total cost= 715.92 x ₹5 = ₹ 3579*60.
Q.40. The mean of the following distribution is 132 and the sum of the frequencies is 50. Find the missing frequencies f1 and f2. (4 Mark)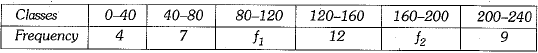
Ans. 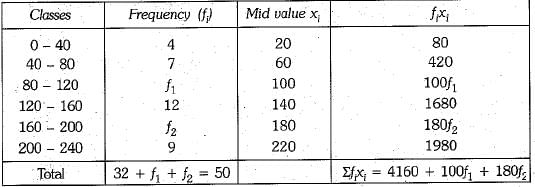
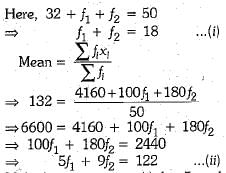
Multiplying equation (i) by 5 and subtracting from (ii), we have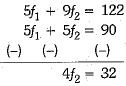
⇒ f2 = 8
From (i), we have
f1 + f2 = 18
⇒ f1 + 8 = 18
⇒ f1 = 10
Hence, missing frequencies are 10 and 8.
|
127 videos|584 docs|79 tests
|
FAQs on Class 10 Mathematics: CBSE Sample Question Paper (2019-20) - 7 - Mathematics (Maths) Class 10
| 1. What is the purpose of CBSE sample question papers? |  |
| 2. How can CBSE sample question papers be helpful in exam preparation? |  |
| 3. Are the CBSE sample question papers similar to the actual board exam papers? |  |
| 4. Can solving CBSE sample question papers guarantee success in the board exams? |  |
| 5. Where can I find CBSE sample question papers for Class 10 Mathematics? |  |





















