JEE Advanced (Subjective Type Questions): Gravitation | Chapter-wise Tests for JEE Main & Advanced PDF Download

Q.1. Two satellites S₁ and S₂ revolve round a planet in coplanar circular orbits in the same sense. Their periods of revolution are 1 hour and 8 hours respectively. The radius of the orbit of S₁ is 10⁴ km. When S2 is closest to S₁, find
(i) the speed of S₂ relative to S₁,
(ii) the angular speed of S₂ as actually observed by an astronaut in S₁.

Ans. (i) -π ×10⁴ km/hr (ii)  3 ×  10^-4 rad/s

Soltuion. (i) According to Kepler's third law

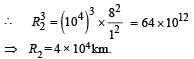
Linear speed of satellite S₁

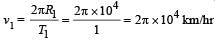
Linear speed of satellite S₂,

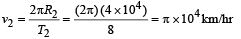
The speed of satellite S₂ w.r.t. S₁ 

= v₂ – v₁ = π × 10⁴ – 2π × 10⁴ = – π × 10⁴ km/hr

(ii) Angular speed of S₂ w.r.t. S₁

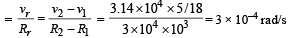
Q.2. Three particles, each of mass m, are situated at the vertices of an equilateral triangle of side length a. The only forces acting on the particles are their mutual gravitational forces.

It is desired that each particle moves in a circle while maintaining the original mutual separation a. Find the intial velocity that should be given to each particle and also the time period of the circular motion. (1988 - 5 Marks)

Ans. 

Solution. The radius of the circle 

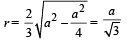
Let v be the velocity given. The centripetal force is provided by the resultant gravitational attraction of the two masses.

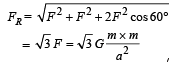
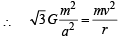
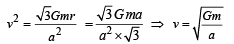
Time period of circular motion

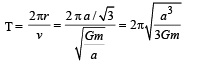
Q.3. An artificial satellite is moving in a circular orbit around the earth with a speed equal to half the magnitude of escape velocity from the earth.                (1990 - 8 Mark) 

(i) Determine the height of the satellite above the earth’s surface. 
(ii) If the satellite is stopped suddenly in its orbit and allowed to fall freely onto the earth, find the speed with which it hits the surface of the earth.

Ans. (i)  6400 km   (ii) 7.92 km/s

Solution. (i) KEY CONCEPT :  Since the satellite is revolving in a circular orbit, the centripetal force is provided by the gravitational pull.

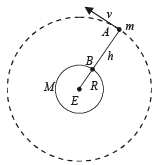
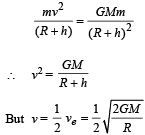
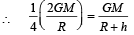
⇒ 2R + 2h = 4R ⇒ h = R = 6400 km. 

(ii) KEY CONCEPT :  When the satellite is stopped, its kinetic energy is zero. When it falls freely on the Earth, its potential energy decreases and converts into kinetic energy.

∴ (P.E.)_A – (P.E.)_B = K.E.

Q.4. Distance between the centres of two stars is 10a. The masses of these stars are M and 16M and their radii a and 2a, respectively. A body of mass m is fired straight from the surface of the larger star towards the smaller star. What should be its minimum initial speed to reach the surface of the smaller star? Obtain the expression in terms of G, M and a.       (1996 - 5 Marks)

Ans. 

Solution.

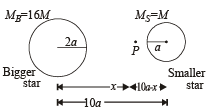
The force of attraction is zero at say x from the bigger star.

Then force on mass m due to bigger star = Force on mass m due to small star

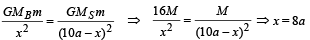
If we throw a mass m from bigger star giving it such a velocity that is sufficient to bring it to P, then later on due to greater force by the star M_S it will pull it towards itself [without any external energy thereafter].

The energy of the system (of these masses) initially = Final energy when m is at P

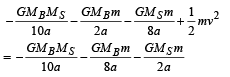
[∴ M_B = 16M ; M_S =M]

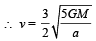
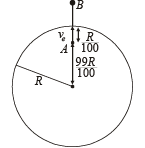
Q.5. A body is projected vertically upwards from the bottom of a crater of moon of depth R/100 where R is the radius of moonwith a velocity equal to the escape velocity on the surface of moon. Calculate maximum height attained by the body from the surface of the moon.       (2003 - 4 Marks)

Ans. 99.5 R

Solution. Total energy at A = Total energy at B 

(K.E.)_A + (P.E.)_A = (P.E.)_B

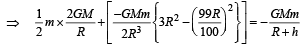
On solving we get h = 99.5 R.