JEE Advanced (Matrix Match & Integer Answer): Heat & Thermodynamics | Chapter-wise Tests for JEE Main & Advanced PDF Download
Each question contains statements given in two columns, which have to be matched. The statements in Column-I are labelled A, B, C and D, while the statements in ColumnII are labelled p, q, r and s. Any given statement in Column-I can have correct matching with ONE OR MORE statement(s) in Column-II. The appropriate bubbles corresponding to the answers to these questions have to be darkened as illustrated in the following example : If the correct matches are A-p, s and t; B-q and r; C-p and q; and D-s then the correct darkening of bubbles will look like the given.

Q.1.Heat given to process is positive, match the following option of Column I with the corresponding option of column II :
| (A) JK | (p) vW > 0 |
| (B) KL | (q) ΔQ < 0 |
| (C) LM | (r) ΔW < 0 |
| (D) MJ | (s) ΔQ > 0 |
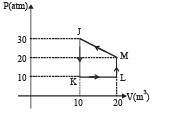
Ans.(A)-(q); (B)-(p, s); (C)-(s); (D)-(q, r)
Solution.
(A) → (q) : JK is a isovolumic process. Therefore work done is zero. But there is decrease in pressure. Now ΔQ =ΔU +ΔW. Therefore ΔQ = ΔU. In this case ΔU = nCvΔT and P µT.
Since pressure has decreased means temperature has decreased. Therefore ΔU is negative and so is ΔQ.
(B) → (p, s) : KL is a isobaric process. Pressure is constant.
The volume is increasing therefore ΔW > 0. Also there is an increase in temperature. For both the case heat is absorbed.
Therefore ΔQ > 0.
(C) → (s) : LM is a isovolumic process. Therefore work done is zero. The process is accompanied by increases in pressure. In this case, the temperature has increased and therefore ΔU > 0. Therefore ΔQ > 0.
(D)→ (q, r) : The process MJ is accompained with decrease in volume. Therefore ΔW < 0. Also from the graph we can conclude that the temperature in the process decreases.
Therefore DU is also negative ⇒ ΔQ < 0.
Each question contains statements given in two columns, which have to be matched. The statements in Column-I are labelled A, B, C and D, while the statements in ColumnII are labelled p, q, r and s. Any given statement in Column-I can have correct matching with ONE OR MORE statement(s) in Column-II. The appropriate bubbles corresponding to the answers to these questions have to be darkened as illustrated in the following example : If the correct matches are A-p, s and t; B-q and r; C-p and q; and D-s then the correct darkening of bubbles will look like the given.

Q.2. Column I contains a list of processes involving expansion of an ideal gas. Match this with Column II describing the thermodynamic change during this process. Indicate your answer by darkening the appropriate bubbles of the 4 × 4 matrix given in the ORS.
| Column I | Column II |
An insulated container has two chambers separated by a valve. Chamber I contains an ideal gas and the Chamber II has vacuum. The valve is opened. 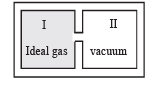 | (p) The temperature of the gas decreases |
| An ideal monoatomic gas expands to twice its remains original volume such that its pressure P ∝ 1/V2 where V is the volume of the gas | q) The temperature of the gas increases or constant |
| An ideal monoatomic gas expands to twice its original volume such that its pressure P ∝ 1/V4/3 | (r) The gas loses heat where V is its volume |
An ideal monoatomic gas expands such that its pressure P and volume V follows the behaviour shown in the graph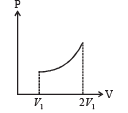 | (s) The gas gains heat |
Ans. (A)-(q); (B)-(p, r); (C)-(p, s); (D)-(q, s)
Solution. (A)-(q) : As the ideal gas expands in vacuum, no work is done (W = 0). Also the container is insulated therefore no heat is lost or gained (Q = 0). According to first law of thermodynamics 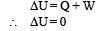
⇒ There is no change in the temparature of the gas (B)-(p, r) : Given PV2 = constant ....(i)
Also for an ideal gas PV/T = constant
From (i) & (ii) V × T = constant As the gas expands its volume increases and temperature decreases
∴ (p) is the correct option To find whether heat is released or absorbed let us find a relationship between Q and change in temperature ΔT.
We know that Q = n C Δ T ...(i)
where C = molar specific heat Also for a polytropic process we have
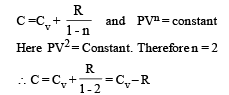
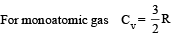
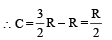
Substituting this value in (1) we get
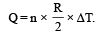
In this case the temperature decreases i.e. ΔT is negative.
Therefore Q is negative. This in turn means that heat is lost by the gas during the process. (r) is the correct option. (C)-(p, s) : Proceeding in the same way we get in this case V1/3× T = constant
⇒ As the gas expands and volume increases, the temperature decreases. Therefore (p) is the correct option In this process, 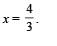
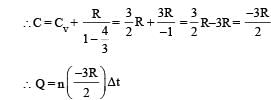
As ΔT is negative, Q is positive. This in turn means that heat is gained by the gas during the process (s) is the correct option.
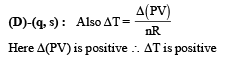
∴ temperature increase s (q) is the correct option From the graph it is clear that during the process the pressure of the gas increases which shows that the internal energy of the gas has increased. Also the volume increases which means work is done by the system which needs energy.From these two interpretation we can comfortably conclude that the gas gains heat during the process. (s) is the correct option.
Each question contains statements given in two columns, which have to be matched. The statements in Column-I are labelled A, B, C and D, while the statements in ColumnII are labelled p, q, r and s. Any given statement in Column-I can have correct matching with ONE OR MORE statement(s) in Column-II. The appropriate bubbles corresponding to the answers to these questions have to be darkened as illustrated in the following example : If the correct matches are A-p, s and t; B-q and r; C-p and q; and D-s then the correct darkening of bubbles will look like the given.

Q.3. One mole of a monatomic gas is taken through a cycle ABCDA as shown in the P-V diagram. Column II give the characteristics involved in the cycle. Match them with each of the processes given in Column I.
| Column I | Column II |
(A) Process A → B | (p) Internal energy decreases |
| (B) Process B → C | (q) Internal energy increases |
| (C) Process C → D | (r) Heat is lost |
| (D) Process D → A | (s) Heat is gained |
| (t) Work is done on the gas |
Ans. (A)-(p, r, t); (B)-(p, r); (C)-(q, s); (D)-(r, t)
Solution. (A) Process A→ B This is an isobaric process in which the volume of the gas decreases. Therefore work is done on the gas.
W = P (3V – V) = 2PV
Also temerature at B is less than temperature at A \ Heat is lost & internal energy decreases. (p, r, t) are correct matching
(B) Process B → C This is an isovolumic/isochoric process in which the pressure decreases Here temperature at B is less than temperature at C. \ Heat is lost and internal energy decreases. (p, r) are correct matching.
(C) Process C → D This is isobaric expansion where temperature at D is greater than temperature at C. Therefore internal energy increases and heat is gained. (q, s) are correct matching
(D) D → A
This is a process in which volume decreases. Therefore work is done on the gas.
Applying PV = nRT
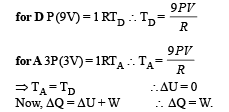
The energy obtained by the gas by work done on it is lost to the surroundings as Δ U = 0.
∴ (r, t) are correct matching.
Following question has matching lists. The codes for the list have choices (a), (b), (c) and (d) out of which ONLY ONE is correct.
Q.4. One mole of a monatomic ideal gas is taken along two cyclic processes E → F → G v E and E → F v H v E as shown in the PV diagram. The processes involved are purely isochoric, isobaric, isothermal or adiabatic.
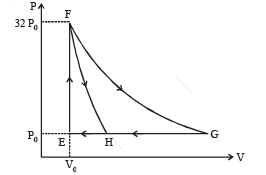
Match the paths in List I with the magnitudes of the work done in List II and select the correct answer using the codes given below the lists.
| P. G → E | 1. 160 P0V0 ln2 |
| Q. G → H | 2. 36 P0V0 |
| R. F → H | 3. 24 P0V0 |
S. F → G | 4. 31 P0V0 |
Ans. (a)
Solution.
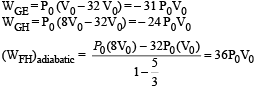

|
446 docs|930 tests
|





















