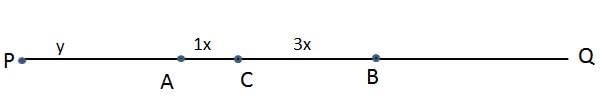Speed, Time & Distance: Solved Examples- 2 | CSAT Preparation - UPSC PDF Download

Question 8: Car A trails car B by 50 meters. Car B travels at 45km/hr. Car C travels from the opposite direction at 54km/hr. Car C is at a distance of 220 meters from Car B. If car A decides to overtake Car B before cars B and C cross each other, what is the minimum speed at which car A must travel? 
A. 36 km/hr
B. 45 km/hr
C. 67.5 km/hr
D. 18 km/hr
Answer:
Option: C
Explanation:
To begin with, let us ignore car A. Car B and car C travel in opposite directions.
Their relative speed = Sum of the two speeds = 45 + 54 kmph = 99kmph.
= 99 * 5/18m/s = 55/2m/s = 27.5m/s
The relative distance = 220m. So, time they will take to cross each other = 5/18 = 8 seconds
Now, car A has to overtake car B within 8 seconds. The relative distance = 50m 

=> Relative speed should be at least 50/8m/s = 6.25 m/s.
=>6.25 * 18/5kmph = 22.5 kmph
The question is " If car A decides to overtake Car B before cars B and C cross each other, what is the minimum speed at which car A must travel? "
Car B travels at 45kmph, so car A should travel at at least 45 + 22.5 = 67.5kmph.
Hence, the answer is 67.5kmph.

Question 9: A and B stand at distinct points of a circular race track of length 120m. They run at speeds of a m/s and b m/s respectively. They meet for the first time 16 seconds after they start the race and for the second time 40 seconds from the time they start the race. Now, if B had started in the opposite direction to the one he had originally started, they would have meet for the first time after 40 seconds. If B is quicker than A, find B’s speed.
A. 3 m/s
B. 4 m/s
C. 5 m/s
D. 8 m/s
Answer:
Option: A
Explanation:
They meet for the first time 16 seconds after they start the race and for the second time 40 seconds from the time they start the race.
Now, we do not know their relative positions when they start the race. But we know that the time between the first and second meeting is 24 seconds. This is the time when they cover a relative distance of one lap length.
Laplength/RelativeSpeed  = 24; or relative speed = 5 m/s.
The question says – Now, if B had started in the opposite direction to the one he had originally started, they would have met for the first time after 40 seconds.

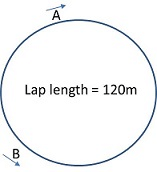
Now, B would have crossed each other after 40 seconds if B had reversed direction. This is higher than the 24 seconds it takes them to cover the relative distance of a lap in the first instance.
Or, in the first instance they were travelling towards each other.
Or, a + b = 5 m/s.
They meet for the first time 16 seconds after they start the race.
The question is "If B is quicker than A, find B’s speed."
Hence, the answer is 3 m/s

Question 10: City A to City B is a downstream journey on a stream which flows at a speed of 5km/hr. Boats P and Q run a shuttle service between the two cities that are 300 kms apart. Boat P, which starts from City A has a still-water speed of 25km/hr, while boat Q, which starts from city B at the same time has a still-water speed of 15km/hr. When will the two boats meet for the first time? (this part is easy) When and where will they meet for the second time?
A. 7.5 hours and 15 hours
B. 7.5 hours and 18 hours
C. 8 hours and 18 hours
D. 7.5 hours and 20 hours
Answer:
Option: D
Explanation:
Boat P starts from city A and Q starts from city B.
When boat P travels downstream, it will effectively have a speed to 30kmph. Likewise, Q will have an effective speed of 10kmph. The relative speed = 40kmph. So, the two boats will meet for the first time after 300/40 hours (Distance/relative speed) = 7.5 hours (Actually, for this part we do not need the speed of the stream)

The second part is more interesting, because the speed of the boats change when they change direction. Boat P is quicker, so it will reach the destination sooner. Boat P will reach City B in 10 hours 300/30. When boat P reaches city B, boat Q will be at a point 100kms from city B.
After 10 hours, both P and Q will be travelling upstream,
P's speed = 20 km/hr
Q's speed = 10 km/hr
Relative speed = 10km/hr
Q is ahead of P by 100 kms
P will catch up with Q after 10 more hours Distance/RelativeSpeed − 100/10.
So, P and Q will meet after 20 hours at a point 200 kms from city B
The question is " When will the two boats meet for the first time? When and where will they meet for the second time?"
Hence, the answer is 7.5 hours and 20 hours

Question 11: Cities M and N are 600km apart. Bus A starts from city M towards N at 9AM and bus B starts from city N towards M at the same time. Bus A travels the first one-third of the distance at a speed of 40kmph, the second one-third at 50kmph and the third one-third at 60kmph. Bus B travels the first one-third of the total time taken at a speed of 40kmph, the second one-third at 50kmph and the third one-third at 60kmph. When and where will the two buses cross each other?
A. 300 kms from M
B. 280 kms from M
C. 305 kms from M
D. 295 kms from M
Answer:
Option: D
Explanation:
Bus A
Travels 200km @ 40kmph
the next 200km @ 50kmph and
the final 200km @ 60kmph
So, Bus A will be at a distance of 200km from city M after 5 hours, and at a distance of 400km after 9 hours, and reach N after 12 hours and 20 mins

Bus B
Travels at an overall average speed of 50kmph, so will take 12 hours for the entire trip.
So, Bus B will travel 160 kms in the first 4 hours , 200 kms in the next 4 hours and 240 in the final 4 hours So, both buses cross each other when they are in their middle legs.
After 5 hours, bus A will be at a position 200kms from city M. At the same time, bus B will be at a distance 210kms from city N (4 * 40 + 50).
The distance between them will be 190kms (600 - 200 - 210). Relative speed = Sum of the the two speeds = 50 + 50 = 100 kmph.
Time taken = 190/100= 1.9 hours. = 1 hour and 54 minutes. So, the two buses will meet after 6 hours and 54 minutes. Bus B will have travelled 210 + 95 = 305 kms. So, the two buses will meet at a point that is 305 kms from City N and 295 kms from city A.
The question is "When and where will the two buses cross each other?
Hence, the buses cross 295 kms from city A.

Question 12: A car of length 4m wants to overtake a trailer truck of length 20m travelling at 36km/hr within 10 seconds. At what speed should the car travel?A. 12 m/s
B. 14.8 m/s
C. 12.4 m/s
D. 7.6 m/s
Answer:
Option: C
Explanation:
Given :
car of length = 4 m
length of truck = 20 m
Speed of truck = 36 km/hr
Approach:
Total distance to be covered by the car 'd' = 20 + 4 = 24 m
Let speed of car be S1 m/s
Speed of truck S2 = 36 km/hr = 10 m/s
Both the car and the truck are travelling in the same direction and we know that to overtake the truck, the speed of the ca should be more than that of the truck.
Hence, relative speed = |S1 - S2|
Time to overtake = 10s
S = d/t
|S1 - 10| = 24/10
S1 - 10 = 2.4
S1 = 10 + 2.4 = 12.4 m/s
The question is " At what speed should the car travel? "
Hence, the answer is 12.4 m/s

Question 13: Train A travelling at 63 kmph takes 27 to sec to cross Train B when travelling in opposite direction whereas it takes 162 seconds to overtake it when travelling in the same direction. If the length of train B is 500 meters, find the length of Train A.
A. 400 m
B. 810 m
C. 500 m
D. 310 m
Answer:
Option: C
Explanation:
Let the length of Train A be x meters
Let speed of Train B be y kmph
Relative distance = Relative speed * time taken to cross/overtake

Crossing scenario:
Relative speed of 2 trains = 63 + y
Time taken to cross = 27 sec or 27/3600 hrs
Relative distance between 2 trains = Length of Train A + length of train B = (x + 0.5) km
Therefore, x + 0.5 = (63 + y) * 27 / 3600 ----- (1)

Overtaking scenario:
Relative speed of 2 trains = 63 – y
Time taken to overtake = 162 sec or 162/3600 hrs
Relative distance between 2 trains = x + 0.5
Therefore, x + 0.5 = (63 – y) * 162/3600 --- (2)
From (1) and (2), solve for y.
(63 + y) * 27 = (63 – y) * 162
27y + 162 y = 63*162 – 63 *27
189y = 63 * 135 or y = 45 kmph

Substitute in (2) to get x.
x + 0.5 = (63 – 45) * 162/3600
Or x = 0.31 km or 310 meters
The question is "If the length of train B is 500 meters, find the length of Train A."
Hence, the answer is 310 m

Question 14: P cycles at a speed of 4 m/s for the first 8 seconds, 5 m/s for the next 8 seconds, 6 m/s for the next 8 and so on. Q cycles at a constant speed of 6.5 m/s throughout. If P and Q had to cycle for a 400 m race, how much lead in terms of distance, can P give Q and still finish at the same time as Q?
A. 43.4 m
B. 56.6 m
C. 32.1 m
D. P cannot give a lead as Q is always ahead of P
Answer:
Option: C
Explanation:
Distance covered by P in 8 sec = 4 * 8 = 32 m
Distance covered by P in 16 sec = 32 + 5 * 8 = 72 m
Distance covered by P in 24 sec = 72 + 6 * 8 = 120 m
Distance covered by P in 32 sec = 120 + 7 * 8 = 176 m
Distance covered by P in 40 sec = 176 + 8 * 8 = 240 m
Distance covered by P in 48 sec = 240 + 9 * 8 = 312 m
Total distance in 48 sec = 312m
To cover balance 86 m with speed = 10 m/sec, time taken = 86/10 = 8.6 sec
So P would finish 400 m in 48 + 8.6 = 56.6 seconds.
In 56.6 seconds, Q cycles 56.6 * 6.5 = 367.9 m or B should have a 32.1 m lead to result in a dead heat.
The question is "If P and Q had to cycle for a 400 m race, how much lead in terms of distance, can P give Q and still finish at the same time as Q?"
Hence, the answer is 32.1 m

Question 15 :A bus starts from a bus stop P and goes to another bus stop Q. In between P and Q, there is a bridge AB of certain length. A man is standing at a point C on the bridge such that AC:CB = 1:3. When the bus starts at P and if the man starts running towards A, he will meet the bus at A. But if he runs towards B, the bus will overtake him at B. Which of the following is true?
A. Bus travels 3x times faster than the man
B. Bus travels 2x times faster than the man
C. The bus and the man travel at the same speed
D. 4x the speed of the man is equal to 3x the speed of the bus
Answer:
Option: B
Explanation:

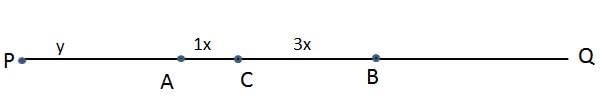
Let the speed of the bus be ‘b’ and man be ‘m’.
Let the distance between P and A be y.
AC:CB = 1:3 implies AC = x and CB = 3x

When bus goes from P to A and the man from C to A, time taken by both are equal and they meet at A.
y/b = x/m or
b/m = y/x --- (1)

When bus goes from P to B and the man from C to B, again time taken by both are equal and they meet at B.
(y+x+3x)/b = 3x/m
b/m = (4x+y)/3x ---(2)

Equating (1) and (2), we get y/x = (4x+y)/3x
3y = 4x + y or y = 2x

Therefore ratio of speeds b/m = 2x/x or 2 : 1
The question is "When the bus starts at P and if the man starts running towards A, he will meet the bus at A. But if he runs towards B, the bus will overtake him at B. Which of the following is true?"
Hence, the Bus travels 2x times faster than the man.