NCERT Exemplar: Probability- 2 | Mathematics (Maths) Class 12 - JEE PDF Download
LONG ANSWER TYPE QUESTIONS
Q.41. Three bags contain a number of red and white balls as follows:
Bag 1 : 3 red balls,
Bag 2 : 2 red balls and 1 white ball
Bag 3 : 3 white balls.
The probability that bag i will be chosen and a ball is selected from it is i/6, i = 1, 2, 3. What is the probability that
(1) a red ball will be selected?
(2) a white ball is selected?
Ans. Given that:
Bag I: 3 red balls and no white ball
Bag II: 2 red balls and 1 white ball
Bag III: no red ball and 3 white balls Let E1, E2 and E3 be the events of choosing Bag I, Bag II and Bag III respectively and a ball is drawn from it.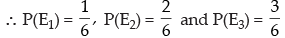
(i) Let E be the event that red ball is selected
∴ P(E) = P(E1).P (E/E1) + P(E2).P (E/E2)+ P(E3).P(E/E3)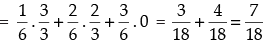
(ii) Let F be the event that a white ball is selected
∴ P(F) = 1 – P(E)
[P(E) + P(F) = 1]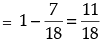
Hence, the required probabilities are 7/18 and 11/8.
Q.42. Refer to Question 41 above. If a white ball is selected, what is the probability that it came from
(1) Bag 2
(2) Bag 3
Ans. Referring to Exercise Q.41, we will use here Bayes’ Theorem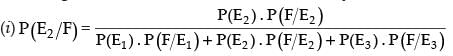
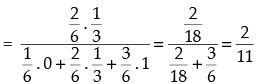
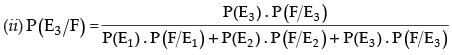
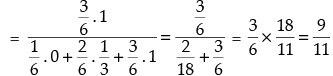
Hence, the required probabilities are 2/11 and 9/11.
Q.43. A shopkeeper sells three types of flower seeds A1, A2 and A3. They are sold as a mixture where the proportions are 4:4:2 respectively. The germination rates of the three types of seeds are 45%, 60% and 35%. Calculate the probability
(1) Of a randomly chosen seed to germinate
(2) That it will not germinate given that the seed is of type A3,
(3) That it is of the type A2 given that a randomly chosen seed does not germinate.
Ans. Given that A1 : A2 : A3 = 4 : 4 : 2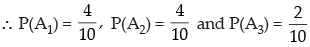
where A1, A2 and A3 are the three types of seeds.
Let E be the event that a seed germinates and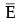 be the event that a seed does not germinate
be the event that a seed does not germinate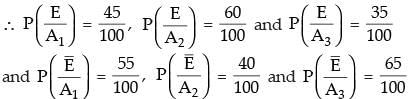
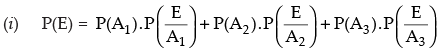
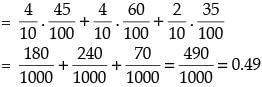
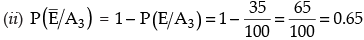
(iii) Using Bayes’ Theorem, we get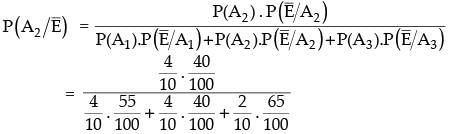
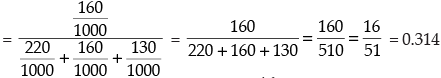
Hence, the required probability is 16/51 or 0.314.
Q.44. A letter is known to have come either from TATA NAGAR or from CALCUTTA. On the envelope, just two consecutive letter TA are visible. What is the probability that the letter came from TATA NAGAR.
Ans.
Let E1 : The event that the letter comes from TATA NAGAR
and E2 : The event that the letter comes from CALCUTTA
Also E3 : The event that on the letter, two consecutive letters TA are visible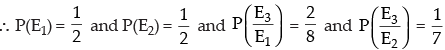
[∵ For TATA NAGAR, the two consecutive letters visible are TA, AT, TA, AN, NA, AG, GA, AR]
∴ P (E3/E1) = 2/8 and [For CALCUTTA, the two consecutive letters visible are CA, AL, LC, CU, UT, TT and TA] So, P (E3/E2) = 1/7
Now using Bayes’ Theorem, we have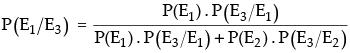
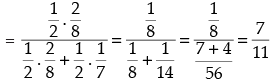
Hence, the required probability is 7/11.
Q.45. There are two bags, one of which contains 3 black and 4 white balls while the other contains 4 black and 3 white balls. A die is thrown. If it shows up 1 or 3, a ball is taken from the Ist bag; but it shows up any other number, a ball is chosen from the second bag. Find the probability of choosing a black ball.
Ans. Let E1 be the event of selecting Bag I
and E2 be the event of selecting Bag II
Let E3 be the event that black ball is selected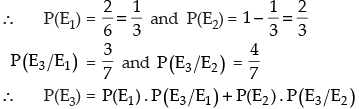
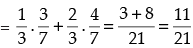
Hence, the required probability is 11/21.
Q.46. There are three urns containing 2 white and 3 black balls, 3 white and 2 black balls, and 4 white and 1 black balls, respectively. There is an equal probability of each urn being chosen. A ball is drawn at random from the chosen urn and it is found to be white. Find the probability that the ball drawn was from the second urn.
Ans. We have 3 urns: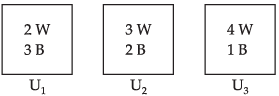
∴ Probabilities of choosing either of the urns are
P(U1) = P(U2) = P(U3) = 1/3
Let H be the event of drawing white ball from the chosen urn.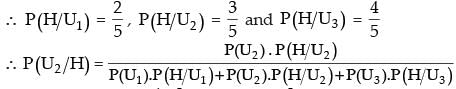
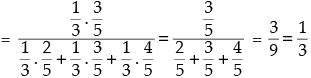
Hence, the required probability is 1/3.
Q.47. By examining the chest X ray, the probability that TB is detected when a person is actually suffering is 0.99. The probability of an healthy person diagnosed to have TB is 0.001. In a certain city, 1 in 1000 people suffers from TB. A person is selected at random and is diagnosed to have TB. What is the probability that he actually has TB?
Ans.
Let E1 : Event that a person has TB
E2 : Event that a person does not have TB
and H : Event that the person is diagnosed to have TB.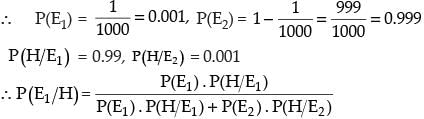
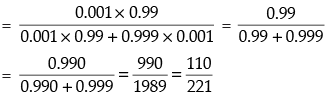
Hence, the required probability is 110/221.
Q.48. An item is manufactured by three machines A, B and C. Out of the total number of items manufactured during a specified period, 50% are manufactured on A, 30% on B and 20% on C. 2% of the items produced on A and 2% of items produced on B are defective, and 3% of these produced on C are defective. All the items are stored at one godown. One item is drawn at random and is found to be defective. What is the probability that it was manufactured on machine A?
Ans.
Let E1 : The event that the item is manufactured on machine A
E2 : The event that the item is manufactured on machine B
E3 : The event that the item is manufactured on machine C
Let H be the event that the selected item is defective.
∴ Using Bayes’ Theorem,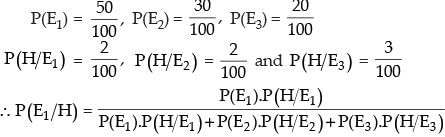
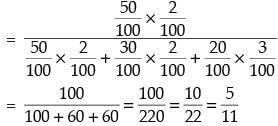
Hence, the required probability is 5/11.
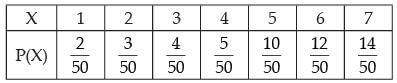
Q.49. Let X be a discrete random variable whose probability distribution is defined as follows: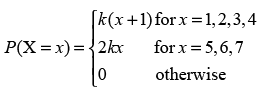
where k is a constant. Calculate
(i) The value of k
(ii) E (X)
(iii) Standard deviation of X.
Ans.
(i) Here, P(X = x) = k(x + 1) for x = 1, 2, 3, 4
So, P(X = 1) = k(1 + 1) = 2k; P(X = 2) = k(2 + 1) = 3k
P(X = 3) = k(3 + 1) = 4k; P(X = 4) = k(4 + 1) = 5k
Also, P(X = x) = 2kx for x = 5, 6, 7
P(X = 5) = 2(5)k = 10k; P(X = 6) = 2(6)k = 12k
P(X = 7) = 2(7)k = 14k
and for otherwise it is 0.
∴ The probability distribution is given by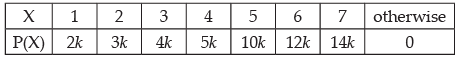
We know that
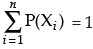
So, 2 k + 3k + 4k + 5k + 10k + 12k + 14k = 1
⇒ 50k = 1
⇒ k = 1/50
Hence, the value of k is 1/50
(ii) Now the probability distribution is
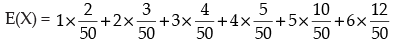
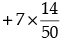
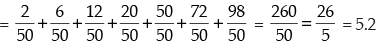
(ii) We know that Standard deviation (SD) =
Variance = E(X2) – [E(X)]2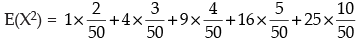
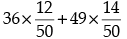
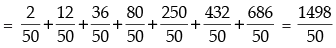
∴ Variance (X)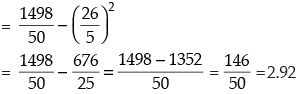
∴ S.D = √2.92 = 1.7 (approx.)
Q.50. The probability distribution of a discrete random variable X is given as under: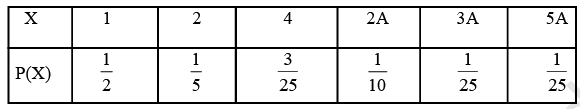
Calculate :
(i) The value of A if E(X) = 2.94
(ii) Variance of X.
Ans.
(i) We know that: E(X) =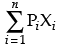
∴ 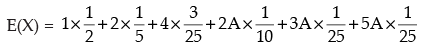
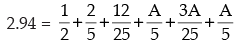
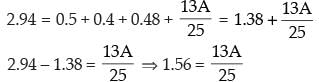
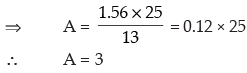
(ii) Now the distribution becomes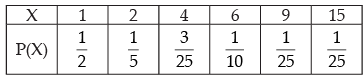
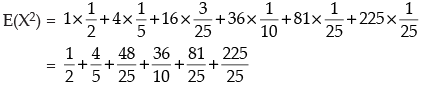
= 0.5 + 0.8 + 1.92 + 3.6 + 3.24 + 9.00 = 19.06
Variance (X) = E(X2) – [E(X)]2
= 19.06 – (2.94)2 = 19.06 – 8.64 = 10.42
Q.51. The probability distribution of a random variable x is given as under: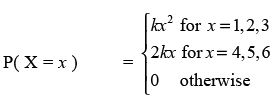
where k is a constant. Calculate
(1) E(X)
(2) E (3X2)
(3) P(X ≥ 4)
Ans.
Given that:
P(X = x) =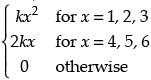
∴ Probability distribution of random variable X is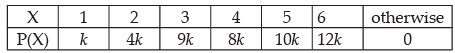
We know that 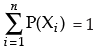
∴ k + 4k + 9k + 8k + 10k + 12k = 1
⇒ 44k = 1
⇒ k = 1/44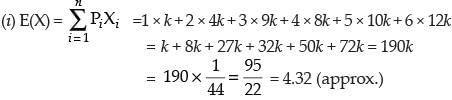
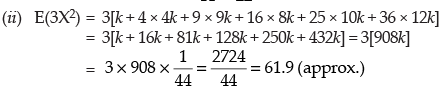
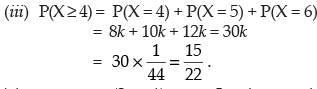
Q.52. A bag contains (2n + 1) coins. It is known that n of these coins have a head on both sides where as the rest of the coins are fair. A coin is picked up at random from the bag and is tossed. If the probability that the toss results in a head is 31/42, determine the value of n.
Ans.
Given that n coins are two headed coins and the remaining (n + 1) coins are fair.
Let E1 : the event that unfair coin is selected
E2 : the event that the fair coin is selected
E : the event that the toss results in a head
∴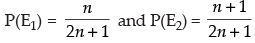
P(E/E1) = 1 (sure event) and P (E/E2) = 1/2
∴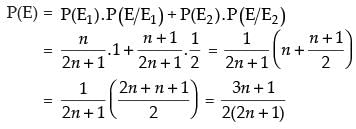
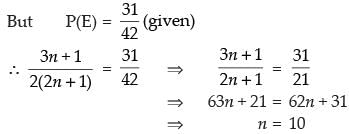
Hence, the required value of n is 10.
Q.53. Two cards are drawn successively without replacement from a well shuffled deck of cards. Find the mean and standard variation of the random variable X where X is the number of aces.
Ans.Let X be the random variable such that X = 0, 1, 2
and E = the event of drawing an ace
and F = the event of drawing non-ace.
We have Distribution Table: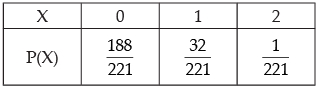
Now, Mean E(X) =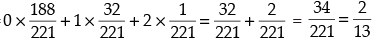
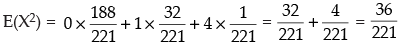
∴ Variance = E(X2) – [E(X)]2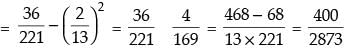
Standard deviation =
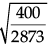 = 0.377(approx.)
= 0.377(approx.)
Q.54. A die is tossed twice. A ‘success’ is getting an even number on a toss. Find the variance of the number of successes.
Ans.
Let E be the event of getting even number on tossing a die.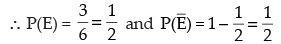
Here X = 0, 1, 2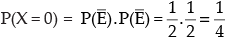
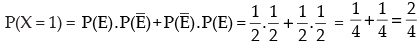
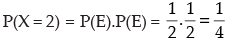
∴ Probability distribution table is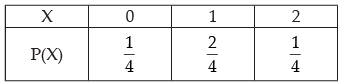
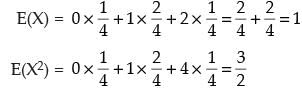
∴ Variance (X) = E(X2) – [E(X)]2
=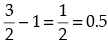
Q.55. There are 5 cards numbered 1 to 5, one number on one card. Two cards are drawn at random without replacement. Let X denote the sum of the numbers on two cards drawn. Find the mean and variance of X.
Ans.Here, sample space S = {(1,2), (2,1),(1,3), (3,1),(2,3),(3,2),(1,4),(4, 1),(1, 5), (5,1), (2,4),(4, 2),(2,5),(5,2), (3,4), (4,3), (3,5), (5,3), (5,4),(4,5)}
∴ n(S) = 20
Let X be the random variable denoting the sum of the numbers on two cards drawn.
∴ X = 3, 4, 5, 6, 7, 8, 9
So,
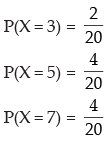
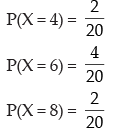
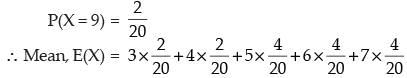
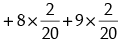
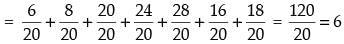
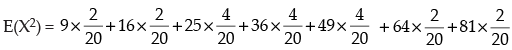
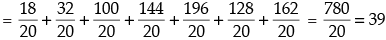
∴Variance (X) = E(X2) – [E(X)]2 = 39 – (6)2 = 39 – 36 = 3
OBJECTIVE TYPE QUESTIONS
Choose the correct answer from the given four options in each of the exercises from 56 to 82.
Q.56. If P(A) = 4/5 and P(A ∩ B) = 7/10 then P (B/A) is equal to
(1) 1/10
(2) 1/8
(3) 7/8
(4) 17/20
Ans.
Given that: P(A) = 4/5 and P(A ∩ B) = 7/10
∴ 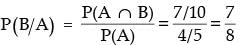
Hence, the correct option is (3).
Q.57. If P(A ∩ B) = 7/10 and P(B) =17/20, then P (A | B) equals
(1) 14/17
(2) 17/20
(3) 7/8
(4) 1/8
Ans.
Given that: P(A ∩ B) = 7/10 and P(B) = 17/20
∴ 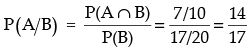
Hence, the correct option is (1).
Q.58. If P(A) = 3/10, P (B) = 2/5 and P(A ∪ B) = 3/5, then P (B | A) + P (A | B) equals
(1) 1/4
(2) 1/3
(3) 5/12
(4) 7/2
Ans.
Here, P(A) = 3/10, P(B) = 2/5 and P(A ∪ B) = 3/5
P(A ∪ B) = P(A) + P(B) - P(A ∩ B)
3/5 = 3/10 + 2/5 - P(A ∩ B)
Now P (A/B) +P (B/A) =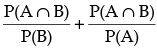
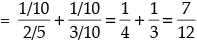
Hence, the correct option is (4).
Q.59. If P(A) = 2/5, P(B) = 3/10 and P (A ∩ B) =1/5, then P (A′|B′). P (B'|A')is equal to
(1) 5/6
(2) 5/7
(3) 25/42
(4) 1
Ans.
Given that: P(A) = 2/5, P(B) = 3/10 and P(A ∩ B) = 1/5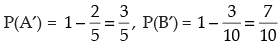
and P(A' ∩ B') = 1 - P(A ∪ B) = 1 - [P(A) + P(B) - P(A ∩ B)]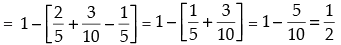
∴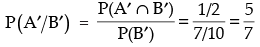
and 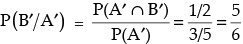
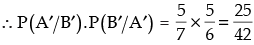
Hence, the correct option is (3).
Q.60. If A and B are two events such that P(A) = 1/2, P(B) = 1/3, P(A/B) = 1/4, then P(A′ ∩ B′ ) equals
(1) 1/12
(2) 3/4
(3) 1/4
(4) 3/16
Ans.
Given that: P(A) = 1/2 ,P(B) = 1/3 and P (A/B) = 1/4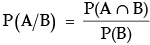
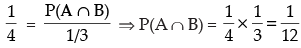
Now P(A' ∩ B') = 1 - P(A ∪ B)
= 1 - [P(A) + P(B) - P(A ∩ B)]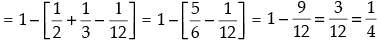
Hence, the correct option is (3).
Q.61. If P(A) = 0.4, P(B) = 0.8 and P(B|A) = 0.6, then P(A ∪ B) is equal to
(1) 0.24
(2) 0.3
(3) 0.48
(4) 0.96
Ans.
Given that: P(A) = 0.4, P(B) = 0.8 and P (B/A) = 0.6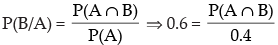
∴ P(A ∩ B) = 0.6 × 0.4 = 0.24
P(A ∪ B) = P(A) + P(B) - P(A ∩ B)
= 0.4 + 0.8 – 0.24 = 1.20 – 0.24 = 0.96
Hence, the correct option is (4).
Q.62. If A and B are two events and A ≠ φ, B ≠ φ, then
(1) P(A|B) = P(A).P(B)
(2) P(A|B) = P(A ∩ B)/P(B)
(3) P(A|B).P(B|A)=1
(4) P(A|B) = P(A)|P(B)
Ans.
Given that: A = φ and B ≠ φ,
then 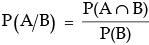
Hence, the correct option is (2).
Q.63. A and B are events such that P(A) = 0.4, P(B) = 0.3 and P(A ∪ B) = 0.5. Then P (B′ ∩ A) equals
(1) 2/3
(2) 1/2
(3) 3/10
(4) 1/5
Ans.
Given that: P(A) = 0.4, P(B) = 0.3 and P(A ∪ B) = 0.5
P(A ∪ B) = P(A) + P(B) - P(A ∩ B)
0.5 = 0.4 + 0.3 – P(A ∩ B)
P(A ∩ B) = 0.4 + 0.3 - 0.5 = 0.2
∴ P(B' ∩ A) = P(A) - P(A ∩ B)
= 0.4 – 0.2 = 0.2 = 1/5
Hence, the correct option is (4).
Q.64. You are given that A and B are two events such that P(B)= 3/5, P(A | B) = 1/2 and P(A ∪ B) = 4/5, then P(A) equals
(1) 3/10
(2) 1/5
(3) 1/2
(4) 3/5
Ans.
Given that: P(B) = 3/5, P (A/B) = 1/2 and P(A ∪ B) = 4/5
We know that P (A/B) = P(A ∩ B)/P(B)
1/2 =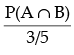
∴ P(A ∩ B) = 3/10
Now P(A ∪ B) = P(A) + P(B) - P(A ∩ B)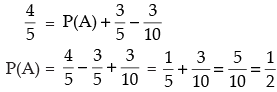
Hence, the correct option is (3).
Q.65. In Exercise 64 above, P(B | A′) is equal to
(1) 1/5
(2) 3/10
(3) 1/2
(4) 3/5
Ans.According to Exercise 64, we have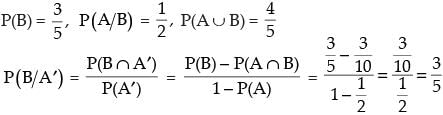
Hence, the correct option is (4).
Q.66. If P(B) = 3/5, P(A | B) = 1/2 and P(A ∪ B) =4/5, then P(A ∪ B )′ + P( A′ ∪ B) =
(1) 1/5
(2) 4/5
(3) 1/2
(4) 1
Ans. Given that: P(B) = 3/5, P (A/B) = 1/2 and P(A ∪ B) = 4/5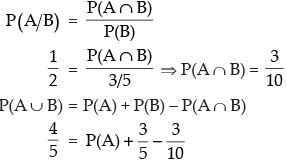
∴ 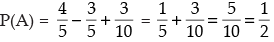
Now P(A ∪ B)' + P(A' ∪B)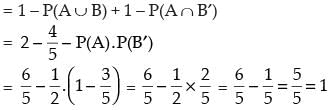
Hence, the correct option is (4).
Q.67. Let P(A) = 7/13, P(B) = 9/13 and P(A ∩ B) =4/13. Then P( A′ | B) is equal to
(1) 6/13
(2) 4/13
(3) 4/9
(4) 5/9
Ans.
Given that: P(A) = 7/13, P(B) = 9/13 and P(A ∩ B) = 4/13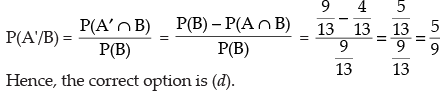
Q.68. If A and B are such events that P(A) > 0 and P(B) ≠ 1, then P( A′ | B′ ) equals.
(1) 1 – P(A | B)
(2) 1– P( A ′ | B)
(3)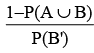
(4) P(A′) | P( B′)
Ans.
Given that: P(A) > 0 and P(B) ≠ 1
∴ 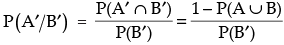
Hence, the correct option is (3).
Q.69. If A and B are two independent events with P(A) = 3/5 and P(B) =4/9, then P( A′ ∩ B′) equals
(1) 4/15
(2) 8/45
(3) 1/3
(4) 2/9
Ans. Given that: A and B are independent events
such that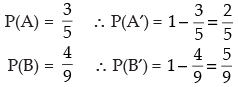
∴ 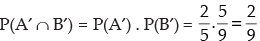
Hence, the correct option is (d).
Q.70. If two events are independent, then
(1) They must be mutually exclusive
(2) The sum of their probabilities must be equal to 1
(3) (1) and (2) both are correct
(4) None of the above is correct
Ans. For independent events A and B, P(A).P(B) = P(A ∩ B)
So, they will not be mutually exclusive.
If P(A) + P(B) = 1, they are exhaustive events and for independent events A and
P(A ∩ B) ≠ 0.
Hence, the correct option is (4).
Q.71. Let A and B be two events such that P(A) = 3/8, P(B) = 5/8 and P(A ∪ B) =3/4. Then P(A | B).P(A′ | B) is equal to
(1) 2/5
(2) 3/8
(3) 3/20
(4) 6/25
Ans.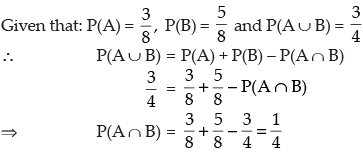
Now P (A/B) .P (A'/B)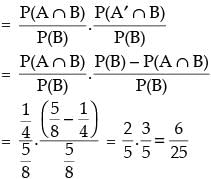
Hence, the correct option is (4).
Q.72. If the events A and B are independent, then P(A ∩ B) is equal to
(1) P (A) + P (B)
(2) P(A) – P(B)
(3) P (A) . P(B)
(4) P(A) | P(B)
Ans.
Since A and B are two independent events
∴ P(A ∩ B) = P(A).P(B)
Hence, the correct option is (3).
Q.73. Two events E and F are independent. If P(E) = 0.3, P(E ∪ F) = 0.5, then P(E | F)–P(F | E) equals
(1) 2/7
(2) 3/35
(3) 1/70
(4) 1/7
Ans.
Given that: E and F are independent events such that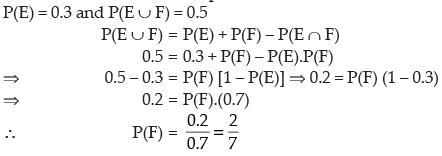
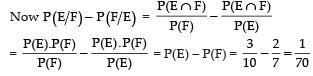
Hence, the correct option is (3).
Q.74. A bag contains 5 red and 3 blue balls. If 3 balls are drawn at random without replacement the probability of getting exactly one red ball is
(1) 45/196
(2) 135/392
(3) 15/56
(4) 15/29
Ans.
Given that: Bag contains 5 red and 3 blue balls.
Probability of getting exactly one red ball if 3 balls are randomly drawn without replacement
= P(R) . P(B) . P(B) + P(B) . P(R) . P(B) + P(B) . P(B) . P(R)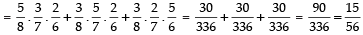
Hence, the correct option is (3).
Q.75. Refer to Question 74 above. The probability that exactly two of the three balls were red, the first ball being red, is
(1) 1/3
(2) 4/7
(3) 15/28
(4) 5/28
Ans.
According to Question 74,
Let E1 be the event that first ball is red.
E2 be the event that exactly two of the three balls are red.
∴ P(E1) = P(R).P(R).P(B) + P(R).P(R).P(R) + P(R).P(B).P(R) + P(R).P(B).P(B)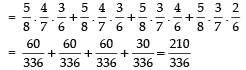
P(E1 ∩ E2) = P(R) . P(B) . P(R) + P(R) . P(R) . P(B)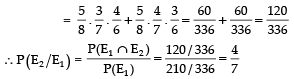
Hence, the correct option is (2).
Q.76. Three persons, A, B and C, fire at a target in turn, starting with A. Their probability of hitting the target are 0.4, 0.3 and 0.2 respectively. The probability of two hits is
(1) 0.024
(2) 0.188
(3) 0.336
(4) 0.452
Ans.
Given that: P(A) = 0.4, P(B) = 0.3 and P(C) = 0.2
Also = 1 – 0.4 = 0.6,
= 1 – 0.4 = 0.6,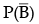 = 1 – 0.3 = 0.7
= 1 – 0.3 = 0.7
and 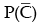 = 1 – 0.2 = 0.8
= 1 – 0.2 = 0.8
∴ Probabilities of two hits
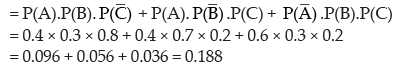
Hence, the correct option is (2).
Q.77. Assume that in a family, each child is equally likely to be a boy or a girl. A family with three children is chosen at random. The probability that the eldest child is a girl given that the family has at least one girl is
(1) 1/2
(2) 1/3
(3) 2/3
(4) 4/7
Ans.
Let G denotes the girl and B denotes the boy of the given family.
So, n(S) = {(BGG), (GBG), (GGB), (GBB), (BGB), (BBG), ( BBB), ( GGG)}
Let E1 be the event that the family has alteast one girl.
∴ E1 = {(BGG), (GBG), (GGB), (GBB), (BGB), (BBG), (GGG)}
Let E2 be the event that the eldest child is a girl.
∴E2 = {(GBG), (GGB), (GBB), (GGG)}
(E1 ∩ E2) = {(GBB), (GGB), (GBG), (GGG)}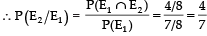
Hence, the correct option is (4).
Q.78. A die is thrown and a card is selected at random from a deck of 52 playing cards. The probability of getting an even number on the die and a spade card is
(1) 1/2
(2) 1/4
(3) 1/8
(4) 3/4
Ans.
Let E1 be the event of getting even number on the die. E2 be the event of selecting a spade card.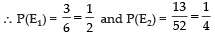
So, 
Hence, the correction option is (3).
Q.79. A box contains 3 orange balls, 3 green balls and 2 blue balls. Three balls are drawn at random from the box without replacement. The probability of drawing 2 green balls and one blue ball is
(1) 3/28
(2) 2/21
(3) 1/28
(4) 167/168
Ans. Probability of drawing 2 green and 1 blue balls
= P(G).P(G).P(B) + P(G) . P(B).P(G) + P(B).P(G).P(G)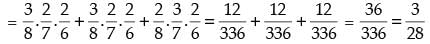
Hence, the correct option is (1).
Q.80. A flashlight has 8 batteries out of which 3 are dead. If two batteries are selected without replacement and tested, the probability that both are dead is
(1) 33/56
(2) 9/64
(3) 1/14
(4) 3/28
Ans.
Required probability = P(dead).P(dead)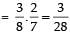
Hence, the correct option is (4)
Q.81. If eight coins are tossed together. The probability of getting exactly 3 heads is
(1) 1/256
(2) 7/32
(3) 5/32
(4) 3/32
Ans.
Here, n = 8, p = 1/2, q = 1-(1/2) = 1/2 and r = 3
we know that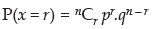
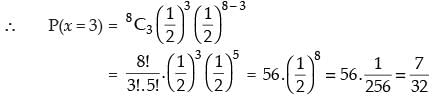
Hence, the correct option is (2).
Q.82. Two dice are thrown. If it is known that the sum of numbers on the dice was less than 6, the probability of getting a sum 3, is
(1) 1/18
(2) 5/18
(3) 1/5
(4) 2/5
Ans.
Let E1 be the event showing the sum of the numbers on the two dice was less than 6 and E2 be the event that the sum of the numbers is 3.
∴ E1 = {(1, 1), (1, 2), (2, 1), (1, 3), (3, 1), (1, 4), (4, 1), (2, 2), (2, 3), (3, 2)}
n(E1) = 10
and E2 = {(1, 2), (2, 1)}
⇒ n(E2) = 2 and n(E1 ∩ E2) = 2
∴ Required probability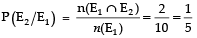
Hence, the correct option is (3).
Q.83. Which one is not a requirement of a binomial distribution?
(1) There are 2 outcomes for each trial
(2) There is a fixed number of trials
(3) The outcomes must be dependent on each other
(4) The probability of success must be the same for all the trials
Ans.
We know that for a Binomial distribution, the outcomes must not be dependent on each other.
Hence, the correct option is (3).
Q.84. Two cards are drawn from a well shuffled deck of 52 playing cards with replacement. The probability, that both cards are queens, is
(1)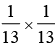
(2)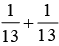
(3)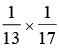
(4)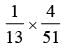
Ans.
Probability of getting Queen = 4/52
So, the required probability = P(Queen).P(Queen)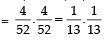
(with replacement)
Hence, the correct option is (1).
Q.85. The probability of guessing correctly at least 8 out of 10 answers on a true-false type examination is
(1) 7/64
(2) 7/128
(3) 45/1024
(4) 7/41
Ans.
Here, n = 10, p = 1/2 and q = 1/2 (for true/false questions)
and r ≥ 8 i.e. 8, 9, 10
∴ P(X ≥ 8) = P(x = 8) + P(x = 9) + P(x = 10)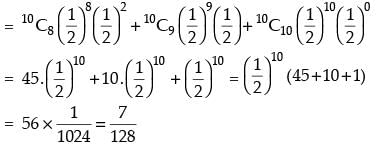
Hence, the correct option is (2).
Q.86. The probability that a person is not a swimmer is 0.3. The probability that out of 5 persons 4 are swimmers is
(1) 5C4 (0.7)4 (0.3)
(2) 5C1 (0.7) (0.3)4
(3) 5C4 (0.7) (0.3)4
(4) (0.7)4 (0.3)
Ans.
Given that: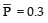
∴ p = 0.7 and q = 1 – 0.7 = 0.3
n = 5 and r = 4
We know that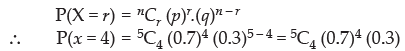
Hence, the correct option is (1).
Q.87. The probability distribution of a discrete random variable X is given below:
| X | 2 | 3 | 4 | 5 |
| P(X) | 5/k | 7/k | 9/k | 11/k |
The value of k is
(1) 8
(2) 16
(3) 32
(4) 48
Ans. We know that 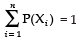
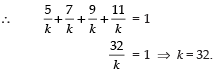
Hence, the correct option is (3).
Q.88. For the following probability distribution:
| X | -4 | -3 | -2 | -1 | 0 |
| P(X) | 0.1 | 0.2 | 0.3 | 0.2 | 0.2 |
E(X) is equal to :
(1) 0
(2) –1
(3) –2
(4) –1.8
Ans. We know that:
= (– 4)(0.1) + (– 3)(0.2) + (– 2)(0.3) + (– 1)(0.2) + 0(0.2)
= – 0.4 – 0.6 – 0.6 – 0.2 = – 1.8
Hence, the correct option is (d).
Q.89. For the following probability distribution
| X | 1 | 2 | 3 | 4 |
| P(X) | 1/10 | 1/5 | 3/10 | 2/5 |
E(X2) is equal to
(1) 3
(2) 5
(3) 7
(4) 10
Ans.We know that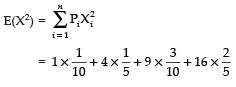
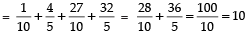
Hence, the correct option is (4).
Q.90. Suppose a random variable X follows the binomial distribution with parameters n and p, where 0 < p < 1. If P(x = r) / P(x = n–r) is independent of n and r, then p equals
(1) 1/2
(2) 1/3
(3) 1/5
(4) 1/7
Ans.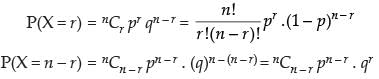
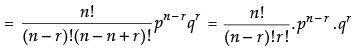
Now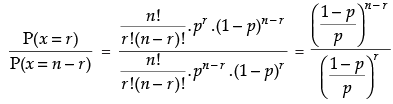
The above expression will be independent of n and r if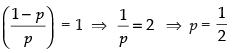
Hence, the correct option is (1).
Q.91. In a college, 30% students fail in physics, 25% fail in mathematics and 10% fail in both. One student is chosen at random. The probability that she fails in physics if she has failed in mathematics is
(1) 1/10
(2) 2/5
(3) 9/20
(4) 1/3
Ans.
Let E1 be the event that the student fails in Physics and E2 be the event that she fails in Mathematics.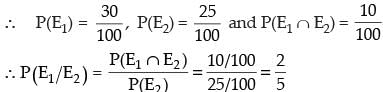
Hence, the correct option is (2).
Q.92. A and B are two students. Their chances of solving a problem correctly are 1/3 and 1/4, respectively. If the probability of their making a common error is, 1/20 and they obtain the same answer, then the probability of their answer to be correct is
(1) 1/12
(2) 1/40
(3) 13/120
(4) 10/13
Ans.
Let E1 be the event that both of them solve the problem.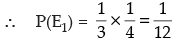
and E2 be the event that both of them same incorrectly the problem.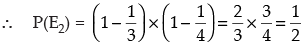
Let H be the event that both of them get the same answer.
Here, P (H/E1 ) = 1, P (H/E2 ) = 1/20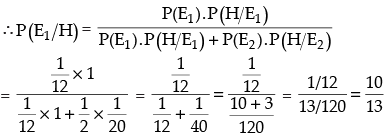
Hence, the correct option is (4).
Q.93. A box has 100 pens of which 10 are defective. What is the probability that out of a sample of 5 pens drawn one by one with replacement at most one is defective?
(A)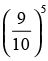
(B)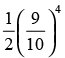
(C)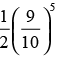
(D)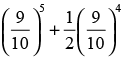
Ans.
Here, n = 5, p = 10/100 = 1/10 and q = 1-(1/10) = 9/10 and r ≤ 1
we know that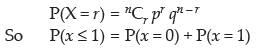
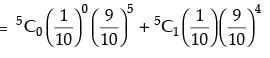
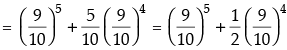
Hence, the correct option is (4).
State True or False for the statements in each of the Exercises 94 to 103.
Q.94. Let P(A) > 0 and P(B) > 0. Then A and B can be both mutually exclusive and independent.
Ans. False
Q.95. If A and B are independent events, then A ′ and B′ are also independent.
Ans. True
Q.96. If A and B are mutually exclusive events, then they will be independent also.
Ans. False
Q.97. Two independent events are always mutually exclusive.
Ans. False
Q.98. If A and B are two independent events then P(A and B) = P(A).P(B).
Ans. True
Q.99. Another name for the mean of a probability distribution is expected value.
Ans. True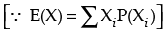
Q.100. If A and B′ are independent events, then P(A'∪ B) = 1 – P (A) P(B')
Ans. True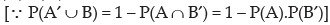
Q.101. If A and B are independent, then
P (exactly one of A, B occurs) = P (A) P (B′) + P ( B) P ( A′ )
Ans. True
Q.102. If A and B are two events such that P(A) > 0 and P(A) + P(B) >1, then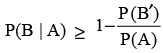
Ans. False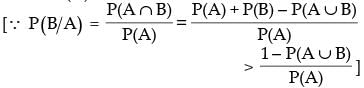
Q.103. If A, B and C are three independent events such that P(A) = P(B) = P(C) = p, then P (At least two of A, B, C occur) = 3p2 − 2p3
Ans. True
Since P (atleast two of A, B and C occur)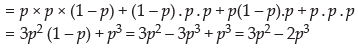
Q.104. If A and B are two events such that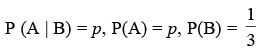
and 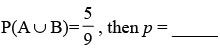
Ans.
Given that: P(A) = p, P(B) = 1/3 and P(A ∪ B) = 5/9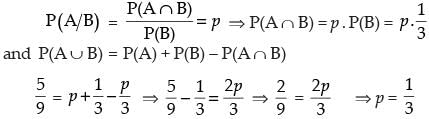
Hence, p is equal to 1/3 .
Q.105. If A and B are such that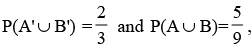
then P(A') + P(B') = _______
Ans.
Here, 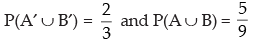
∴
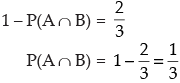
Now P(A') + P(B') = 1 - P(A) + 1 - P(B) = 2 - [P(A) + P(B)]
= 2 - [P(A ∪ B) + P(A ∩ B)]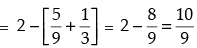
Hence, the value of the filler is 10/9.
Q.106. If X follows binomial distribution with parameters n = 5 , p and P (X = 2) = 9, P (X = 3), then p = _______
Ans.
Given that: P(X = 2) = 9P(X = 3)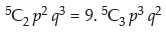
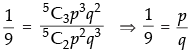 [∵ 5C3 = 5C2]
[∵ 5C3 = 5C2]
⇒ 9p = q
⇒ 9p = 1 – p
⇒ 9p + p = 1
⇒ 10p = 1
∴ p = 1/10
Hence, the value of the filler is 1/10 .
Q.107. Let X be a random variable taking values x1, x2,..., xn with probabilities p1, p2, ..., pn, respectively. Then var (X) = _______
Ans.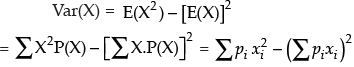
Hence, Var (X) is equal to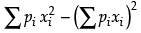
Q.108. Let A and B be two events. If P(A | B) = P(A), then A is ______ of B.
Ans.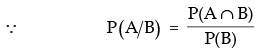
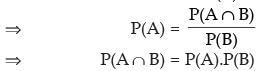
So, A is independent of B.
|
203 videos|377 docs|167 tests
|





















