Introduction: Order of Magnitude Estimates | Physics for EmSAT Achieve PDF Download

What does "order of magnitude" mean?

• The concept of "order of magnitude" refers to a significant change in value, either increasing or decreasing by a factor of 10. This term is commonly employed in scientific notation that uses powers of 10.
• The purpose of using the order of magnitude is to simplify the understanding of numerical values and measurements. It allows for approximate comparisons between two quantities. For instance, when comparing the circumference of the Sun to that of the Earth, we would describe the Sun's circumference as being several orders of magnitude greater than that of the Earth.

How is the order of magnitude determined?

• The order of magnitude is determined by considering the scale or class to which a numerical value belongs. Each class on a logarithmic scale, such as base 10, contains values that have a fixed ratio to the preceding class.
• In a base 10 logarithmic scale, increasing the order of magnitude by one is equivalent to multiplying a quantity by 10. This results in an increase of the exponent by one, moving to the next power of 10. Increasing the order of magnitude by two is equivalent to multiplying by 100, or 10². Generally, increasing the order of magnitude by "n" is equivalent to multiplying a quantity by 10ⁿ. For example, 2,315 is one order of magnitude larger than 231.5, which itself is one order of magnitude larger than 23.15.
• Conversely, as values decrease, decreasing the order of magnitude by one is the same as multiplying a quantity by 0.1. Decreasing the order of magnitude by two is equivalent to multiplying by 0.01, or 10^-2. Generally, decreasing the order of magnitude by "n" is equivalent to multiplying a quantity by 10^-n. For instance, 23.15 is one order of magnitude smaller than 231.5, and 231.5 is one order of magnitude smaller than 2,315. As the order of magnitude decreases, the decimal point moves to the left.
• In the International System of Units (SI), various quantities can be expressed in multiples or fractions based on the order of magnitude. For instance, adding the prefix "kilo-" to a unit increases its size by three orders of magnitude, or 1,000 (10³). Adding the prefix "micro-" decreases the size of a unit by six orders of magnitude, equivalent to multiplying it by one millionth (10^-6). Scientists and engineers have designated prefix multipliers ranging from septillionths (10^-24) to septillions (10²⁴), covering a span of 48 orders of magnitude.

Order of magnitude with powers of 10 

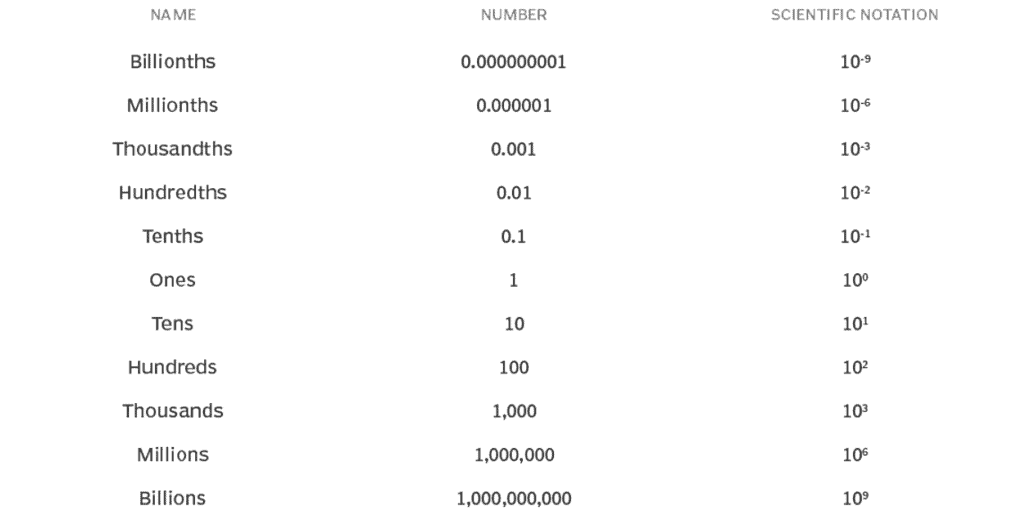
Order of magnitude uses and examples

Order of magnitude is used to make estimates and approximate comparisons in scientific notation. It is a way to represent numbers that are comparatively larger or smaller than other numbers.

Some examples of very large and very small numbers that benefit from order of magnitude representation include the following:

• the weight of a carbon atom
• the size of an electron
• the length of a tardigrade
• the speed of a supercomputer
• the age of the Earth
• the amount of data in a data lake
• the speed of flash storage

Here are some alternative ways in which the concept of order of magnitude can be applied:

• Magnitude Differences: This refers to the number of powers of 10 that separate two values. For instance, if one value is 100 times greater than another, it can be said that the first value is two orders of magnitude larger than the second.
• Approximations: Order of magnitude can be utilized to make rough estimates of variables with unknown precise values. For example, when estimating the global population, which is approximately 7.9 billion, the exact figure may be uncertain, but it falls within the range of 1 to 10 billion. By rounding to the nearest order of magnitude, one might approximate the Earth's population to be around 10 billion, as it is a multiple of 10.