Unit Test (Solutions): Real Numbers | Mathematics (Maths) Class 10 PDF Download

Clear all your Class 10 doubts with EduRev Join for Free

Join for Free

Time: 1 hour

M.M. 30

Attempt all questions.

• Question numbers 1 to 5 carry 1 mark each.
• Question numbers 6 to 8 carry 2 marks each.
• Question numbers  9 to 11 carry 3 marks each.
• Question number 12 & 13 carry 5 marks each.

Q1: Which of the following numbers is irrational?  (1 Mark)  
(a) √25
(b) 3.14
(c) π
(d) -7

Ans: (c)
Sol: An irrational number is a number that cannot be expressed as a fraction of two integers. Option c) π is a non-repeating, non-terminating decimal and cannot be expressed as a fraction.

Q2: What is the value of (5² + 12²)?  (1 Mark)  
(a) 169
(b) 144
(c) 25
(d) 169√2

Ans: (a)
Sol:The given expression is (5² + 12²) = (25 + 144) = 169.

Q3: Which one is not a prime number?  (1 Mark)  
(a) 1
(b) 2
(c) 3
(d) 5

Ans: (a)
Sol: A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. However, 1 does not meet this criteria, as it only has one positive divisor.

Q4: State whether "√16" is a rational number or not.  (1 Mark)  

Ans:  "√16" is a rational number.
Sol: √16 = 4, which is a rational number since it can be expressed as the fraction 4/1.

Q5: What is the prime factorization of 96?  (1 Mark)

Ans: 2⁵ x  3
Sol: The prime factorization of 96 is: 2 × 2 × 2 × 2 × 2 × 3.

Q6: Prove that the square of any positive integer of the form (5k + 1) is one more than a multiple of 8, where "k" is an integer. (2 Marks) 

Ans: The square of any number of the form (5k+1) is always 1 more than a multiple of 8
Sol: 

Let's assume the positive integer be "n" in the form of (5k + 1).
Step 1: Square of "n"
n² = (5k + 1)² = 25k² + 10k + 1 = 5(5k² + 2k) + 1
Step 2: Express (5k² + 2k) as an integer "m"

Let (5k² + 2k) = m (where m is an integer)
Step 3: Express n² in terms of "m"
n² = 5m + 1
Step 4: Prove that n² is one more than a multiple of 8

n² = 5m + 1 = 8k + (5m - 8k + 1)
Since (5m - 8k + 1) is an integer, let's say it equals "p"
n² = 8k + p
Thus, n² is one more than a multiple of 8.

Q7:  Prove that √2 is irrational (2 Marks)

Ans: To prove that √2 is an irrational number, we will use the contradiction method.

Let us assume that √2 is a rational number with p and q as co-prime integers and q ≠ 0

⇒ √2 = p/q

On squaring both sides we get,

⇒ 2q² = p²

⇒ Here, 2q² is a multiple of 2 and hence it is even. Thus, p² is an even number. Therefore, p is also even.

So we can assume that p = 2x where x is an integer.

By substituting this value of p in 2q² = p², we get

⇒ 2q² = (2x)²

⇒ 2q² = 4x²

⇒ q² = 2x²

⇒ q² is an even number. Therefore, q is also even.

Since p and q both are even numbers, they have 2 as a common multiple which means that p and q are not co-prime numbers as their HCF is 2.

This leads to the contradiction that root 2 is a rational number in the form of p/q with "p and q both co-prime numbers" and q ≠ 0.

Q8: Find the LCM (Least Common Multiple) of 15 and 20.  (2 Marks)

Ans: The LCM of 15 and 20 is 60.
Sol: To find the LCM, we can use the prime factorization method. 
The prime factorization of 15 is 3 x 5, and the prime factorization of 20 is 2 x 2 x 5. 
The LCM is the product of the highest powers of all the prime factors involved, which is 2 x 2 x 3 x 5 = 60.

Q9: Sonia takes 18 minutes to complete one round of a circular track, while Ravi takes 12 minutes for the same. If they start together from the same point, after how many minutes will they meet again at the starting point?  (3 Marks) 

​Ans:

Time taken by Sonia is more than Ravi to complete one round. Now, we have to find after how many minutes will they meet again at the same point. For this, there will be a number that is divisible by both 18 and 12, and that will be the time when both meet again at the starting point. To find this we have to take LCM of both numbers.

Let's find LCM of 18 and 12 by prime factorization method.

18 = 2 × 3 × 3

12 = 2 × 2 × 3

LCM of 12 and 18 = 2 × 2 × 3 × 3 = 36

Therefore, Ravi and Sonia will meet together at the starting point after 36 minutes.

Q10: Find the HCF (Highest Common Factor) of 72 and 96 using the prime factorization method.  (3 Marks) 

Ans: The HCF of 72 and 96 is 24.
To find the HCF, we can use the prime factorization method.
Prime factorization of 72: 72 = 2³ x 3²
Prime factorization of 96: 96 = 2⁵ x 3¹
HCF = Product of the common prime factors with their lowest powers
HCF = 2³ x 3¹ = 8 x 3 = 24

Q11: Check whether 6ⁿ can end with the digit 0 for any natural number n. (3 Marks) 

Ans:  If the number 6ⁿ ends with the digit zero (0), then it should be divisible by 5, as we know any number with a unit place as 0 or 5 is divisible by 5.

Prime factorization of 6ⁿ = (2 × 3)ⁿ

Therefore, the prime factorization of 6ⁿ doesn’t contain the prime number 5.

Hence, it is clear that for any natural number n, 6ⁿ is not divisible by 5 and thus it proves that 6ⁿ cannot end with the digit 0 for any natural number n.

Q12: Given that p is a rational number and q is an irrational number, prove that their sum (p + q) is an irrational number.  (5 Marks) 

Ans:

1. Definitions:

• A rational number p can be expressed as a/b, where a and b are integers and b is not equal to 0.
• An irrational number q cannot be expressed as a fraction of integers.

2. Assumption: Assume for the sake of contradiction that p + q is rational. This means we can express p + q as c/d, where c and d are integers and d is not equal to 0.

3. Rearranging the Equation: From our assumption, we have:
   p + q = c/d
   Rearranging this gives:
   q = c/d - p

4. Substituting the Rational Number: Since p is rational, we can express it as a/b:
   q = c/d - a/b
   To combine these fractions, we find a common denominator:
   q = (cb - ad) / bd

5. Conclusion: Since cb - ad and bd are both integers (as they are products and sums of integers), q can be expressed as a fraction of two integers, which contradicts our original statement that q is irrational.

6. Final Statement: Thus, our assumption that p + q is rational must be false. Therefore, p + q is irrational.

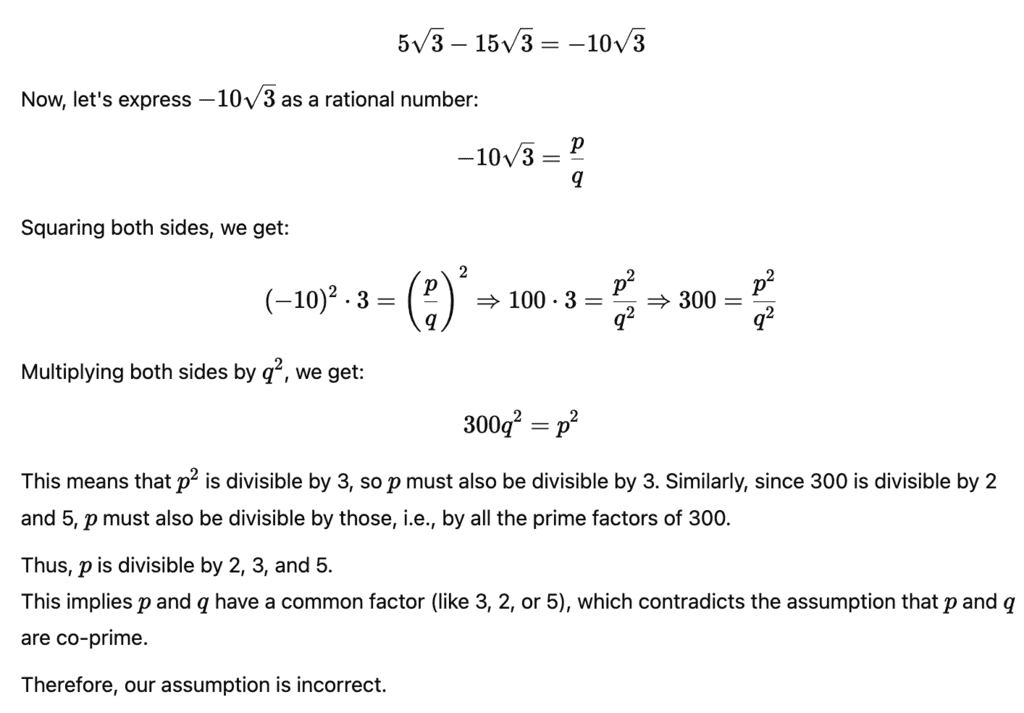
Q13: Prove that 5√3 - 3√75 is an irrational number.  (5 Marks)  

Ans: To prove that 5√3 - 3√75 is an irrational number, we assume the contrary, i.e., let's assume 5√3 - 3√75 is a rational number. 
So, it can be expressed as 5√3 - 3√75 = p/q, where p and q are co-prime integers (i.e., they have no common factors other than 1) and q ≠ 0.
Now, let's work on simplifying the expression:
5√3 - 3√75
Step 1: Factorize the numbers inside the radicals.
√3 cannot be simplified further as it is a prime number.
√75 = √(5 * 5 * 3) = 5√3
Step 2: Substitute the factorized value back into the expression.
5√3 - 3√75 = 5√3 - 3 * 5√3
Step 3: Combine like terms.



Hence, 5√3 - 3√75 is an irrational number.