Simple and Compound Interest: Shortcuts & Tricks | Quantitative Techniques for CLAT PDF Download
Interest rates are highly influential and fascinating mathematical principles that play a central role in our banking and financial sector. Even a small adjustment in these rates can result in profound and remarkable effects on the economy.
Interest and Interest Rates
Interest is the amount charged by the lender from the borrower on the principal loan sum. It is basically the cost of renting money. And, the rate at which interest is charged on the principal sum is known as the interest rate. The rate at which interest is charged depends on two factors
- The value of money doesn’t remain same over time. It changes with time. The net worth of ₹ 100 today will not be same tomorrow i.e. If 5 pens could be bought presently with a INR 100 note then in future, maybe only 4 pens can be bought with the same ₹ 100 note. The reason behind this the inflation or price rise. So, the interest rate includes this factor of inflation
- The credibility of the borrower, if there is more risk and chance of default on borrower’s part then more interest will be charged. And, if there is less chance of payment failure on the part of borrower then the rate of interest would be lower.
The above two reason becomes the basis of why interest rates are so important and have a great effect on markets and economy. Since a minor rise in interest rates increases the cost of borrowing for the borrower and as a result, he has to pay more interest on his loan amount and thus, a decline in his money income that he could spend on other products which create a ripple effect of decreased spending throughout the economy and vice versa. Since change in interest rate has a chain effect in the market, it has a great deal of importance in the study of market, finance, and economy. And that’s why, forms an integral part of the curriculum in the MBA programs. But, a relatively simpler level of questions is asked in the CAT based on the concepts learned at the time of high school.
These concepts are categorized into type of interests
- Simple Interest
- Compound Interest
Let’s first start and understand Simple Interest because as the name suggests it is simple and comparatively easy to comprehend.
Simple interest is that type of interest which once credited does not earn interest on itself. It remains fixed over time.
The formula to calculate Simple Interest is
SI = {(P x R x T)/ 100}
Where, P = Principal Sum (the original loan/ deposited amount)
R = rate of interest (at which the loan is charged)
T = time period (the duration for which money is borrowed/ deposited)
So, if P amount is borrowed at the rate of interest R for T years then the amount to be repaid to the lender will be
A = P + SI
Consider a basic example of SI to understand the application of above formula such as Find the simple interest on ₹ 68000 at 16 2/3 % p.a. for 9 months.
Here, P = ₹68000
R = 162/3 % = 50/3% p.a.
T = 9 months = 9/12 years = ¾ years
SI = (68000 x 50/3 x ¾ x 1/100) = ₹8500
Some useful results based on Simple Interest
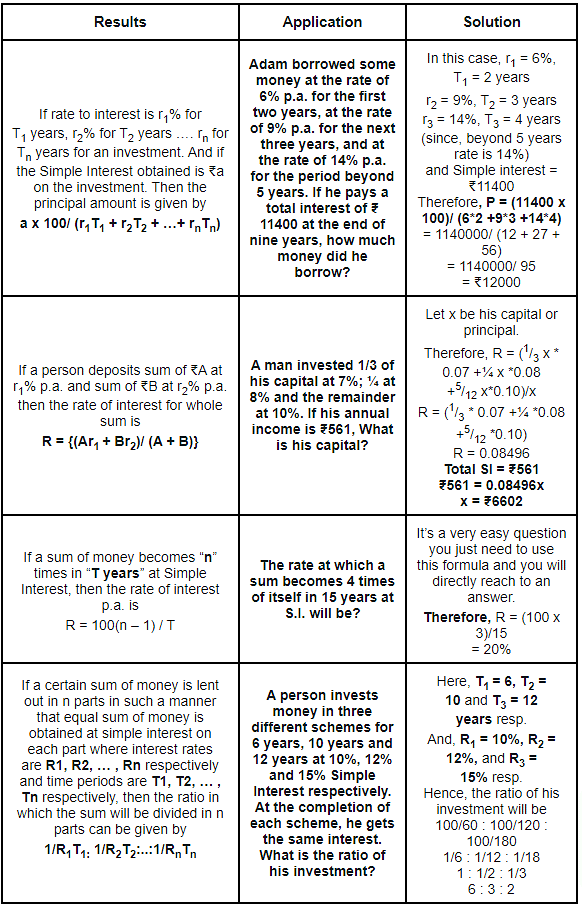
Compound Interest
This the most usual type of interest that is used in the banking system and economics. In this kind of interest along with one principal further earns interest on it after the completion of 1-time period. Suppose an amount P is deposited in an account or lent to the borrower that pays compound interest at the rate of R% p.a. Then after n years the deposit or loan will accumulate to:
P(1+R/100)n
Consider this example, if an amount of 100 is deposited in saving bank account for 3 years at the interest rate of 6% p.a. Then, after one year the ₹100 will accumulate to ₹106. Since in compound interest, interest itself earns interest, therefore, after 1-year interest for the 2nd will be calculated on ₹106 unlike to that of Simple interest where interest will be calculated on ₹100 only. Thus, after the end of the third year the total amount will become ₹100(1.06)3 = ₹119.1016.
IMPORTANT FORMULAS
- When the interest is compounded Annually:
Amount= P (1 + R/100) n
- When the interest is compounded Half-yearly:
Amount = P (1 + (R/2)/100)2n
- When the interest is compounded Quarterly:
Amount = P (1 + (R/4)/100)4n
- When the rates are different for different years, say R1%, R2% and R3% for 1 year, 2 years and 3-year resp. Then,
Amount = P (1 + R1/100) (1 + R2/100) (1 + R3/100)
- Present worth of ₹ x due n years hence is given by:
Present worth = x/ (1 + R/100)n
- If a certain sum becomes “x” times in n years, then the rate of compound interest will be
R = 100(x1/n – 1)
- If a sum of money P amounts to A1 after T years at CI and the same sum of money amounts to A2 after (T + 1) years at CI, then
R = (A2 – A1)/ A1 x 100
Miscellaneous Examples of application of Compound Interest
Question 1: A man invests ₹ 5000 for 3 years at 5% p.a. compounded interest reckoned yearly. Income tax at the rate of 20% on the interest earned is deducted at the end of each year. Find the amount at the end of third year.
Sol: Here, P = ₹5000, T = 3 years, r = 5%
Therefore, Interest at the end of 1st year = 5000 (1 + 0.05) – 5000 = ₹250
Now Income tax is 20% on the interest income so the leftover interest income after deducing income
tax = (1 – 0.2) * 250 = ₹200
Total Amount at the end of 1st year = ₹5000 + 200 = ₹5200
Interest at the end of 2nd year = 5200 (1 + 0.05) – 5200 = ₹260
Interest income after Income tax = 0.8 * ₹260 = ₹208
Total Amount at the end of 2nd year = ₹5200 + 208 = ₹5408
Interest at the end of 3rd year = ₹5408 (1.05) – 5408 = ₹270.4
Interest income after Income tax = 0.8 * ₹270.4 = ₹216.32
Total Amount at the end of 2rd year = ₹5408 + 216.32 = ₹5624.32
Question 2: A sum of ₹12000 deposited at compound interest becomes double after 5 years. After 20 years, it will become?
Sol: The rate of interest at which ₹12000 doubles after 5 years is given by
R = 100(x1/n – 1)
= 100(21/5 – 1)
=100 x (1.1486 – 1)
= 100 x 0.1486 = 14.86%
Therefore, after 20 years it becomes,
A = ₹12000(1 + 14.86/100)20
= ₹12000 (1.1486)20
= ₹12000 x 15.97
= ₹ 191671.474
Compound Interest Installments
Let a person takes a loan from bank at r% and agrees to pay loan in equal installments for n years. Then, the value of each installment is given by
P = X/ (1 + r/100)n………X/ (1 + r/100)2 + X/ (1 + r/100)
For better understanding, let’s understand with the help of example.
One can purchase a flat from a house building society for ₹ 55000 or on the terms that he should pay ₹ 4275 as cash down payment and the rest in three equal installments. The society charges interest at the rate 16% p.a. compounded half-yearly. If the flat is purchased under installment plan, find the value of each installment.
Sol: The cost of the flat is ₹ 55000. Now, if the person could either buy flat by paying ₹55000 or through installment plan. Since the flat was purchased through installment plan then the loan amount = ₹55000 – 4275 (down payment) = ₹50725.
Here r = 16% compounded Half-yearly in 3 equal instalments. Let x be the amount of installment. Then,
₹50725 = x/ (1 + 16/200)3 + x/ (1 + 16/200)2 + x/ (1 + 16/200)
₹50725 = x (1/1.2591 + 1/1.1664 + 1/1.08)
₹50725 = x (0.79421 + 0.85722 + 0.9259)
₹50725 = x (2.577)
₹50725/2.5777 = x
x = ₹19683
|
49 videos|155 docs|73 tests
|
FAQs on Simple and Compound Interest: Shortcuts & Tricks - Quantitative Techniques for CLAT
| 1. What is the difference between simple interest and compound interest? |  |
| 2. How is simple interest calculated? |  |
| 3. How is compound interest calculated annually? |  |
| 4. Are there any shortcuts or tricks to calculate compound interest quickly? |  |
| 5. How can I compare different investment options with different interest rates and compounding frequencies? |  |















