Particle in a One-Dimensional Box | Chemistry Optional Notes for UPSC PDF Download
Introduction
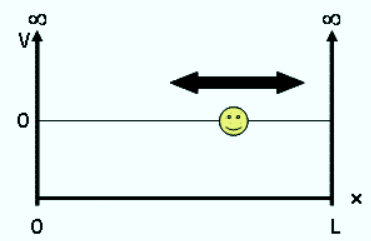
The particle in a box problem is a common application of a quantum mechanical model to a simplified system consisting of a particle moving horizontally within an infinitely deep well from which it cannot escape. The solutions to the problem give possible values of E and ψ that the particle can possess. E represents allowed energy values and ψ(x) is a wavefunction, which when squared gives us the probability of locating the particle at a certain position within the box at a given energy level.
Step 1: Define the Potential Energy V
The potential energy is 0 inside the box (V = 0 for 0 < x < L) and goes to infinity at the walls of the box (V = ∞ for x < 0 or x > L). We assume the walls have infinite potential energy to ensure that the particle has zero probability of being at the walls or outside the box. Doing so significantly simplifies our later mathematical calculations as we employ these boundary conditions when solving the Schrödinger Equation.
Step 2: Solve the Schrödinger Equation
The time-independent Schrödinger equation for a particle of mass m moving in one direction with energy E is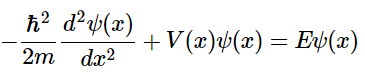 (4.8.1)
(4.8.1)
with
- ℏ is the reduced Planck constant where ℏ = h/2π
- m is the mass of the particle
- ψ(x) is the stationary time-independent wavefunction
- V(x) is the potential energy as a function of position
- E is the energy, a real number
This equation can be modified for a particle of mass m free to move parallel to the x-axis with zero potential energy (V = 0 everywhere) resulting in the quantum mechanical description of free motion in one dimension:
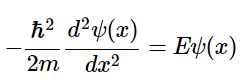 (4.8.2)
(4.8.2)
This equation has been well studied and gives a general solution of:
ψ(x) = Asin (kx) + Bcos (kx) (4.8.3)
where A, B, and k are constants.
Step 3: Define the Wavefunction
The solution to the Schrödinger equation we found above is the general solution for a 1-dimensional system. We now need to apply our boundary conditions to find the solution to our particular system. According to our boundary conditions, the probability of finding the particle at x = 0 or x = L is zero. When x = 0, then sin(0) = 0 and cos(0) = 1; therefore, B must equal 0 to fulfill this boundary condition giving:
ψ(x) = Asin (kx) (4.8.4)
We can now solve for our constants (A and k) systematically to define the wave function.
Solving for k
Differentiate the wavefunction concerning x:
dψ/dx = kA cos (kx) (4.8.5)
Differentiate the wavefunction again concerning x: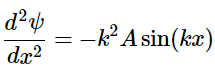 (4.8.6)
(4.8.6)
Since ψ(x) = A sin (kx), then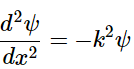 (4.8.7)
(4.8.7)
If we then solve for k by comparing with the Schrödinger equation above, we find: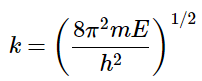 (4.8.9)
(4.8.9)
Solving for A
To determine A, we have to apply the boundary conditions again. Recall that the probability of finding a particle at x = 0 or x = L is zero.
When x = L: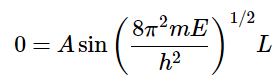 (4.8.10)
(4.8.10)
This is only true when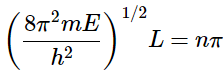 (4.8.11)
(4.8.11)
where n = 1,2,3,…
Plugging this back in gives us: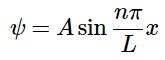 (4.8.12)
(4.8.12)
To determine A, recall that the total probability of finding the particle inside the box is 1, meaning there is no probability of it being outside the box. When we find the probability and set it equal to 1, we are normalizing the wavefunction.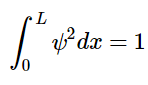 (4.8.13)
(4.8.13)
For our system, the normalization looks like: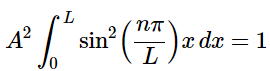 (4.8.14)
(4.8.14)
Using the solution for this integral from an integral table, we find our normalization constant, A: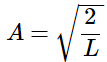 (4.8.15)
(4.8.15)
Which results in the normalized wavefunctions for a particle in a 1-dimensional box: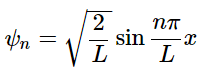 (4.8.16)
(4.8.16)
where n=1,2,3,…
Step 4: Determine the Allowed Energies
Solving for the energy of each ψ requires substituting Equation 4.8.16 into Equation 4.8.2 to get the allowed energies for a particle in a box: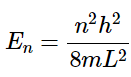 (4.8.17)
(4.8.17)
Equation 4.8.17 is a very important result and tells us that:
- The energy of a particle is quantized.
- The lowest possible energy of a particle is NOT zero. This is called the zero-point energy and means the particle can never be at rest because it always has some kinetic energy.
This is also consistent with the Heisenberg Uncertainty Principle: if the particle had zero energy, we would know where it was in both space and time.
What does all this mean?
The wavefunction for a particle in a box at the n = 1 and n = 2 energy levels look like this:
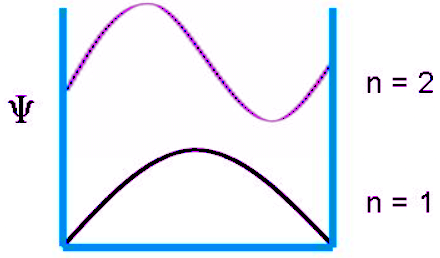
The probability of finding a particle a certain spot in the box is determined by squaring ψ. The probability distribution for a particle in a box at the n = 1 and n = 2 energy levels looks like this: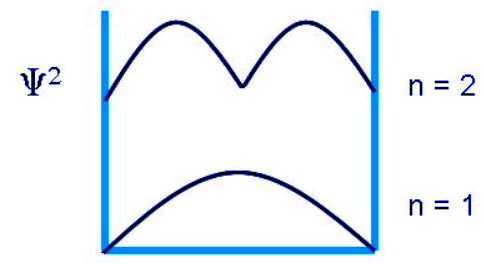 Notice that the number of nodes (places where the particle has zero probability of being located) increases with increasing energy n. Also note that as the energy of the particle becomes greater, the quantum mechanical model breaks down as the energy levels get closer together and overlap, forming a continuum. This continuum means the particle is free and can have any energy value. At such high energies, the classical mechanical model is applied as the particle behaves more like a continuous wave. Therefore, the particle in a box problem is an example of Wave-Particle Duality.
Notice that the number of nodes (places where the particle has zero probability of being located) increases with increasing energy n. Also note that as the energy of the particle becomes greater, the quantum mechanical model breaks down as the energy levels get closer together and overlap, forming a continuum. This continuum means the particle is free and can have any energy value. At such high energies, the classical mechanical model is applied as the particle behaves more like a continuous wave. Therefore, the particle in a box problem is an example of Wave-Particle Duality.
Solved Example
Example: What is the ΔE between the n = 4 and n = 5 states for an F2 molecule trapped within in a one-dimension well of length 3.0 cm? At what value of n does the energy of the molecule reach ¼kBT at 450 K, and what is the separation between this energy level and the one immediately above it?
Ans: Since this is a one-dimensional particle in a box problem, the particle has only kinetic energy (V = 0), so the permitted energies are: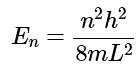
with n = 1,2,... The energy difference between n = 4 and n = 5 is then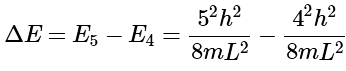
Using Equation 4.8.17 with the mass of F2 (37.93 amu = 6.3 × 10−26kg) and the length of the box (L = 3 × 3.0 × 10−2m2: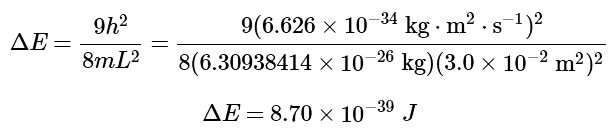
The n value for which the energy reaches 1/4kBT: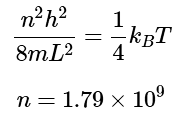
The separation between n + 1 and n: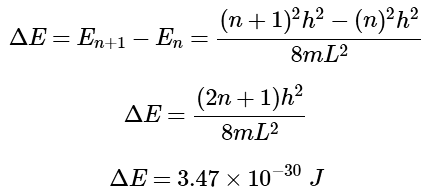
Important Facts to Learn from the Particle in the Box
- The energy of a particle is quantized. This means it can only take on discreet energy values.
- The lowest possible energy for a particle is NOT zero (even at 0 K). This means the particle always has some kinetic energy.
- The square of the wavefunction is related to the probability of finding the particle in a specific position for a given energy level.
- The probability changes with increasing energy of the particle and depends on the position in the box you are attempting to define the energy for
- In classical physics, the probability of finding the particle is independent of the energy and the same at all points in the box.
FAQs on Particle in a One-Dimensional Box - Chemistry Optional Notes for UPSC
| 1. What is a particle in a one-dimensional box? |  |
| 2. What is the significance of a particle in a one-dimensional box? |  |
| 3. How is the energy of a particle in a one-dimensional box determined? |  |
| 4. What are the boundary conditions for a particle in a one-dimensional box? |  |
| 5. What are some real-life applications of the concept of a particle in a one-dimensional box? |  |






















