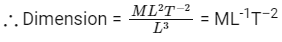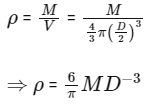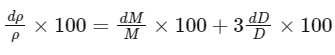[JEE Mains MCQs]
Q1: A student measuring the diameter of a pencil of circular cross-section with the help of a vernier scale records the following four readings 5.50 mm, 5.55 mm, 5.45 mm, 5.65 mm. The average of these four readings is 5.5375 mm and the standard deviation of the data is 0.07395 mm. The average diameter of the pencil should therefore be recorded as :
(a) (5.54 ± 0.07) mm
(b) (5.5375 ± 0.0740) mm
(c) (5.5375 ± 0.0739) mm
(d) (5.538 ± 0.074) mm
Ans: (a)
Given, dav = 5.5375 mm
Δ d = 0.07395 mm
Significant rule says that reading should has same significant figure as that of reading given.
∵ Measured data are up to two digits after decimal.
∴ 5.5375 rounded to → 5.54
Q2: A screw gauge has 50 divisions on its circular scale. The circular scale is 4 units ahead of the pitch scale marking, prior to use. Upon one complete rotation of the circular scale, a displacement of 0.5mm is noticed on the pitch scale. The nature of zero error involved and the least count of the screw gauge, are respectively :
(a) Positive, 0.1 mm
(b) Positive, 0.1 μm
(c) Positive, 10 μm
(d) Negative, 2 μm
Ans: (c)
Least count of screw gauge
= 0.5/50
= 1 × 10-5 m
= 10 μm Zero error in positive.
Q3: The quantities  defined where C-capacitance, R-Resistance, l-length, E-Electric field, B-magnetic field and ε0 , μ0 , - free space permittivity and permeability respectively. Then:
defined where C-capacitance, R-Resistance, l-length, E-Electric field, B-magnetic field and ε0 , μ0 , - free space permittivity and permeability respectively. Then:
(a) Only y and z have the same dimension
(b) x, y and z have the same dimension
(c) Only x and y have the same dimension
(d) Only x and z have the same dimension
Ans: (b)

So, x, y, z all have the same dimensions.
Q4: A physical quantity z depends on four observables a, b, c and d, as  The percentages of error in the measurement of a, b, c and d are 2%, 1.5%, 4% and 2.5% respectively. The percentage of error in z is :
The percentages of error in the measurement of a, b, c and d are 2%, 1.5%, 4% and 2.5% respectively. The percentage of error in z is :
(a)13.5 %
(b) 14.5%
(c)16.5%
(d) 12.25%
Ans: (b)


% error in z

= (4 + 1 + 2 + 7.5) %
= 14.5 %
Q5: A quantity x is given by  in terms of moment of inertia I, force F, velocity v, work W and Length L. The dimensional formula for x is same as that of :
in terms of moment of inertia I, force F, velocity v, work W and Length L. The dimensional formula for x is same as that of :
(a) Coefficient of viscosity
(b) Force constant
(c) Energy density
(d) Planck's constant
Ans: (c)


= [ML-1T-2]
= [Energy density]
Q6: Dimensional formula for thermal conductivity is (here K denotes the temperature):
(a) MLT–3K–1
(b) MLT–2K–2
(c) MLT–2K
(d) MLT–3K
Ans: (a)

Q7: Amount of solar energy received on the earth’s surface per unit area per unit time is defined a solar constant. Dimension of solar constant is :
(a) MLT–2
(b) ML0T–3
(c) M2L0T–1
(d) ML2T–2
Ans: (b)
Solar constant = E/AT

= [ ML0T–3]
Q8: Using screw gauge of pitch 0.1 cm and 50 divisions on its circular scale, the thickness of an object is measured. It should correctly be recorded as
(a) 2.123 cm
(b) 2.124 cm
(c) 2.125 cm
(d) 2.121 cm
Ans: (b)
Using a screw gauge of pitch 0.1 cm and 50 divisions on its circular scale, the thickness of an object is measured as:
Measurement = (Main scale reading) + (Circular scale reading × Least count)
where the least count is calculated as the pitch of the screw gauge divided by the number of divisions on the circular scale:
Least count = (Pitch of screw gauge) / (Number of circular scale divisions)
Least count = 0.1/50 = 0.002 cm
Now if we multiply division of circular scale with least count then we get 0th digit of fraction part even.
Here only option B has 0th digit of fraction part even.
Q9: If momentum (P), area (A) and time (T) are taken to be the fundamental quantities then the dimensional formula for energy is
(a) [P2AT-2]
(b) 
(c) 
(d) [PA–1T–2]
Ans: (c)
Let [E] = K[P]x[A]y [T]z
[ML2T–2] = [MLT–1]x[L2]y[T]z
[ML2T–2] = [Mx][Lx+2y][T–x+z]
Comparing both side we get,
x = 1
x + 2y = 2
⇒ 1 + 2y= 2 or y = 1/2
z – x = –2 ⇒ z–1 = –2 or z = –1

Q10: If speed V, area A and force F are chosen as fundamental units, then the dimension of Young’s modulus will be
(a) FA–1V0
(b) FA2V–1
(c) FA2V–2
(d) FA2V–3
Ans: (a)
Y = k [F]x [A]y [V]z
[M1L1T –2] = [MLT–2]x [L2]y [LT–1]z
[M1L1T –2] = [M]x [L]x+2y+z[T]–2x–z
Comparing power of M, L and T
x = 1 ……(1)
x + 2y + z = –1 ……(2)
–2x – z = –2 ……(3)
After solving
x = 1
y = –1
z = 0
∴ Y = FA–1V0
Q11: The least count of the main scale of a vernier callipers is 1 mm. Its vernier scale is divided into 10 divisions and coincide with 9 divisions of the main scale. When jaws are touching each other, the 7th division of vernier scale coincides with a division of main scale and the zero of vernier scale is lying right side of the zero of main scale. When this vernier is used to measure length of a cylinder the zero of the vernier scale between 3.1 cm and 3.2 cm and 4th VSD coincides with a main scale division. The length of the cylinder is : (VSD is vernier scale division)
(a) 3.21 cm
(b) 2.99 cm
(c) 3.07 cm
(d) 3.2 cm
Ans: (c)
Least count = 1 mm or 0.01 cm
Zero error = 0 + 0.01 × 7 = 0.07 cm
Reading = 3.1 + (0.01 × 4) – 0.07
= 3.1 + 0.04 – 0.07
= 3.1 – 0.03
= 3.07 cm
Q12: For the four sets of three measured physical quantities as given below. Which of the following options is correct ?
(i) A1 = 24.36, B1 = 0.0724, C1 = 256.2
(ii) A2 = 24.44, B2 = 16.082, C2 = 240.2
(iii) A3 = 25.2, B3 = 19.2812, C3 = 236.183
(iv) A4 = 25, B4 = 236.191, C4 = 19.5
(a) A1 + B1 + C1 = A2 + B2 + C2 = A3 + B3 + C3 = A4 + B4 + C4
(b) A4 + B4 + C4 < A1 + B1 + C1 < A3 + B3 + C3 < A2 + B2 + C2
(c) A4 + B4 + C4 < A1 + B1 + C1 = A2 + B2 + C2 = A3 + B3 + C3
(d) A4 + B4 + C4 > A3 + B3 + C3 = A2 + B2 + C2 > A1 + B1 + C1
Ans: (d)
A1 + B1 + C1 = 24.36 + 0.0724 + 256.2
= 280.6324 = 280.6 (After rounding off)
A2 + B2 + C2 = 24.44 + 16.082 + 240.2
= 280.722 = 280.7 (After rounding off)
A3 + B3 + C3 = 25.2 + 19.2812 + 236.183
= 280.6642 = 280.7 (After rounding off)
A4 + B4 + C4 = 25 + 236.191 + 19.5
= 280.691 = 281 (After rounding off)
Q13: A quantity f is given by  where c is speed of light, G universal gravitational constant and h is the Planck's constant. Dimension of f is that of :
where c is speed of light, G universal gravitational constant and h is the Planck's constant. Dimension of f is that of :
(a) Energy
(b) Momentum
(c) Area
(d) Volume
Ans: (a)
[h] = M1L2T–1
[C] = L1T–1
[G] = M–1L3T–2

Q14: If the screw on a screw-gauge is given six rotations, it moves by 3 mm on the main scale. If there are 50 divisions on the circular scale the least count of the screw gauge is :
(a) 0.001 mm
(b) 0.01 cm
(c) 0.02 mm
(d) 0.001 cm
Ans: (d)
Pitch = 3/6 mm = 0.5 mm
Least count = 0.5/50 mm
= 1/100 = 0.01 mm = 0.001 cm
Q15: The dimension of stopping potential V0 in photoelectric effect in units of Planck's constant 'h', speed of light 'c' and Gravitational constant 'G' and ampere A is :
(a) h1/3 G2/3 c1/3 A–1
(b) h0 c5 G-1 A-1
(c) h2/3 c5/3 G1/3 A–1
(d) h2 G3/2 c1/3 A–1
Ans: (b)
V0 ∝ hPcQGRIS
[V0] = [M1L2T–3A–1]
[c] = [L1T–1]
[h] = [M1L2T–1]
[G] = [M–1L3T–2]
[I] = [A]
∴ [M1L2T–3A–1] = [MP–R L2P+Q+3R T–P–Q–2R AS]
Comparing dimension of M, L, T, A, we get
P – R = 1 ; 2P + Q + 3R = 2
– P – Q – 2R = – 3 ; S = – 1
⇒ P = 0, Q = 5, R = –1, S = –1
∴ V0 ∝ h0 c5 G-1 A-1
Q16: The dimension of  where B is magnetic field and μ0 is the magnetic permeability of vacuum, is :
where B is magnetic field and μ0 is the magnetic permeability of vacuum, is :
(a) ML2T–2
(b) MLT–2
(c) ML-1T–2
(d) ML2T–1
Ans: (c)
As  = Energy per unit volume
= Energy per unit volume

[JEE Mains Numericals]
Q17: The density of a solid metal sphere is determined by measuring its mass and its diameter. The maximum error in the density of the sphere is (x/100) %. If the relative errors in measuring the mass and the diameter are 6.0% and 1.5% respectively, the value of x is_______.
Ans: 1050

For maximum error

= 6 + 3 × 1.5
= 10.5 %
= (1050/100) %
∴ x = 1050
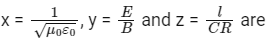 defined where C-capacitance, R-Resistance, l-length, E-Electric field, B-magnetic field and ε0 , μ0 , - free space permittivity and permeability respectively. Then:
defined where C-capacitance, R-Resistance, l-length, E-Electric field, B-magnetic field and ε0 , μ0 , - free space permittivity and permeability respectively. Then: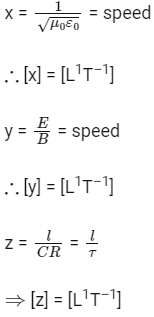
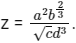 The percentages of error in the measurement of a, b, c and d are 2%, 1.5%, 4% and 2.5% respectively. The percentage of error in z is :
The percentages of error in the measurement of a, b, c and d are 2%, 1.5%, 4% and 2.5% respectively. The percentage of error in z is :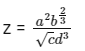
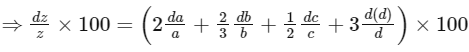
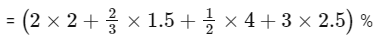
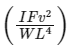 in terms of moment of inertia I, force F, velocity v, work W and Length L. The dimensional formula for x is same as that of :
in terms of moment of inertia I, force F, velocity v, work W and Length L. The dimensional formula for x is same as that of :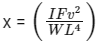
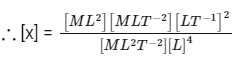
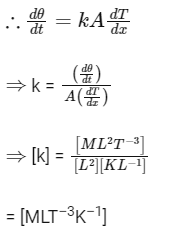
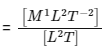
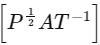
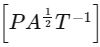
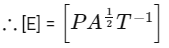
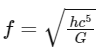 where c is speed of light, G universal gravitational constant and h is the Planck's constant. Dimension of f is that of :
where c is speed of light, G universal gravitational constant and h is the Planck's constant. Dimension of f is that of :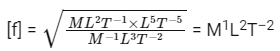
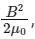 where B is magnetic field and μ0 is the magnetic permeability of vacuum, is :
where B is magnetic field and μ0 is the magnetic permeability of vacuum, is :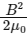 = Energy per unit volume
= Energy per unit volume