| Table of contents |

|
| Viscosity |

|
| Stoke's Law |

|
| Intermolecular Forces |

|
| Surface Tension |

|
| Capillarity |

|
Viscosity
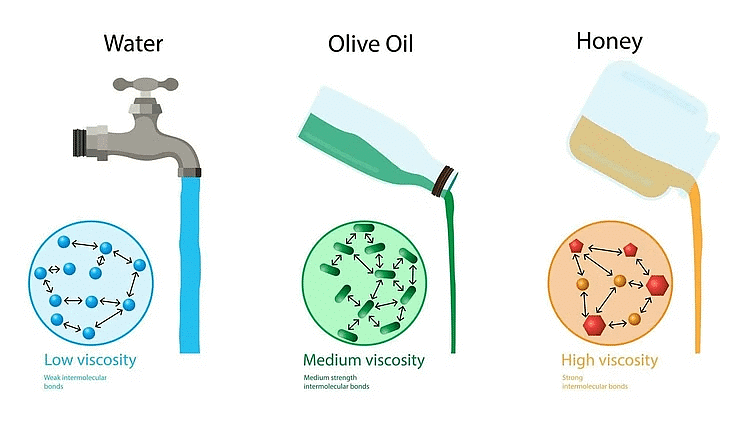
Most fluids don't flow easily and offer some resistance when you try to move them. This resistance is called viscosity, which is like internal friction in solids moving on a surface. Imagine two glass plates with oil between them. If you move the top plate, it requires less force than if you replace the oil with honey. So, we say honey is more viscous than oil. When fluid layers move relative to each other, it creates forces between them, known as laminar flow.
 Viscosity Examples
Viscosity Examples
When fluid flows in a pipe, the layer along the pipe's axis has maximum velocity, decreasing towards the walls where it becomes zero. As the fluid flows, it undergoes shear strain, and its viscosity is the ratio of shearing stress to strain rate. 
As the liquid moves, a part of it, initially shaped as ABCD, changes to AEFD after a short time (∆t). In this time, the liquid experiences shear strain (∆x/l). Unlike solids, where stress depends on strain, in flowing fluids, stress is determined by the 'rate of change of strain' or 'strain rate,' represented as ∆x/(l∆t) or v/l instead of the strain itself. The viscosity of a fluid, denoted by the symbol 'eta' (η), is defined as the ratio of shearing stress to the strain rate, expressed as
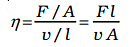
The unit of viscosity is poiseiulle (Pl), with dimensions [ML-1T-1]. Thinner liquids like water are less viscous than thicker ones like blood. Blood remains more viscous than water, and its relative viscosity is constant between 0oC and 37oC.
Generally, the viscosity of liquids decreases with temperature, while for gases, it increases.
Ex. A metal plate of area 2.5×10-4m2 is placed on a 0.25×10-3m thick layer of castor oil. If a force of 2.5 N is needed to move the plate with a velocity 3×10-2m s-1, calculate the coefficient of viscosity of castor oil. Given: A=2.5×10-4 m2, dx = 0.25×10-3m, F=2.5N and dv = 3×10-2 m s-1.
Solution:
Stoke's Law
When an object falls through a fluid, it pulls along the fluid close to it, creating a relative motion between different fluid layers. This results in a retarding force acting on the falling object. Examples of this include raindrops falling or a pendulum bob swinging. The viscous force that slows down the object is proportional to its velocity and opposite to its direction of motion.
Sir George G. Stokes explained this viscous drag force with an equation known as Stokes' law:
F=6πηav
This law demonstrates how the retarding force is related to the viscosity (η) of the fluid and the radius (a) of the object.
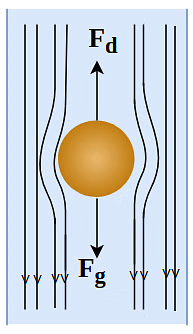 Stoke's Law Representation
Stoke's Law Representation
Stokes' law has interesting consequences for an object, like a raindrop falling through a viscous medium. Initially, the object accelerates due to gravity. As its velocity increases, the retarding force also increases. Eventually, when the combined effect of viscous force and buoyant force equals the force due to gravity, the net force becomes zero, and the object reaches a constant velocity called the terminal velocity (vt). The formula for terminal velocity is given by
6πηavt = (4π/3)a3(ρ-σ)g
vt = 2a(ρ - σ)g/9η
where ρ and σ are the mass densities of the sphere and the fluid, respectively. This shows that the terminal velocity depends on the square of the sphere's radius and inversely on the viscosity of the medium.
Intermolecular Forces
Intermolecular forces are the interactions between molecules in a substance, and they can be divided into two types:
1. Cohesive Force: This is the force that attracts molecules of the same substance to each other. For example, water molecules stick together, forming droplets due to cohesive forces.
2. Adhesive Force: This force occurs between molecules of different substances. For instance, water sticks to a glass surface because of adhesive forces.
Both cohesive and adhesive forces are attractive forces and are most effective within a range of 10-9 meters to 1 angstrom.

Surface Tension
Surface tension is a property of liquids where the free surface, when at rest, behaves like a stretched membrane trying to minimize its surface area. It is a force per unit length acting in the plane of the liquid's surface. This extra energy at the surface is compared to the interior of the liquid.
Surface tension (T) can be expressed as T = F/l, where T is surface tension, F is the force acting on the surface (cohesive force), and l is the unit length.
Surface tension allows insects to float on water and enables a needle to float on a liquid surface. Some applications of surface tension include the ease of spreading cleaning agents like Dettol and toothpaste, the floating of mosquito eggs and water spiders, and the enhanced cleaning ability of soap due to reduced surface tension compared to water.
:max_bytes(150000):strip_icc()/139802493-56a12f615f9b58b7d0bcde91.jpg) Example of Surface Tension
Example of Surface Tension
Surface Energy
Surface energy is the potential energy of molecules on the surface of a substance. It is calculated by multiplying the surface tension (T) of the liquid by the increase in surface area (ΔA). The formula is given as
Surface Energy = T × ΔA.
 |
Download the notes
Viscosity and Surface Tension
|
Download as PDF |
Angle of Contact
When a liquid meets another medium, the surface near the contact point is usually curved. The angle (θ) between the tangent to the liquid surface at the contact point and the solid surface inside the liquid is called the angle of contact.
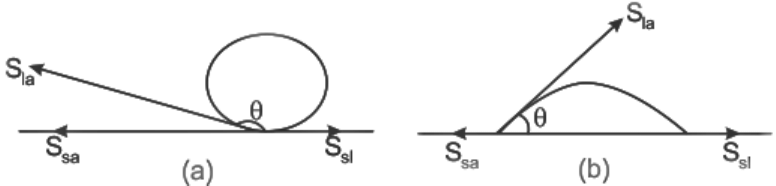 Angle of Contact
Angle of Contact
It's essential to measure this angle through the liquid surface.
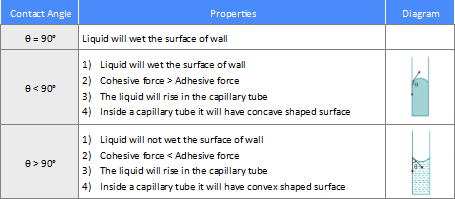 Different Angle of Contacts
Different Angle of Contacts
Capillarity
Capillarity refers to the rising and falling of liquid inside a hollow cylindrical glass tube called a capillary tube. The height to which the liquid rises depends on the atmospheric pressure outside the tube, the liquid pressure inside the tube, the surface tension (T), the density of the liquid (ρ), the angle of contact (θ), and the radius of the capillary tube (a).
The formula for the rise of liquid is given as
h = Tcosθρga.
Applications of Capillarity
Capillarity has practical applications, such as filling ink in fountain pens, the upward movement of water in plant roots, and the rise of oil in lamp wicks. These phenomena occur due to the capillary action governed by the mentioned factors.






















