Best Study Material for UPSC Exam
UPSC Exam > UPSC Notes > Management Optional Notes for UPSC > Sampling Distributions
Sampling Distributions | Management Optional Notes for UPSC PDF Download
| Table of contents |
|
| Introduction |
|
| Properties of the Sampling Distribution of the Mean |
|
| Applications of Sampling Distribution |
|
| Conclusion |
|
Introduction
- Sampling distribution, also known as finite-sample distribution, represents the probability distribution of a specific statistic derived from a random sample. These distributions hold significant importance in statistics as they simplify the process of drawing statistical inferences. Essentially, they allow analytical considerations to be based on the sampling distribution of a statistic rather than the joint probability distribution of all individual sample values.
- In essence, the sampling distribution emerges from the collection of real data. An essential characteristic of a sample is its finite nature, denoted by the countable number of scores represented by the letter 'n'. The value of a statistic varies across different samples, rendering it a random variable. Consequently, the probability distribution associated with it is referred to as its sampling distribution.
For example, consider the following dataset that contributes to the development of a sampling distribution:
15 14 15 18 15 20 15 16 17 14 17 13 11 14 18 12 17 12 21 8 14 17 14 12 13 15 15 16 17 14 16 13 14 15 18 16 16 17 14 15 16 15 17 12 14 14 13 13 13 14
This dataset serves as a basis for analyzing the sampling distribution.
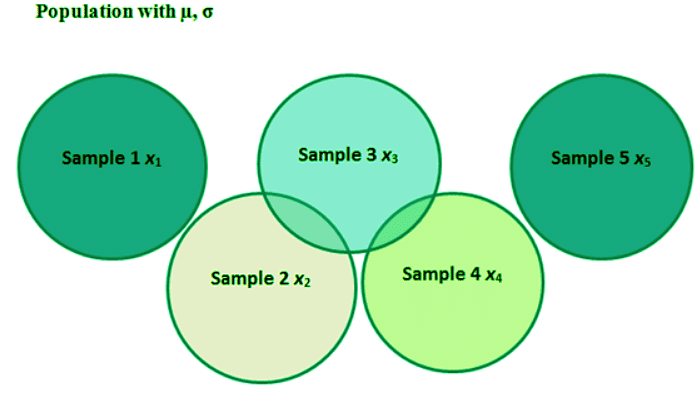
In the given illustration, the rectangle symbolizes a sizable population, with each circle depicting a sample of size 'n'. Since the entries in each sample may vary, the sample means can also exhibit variations. For instance, the mean of Sample 1 is denoted as 'x1', Sample 2 as 'x2', and so forth. The sampling distribution, comprising the sample means of size 'n' for this population, encompasses 'x1', 'x2', 'x3', and beyond. Notably, if the samples are drawn with replacement, an infinite number of samples can be derived from the population.
Question for Sampling Distributions
Try yourself:
What is the sampling distribution?View Solution
Properties of the Sampling Distribution of the Mean
- Unbiasedness: The mean of the sampling distribution equals the population mean.
- Efficiency: The sample mean tends to approach the population mean more closely than any other unbiased estimator.
- Consistency: As the sample size increases, the variability of the sample mean from the population mean decreases.
Applications of Sampling Distribution
- Sampling distributions, such as the normal distribution, serve as descriptive models and are utilized to depict real-world scenarios.
- They are invaluable for making probabilistic statements about specific observations.
- Investigators, researchers, and modelers employ sampling distributions for estimation and hypothesis testing.
 |
Download the notes
Sampling Distributions
|
Download as PDF |
Download as PDF
Conclusion
Sampling involves selecting observations from a larger group or population. The sampling distribution is characterized as the frequency distribution of a statistic across numerous samples. Specifically, it pertains to the distribution of means and is often referred to as the sampling distribution of the mean. This theoretical distribution of a sample statistic varies depending on the specific statistic being analyzed and is characterized by parameters, including µ (the population mean) and σ (the standard error). Notably, the sampling distribution of the mean is a special case within the broader context of sampling distributions.
Question for Sampling Distributions
Try yourself:
Which property of the sampling distribution of the mean states that as the sample size increases, the variability of the sample mean from the population mean decreases?View Solution
The document Sampling Distributions | Management Optional Notes for UPSC is a part of the UPSC Course Management Optional Notes for UPSC.
All you need of UPSC at this link: UPSC
FAQs on Sampling Distributions - Management Optional Notes for UPSC
| 1. What are the properties of the sampling distribution of the mean? |  |
| 2. What are some applications of the sampling distribution? | |
Ans. The sampling distribution has several applications, including:
- Estimating population parameters: By using the sampling distribution of the mean, we can estimate the population mean by calculating the mean of the sample.
- Hypothesis testing: The sampling distribution helps in hypothesis testing by comparing the sample mean to the population mean and determining if there is a significant difference.
- Confidence intervals: The sampling distribution is used to calculate confidence intervals, which provide a range of values within which the population mean is likely to fall.
- Quality control: Sampling distributions are used in quality control to monitor and improve processes by taking samples, calculating means, and comparing them to target values.
- Predictive modeling: In predictive modeling, sampling distributions are used to estimate the uncertainty and variability in the predictions made by the model.
| 3. What is the mean of the sampling distribution of the mean? | |
Ans. The mean of the sampling distribution of the mean is equal to the population mean. This means that if we take multiple random samples of the same size from a population and calculate the mean of each sample, the average of those sample means will be equal to the true population mean.
| 4. How does the sample size affect the sampling distribution of the mean? | |
Ans. The sample size has a significant effect on the sampling distribution of the mean. As the sample size increases, the sampling distribution becomes more closely approximated by a normal distribution, regardless of the shape of the population distribution. The standard deviation of the sampling distribution, also known as the standard error, decreases as the sample size increases. This means that larger sample sizes result in more precise estimates of the population mean.
| 5. What is the standard deviation of the sampling distribution of the mean? | |
Ans. The standard deviation of the sampling distribution of the mean, also known as the standard error, is equal to the population standard deviation divided by the square root of the sample size. It represents the average amount of variation or spread in the sample means. A smaller standard deviation of the sampling distribution indicates less variability in the sample means and a more precise estimate of the population mean.
Related Exams
About this Document
Apr 21, 2025
Last updated
Document Description: Sampling Distributions for UPSC 2025 is part of Management Optional Notes for UPSC preparation.
The notes and questions for Sampling Distributions have been prepared according to the UPSC exam syllabus. Information about Sampling Distributions covers topics
like Introduction, Properties of the Sampling Distribution of the Mean, Applications of Sampling Distribution, Conclusion and Sampling Distributions Example, for UPSC 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Sampling Distributions.
Introduction of Sampling Distributions in English is available as part of our Management Optional Notes for UPSC
for UPSC & Sampling Distributions in Hindi for Management Optional Notes for UPSC course.
Download more important topics related with notes, lectures and mock test series for UPSC
Exam by signing up for free. UPSC: Sampling Distributions | Management Optional Notes for UPSC
Description
Full syllabus notes, lecture & questions for Sampling Distributions | Management Optional Notes for UPSC - UPSC | Plus excerises question with solution to help you revise complete syllabus for Management Optional Notes for UPSC | Best notes, free PDF download
Information about Sampling Distributions
In this doc you can find the meaning of Sampling Distributions defined & explained in the simplest way possible. Besides explaining types of
Sampling Distributions theory, EduRev gives you an ample number of questions to practice Sampling Distributions tests, examples and also practice UPSC
tests
Related Searches
Sample Paper
,Semester Notes
,Important questions
,practice quizzes
,study material
,Sampling Distributions | Management Optional Notes for UPSC
,shortcuts and tricks
,Summary
,Viva Questions
,Sampling Distributions | Management Optional Notes for UPSC
,Objective type Questions
,video lectures
,mock tests for examination
,Free
,past year papers
,Previous Year Questions with Solutions
,MCQs
,ppt
,Extra Questions
,Sampling Distributions | Management Optional Notes for UPSC
,Exam
;
Additional Information about Sampling Distributions for UPSC Preparation
Sampling Distributions Free PDF Download
The Sampling Distributions is an invaluable resource that delves deep into the core of the UPSC exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Sampling Distributions now and kickstart your journey towards success in the UPSC exam.
Importance of Sampling Distributions
The importance of Sampling Distributions cannot be overstated, especially for UPSC aspirants.
This document holds the key to success in the UPSC exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Sampling Distributions Notes
Sampling Distributions Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Sampling Distributions.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Sampling Distributions Notes on EduRev are your ultimate resource for success.
Sampling Distributions UPSC Questions
The "Sampling Distributions UPSC Questions" guide is a valuable resource for all aspiring students preparing for the
UPSC exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Sampling Distributions on the App
Students of UPSC can study Sampling Distributions alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Sampling Distributions,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Sampling Distributions is prepared as per the latest UPSC syllabus.
|
© EduRev
|
Education Revolution
|
|
Signup on EduRev and stay on top of your study goals
10M+ students crushing their study goals daily