Important Formula: Geometry | Mathematics for RRB NTPC / ASM - RRB NTPC/ASM/CA/TA PDF Download
Geometry formulas are essential for calculating dimensions, perimeter, area, surface area, volume, and other properties of geometric figures. Geometry, a branch of mathematics, focuses on the relationships between points, lines, angles, surfaces, and solids, as well as their measurements and characteristics.
Geometry can be divided into two main categories: 2D or plane geometry and 3D or solid geometry.
2D shapes, such as squares, circles, and triangles, are flat figures with only two dimensions: length and width. In contrast, 3D shapes like cubes, cuboids, spheres, cylinders, and cones are solid figures with three dimensions: length, width, and height or depth. The following sections will explore various geometry formulas along with solved examples for better understanding.
What are Geometry Formulas?
The formulas used for finding dimensions, perimeter, area, surface area, volume, etc. of 2D and 3D geometric shapes are known as geometry formulas. 2D shapes consist of flat shapes like squares, circles, and triangles, etc., and cube, cuboid, sphere, cylinder, cone, etc are some examples of 3D shapes. The basic geometry formulas are given as follows: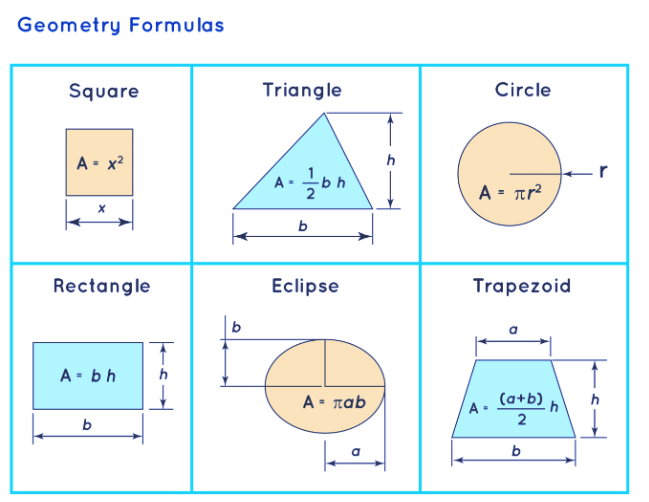
Basic Geometry Formulas
Let us see the list of all Basic Geometry Formulas here.
2D Geometry Formulas
Here is the list of various 2d geometry formulas according to the geometric shape. It also includes a few formulas where the mathematical constant π(pi) is used.
- Perimeter of a Square = 4(Side)
- Perimeter of a Rectangle = 2(Length + Breadth)
- Area of a Square = Side2
- Area of a Rectangle = Length × Breadth
- Area of a Triangle = 1/2 × base × height
- Area of a Trapezoid = 1/2 × (base1 + base2) × height
- Area of a Circle = A = π×r2
- Circumference of a Circle = 2πr
 |
Download the notes
Important Formula: Geometry
|
Download as PDF |
3D Geometry Formulas
The basic 3D geometry formulas are given as follows. It should be noted that the following formulas have used the mathematical constant π(pi)
- The curved surface area of a Cylinder = 2πrh
- Total surface area of a Cylinder = 2πr(r + h)
- Volume of a Cylinder = V = πr2h
- The curved surface area of a cone = πrl
- Total surface area of a cone = πr(r+l) = πr[r+√(h2+r2)]
- Volume of a Cone = V = ⅓×πr2h
- Surface Area of a Sphere = S = 4πr2
- Volume of a Sphere = V = 4/3×πr3
where,
- r = Radius;
- h = Height. and,
- l = Slant height
The formula table depicts the 2D geometry formulas and 3D geometry formulas.
SHAPES | FORMULAS |
1. Right Triangle | Pythagoras Theorem: base2 + height2 = hypotenuse2 Area = ½ × base × height Perimeter = base + height + hypotenuse |
2. Triangle | Perimeter, P = a + b + c Where, a, b, and c are the sides of a triangle. Area, A = ½ base × height |
3. Rectangle | Perimeter = 2(l + w) Area = lw Diagonal, d = √(l2 + w2) Where, l = length of a rectangle w = width of a rectangle |
4. Parallelogram | Perimeter, P = 2(a + b) Where, a and b are the sides of a parallelogram Area of parallelogram, A = base × height Height, h = Area/base Base, b = Area/height |
5. Trapezium | Area, A = ½(a + b)h Where, a and b are the parallel sides h = distance between two parallel sides |
6. Circle | Circumference = 2πr Area = πr2 Diameter = 2r Where, r = radius of a circle |
7. Square | Perimeter, P = 4a Area, A = a2 Diagonal, d = a√2 Side, a = √A Where, a = side of a square |
8. Arc | Arc Length, L = rθ Here, θ is the central angle in radians and r = radius |
9. Cube | Area, A = 6a2 Volume, V = a3 Edge, a = Volume⅓ Space diagonal = a√3 Where, a = side of a cube |
10. Cuboid | Surface Area, A = 2(lb + bh + hl) Volume, V = lbh Space diagonal, d = √( l2 + b2 +h2) Where, l= length b= breadth h= height |
11. Cylinder | Total Surface Area, A = 2πrh + 2πr2 Curved Surface Area, Ac = 2πrh Volume, V = πr2h Base Area, Ab = πr2 Radius, r = √(V/πh) Where, r= radius of a cylinder h= height of a cylinder |
12. Cone | Total Surface Area, A = πr(r+l) = πr[r+√(h2+r2)] Curved Surface Area, Ac = πrl Volume, V = ⅓πr2h Slant Height, l = √(h2+r2) Base Area, Ab = πr2 Where, r= radius of a cone h= height of a cone l = slant height |
13. Sphere | Surface Area, A = 4πr2 Volume, V = ⁴⁄₃πr3 Diameter = 2r Where, r= radius of a sphere |
Solved Examples
Example 1: Using geometry formulas of the cube, calculate the surface area and volume of a cube whose edge is 6 units.
Solution: To Find: The surface area and volume of a cube whose edge is 6 units
Using geometry formulas of cube,
Surface area of cube is = A = 6a2
A = 6 (6)2
A = 6 × 36 = 216 units2
Volume of a cube, V = a3
V = (6)3
V = 216 units3
Example 2: Calculate the circumference and the area and of a circle by using geometry formulas if the radius of the circle is 21 units.
Solution: To find the area and the circumference of the circle.
Given: Radius of a circle = 21 units
Using geometry formulas for circle,
Area of circle = π × r2
= 3.142857 × 212
= 1385.44
Now for the circumference of the circle,
Using geometry formulas for circle,
Circumference of a Circle = 2πr
= 2(3.142857)(21)
= 131.95
|
146 videos|96 docs|98 tests
|
FAQs on Important Formula: Geometry - Mathematics for RRB NTPC / ASM - RRB NTPC/ASM/CA/TA
| 1. What are the most important geometry formulas to remember for exams like RRB NTPC? |  |
| 2. How do you calculate the area of different shapes in geometry? |  |
| 3. What is the formula for the volume of a cylinder? |  |
| 4. How are perimeter and area different in geometry? |  |
| 5. Why is it important to memorize geometry formulas for competitive exams? |  |