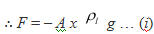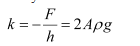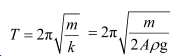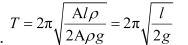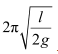Q13.1: Which of the following examples represents periodic motion?
(a) A swimmer completing one (return) trip from one bank of a river to the other and back.
(b) A freely suspended bar magnet displaced from its N-S direction and released.
(c) A hydrogen molecule rotating about its center of mass.
(d) An arrow released from a bow.
Ans: (a) The swimmer’s motion is not periodic. The motion of the swimmer between the banks of a river is back and forth. However, it does not have a definite period. This is because the time taken by the swimmer during his back-and-forth journey may not be the same.
(b) The motion of a freely-suspended magnet, if displaced from its N-S direction and released, is periodic. This is because the magnet oscillates about its position within a definite period of time.
(c) When a hydrogen molecule rotates about its center of mass, it comes to the same position again and again after an equal interval of time. Such motion is periodic.
(d) An arrow released from a bow moves only in the forward direction. It does not come backward. Hence, this motion is not periodic.
Q13.2: Which of the following examples represents (nearly) simple harmonic motion and which represents periodic but not simple harmonic motion?
(a) the rotation of the earth about its axis.
(b) motion of an oscillating mercury column in a U-tube.
(c) motion of a ball bearing inside a smooth curved bowl, when released from a point slightly above the lowermost point.
(d) general vibrations of a polyatomic molecule about its equilibrium position.
Ans: (b) and (c) are SHMs
(a) and (d) are periodic, but not SHMs
(a) During its rotation about its axis, the earth comes to the same position again and again in equal intervals of time. Hence, it is a periodic motion. However, this motion is not simple harmonic. This is because the earth does not have a to-and-fro motion about its axis.
(b) An oscillating mercury column in a U-tube is simple harmonic. This is because the mercury moves to and fro on the same path, about the fixed position, within a certain period of time.
(c) The ball moves to and fro about the lowermost point of the bowl when released. Also, the ball comes back to its initial position in the same period of time, again and again. Hence, its motion is periodic as well as simple harmonic.
(d) A polyatomic molecule has many natural frequencies of oscillation. Its vibration is the superposition of individual simple harmonic motions of a number of different molecules. Hence, it is not simply harmonic, but periodic.
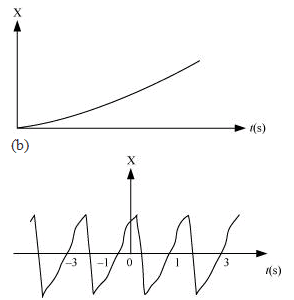
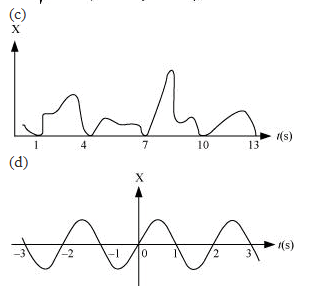
Q13.3: Figure 13.18 depicts four x-t plots for the linear motion of a particle. Which of the plots represents periodic motion? What is the period of motion (in the case of periodic motion)?



Ans: (b) and (d) are periodic
(a) It is not a periodic motion. This represents a unidirectional, linear uniform motion. There is no repetition of motion in this case.
(b) In this case, the motion of the particle repeats itself after 2 s. Hence, it is a periodic motion, having a period of 2 s.
(c) It is not a periodic motion. This is because the particle repeats the motion in one position only. For a periodic motion, the entire motion of the particle must be repeated in equal intervals of time.
(d) In this case, the motion of the particle repeats itself after 2 s. Hence, it is a periodic motion, having a period of 2 s.
Q13.4: Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give a period for each case of periodic motion (ω is any positive constant):
(a) sin ωt – cos ωt
(b) sin3 ωt
(c) 3 cos (π/4 – 2ωt)
(d) cos ωt + cos 3ωt + cos 5ωt
(e) exp (–ω2t2)
(f) 1 + ωt + ω2t2
Ans: (a) SHM
The given function is:

This function represents SHM as it can be written in the form: 
Its period is: 
(b) Periodic, but not SHM
The given function is:

The terms sin ωt and sin ωt individually represent simple harmonic motion (SHM). However, the superposition of two SHM is periodic and not simple harmonic.
(c) SHM
The given function is:

This function represents simple harmonic motion because it can be written in the form:
Its period is: 
(d) Periodic, but not SHM
The given function is  .
.
Each individual cosine function represents SHM. However, the superposition of three simple harmonic motions is periodic, but not simple harmonic.
(e) Non-periodic motion
The given function  is an exponential function. Exponential functions do not repeat themselves. Therefore, it is a non-periodic motion.
is an exponential function. Exponential functions do not repeat themselves. Therefore, it is a non-periodic motion.
(f) The given function 1 + ωt + ω2t2 is non-periodic.
Q13.5: A particle is in linear simple harmonic motion between two points, A and B, 10 cm apart. Take the direction from A to B as the positive direction and give the signs of velocity, acceleration and force on the particle when it is
(a) at the end A,
(b) at the end B,
(c) at the mid-point of AB going towards A,
(d) at 2 cm away from B going towards A,
(e) at 3 cm away from A going towards B, and
(f) at 4 cm away from B going towards A.
Ans: (a) Zero, Positive, Positive
(b) Zero, Negative, Negative
(c) Negative, Zero, Zero
(d) Negative, Negative, Negative
(e) Zero, Positive, Positive
(f) Negative, Negative, Negative
Explanation:
The given situation is shown in the following figure. Points A and B are the two end points, with AB = 10 cm. O is the midpoint of the path.

A particle is in linear simple harmonic motion between the end points
(a) At the extreme point A, the particle is at rest momentarily. Hence, its velocity is zero at this point.
Its acceleration is positive as it is directed along AO.
Force is also positive in this case as the particle is directed rightward.
(b) At the extreme point B, the particle is at rest momentarily. Hence, its velocity is zero at this point.
Its acceleration is negative as it is directed along B.
Force is also negative in this case as the particle is directed leftward.
(c) 
The particle is executing a simple harmonic motion. O is the mean position of the particle. Its velocity at the mean position O is the maximum. The value for velocity is negative as the particle is directed leftward. The acceleration and force of a particle executing SHM is zero at the mean position.
(d) 
The particle is moving toward point O from the end B. This direction of motion is opposite to the conventional positive direction, which is from A to B. Hence, the particle’s velocity and acceleration, and the force on it are all negative.
(e) 
The particle is moving toward point O from the end A. This direction of motion is from A to B, which is the conventional positive direction. Hence, the values for velocity, acceleration, and force are all positive.
(f) 
This case is similar to the one given in (d).
Q13.6: Which of the following relationships between the acceleration a and the displacement x of a particle involve simple harmonic motion?
(a) a = 0.7x
(b) a = –200x2
(c) a = –10x
(d) a = 100x3
Ans: (c) A motion represents simple harmonic motion if it is governed by the force law:
F = –kx
ma = –k

Where,
F is the force
m is the mass (a constant for a body)
x is the displacement
a is the acceleration
k is a constant
Among the given equations, only equation a = –10 x is written in the above form with k/m = 10
Hence, this relation represents SHM.
Q13.7: The motion of a particle executing simple harmonic motion is described by the displacement function,
x (t) = A cos (ωt φ).
If the initial (t = 0) position of the particle is 1 cm and its initial velocity is ω cm/s, what are its amplitude and initial phase angle? The angular frequency of the particle is π s–1. If instead of the cosine function, we choose the sine function to describe the SHM: x = B sin (ωt α), what are the amplitude and initial phase of the particle with the above initial conditions.
Ans: Initially, at t = 0:
Displacement, x = 1 cm
Initial velocity, v = ω cm/sec.
Angular frequency, ω = π rad/s–1
It is given that:


Squaring and adding equations (T) and (ii)= we get:

Dividing equation (ii) by equation (j). we get:

SHM is given as:

Putting the given values in this equation, we get;

Substituting the given values, we get:

Squaring and adding equations (iii) and (iv)= we get:

Dividing equation (iii) by equation (iv): we get:


Q13.8: A spring balance has a scale that reads from 0 to 50 kg. The length of the scale is 20 cm. A body suspended from this balance, when displaced and released, oscillates with a period of 0.6 s. What is the weight of the body?
Ans: Maximum mass that the scale can read, M = 50 kg
Maximum displacement of the spring = Length of the scale, l = 20 cm = 0.2 m
Time period, T = 0.6 s
Maximum force exerted on the spring, F = Mg
Where,
g = acceleration due to gravity = 9.8 m/s2
F = 50 x 9.8 = 490
∴ Spring constant, 
Mass m, is suspended from the balance.
Time period,

∴Weight of the body = mg = 22.36 x 9.8 = 219.167 N
Hence, the weight of the body is about 219 N.
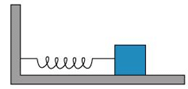
Q13.9: A spring with a spring constant of 1200 N m–1 is mounted on a horizontal table as shown in Fig. A mass of 3 kg is attached to the free end of the spring. The mass is then pulled sideways to a distance of 2.0 cm and released.

Determine (i) the frequency of oscillations, (ii) the maximum acceleration of the mass, and (iii) the maximum speed of the mass.
Ans: Spring constant, k = 1200 N m–1
Mass, m = 3 kg
Displacement, A = 2.0 cm = 0.02 cm
(i) Frequency of oscillation v, is given by the relation:

Hence, the frequency of oscillations is 3.18 cycles per second.
(ii) Maximum acceleration (a) is given by the relation:
a = ω2 A
Where,

Hence, the maximum acceleration of the mass is 8.0 m/s2.
(iii) Maximum velocity, vmax = Aω

Hence, the maximum velocity of the mass is 0.4 m/s.
Ques 13.10: In Exercise 13.9, let us take the position of mass when the spring is unstretched as x = 0, and the direction from left to right as the positive direction of x-axis. Give x as a function of time t for the oscillating mass if at the moment we start the stopwatch (t = 0), the mass is
(a) at the mean position,
(b) at the maximum stretched position, and
(c) at the maximum compressed position.
In what way do these functions for SHM differ from each other, in frequency, in amplitude or the initial phase?
Ans: (a) x = 2sin 20t
(b) x = 2cos 20t
(c) x = –2cos 20t
The functions have the same frequency and amplitude, but different initial phases.
Distance travelled by the mass sideways, A = 2.0 cm
Force constant of the spring, k = 1200 N m–1
Mass, m = 3 kg
Angular frequency of oscillation:

= 20 rad s–1
(a) When the mass is at the mean position, initial phase is 0.
Displacement, x = Asin ωt
= 2sin 20t
(b) At the maximum stretched position, the mass is toward the extreme right. Hence, the initial phase is .
Displacement, 

= 2cos 20t
(c) At the maximum compressed position, the mass is toward the extreme left. Hence, the initial phase is .
Displacement, 
Che functions have the same frequency  and amplitude (2 cm): but different initial phases
and amplitude (2 cm): but different initial phases 
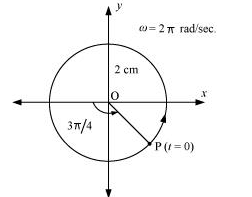
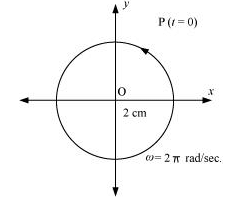
Q13.11: Figures 13.20 correspond to two circular motions. The radius of the circle, the period of revolution, the initial position, and the sense of revolution (i.e. clockwise or anti-clockwise) are indicated on each figure.


Obtain the corresponding simple harmonic motions of the x-projection of the radius vector of the revolving particle P, in each case.
Ans: (a) Time period, T = 2 s
Amplitude, A = 3 cm
At time, t = 0, the radius vector OP makes an angle  with the positive x-axis, i.e., phase angle
with the positive x-axis, i.e., phase angle 
Therefore, the equation of simple harmonic motion for the x-projection of OP, at time t, is given by the displacement equation:

(b) Time period, T = 4 s
Amplitude, a = 2 m
At time t = 0, OP makes an angle π with the x-axis, in the anticlockwise direction. Hence, phase angle, Φ = π
Therefore, the equation of simple harmonic motion for the x-projection of OP, at time t, is given as:

Q13.12: Plot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t = 0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case: (x is in cm and t is in s).
(a) x = –2 sin (3t π/3)
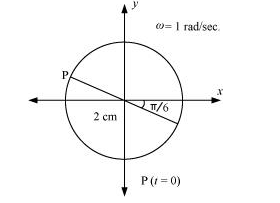
(b) x = cos (π/6 – t)
(c) x = 3 sin (2πt π/4)
(d) x = 2 cos πt
Ans: (a) 

If this equation is compared with the standard SHM equation , then we get:


The motion of the particle can be plotted as shown in the following figure.

(b) 
If this equation is compared with the standard SHM equation , then we get:


The motion of the particle can be plotted as shown in the following figure.

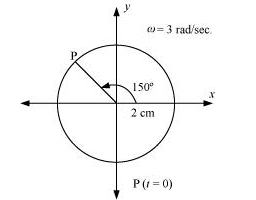
(c) 

If this equation is compared with the standard SHM equation , then we get:

Amplitude, A = 3 cm
Phase angle,  = 135
= 135
Angular velocity,

The motion of the particle can be plotted as shown in the following figure.

(d) x = 2 cos πt
If this equation is compared with the standard SHM equation , then we get:

Amplitude, A = 2 cm
Phase angle, Φ = 0
Angular velocity, ω = π rad/s
The motion of the particle can be plotted as shown in the following figure.

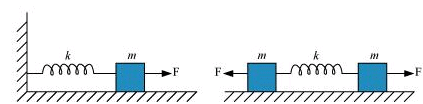
Q13.13: Figure 13.21 (a) shows a spring of force constant k clamped rigidly at one end and a mass m attached to its free end. A force F applied at the free end stretches the spring. Figure 13.30 (b) shows the same spring with both ends free and attached to a mass m at either end. Each end of the spring in Fig. 13.30(b) is stretched by the same force F.

(a) What is the maximum extension of the spring in the two cases?
(b) If the mass in Fig. (a) and the two masses in Fig. (b) are released, what is the period of oscillation in each case?
Ans: (a) For the one block system:
When a force F, is applied to the free end of the spring, an extension l, is produced. For the maximum extension, it can be written as:
F = kl
Where, k is the spring constant
Hence, the maximum extension produced in the spring,

For the two block system:
The displacement (x) produced in this case is:

(b) For the one block system:
For mass (m) of the block, force is written as:

Where, x is the displacement of the block in time t

It is negative because the direction of elastic force is opposite to the direction of displacement.



Where,
ω is angular frequency of the oscillation
∴Time period of the oscillation.; 

For the two block system:

It is negative because the direction of elastic force is opposite to the direction of displacement.

Angular frequency. 
∴Time period,

Q13.14: The piston in the cylinder head of a locomotive has a stroke (twice the amplitude) of 1.0 m. If the piston moves with simple harmonic motion with an angular frequency of 200 rad/min, what is its maximum speed?
Ans: Stroke = 1.0 m
Amplitude, 
The maximum speed (vmax) of the piston is give by the relation

Q13.15: The acceleration due to gravity on the surface of moon is 1.7 ms–2. What is the time period of a simple pendulum on the surface of moon if its time period on the surface of earth is 3.5 s? (g on the surface of earth is 9.8 ms–2)
Ans: Acceleration due to gravity on the surface of moon, = 1.7 m s–2
Acceleration due to gravity on the surface of earth, g = 9.8 m s–2
Time period of a simple pendulum on earth, T = 3.5 s

Where,

The length of the pendulum remains constant.
On moon's surface, time period, 

Hence, the time period of the simple pendulum on the surface of moon is 8.4 s.
Q13.16: A simple pendulum of length l and having a bob of mass M is suspended in a car. The car is moving on a circular track of radius R with a uniform speed v. If the pendulum makes small oscillations in a radial direction about its equilibrium position, what will be its time period?
Ans: The bob of the simple pendulum will experience the acceleration due to gravity and the centripetal acceleration provided by the circular motion of the car.
Acceleration due to gravity = g
Centripetal acceleration

Where,
v is the uniform speed of the car
R is the radius of the track
Effective acceleration (aeff) is given as:

Time period,

Where, l is the length of the pendulum
∴Time period, 
Q13.17: Cylindrical piece of cork of density of base area A and height h floats in a liquid of density . The cork is depressed slightly and then released. Show that the cork oscillates up and down simple harmonically with a period

where ρ is the density of cork. (Ignore damping due to viscosity of the liquid).
Ans: Base area of the cork = A
Height of the cork = h
Density of the liquid =
Density of the cork = ρ
In equilibrium:
Weight of the cork = Weight of the liquid displaced by the floating cork
Let the cork be depressed slightly by x. As a result, some extra water of a certain volume is displaced. Hence, an extra up-thrust acts upward and provides the restoring force to the cork.
Up-thrust = Restoring force, F = Weight of the extra water displaced
F =  –(Volume x Density x g)
–(Volume x Density x g)
Volume = Area x Distance through which the cork is depressed
Volume = Ax

According to the force law:
F = kx

Where, £is a constant

The time period of the oscillations of the cork:

Where,
m = Mass of the cork
= Volume of the cork x Density
= Base area of the cork x Height of the cork x Density of the cork

Hence, the expression for the time period becomes:

Q13.18: One end of a U-tube containing mercury is connected to a suction pump and the other end to atmosphere. A small pressure difference is maintained between the two columns. Show that, when the suction pump is removed, the column of mercury in the U-tube executes simple harmonic motion.
Ans: Area of cross-section of the U-tube = A
Density of the mercury column = ρ
Acceleration due to gravity = g
Restoring force, F = Weight of the mercury column of a certain height
F = –(Volume x Density x g)
F = –(A x 2h x ρ xg) = –2Aρgh = –k x Displacement in one of the arms (h)
Where,
2h is the height of the mercury column in the two arms
k is a constant, given by

Time period,

Where,
m is the mass of the mercury column
Let l be the length of the total mercury in the U-tube.
Mass of mercury, m = Volume of mercury x Density of mercury = Alρ
∴ 
Hence, the mercury column executes simple harmonic motion with time period 
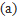
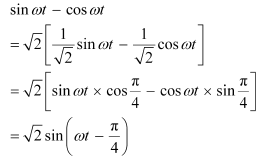
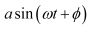
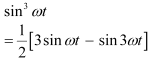
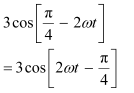
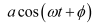
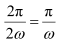
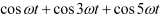 .
.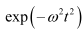 is an exponential function. Exponential functions do not repeat themselves. Therefore, it is a non-periodic motion.
is an exponential function. Exponential functions do not repeat themselves. Therefore, it is a non-periodic motion.
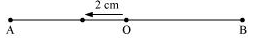
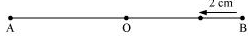
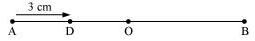
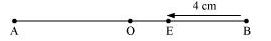
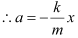










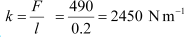
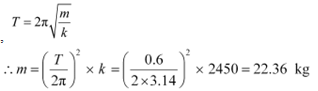
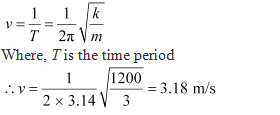
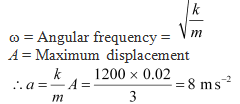

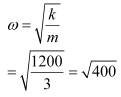
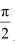
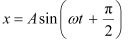

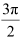
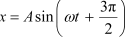
 and amplitude (2 cm): but different initial phases
and amplitude (2 cm): but different initial phases 


 with the positive x-axis, i.e., phase angle
with the positive x-axis, i.e., phase angle 
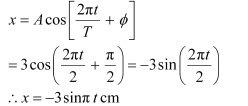
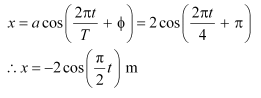
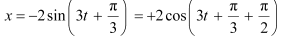
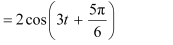
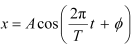
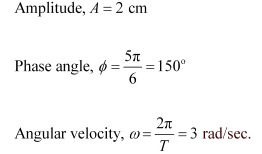
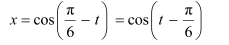
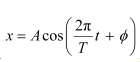
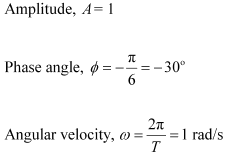
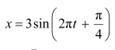
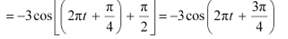
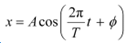
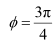 = 135
= 135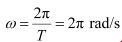
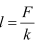
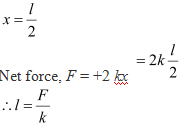






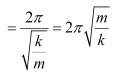






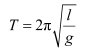



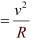
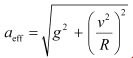
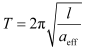
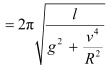
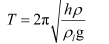
 –(Volume x Density x g)
–(Volume x Density x g)