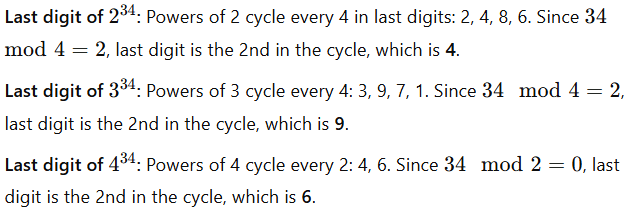Cyclicity & Factorial: Number System | General Aptitude for GATE - Mechanical Engineering PDF Download
| Table of contents |

|
| Cyclicity |

|
| Factors |

|
| Factorial |

|
| Multiplying the Last Two-Digit of the Exponents in Ten Seconds |

|
Cyclicity
- At times there are questions that require the students to find the units digit in case of the numbers occurring in powers.
- If anyone asks you to find the unit digit of 33, you will easily calculate it also you can calculate for 35, but if anyone asks you the unit digit of 17399, it will be hard to calculate easily.
- But it’s very simple if we understand that the units digit of a product is determined by whatever is the digit at the units place irrespective of the number of digits.
Example: 5 × 5 ends in 5 & 625 × 625 also ends in 5. - Now let’s examine the pattern that a number generates when it occurs in powers of itself. See the last digit of different numbers.
Table: Showing the Unit Digit of a Number for Different Exponents
From the above table, we can conclude that a number repeats the unit digit after an interval of 1, 2 or 4. Precisely we can say that the universal cyclicity of all the numbers is 4. i.e. after 4, all the numbers start repeating their unit digits.
Therefore, to calculate the unit digit for any exponent of a given number, we have to follow the following steps:
Step 1: Divide the exponent of the given number by 4 and calculate the remainder.
Step 2: The unit digit of the number is the same as the unit digit of the number raised to the power of the calculated remainder.
Step 3: If the remainder is zero, then the unit digit will be the same as the unit digit of N4.
Example 1. Find the last digit of (173)99.
- We notice that the exponent is 99. On dividing, 99 by 4 we get 24 as the quotient & 3 as the remainder.
- Now, these 24 pairs of 4 each do not affect the no. at the units place So, (173)99 ≈ (173)3. Now, the number at the units place is 33 = 27. so last digit is 7
Factors
A Factor is a number that divides another number completely.
Example: Factors of 24 are: 1, 2, 3, 4, 6, 8, 12, 24.
 Number of Factors
Number of Factors
If we have a number, N = pa × qb × rc Where p, q, and r are prime numbers and a, b, and c are the no. of times each prime number occurs, then the number of factors of n is found by (a + 1) (b +1)(c + 1).
Example: Find the number of factors of 24 × 32.
Number of factors = (4 + 1) (2 + 1) = (5)(3) = 15
Edurev Tip: All the perfect squares have odd number of factors and other number have even number of factors.
Number of Ways of Expressing a Given Number as a Product of Two Factors
- When a number is having even number of factors, then it can be written as a product of two numbers in
ways.
- But if a number has the odd number of factors, then it can be written as a product of two different numbers in
ways and can be written as a product of two numbers (different or similar) in
ways.
Examples:
(i) 148 can be expressed as 371 x 22. {So (p + 1) (q + 1) (r + 1) in the case of 148 is equal to 6 which is even}So product of two factors in 6/2 or 3 ways using
.
(ii) 144 can be expressed as (24.32).{So (p + 1) (q + 1) (r + 1) in the case of 144 is equal to 15 which is odd} can be written as a product of two different numbers in, i.e. 7 ways using.
Sum of the Factors of a Number
- If a number N is written in the form of N = ap.bq.cr , where a, b & c are prime numbers and p, q & r are positive integers, then the sum of all the factors of the number is given by the formula:
- Sum of factors =
Number of Co-primes to N
- Number of co primes to N, which are less than N
Factorial
- Factorial is defined for any positive integer. It is denoted by
or !. Thus “Factorial n” is written as n! or
- n! is defined as the product of all the integers from 1 to n.
- Thus n! = 1.2.3. …. n. (n! = n(n – 1)!)
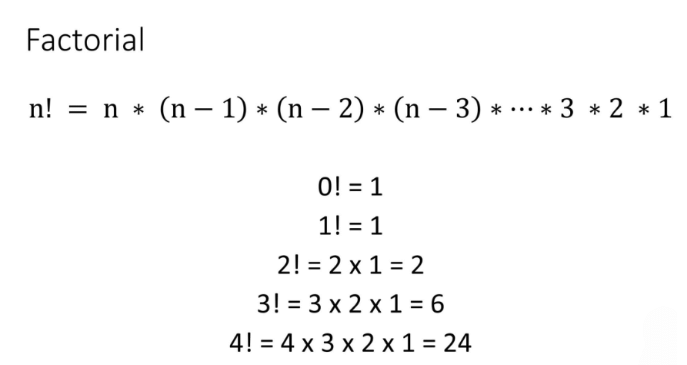
Example 1: Find the largest power of 3 that can divide 95! without leaving any remainder.
- First look at the detailed explanation and then look at a simpler method for solving the problem.
- When we write 95! in its full form, we have 95 × 94 × 93 ….. × 3 × 2 × 1.
- When we divide 95! by a power of 3, we have these 95 numbers in the numerator. The denominator will have all 3’s. The 95 numbers in the numerator have 31 multiples of 3 which are 3, 6, 9….90, 93. Corresponding to each of these multiplies we can have a 3 in the denominator which will divide the numerator completely without leaving any remainder, i.e. 331 can definitely divide 95!.
- Further, every multiple of 9, i.e. 9, 18, 27, etc. after cancelling out a 3 above, will still have one more 3 left. Hence for every multiple of 9 in the numerator, we have an additional 3 in the denominator. There are 10 multiples of 9 in 95, i.e. 9, 18….81, 90. So we can take 10 more 3’s in the denominator.
- Similarly, for every multiple of 33 we can take an additional 3 in the denominator.
- Since there are 3 multiples of 27 in 91 (they are 27, 54 and 81), we can have three more 3’s in the denominator.
- Next, corresponding to every multiple of 34, i.e. 81 we can have one more 3 in the denominator. Since there is one multiple of 81 in 95, we can have one additional 3 in the denominator.
- Hence the total number of 3’s we can have in the denominator is 31 + 10 + 3 + 1, i.e., 45. So 345 is the largest power of 3 that can divide 95! without leaving any remainder.
The same can be done in a simpler manner, also, which is explained below:
- Divide 95 by 3 you get a quotient of 31. Divide this 31 by 3 we get a quotient of 10. Divide this 10 by 3 we get a quotient of 3. Divide this quotient of 3 once again by 3 we get a quotient of 1.
- Since we cannot divide the quotient any more by 3, we stop here. Add all the quotients, i.e. 31 + 10 + 3 + 1 which gives 45 which is the highest power of 3.
- Add all the quotients 31 + 10 + 3 + 1, which give 45.
Note: This type of division, where the quotient of one step is taken as the dividend in the subsequent step, is called “Successive Division”. In general, in the successive division, the divisor need not be the same (as it is here). Here, the number 95 is being successively divided by 3.
Note:
- This method is applicable only if the number whose largest power is to be found out is a prime number.
- If the number is not a prime number, then we have to write the number as the product of relative primes, find the largest power of each of the factors separately for the smallest, among the largest powers of all these relative factors of the given number will give the largest power required.
Example 2. Find the largest power of 12 that can divide 200!
- Here we cannot apply the Successive Division method because 12 is not a prime number. Resolve 12 into a set of prime factors.
- We know that 12 can be written as 3 × 4. So, we will find out the largest power of 3 that can divide 200! and the largest power of 4 that can divide 200! and take the LOWER of the two as the largest power of 12 that can divide 200!
- To find out the highest power of 4, since 4 itself is not a prime number, we cannot directly apply the successive division method.
We first have to find out the highest power of 2 that can divide 200!- Since two 2’s taken together will give us a 4, half the power of 2 will give the highest power of 4 that can divide 200!
We find that 197 is the largest power of 2 that can divide 200!
Half this figure-98-will be the largest power of 4 that can divide 200!- Since the largest power of 3 and 4 that can divide 200! are 97 an 98 respectively, the smaller of the two, i.e., 97 will be the largest power of 12 that can divide 200! without leaving any remainder.
Example 3. What is the last digit of 234 × 334 × 434.
- Given = (24)34
Now multiply the last digits: 4×9=36 (last digit 6)
- Answer is 6
Example 4. What is the rightmost non-zero digit of (270)270
- The required answer is the last digit of 7270.
- The last digit of 7 powers repeats after every 4.
- So, the last digit of 7270 is the last digit of 72 = 9.
Example 5. How many factors do 1296 have?
- 1296 = 4 × 324
- 1296 = 4 × 4 × 81
- 1296 = 24 × 34
- Number of factors = (4 + 1) (4 + 1) = 25.
Example 6. If x is the sum of all the factors of 3128 and y is the number of factors of x and z is the number of ways of writing ‘y’ as a product of two numbers, then z =?
- 3128 = 4 × 782
- 3128 = 4 × 2 × 391
- 3128 = 23 × 17 × 23
- x = 15 × (17 + 1) (23 + 1)
- x = 3 × 5 × 9 × 2 × 8 × 3
- x = 24 × 34 × 5
- y = (4 + 1) (4 + 1) (1 + 1)
- y = 2 × 52
Example 7. How many co-primes are there for 240, which are less than 240?
- 240 = 16 × 15
- 240 = 24 × 3 × 5
- Number of co primes to N, which are less than N
- If N = ap × bq × - - - - (a, b, - - - - are Prime no.s)
Example 8. What is the sum of all the co-primes to 748? Which are less than N?
- 748 = 4 × 187
748 = 22 × 11 × 17- Number of co primes to N, which are less than N
- Sum of all the co primes to N. which are less than N is N/2 (number of co primes to N, which are less than N.
- Sum = 748/2 * 320 = 119680
Example 9. In how many ways 5544 can be written as a product of 2 co primes?
- If N = ap × bq × - - - -, where a, b, - - - - are prime numbers
- N can be written as a product of two co primes in 2n-1 ways, where n is the number of prime factors to N.
- 5544 = 11 × 504
= 11 × 9 × 56
= 11 × 9 × 8 × 7
= 23 × 32 × 7 × 11
Number of Ways in which N can be written as product of Co-primes = 2n-1
- Ans = 24-1 = 23 = 8. (Because, 2, 3, 7 & 11 are four different prime factors).
Multiplying the Last Two-Digit of the Exponents in Ten Seconds
- Follow this trick and shortcut method to multiply the numbers in your head. This can be a huge advantage for those preparing for competitive exams.
- The tricks used in this video is simple and straight. You might not need a paper and pen to solve this type of question after learning the shortcuts.
- The last digit of a number in form: an
The simple trick followed to know the last digit is to remember the cyclicity of the last digit of the number given. Every digit from 1 to 9 follow a pattern when exponential power is applied. Here is the table that will be handy in solving these type of questions.
Last digit / unit's digit of an
|
229 videos|191 docs|158 tests
|
FAQs on Cyclicity & Factorial: Number System - General Aptitude for GATE - Mechanical Engineering
| 1. What is cyclicity in the context of number systems? |  |
| 2. How do factors relate to factorial cyclicity in number systems? |  |
| 3. How can one calculate factorial cyclicity in a given number system? |  |
| 4. What are some common examples of factorial cyclicity in different number systems? |  |
| 5. How does understanding factorial cyclicity help in number theory and mathematical calculations? |  |