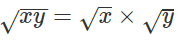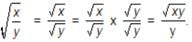Important Formulas: Square Root & Cube Root | CSAT Preparation - UPSC PDF Download
♦ Square Root
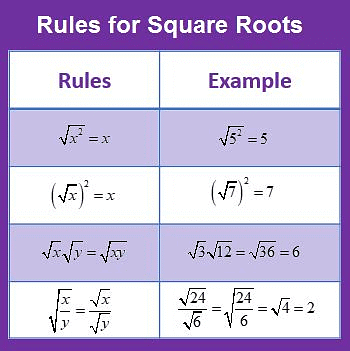
- The square root of a number is a value which, when multiplied by itself, gives the original number. It is represented by a radical symbol √
- “Square Root” is often used to refer to the principal square root.
- Square Root Formula
The square root of a number has the exponent of 1/2. The square root formula is used to find the square root of a number. We know the exponent formula:
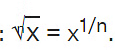
When n = 2, we call it square root. We can use any of the above methods for finding the square root, such as prime factorization, and so on. 91/2 = √9 = √(3×3) = 3. So, the formula for writing the square root of a number is √x= x1/2
- Thus, √4 = 2, √9 = 3, √196 = 14.
♦ Cube Root
- The cube root of a number is the value that, when multiplied by itself three times, gives the original number. Whenever a number (x) is multiplied three times, then the resultant number is known as the cube of that number.
- We denote the cube root of x by ∛x.
- Cube Root Formula
The cube root formula helps in the calculation of the cube root of any given number that is expressed in a radical form using the symbol ∛. It can be calculated by first finding out the prime factorization of the given number and then later applying the cube root formula. Suppose, x is any number such that, x = y × y × y.
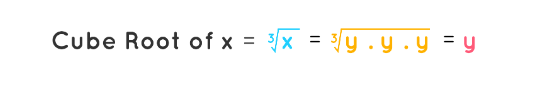
- Thus,
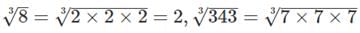
Important Points:
(a)
(b)
Easy Tricks To Find Square Roots And Cube Roots
- To find the square root or cube root of a number is not an easy task. When you’re giving a time-bound exam like CAT, CMAT, CET, NMAT,GMAT , Bank Exams etc. it can drain you of your precious time. This is a worse deal when finding square or cube root is only part of a bigger problem, like in Data Interpretation or Compound Interest problems in Quantitative Aptitude.
- So if your Mental Mathematics is a little weak, let us learn how to quickly and easily find the square root or cube root of a number. This trick is sure to save you at least 40 seconds of calculations per question. At first, you will find it difficult but with practice, you will be able to find square root or cube root of any number. Then let us start.
Finding Square Root
We can estimate the square root of a perfect square number using a trick. To determine the square root without using a long division, we must know the unit digits of squares of the first ten numbers.
1 = 1
2 = 4
3 = 9
4 = 6
5 = 5
6 = 6
7 = 9
8 = 4
9 = 1
10 = 0
It is possible to calculate the square root of a number having 4 digits as well as 5 digits. Let us learn to calculate the square root of numbers using the trick.
1. Square Root Trick for 4 Digit Numbers
The steps to estimate the square root of a 4 digit number are:- Step 1: Pair the digits starting from right to left.
- Step 2: Match the unit digit of number from the chart and determine the possible values of the square root of the unit digit.
- Step 3: Let us consider the first pair of digits. Let it be "n".
- Step 4: Determine between which two squares this number lies, √a2 < n < √b2. This concludes that a < n < b. Thus, the tens digit of the desired square root is "a".
- Step 5: As referred to in the chart of squares, there are only two numbers whose squares do not repeat i.e, 5 and 10. Check if the unit digit obtained in Step 2 is anyone of them.
- Step 6: Once, it is checked if the obtained number is 5 or 10, then they are written as it is, else we find out the unit digit by using the below steps.
Steps to find units digit if the unit digit obtained in Step 2 is apart from 5 or 10 are:
- Step 7: Now, Multiply a and b.
- Step 8: If ab≤ n, then choose b, else choose a.
2. Square Root Trick for 5 Digit Numbers
The steps to estimate the square root of a 5 digit number are:
- Step 1: First pair the digits starting from right to left.
- Step 2: Match the unit digit of number from the chart and determine the possible values of the square root of the unit digit.
- Step 3: Let us consider the group of the first three digits. Let it be "n".
- Step 4: Determine between which two squares this number lies, √a2 < n < √b2. This concludes that a < n < b. Thus, the tens digit of the desired square root is "a".
- Step 5: As referred to in the chart of squares, there are only two numbers whose squares do not repeat i.e, 5 and 10. Check if the unit digit obtained in Step 2 is anyone among them.
- Step 6: Once, it is checked if the obtained number is 5 or 10, then they are written as it is, else we find out the unit digit by using the steps below.
Steps to find units digit if the unit digit obtained in Step 2 is apart from 5 or 10 are:
- Step 7: Now, Multiply a and b.
- Step 8: If ab≤ n, then choose b, else choose a.
Example: Find the square root of 10816.
Solution: The steps to determine the square root of 10816 are:
- The digits of 10816 are paired as 108 16.
- The unit digit of 10816 is 6. Hence, the possible unit digits after taking square root could be 4 and 6.
- When we consider the first three digits of 10816, that are 108.
- The number 108 lies between two perfect squares 100 and 121 that is, 100 < 108 < 121 which can be written as 102 < 108 < 112. Hence, the tens digit of the square root of 10816 is 10 as 10 < 11.
- As the number obtained in step 2 is, 4 and 6. The numbers can be 104 or 106.
- 108 lies between 102 and 112. Their product gives the value 110.
- As the 110 > 108, the square root of 10816 will be the lesser of 104 and 106 which is 104.
Finding Cube Root
Remembering Units DigitsFirst we need to remember cubes of 1 to 10 and unit digits of these cubes. The figure below shows the unit digits of cubes (on the right) of numbers from 1 to 10 (on the left).
1 = 1
2 = 8
3 = 7
4 = 4
5 = 5
6 = 6
7 = 3
8 = 2
9 = 9
10 = 0
Now with reference to above we can definitely say that:
Whenever unit digit of a number is 9, the unit digit of the cube of that number will also be 9. Similarly, if the unit digit of a number is 9, the unit digit of the cube root of that number will also be 9. Similarly, if unit digit of a number is 2, unit digit of the cube of that number will be 8 and vice versa if unit digit of a number is 8, unit digit of the cube root of that number will be 2. Similarly, it will be applied to unit digits of other numbers as well.
1. Deriving Cube Root From Remaining Digits
Let’s see this with the help of an example. Note that this method works only if the number given is a perfect cube.
Find the cube root of 474552.
- Unit digit of 474552 is 2. So we can say that unit digit of its cube root will be 8.
- Now we find cube root of 447552 by deriving from remaining digits.
- Let us consider the remaining digits leaving the last 3 digits. i.e. 474.
- Since 474 comes in between cubes of 7 and 8.
- So the ten’s digit of the cube root will definitely be 7
i.e. cube root of 474552 will be 78.
Let us take another example.
Find the cube root of 250047.
- Since the unit digit of the number is 7, so unit digit in the cube root will be 3.
- Now we will consider 250.
- Since, 63 < 250 < 73, So tens digit will be 6
- So we find cube root of the number to be 63.
2. For Numbers Between 25 - 50
Time saving techniques are paramount when we have to deal with Quant questions in any competitive exams, which is missing a place in the provided material.
Many times we need to find a square of a number and it gets difficult to remember it beyond 30. So, here is a trick…..
Suppose, we need to find the square of 47.
Step 1: If the number is between 25 and 50.
Find out by how much the given number is smaller than 47. In the above case, it is 3.
Step 2: Write the square of this number in unit’s and ten’s place in this manner Square of 3 is 9.
It is a single digit number, so we can write it as 09
Step 3: Find the difference between the given number and 25
47-25 = 22
Therefore, square of 47 will be 2209.
This is true for the square of any number between 25 and 50.
3. For Numbers Between 51 - 75
Example: Square of 73
Step 1: find the difference between the number (73) and 50, which is 23.
Step 2: Find the square of the difference, 232 = 529. Keep the last two number aside which will be the last two digits of the square of 73.
Step 3: Find the difference between the number for which we have to find the square and add the difference with 5(which is the first digit of the square of the difference)
⇨ (73 - 25) + 5 = 53. This number will be the first two digits of the square.
The square of the number 73 will be = 5329
4. For Numbers Between 76 - 100
Example: Square of 88
Step 1: Subtract the number from 100.. (100 - 88 = 12)
Step 2: Find the square of the number obtained .. 122 = 144
Last two digits of the square of this number will be last 2 digits of square of 88.
Step 3: The first two digits will be obtained by adding the first digit of square of 12. i.e. 1 and the difference between 88 and 12 (88 - 12 = 76)
76 + 1 = 77
The square of the number 88 will be = 7744
Square of 87 = (87 - 13)…….132
74…………….69
7569
|
205 videos|264 docs|136 tests
|
FAQs on Important Formulas: Square Root & Cube Root - CSAT Preparation - UPSC
| 1. How can I find the square root of a number easily? |  |
| 2. Is there a shortcut to finding cube roots quickly? |  |
| 3. Can square roots and cube roots be negative numbers? |  |
| 4. How can I estimate square roots and cube roots without a calculator? |  |
| 5. Are there any tricks to mentally calculate square roots and cube roots? |  |