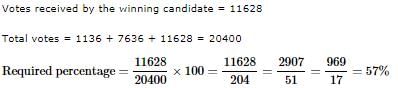All Exams >
UPSC >
CSAT Preparation >
All Questions
All questions of Percentages for UPSC CSE Exam
There are three galleries in a coal mine. On the first day, two galleries are operative and after some time, the third gallery is made operative. With this, the output of the mine became half as large again. What is the capacity of the second gallery as a percentage of the first, if it is given that a four-month output of the first and the third galleries was the same as the annual output of the second gallery?
- a)60%
- b)64%
- c)65%
- d)70%
Correct answer is option 'A'. Can you explain this answer?
There are three galleries in a coal mine. On the first day, two galleries are operative and after some time, the third gallery is made operative. With this, the output of the mine became half as large again. What is the capacity of the second gallery as a percentage of the first, if it is given that a four-month output of the first and the third galleries was the same as the annual output of the second gallery?
a)
60%
b)
64%
c)
65%
d)
70%
|
Tanishq Sengupta answered |
The third gallery making the capacity ‘half as large again’ means an increase of 50%.
Further, it is given that: 4(first + third) = 12 (second) In order to get to the correct answer, try to fit in the options into this situation.
(Note here that the question is asking you to find the capacity of the second gallery as a percentage of the first.)
If we assume option (a) as correct – 70% the following solution follows:
If the second is 70, then first is 100 and the first + second is 170. Then third will be 85 (50% of first + second).
If the second is 70, then first is 100 and the first + second is 170. Then third will be 85 (50% of first + second).
Then the equation:
4 X (100 + 85) should be equal to 12 X 70
But this is not true.
4 X (100 + 85) should be equal to 12 X 70
But this is not true.
Through trial and error, you can see that the third option fits correctly.
4 X (100 + 80) = 12 X 60.
Hence, it is the correct answer.
4 X (100 + 80) = 12 X 60.
Hence, it is the correct answer.
Two tailors X and Y are paid a total of Rs. 550 per week by their employer. If X is paid 120 percent of the sum paid to Y, how much is Y paid per week?
- a)Rs. 200
- b)Rs. 250
- c)Rs. 300
- d)None of these
Correct answer is option 'B'. Can you explain this answer?
a)
Rs. 200
b)
Rs. 250
c)
Rs. 300
d)
None of these
|
Ishani Rane answered |
Let the sum paid to Y per week be Rs. z.
Then, z + 120% of z = 550.
= z + 120/100 z = 550
= 11/5 z = 550
= (550 * 5)/11 = 250.
A fruit seller had some apples. He sells 40% apples and still has 420 apples. Originally, he had:
- a)588 apples
- b)600 apples
- c) 672 apples
- d)700 apples
Correct answer is option 'D'. Can you explain this answer?
a)
588 apples
b)
600 apples
c)
672 apples
d)
700 apples
|
|
Manik Sodhi answered |
Apples sold =40 % apples remaining 60%=420 60% of the apples is 420 so 100% of apples is 420/60×100=700
In an election between two candidates, one got 55% of the total valid votes, 20% of the votes were invalid. If the total number of votes was 7500, the number of valid votes that the other candidate got, was:
- a)2700
- b)2900
- c)3000
- d)3100
Correct answer is option 'A'. Can you explain this answer?
In an election between two candidates, one got 55% of the total valid votes, 20% of the votes were invalid. If the total number of votes was 7500, the number of valid votes that the other candidate got, was:
a)
2700
b)
2900
c)
3000
d)
3100
|
Dhruv Mehra answered |
If 20% of a = b, then b% of 20 is the same as:
- a)4% of a
- b)5% of a
- c)20% of a
- d) None of these
Correct answer is option 'A'. Can you explain this answer?
a)
4% of a
b)
5% of a
c)
20% of a
d)
None of these
|
Subham Basu answered |
20% of a = b => (20/100)a = b
b% of 20 =(b/100) x 20 = (20a/100) x (1/100) x (20) = 4a/100 = 4% of a.
What percentage of numbers from 1 to 70 have 1 or 9 in the unit's digit?
- a)1
- b)14
- c)20
- d)21
Correct answer is 'C'. Can you explain this answer?
a)
1
b)
14
c)
20
d)
21
|
Gowri Chakraborty answered |
Clearly, the numbers which have 1 or 9 in the unit's digit, have squares that end in the digit 1. Such numbers from 1 to 70 are 1, 9, 11, 19, 21, 29, 31, 39, 41, 49, 51, 59, 61, 69.
Number of such number =14
Required percentage = (14/70 * 100)% = 20%
The number of girls appearing for an admission test is twice the number of boys. If 30% of the girls and 45% of the boys get admission, the percentage of candidates who do not get admission is- a)65
- b)50
- c)60
- d)35
Correct answer is option 'A'. Can you explain this answer?
The number of girls appearing for an admission test is twice the number of boys. If 30% of the girls and 45% of the boys get admission, the percentage of candidates who do not get admission is
a)
65
b)
50
c)
60
d)
35
|
Riverdale Learning Institute answered |
Let the number of girls be 2x and number of boys be x.
Girls getting admission = 0.6x
Boys getting admission = 0.45x
Number of students not getting admission = 3x – 0.6x -0.45x = 1.95x
Percentage = (1.95x/3x) * 100 = 65%
Instead of a metre scale, a cloth merchant uses a faulty 120 cm scale while buying, but uses a faulty 80 cm scale while selling the same cloth. If he offers a discount of 20%, what is his overall profit percentage?
- a)25%
- b)20%
- c)40%
- d)15%
Correct answer is option 'B'. Can you explain this answer?
Instead of a metre scale, a cloth merchant uses a faulty 120 cm scale while buying, but uses a faulty 80 cm scale while selling the same cloth. If he offers a discount of 20%, what is his overall profit percentage?
a)
25%
b)
20%
c)
40%
d)
15%
|
Innovative Classes answered |
Seema saved Rs. 900 in the first 3 months. She must saved Rs. (11400 – 900) = Rs. 10500 in the subsequent months.
The sequence will be of the form: 350 + 400 +……….. n terms = 10500
Solving, we get n = 15
The savings of Rs. 10500 is done in 15 months. Seema saved Rs. 11400 in 15+3 = 18 months.
Hence, option A is the correct answer.
The sequence will be of the form: 350 + 400 +……….. n terms = 10500
Solving, we get n = 15
The savings of Rs. 10500 is done in 15 months. Seema saved Rs. 11400 in 15+3 = 18 months.
Hence, option A is the correct answer.
Practice Quiz or MCQ (Multiple Choice Questions) with solution are available for Practice, which would help you prepare for Percentages under Quantitative Aptitude. You can practice these practice quizzes as per your speed and improvise the topic. The same topic is covered under various competitive examinations like - CAT, GMAT, Bank PO, SSC and other competitive examinations.Q. Two students appeared at an examination. One of them secured 9 marks more than the other and his marks was 56% of the sum of their marks. The marks obtained by them are:- a)39, 30
- b)41, 32
- c)42, 33
- d)43, 34
Correct answer is option 'C'. Can you explain this answer?
Practice Quiz or MCQ (Multiple Choice Questions) with solution are available for Practice, which would help you prepare for Percentages under Quantitative Aptitude. You can practice these practice quizzes as per your speed and improvise the topic. The same topic is covered under various competitive examinations like - CAT, GMAT, Bank PO, SSC and other competitive examinations.
Q. Two students appeared at an examination. One of them secured 9 marks more than the other and his marks was 56% of the sum of their marks. The marks obtained by them are:
a)
39, 30
b)
41, 32
c)
42, 33
d)
43, 34
|
Raghavendra Sharma answered |
Let their marks be (x+9) and x.
Then, x+9 = 56/100(x + 9 +x)
=> 25(x+9)
=> 14 (2x + 9)
=> 3x = 99
=> x = 33.
So, their marks are 42 and 33
What percentage of numbers from 1 to 70 have 1 or 9 in the unit's digit?
- a)1
- b)14
- c)20
- d)21
Correct answer is option 'C'. Can you explain this answer?
a)
1
b)
14
c)
20
d)
21
|
Nitya Reddy answered |
Clearly, the numbers which have 1 or 9 in the unit's digit, have squares that end in the digit 1. Such numbers from 1 to 70 are 1, 9, 11, 19, 21, 29, 31, 39, 41, 49, 51, 59, 61, 69.
Number of such number =14
Sailesh is working as a sales executive with a reputed FMCG Company in Hyderabad. As per the Company’s policy, Sailesh gets a commission of 6% on all sales upto Rs. 1,00,000 and 5% on all sales in excess of this amount. If Sailesh remits Rs. 2,65,000 to the FMCG company after deducting his commission, his total sales were worth:- a)Rs. 2,80,000
- b)Rs. 2,90,526
- c)Rs. 2,21,054
- d)Rs. 1,20,000
Correct answer is option 'A'. Can you explain this answer?
Sailesh is working as a sales executive with a reputed FMCG Company in Hyderabad. As per the Company’s policy, Sailesh gets a commission of 6% on all sales upto Rs. 1,00,000 and 5% on all sales in excess of this amount. If Sailesh remits Rs. 2,65,000 to the FMCG company after deducting his commission, his total sales were worth:
a)
Rs. 2,80,000
b)
Rs. 2,90,526
c)
Rs. 2,21,054
d)
Rs. 1,20,000
|
Kishan Darak answered |
94%of ctc =100000
100% of ctc =100000/94×100=106383
95% of ctc=265000-100000=165000
100% of ctc =165000/95×100=173684
overall ctc =106383+173684=280067
(trial and error method)
nearest answer =280000
Therefore Option 'A' is the correct answer
100% of ctc =100000/94×100=106383
95% of ctc=265000-100000=165000
100% of ctc =165000/95×100=173684
overall ctc =106383+173684=280067
(trial and error method)
nearest answer =280000
Therefore Option 'A' is the correct answer
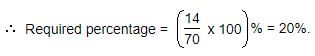
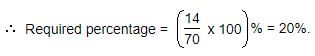
The ratio of number of male and female journalists in a newspaper office is 5:4. The newspaper has two sections, political and sports. If 30 percent of the male journalists and 40 percent of the female journalists are covering political news, what percentage of the journalists (approx.) in the newspaper is currently involved in sports reporting?- a)60 percent
- b)65 percent
- c)70 percent
- d)None of the above
Correct answer is option 'B'. Can you explain this answer?
The ratio of number of male and female journalists in a newspaper office is 5:4. The newspaper has two sections, political and sports. If 30 percent of the male journalists and 40 percent of the female journalists are covering political news, what percentage of the journalists (approx.) in the newspaper is currently involved in sports reporting?
a)
60 percent
b)
65 percent
c)
70 percent
d)
None of the above
|
|
Riverdale Learning Institute answered |
The ratio of number of male and female journalists in a newspaper office is 5:4.
The newspaper has two sections, political and sports.
If 30 percent of the male journalists and 40 percent of the female journalists are covering political news, what percentage of the journalists (approx.) in the newspaper is currently involved in sports reporting?
Let ‘9x’ be the number of total journalists in the office.
Then, we can say that the number of male and female journalists are ‘5x’ and ‘4x’ respectively.
Then, we can say that the number of male and female journalists are ‘5x’ and ‘4x’ respectively.
It is given that 30 percent of the male journalists and 40 percent of the female journalists are covering political news. Hence, total number of journalists who are covering political news = 0.3*5x + 0.4*4x = 3.1x
Therefore, the total number journalists who are covering sports news = 9x – 3.1x = 5.9x.
Hence, the percentage of the journalists in the newspaper is currently involved in sports reporting = 5.9x/9x x 100 ≈
Hence, the percentage of the journalists in the newspaper is currently involved in sports reporting = 5.9x/9x x 100 ≈
65 percent. Therefore, option B is the correct answer.
Instead of a metre scale, a cloth merchant uses a faulty 120 cm scale while buying, but uses a faulty 80 cm scale while selling the same cloth. If he offers a discount of 20%, what is his overall profit percentage?- a)25%
- b)20%
- c)40%
- d)15%
Correct answer is option 'B'. Can you explain this answer?
Instead of a metre scale, a cloth merchant uses a faulty 120 cm scale while buying, but uses a faulty 80 cm scale while selling the same cloth. If he offers a discount of 20%, what is his overall profit percentage?
a)
25%
b)
20%
c)
40%
d)
15%
|
Upsc Rank Holders answered |
Let’s say the cost of the cloth is x rs per metre. Because of the faulty meter, he is paying x for 120 cms when buying.
So cost of 100 cms = 100x/120.
He is selling 80 cms for x, so selling price of 100cms of cloth is 100x/80.
discount = 20%
so the effective selling price is .8*100x/80= x
profit = SP-CP= x – 100x/120 = x/6
Profit % = x/6 divided by 100x/120 = 20%
The income of Amala is 20% more than that of Bimala and 20% less than that of Kamala. If Kamala’s income goes down by 4% and Bimala’s goes up by 10%, then the percentage by which Kamala’s income would exceed Bimala’s is nearest to- a) 31
- b) 29
- c) 28
- d) 32
Correct answer is option 'A'. Can you explain this answer?
The income of Amala is 20% more than that of Bimala and 20% less than that of Kamala. If Kamala’s income goes down by 4% and Bimala’s goes up by 10%, then the percentage by which Kamala’s income would exceed Bimala’s is nearest to
a)
31
b)
29
c)
28
d)
32
|
Ayushi sharma answered |
Earns x amount of income, then Bimala earns 0.8x (20% less than Kamala) and Amala earns 1.2(0.8x) = 0.96x (20% more than Bimala).
When 40% of a number E is added to another number R, B becomes 125% of its previous value. Then which of the following is true regarding the values of E and R?- a)Either (a) or (b) can be true depending upon the values of E and R
- b)R > E
- c)E > R
- d)R = E
Correct answer is option 'A'. Can you explain this answer?
When 40% of a number E is added to another number R, B becomes 125% of its previous value. Then which of the following is true regarding the values of E and R?
a)
Either (a) or (b) can be true depending upon the values of E and R
b)
R > E
c)
E > R
d)
R = E
|
|
Tanishq Shah answered |
Let's start by translating the given information into equations:
- "40% of a number E": this can be written as 0.4E
- "added to another number R": we add 0.4E to R, so we get R + 0.4E
- "B becomes 125% of its previous value": if we call the previous value of B "B0", then we have B = 1.25B0
Putting it all together, we can write:
R + 0.4E = 1.25B0
But we don't know anything about B0, so we need to find another equation to solve for E and R. We can use the fact that B is a certain percentage of its previous value:
B = 1.25B0 = 1.25(B/1.25) = B/0.8
This means that B is 0.8 times its current value. So we can write:
B = 0.8(R + 0.4E)
Now we have two equations with two unknowns, E and R:
R + 0.4E = 1.25B0
B = 0.8(R + 0.4E)
We can solve for E by substituting the second equation into the first:
R + 0.4E = 1.25(0.8(R + 0.4E))
Simplifying:
R + 0.4E = R + 1.0E
0.6E = R
So we have found that 0.6E = R. We can substitute this into either equation to solve for E or R. For example, using the second equation:
B = 0.8(R + 0.4E)
B = 0.8(0.6E + 0.4E)
B = 0.8E
So we have found that B is 0.8 times E. This means that either (a) or (b) can be true depending on the values of E and R:
(a) If E = 1 and R = 0.6, then R + 0.4E = 1 and B = 0.8E = 0.8, which satisfies the conditions.
(b) If E = 0 and R = 0, then R + 0.4E = 0 and B = 0, which also satisfies the conditions.
- "40% of a number E": this can be written as 0.4E
- "added to another number R": we add 0.4E to R, so we get R + 0.4E
- "B becomes 125% of its previous value": if we call the previous value of B "B0", then we have B = 1.25B0
Putting it all together, we can write:
R + 0.4E = 1.25B0
But we don't know anything about B0, so we need to find another equation to solve for E and R. We can use the fact that B is a certain percentage of its previous value:
B = 1.25B0 = 1.25(B/1.25) = B/0.8
This means that B is 0.8 times its current value. So we can write:
B = 0.8(R + 0.4E)
Now we have two equations with two unknowns, E and R:
R + 0.4E = 1.25B0
B = 0.8(R + 0.4E)
We can solve for E by substituting the second equation into the first:
R + 0.4E = 1.25(0.8(R + 0.4E))
Simplifying:
R + 0.4E = R + 1.0E
0.6E = R
So we have found that 0.6E = R. We can substitute this into either equation to solve for E or R. For example, using the second equation:
B = 0.8(R + 0.4E)
B = 0.8(0.6E + 0.4E)
B = 0.8E
So we have found that B is 0.8 times E. This means that either (a) or (b) can be true depending on the values of E and R:
(a) If E = 1 and R = 0.6, then R + 0.4E = 1 and B = 0.8E = 0.8, which satisfies the conditions.
(b) If E = 0 and R = 0, then R + 0.4E = 0 and B = 0, which also satisfies the conditions.
In a group of people, 28% of the members are young while the rest are old. If 65% of the members are literates, and 25% of the literates are young, then the percentage of old people among the illiterates is nearest to
- a)62
- b)55
- c)66
- d)59
Correct answer is option 'C'. Can you explain this answer?
In a group of people, 28% of the members are young while the rest are old. If 65% of the members are literates, and 25% of the literates are young, then the percentage of old people among the illiterates is nearest to
a)
62
b)
55
c)
66
d)
59
|
S.S Career Academy answered |
Let ‘x’ be the strength of group G. Based on the information, 0.65x constitutes of literate people {the rest 0.35x = illiterate}
Of this 0.65x , 75% are old people =(0.75)0.65x old literates.
The total number of old people in group G is 0.72x {72% of the total}.
Thus, the total number of old people who are illiterate = 0.72x - 0.4875x = 0.2325x.
This is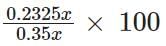
≈ 66& of the total number of illiterates.
Hence, Option C is the correct answer.
Of this 0.65x , 75% are old people =(0.75)0.65x old literates.
The total number of old people in group G is 0.72x {72% of the total}.
Thus, the total number of old people who are illiterate = 0.72x - 0.4875x = 0.2325x.
This is
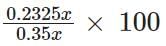
≈ 66& of the total number of illiterates.
Hence, Option C is the correct answer.
Gauri went to the stationers and bought things worth Rs. 25, out of which 30 paise went on sales tax on taxable purchases. If the tax rate was 6%, then what was the cost of the tax free items?- a)Rs. 15
- b)Rs. 15.70
- c)Rs. 19.70
- d)Rs. 20
Correct answer is option 'C'. Can you explain this answer?
Gauri went to the stationers and bought things worth Rs. 25, out of which 30 paise went on sales tax on taxable purchases. If the tax rate was 6%, then what was the cost of the tax free items?
a)
Rs. 15
b)
Rs. 15.70
c)
Rs. 19.70
d)
Rs. 20
|
|
Raghavendra Sharma answered |
Anil buys 12 toys and labels each with the same selling price. He sells 8 toys initially at 20% discount on the labeled price. Then he sells the remaining 4 toys at an additional 25% discount on the discounted price. Thus, he gets a total of Rs 2112, and makes a 10% profit. With no discounts, his percentage of profit would have been- a)55
- b)60
- c)54
- d)50
Correct answer is option 'D'. Can you explain this answer?
Anil buys 12 toys and labels each with the same selling price. He sells 8 toys initially at 20% discount on the labeled price. Then he sells the remaining 4 toys at an additional 25% discount on the discounted price. Thus, he gets a total of Rs 2112, and makes a 10% profit. With no discounts, his percentage of profit would have been
a)
55
b)
60
c)
54
d)
50
|
|
Upsc Rank Holders answered |
Let the CP of the each toy be “x”. CP of 12 toys will be “12x”. Now the shopkeeper made a 10% profit on CP. This means that
12x(1.1)= 2112 or x=160 . Hence the CP of each toy is ₹160.
Now let the SP of each toy be “m”. Now he sold 8 toys at 20% discount. This means that 8m(0.8) or 6.4m
He sold 4 toys at an additional 25% discount. 4m(0.8)(0.75)=2.4m Now 6.4m+2.4m=8.8m=2112 or m=240
Hence CP= 160 and SP=240. Hence profit percentage is 50%.
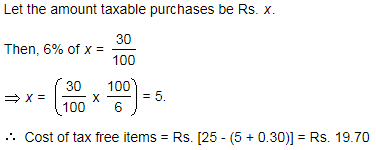
If A = x% of y and B = y% of x, then which of the following is true?
- a)A is smaller than B
- b)A is greater than B
- c)Relationship between A and B cannot be determined
- d)None of these
Correct answer is option 'D'. Can you explain this answer?
a)
A is smaller than B
b)
A is greater than B
c)
Relationship between A and B cannot be determined
d)
None of these
|
Sagar Sharma answered |
Explanation:
To determine the relationship between A and B, we need to understand the meaning of "x% of y" and "y% of x".
- "x% of y" means x% times y, which can be expressed as (x/100) * y.
- "y% of x" means y% times x, which can be expressed as (y/100) * x.
Let's substitute these expressions into the given equations:
A = (x/100) * y
B = (y/100) * x
Comparing A and B:
To compare A and B, we can simplify the expressions:
A = (x/100) * y = xy/100
B = (y/100) * x = xy/100
As we can see, A and B have the same value, xy/100. Therefore, A is equal to B.
Conclusion:
From the given equations and the comparison of A and B, we can conclude that A is equal to B. None of the given options (a, b, or c) is true.
Therefore, the correct answer is option 'D' - None of these.
To determine the relationship between A and B, we need to understand the meaning of "x% of y" and "y% of x".
- "x% of y" means x% times y, which can be expressed as (x/100) * y.
- "y% of x" means y% times x, which can be expressed as (y/100) * x.
Let's substitute these expressions into the given equations:
A = (x/100) * y
B = (y/100) * x
Comparing A and B:
To compare A and B, we can simplify the expressions:
A = (x/100) * y = xy/100
B = (y/100) * x = xy/100
As we can see, A and B have the same value, xy/100. Therefore, A is equal to B.
Conclusion:
From the given equations and the comparison of A and B, we can conclude that A is equal to B. None of the given options (a, b, or c) is true.
Therefore, the correct answer is option 'D' - None of these.
Meena scores 40% in an examination and after review, even though her score is increased by 50%, she fails by 35 marks. If her post-review score is increased by 20%, she will have 7 marks more than the passing score. The percentage score needed for passing the examination is- a)70
- b) 80
- c)60
- d) 75
Correct answer is option 'A'. Can you explain this answer?
Meena scores 40% in an examination and after review, even though her score is increased by 50%, she fails by 35 marks. If her post-review score is increased by 20%, she will have 7 marks more than the passing score. The percentage score needed for passing the examination is
a)
70
b)
80
c)
60
d)
75
|
|
S.S Career Academy answered |
Assuming the maximum marks =100a, then Meena got 40a
After increasing her score by 50%, she will get 40a(1+50/100)=60a
Passing score = 60a+35
Post review score after 20% increase = 60a*1.2=72a
=>Hence, 60a+35+7=72a
=>12a=42 =>a=3.5
=> maximum marks = 350 and passing marks = 210+35=245
=> Passing percentage = 245*100/350 = 70
If equal numbers of people are born on each day, find the approximate percentage of the people whose birthday will fall on 29th February. If we are to consider people born in 20th century (1901-2000) and assuming no deaths.
- a)0.0684
- b)0.5732
- c)0.374
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
If equal numbers of people are born on each day, find the approximate percentage of the people whose birthday will fall on 29th February. If we are to consider people born in 20th century (1901-2000) and assuming no deaths.
a)
0.0684
b)
0.5732
c)
0.374
d)
None of these
|
|
Esha singh answered |
Approximate Percentage of People with Birthday on 29th February
Given that equal numbers of people are born on each day and we are considering people born in the 20th century (1901-2000) with no deaths, we can calculate the approximate percentage of people whose birthday falls on 29th February.
First, let's calculate the total number of days in the 20th century:
Number of Years = 2000 - 1901 + 1 = 100
Total Number of Days = Number of Years * 365 = 100 * 365 = 36500
Next, let's calculate the number of leap years in the 20th century:
Number of Leap Years = Number of Years / 4 = 100 / 4 = 25
Since 29th February occurs only in leap years, we need to consider the number of people born on this day during the 20th century. To find this, we multiply the number of leap years by the number of people born on each day:
Number of People Born on 29th February = Number of Leap Years * Number of People Born Each Day
Since equal numbers of people are born on each day, we can assume that the number of people born on 29th February is the same as the number of people born on any other day. Therefore:
Number of People Born on 29th February = Number of Leap Years * Number of People Born Each Day = 25 * (Total Number of People / Total Number of Days)
Now, let's calculate the percentage of people born on 29th February:
Percentage of People Born on 29th February = (Number of People Born on 29th February / Total Number of People) * 100
Substituting the values:
Percentage of People Born on 29th February = (25 * (Total Number of People / Total Number of Days) / Total Number of People) * 100
Simplifying:
Percentage of People Born on 29th February = (25 / Total Number of Days) * 100
Since we are considering people born in the 20th century, the total number of days is 36500, so:
Percentage of People Born on 29th February = (25 / 36500) * 100 ≈ 0.0685 ≈ 0.374%
Therefore, the approximate percentage of people whose birthday falls on 29th February is approximately 0.374%, which matches option 'A'.
Given that equal numbers of people are born on each day and we are considering people born in the 20th century (1901-2000) with no deaths, we can calculate the approximate percentage of people whose birthday falls on 29th February.
First, let's calculate the total number of days in the 20th century:
Number of Years = 2000 - 1901 + 1 = 100
Total Number of Days = Number of Years * 365 = 100 * 365 = 36500
Next, let's calculate the number of leap years in the 20th century:
Number of Leap Years = Number of Years / 4 = 100 / 4 = 25
Since 29th February occurs only in leap years, we need to consider the number of people born on this day during the 20th century. To find this, we multiply the number of leap years by the number of people born on each day:
Number of People Born on 29th February = Number of Leap Years * Number of People Born Each Day
Since equal numbers of people are born on each day, we can assume that the number of people born on 29th February is the same as the number of people born on any other day. Therefore:
Number of People Born on 29th February = Number of Leap Years * Number of People Born Each Day = 25 * (Total Number of People / Total Number of Days)
Now, let's calculate the percentage of people born on 29th February:
Percentage of People Born on 29th February = (Number of People Born on 29th February / Total Number of People) * 100
Substituting the values:
Percentage of People Born on 29th February = (25 * (Total Number of People / Total Number of Days) / Total Number of People) * 100
Simplifying:
Percentage of People Born on 29th February = (25 / Total Number of Days) * 100
Since we are considering people born in the 20th century, the total number of days is 36500, so:
Percentage of People Born on 29th February = (25 / 36500) * 100 ≈ 0.0685 ≈ 0.374%
Therefore, the approximate percentage of people whose birthday falls on 29th February is approximately 0.374%, which matches option 'A'.
If a certain weight of an alloy of silver and copper is mixed with 3 kg of pure silver, the resulting alloy will have 90% silver by weight. If the same weight of the initial alloy is mixed with 2 kg of another alloy which has 90% silver by weight, the resulting alloy will have 84% silver by weight. Then, the weight of the initial alloy, in kg, is
- a)3.5
- b)2
- c)2.5
- d)3
Correct answer is option 'D'. Can you explain this answer?
If a certain weight of an alloy of silver and copper is mixed with 3 kg of pure silver, the resulting alloy will have 90% silver by weight. If the same weight of the initial alloy is mixed with 2 kg of another alloy which has 90% silver by weight, the resulting alloy will have 84% silver by weight. Then, the weight of the initial alloy, in kg, is
a)
3.5
b)
2
c)
2.5
d)
3
|
Glance Learning Institute answered |
Let the alloy contain x Kg silver and y kg copper
Now when mixed with 3Kg Pure silver
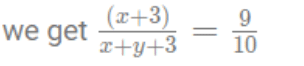
we get 10x+30 =9x+9y+27
9y-x=3 (1)
Now as per condition 2
silver in 2nd alloy = 2(0.9) =1.8
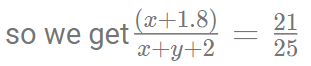
we get 21y-4x =3 (2)
solving (1) and (2) we get y= 0.6 and x =2.4
so x+y = 3
Now when mixed with 3Kg Pure silver
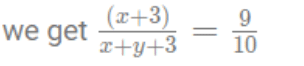
we get 10x+30 =9x+9y+27
9y-x=3 (1)
Now as per condition 2
silver in 2nd alloy = 2(0.9) =1.8
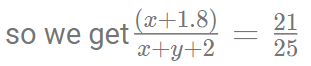
we get 21y-4x =3 (2)
solving (1) and (2) we get y= 0.6 and x =2.4
so x+y = 3
Find 8.33% of 252.- a)18
- b)21
- c)23
- d)22.5
Correct answer is option 'B'. Can you explain this answer?
Find 8.33% of 252.
a)
18
b)
21
c)
23
d)
22.5
|
|
Harsh Kothari answered |
It's easy first take 8% of 252 and then add 0.33% of 252 and the answer is nearby 20.9 i.e 21 option b
Which of the following is correct statement ?- a)Na2S is Sodium sulphide, Na2S03 is Sodium sulphite and Na2S04 is Sodium sulphate
- b)Na2S is Sodium sulphite, Na2S03 is Sodium sulphide and Na2S04 is Sodium sulphate
- c)Na2S is Sodium sulphite, Na2S03 is Sodium sulphate and Na2S04 is Sodium sulphide
- d)Na2S is Sodium sulphide , Na2S03 is Sodium sulphate and Na2S04 is Sodium thiosulphate
Correct answer is option 'A'. Can you explain this answer?
Which of the following is correct statement ?
a)
Na2S is Sodium sulphide, Na2S03 is Sodium sulphite and Na2S04 is Sodium sulphate
b)
Na2S is Sodium sulphite, Na2S03 is Sodium sulphide and Na2S04 is Sodium sulphate
c)
Na2S is Sodium sulphite, Na2S03 is Sodium sulphate and Na2S04 is Sodium sulphide
d)
Na2S is Sodium sulphide , Na2S03 is Sodium sulphate and Na2S04 is Sodium thiosulphate
|
Saranya Sengupta answered |
Na2S is Sodium sulphide, Na2S03 is Sodium sulphite, and Na2S04 is Sodium sulphate.
Explanation:
Sodium (Na) is a chemical element with atomic number 11. It belongs to Group 1 of the periodic table and is highly reactive. Sulfur (S) is a chemical element with atomic number 16. It belongs to Group 16 of the periodic table and can form various compounds with different elements.
Sodium sulfide (Na2S):
- Sodium sulfide is an inorganic compound with the formula Na2S.
- It is composed of two sodium (Na) ions and one sulfur (S) ion.
- Sodium sulfide is a colorless solid and it is highly soluble in water.
- It is commonly used in the leather industry for dehairing hides and in the production of dyes and pigments.
Sodium sulphite (Na2SO3):
- Sodium sulphite is an inorganic compound with the formula Na2SO3.
- It is composed of two sodium (Na) ions, one sulfur (S) ion, and three oxygen (O) ions.
- Sodium sulphite is a white crystalline solid and it is soluble in water.
- It is commonly used as a reducing agent in various chemical reactions and as a preservative in food and beverages.
Sodium sulphate (Na2SO4):
- Sodium sulphate is an inorganic compound with the formula Na2SO4.
- It is composed of two sodium (Na) ions, one sulfur (S) ion, and four oxygen (O) ions.
- Sodium sulphate is a white crystalline solid and it is soluble in water.
- It is commonly used in the manufacturing of detergents, glass, and paper.
Based on the above explanations, it can be concluded that option 'A' is the correct statement. Na2S represents sodium sulfide, Na2SO3 represents sodium sulphite, and Na2SO4 represents sodium sulphate.
Explanation:
Sodium (Na) is a chemical element with atomic number 11. It belongs to Group 1 of the periodic table and is highly reactive. Sulfur (S) is a chemical element with atomic number 16. It belongs to Group 16 of the periodic table and can form various compounds with different elements.
Sodium sulfide (Na2S):
- Sodium sulfide is an inorganic compound with the formula Na2S.
- It is composed of two sodium (Na) ions and one sulfur (S) ion.
- Sodium sulfide is a colorless solid and it is highly soluble in water.
- It is commonly used in the leather industry for dehairing hides and in the production of dyes and pigments.
Sodium sulphite (Na2SO3):
- Sodium sulphite is an inorganic compound with the formula Na2SO3.
- It is composed of two sodium (Na) ions, one sulfur (S) ion, and three oxygen (O) ions.
- Sodium sulphite is a white crystalline solid and it is soluble in water.
- It is commonly used as a reducing agent in various chemical reactions and as a preservative in food and beverages.
Sodium sulphate (Na2SO4):
- Sodium sulphate is an inorganic compound with the formula Na2SO4.
- It is composed of two sodium (Na) ions, one sulfur (S) ion, and four oxygen (O) ions.
- Sodium sulphate is a white crystalline solid and it is soluble in water.
- It is commonly used in the manufacturing of detergents, glass, and paper.
Based on the above explanations, it can be concluded that option 'A' is the correct statement. Na2S represents sodium sulfide, Na2SO3 represents sodium sulphite, and Na2SO4 represents sodium sulphate.
Chapter doubts & questions for Percentages - CSAT Preparation 2025 is part of UPSC CSE exam preparation. The chapters have been prepared according to the UPSC CSE exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for UPSC CSE 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Percentages - CSAT Preparation in English & Hindi are available as part of UPSC CSE exam.
Download more important topics, notes, lectures and mock test series for UPSC CSE Exam by signing up for free.
CSAT Preparation
210 videos|138 docs|138 tests
|
Signup to see your scores go up within 7 days!
Study with 1000+ FREE Docs, Videos & Tests
10M+ students study on EduRev
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup