All Exams >
UPSC >
CSAT Preparation >
All Questions
All questions of Mensuration: Volume, Surface Area & Solid Figures for UPSC CSE Exam
ABCD is a square of side 10 cm. What is the area of the least-sized square that may be inscribed in ABCD with its vertices on the sides of ABCD?- a)0 cm2
- b)25 cm2
- c)50 cm2
- d)66.66 cm2
Correct answer is option 'A'. Can you explain this answer?
ABCD is a square of side 10 cm. What is the area of the least-sized square that may be inscribed in ABCD with its vertices on the sides of ABCD?
a)
0 cm2
b)
25 cm2
c)
50 cm2
d)
66.66 cm2

|
Athira Sen answered |
The vertices of the smaller square will be on the midpoints of the sides of the larger square.
Cylindrical cans of cricket balls are to be packed in a box. Each can has a radius of 7 cm and height of 30 cm. Dimension of the box is l = 76 cm, b = 46 cm, h = 45 cm. What is the maximum number of cans that can fit in the box?- a)15
- b)17
- c)22
- d)21
Correct answer is option 'D'. Can you explain this answer?
Cylindrical cans of cricket balls are to be packed in a box. Each can has a radius of 7 cm and height of 30 cm. Dimension of the box is l = 76 cm, b = 46 cm, h = 45 cm. What is the maximum number of cans that can fit in the box?
a)
15
b)
17
c)
22
d)
21

|
Naroj Boda answered |
This question requires a good deal of visualization. Since, both the box and cans are hard solids, simply dividing the volume won’t work because the shape can’t be deformed.
Each cylindrical can has a diameter of 14 cm and while they are kept erect in the box will occupy height of 30 cm
Number of such cans that can be placed in a row
Number of such rows that can be placed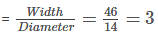
Thus 5 x 3 = 15 cans can be placed in an erect position.
However, height of box = 45cm and only 30 cm has been utilized so far
Remaining height = 15 cm > 14 cm (Diameter of the can)
So, some cans can be placed horizontally on the base.
Number of cans in horizontal row
Number of such rows
∴ 2 x 3 = 6 cans can be placed horizontally
∴ Maximum number of cans = 15+6 = 21
Choice (D) is therefore, the correct answer.
Each cylindrical can has a diameter of 14 cm and while they are kept erect in the box will occupy height of 30 cm
Number of such cans that can be placed in a row
Number of such rows that can be placed
Thus 5 x 3 = 15 cans can be placed in an erect position.
However, height of box = 45cm and only 30 cm has been utilized so far
Remaining height = 15 cm > 14 cm (Diameter of the can)
So, some cans can be placed horizontally on the base.
Number of cans in horizontal row
Number of such rows
∴ 2 x 3 = 6 cans can be placed horizontally
∴ Maximum number of cans = 15+6 = 21
Choice (D) is therefore, the correct answer.
What is the area of an equilateral triangle of side 16 cm?- a)48√3 cm2
- b)128√3 cm2
- c)9.6√3 cm2
- d)64√3 cm2
Correct answer is option 'D'. Can you explain this answer?
What is the area of an equilateral triangle of side 16 cm?
a)
48√3 cm2
b)
128√3 cm2
c)
9.6√3 cm2
d)
64√3 cm2
|
|
Vt Sir - Kota answered |
Area of an equilateral triangle = √3/4 S2
If S = 16, Area of triangle = √3/4 * 16 * 16 = 64√3 cm2
An inverted right circular cone has a radius of 9 cm. This cone is partly filled with oil which is dipping from a hole in the tip at a rate of 1cm2/hour. Currently the level of oil 3 cm from top and surface area is 36π cm2. How long will it take the cone to be completely empty?- a)216π hours
- b)1 hour
- c)3 hours
- d)36π hours
Correct answer is option 'A'. Can you explain this answer?
An inverted right circular cone has a radius of 9 cm. This cone is partly filled with oil which is dipping from a hole in the tip at a rate of 1cm2/hour. Currently the level of oil 3 cm from top and surface area is 36π cm2. How long will it take the cone to be completely empty?
a)
216π hours
b)
1 hour
c)
3 hours
d)
36π hours
|
|
Aarav Sharma answered |
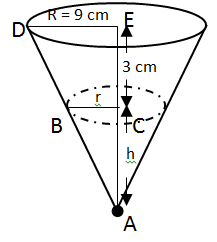
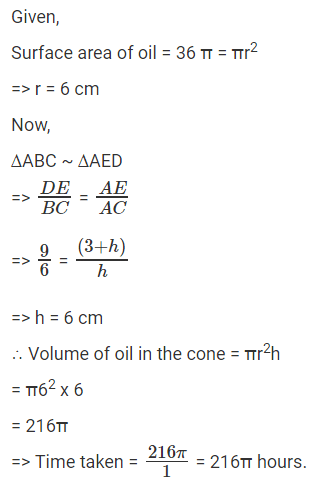
There is a right circular cone with base radius 3 units and height 4 units. The surface of this right circular cone is painted. It is then cut into two parts by a plane parallel to the base so that the volume of the top part (the small cone) divided by the volume of the frustum equals the painted area of the top part divided by the painted area of the bottom part. The height of the small cone is- a)7/3
- b)5/4
- c)5/2
- d)None of these
Correct answer is option 'C'. Can you explain this answer?
There is a right circular cone with base radius 3 units and height 4 units. The surface of this right circular cone is painted. It is then cut into two parts by a plane parallel to the base so that the volume of the top part (the small cone) divided by the volume of the frustum equals the painted area of the top part divided by the painted area of the bottom part. The height of the small cone is
a)
7/3
b)
5/4
c)
5/2
d)
None of these
|
|
Kajal Kumar answered |
Take the total surface area of the initial cone into consideration and then proceed.
PQRST is a pentagon in which all the interior angles are unequal. A circle of radius ‘r’ is inscribed in each of the vertices. Find the area of portion of circles falling inside the pentagon. - a)πr2
- b)1.5πr2
- c)2πr2
- d)1.25πr2
Correct answer is option 'B'. Can you explain this answer?
PQRST is a pentagon in which all the interior angles are unequal. A circle of radius ‘r’ is inscribed in each of the vertices. Find the area of portion of circles falling inside the pentagon.
a)
πr2
b)
1.5πr2
c)
2πr2
d)
1.25πr2
|
|
Preeti Khanna answered |
Since neither angles nor sides are given in the question, immediately the sum of angles of pentagon should come in mind. To use it,
We know the area of the sectors of a circle is given as,
We know the area of the sectors of a circle is given as,
Note => The above concept is applicable for a polygon of n sides.
Choice (B) is therefore, the correct answer.
Correct Answer: 1.5πr2
Choice (B) is therefore, the correct answer.
Correct Answer: 1.5πr2
A sphere of radius r is cut by a plane at a distance of h from its center, thereby breaking this sphere into two different pieces. The cumulative surface area of these two pieces is 25% more than that of the sphere. Find h.- a)
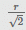
- b)
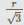
- c)
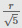
- d)
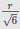
Correct answer is option 'A'. Can you explain this answer?
A sphere of radius r is cut by a plane at a distance of h from its center, thereby breaking this sphere into two different pieces. The cumulative surface area of these two pieces is 25% more than that of the sphere. Find h.
a)
b)
c)
d)
|
|
Anaya Patel answered |
Area = 4πr2
Four spheres each of radius 10 cm lie on a horizontal table so that the centres of the spheres form a square of side 20 cm. A fifth sphere also of radius 10 cm is placed on them so that it touches each of these spheres without disturbing them. How many cm above the table is the centre of the fifth sphere?- a)10√6
- b)10(1 + √2)
- c)10(1 + √3)
- d)10(4 - √2)
Correct answer is option 'B'. Can you explain this answer?
Four spheres each of radius 10 cm lie on a horizontal table so that the centres of the spheres form a square of side 20 cm. A fifth sphere also of radius 10 cm is placed on them so that it touches each of these spheres without disturbing them. How many cm above the table is the centre of the fifth sphere?
a)
10√6
b)
10(1 + √2)
c)
10(1 + √3)
d)
10(4 - √2)

|
Hridoy Mehra answered |
OA= 10
OA= 10
To find the value of PA, go through the options now.
To find the value of PA, go through the options now.
PQRS is a circle and circles are drawn with PO, QO, RO and SO as diameters. Areas A and B are marked. A/B is equal to: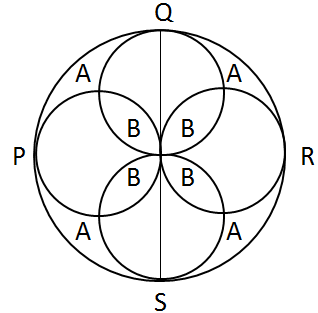
- a)π
- b)1
- c)π/4
- d)2
Correct answer is option 'B'. Can you explain this answer?
PQRS is a circle and circles are drawn with PO, QO, RO and SO as diameters. Areas A and B are marked. A/B is equal to:
a)
π
b)
1
c)
π/4
d)
2
|
|
Preeti Khanna answered |
Such questions are all about visualization and ability to write one area in terms of others.
Here,
Let the radius of PQRS be 2r
∴ Radius of each of the smaller circles = 2r/2 = r
∴ Area A can be written as
A = π (2r)2 – 4 x π(r)2 (Area of the four smaller circles) + B (since, B has been counted twice in the previous subtraction)
=) A = 4πr2 - 4πr2 + B
=) A = B
=) A/B = 1
Choice (B) is therefore, the correct answer.
Correct Answer: 1
Here,
Let the radius of PQRS be 2r
∴ Radius of each of the smaller circles = 2r/2 = r
∴ Area A can be written as
A = π (2r)2 – 4 x π(r)2 (Area of the four smaller circles) + B (since, B has been counted twice in the previous subtraction)
=) A = 4πr2 - 4πr2 + B
=) A = B
=) A/B = 1
Choice (B) is therefore, the correct answer.
Correct Answer: 1
Figure above shows a box which has to be completely wrapped with paper. However, a single Sheet of paper need to be used without any tearing. The dimension of the required paper could be 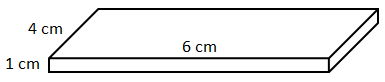
- a)17 cm by 4 cm
- b)12 cm by 6 cm
- c)15 cm by 4 cm
- d)13 cm by 4 cm
Correct answer is option 'B'. Can you explain this answer?
Figure above shows a box which has to be completely wrapped with paper. However, a single Sheet of paper need to be used without any tearing. The dimension of the required paper could be
a)
17 cm by 4 cm
b)
12 cm by 6 cm
c)
15 cm by 4 cm
d)
13 cm by 4 cm

|
Naroj Boda answered |
Total surface area of the box = 2(4x6 + 1x6 + 1x4)
= 2(24 + 6 + 4)
= 68 cm2
As the problem says the paper can’t be torn/cut a portion of paper will need to be fold, so, the area of paper required would be greater than 68 cm2. Only option b) gives the area greater 68 cm2
Choice (B) is therefore, the correct answer.
Correct Answer: 12 cm by 6 cm
= 2(24 + 6 + 4)
= 68 cm2
As the problem says the paper can’t be torn/cut a portion of paper will need to be fold, so, the area of paper required would be greater than 68 cm2. Only option b) gives the area greater 68 cm2
Choice (B) is therefore, the correct answer.
Correct Answer: 12 cm by 6 cm
All five faces of a regular pyramid with a square base are found to be of the same area. The height of the pyramid is 3 cm. The total area of all its surfaces (in cm2) is- a)8
- b)10
- c)12
- d)16
Correct answer is option 'C'. Can you explain this answer?
All five faces of a regular pyramid with a square base are found to be of the same area. The height of the pyramid is 3 cm. The total area of all its surfaces (in cm2) is
a)
8
b)
10
c)
12
d)
16

|
Prateek Menon answered |
Equate the area of the square ABCD and triangle PDC and find a relation between the slant height and the length of the base of the pyramid
A spherical shaped sweet is placed inside a cube of side 5 cm such that the sweet just fits the cube. A fly is sitting on one of the vertices of the cube. What is the shortest distance the fly must travel to reach the sweet?- a)2.5 cm
- b)5(√3 – 1) cm
- c)5(√2 – 1) cm
- d)2.5(√3 – 1) cm
Correct answer is option 'D'. Can you explain this answer?
A spherical shaped sweet is placed inside a cube of side 5 cm such that the sweet just fits the cube. A fly is sitting on one of the vertices of the cube. What is the shortest distance the fly must travel to reach the sweet?
a)
2.5 cm
b)
5(√3 – 1) cm
c)
5(√2 – 1) cm
d)
2.5(√3 – 1) cm

|
Ishani Rane answered |
The question demands visualization. The shortest distance required to be travelled by the fly would be diagonally and be given by:-
Distance =
= (Since diagonal of the cube is given as √3 x l)
= 2.5(√3 – 1)
The area of a rectangular field is 52000 m². This rectangular area has been drawn on a map to the scale 1 cm to 100 m. The length is shown as 3.25 cm on the map. The breadth of the rectangular field is : - a)210 m
- b)150 m
- c)160 m
- d)123 m
Correct answer is option 'C'. Can you explain this answer?
The area of a rectangular field is 52000 m². This rectangular area has been drawn on a map to the scale 1 cm to 100 m. The length is shown as 3.25 cm on the map. The breadth of the rectangular field is :
a)
210 m
b)
150 m
c)
160 m
d)
123 m
|
|
Kavya Saxena answered |
John Nash, an avid mathematician, had his room constructed such that the floor of the room was an equilateral triangle in shape instead of the usual rectangular shape. One day he brought home a bird and tied it to one end of a string and then tied the other end of the string to one of the corners of his room. The next day, he untied the other end of the string from the corner of the room and tied it to a point exactly at the center of the floor of the room. Assuming that the dimensions of the room are relatively large compared to the length of the string, find the number of times, by which the maximum possible space in which the bird can fly, increase.- a)4
- b)5
- c)6
- d)7
Correct answer is option 'B'. Can you explain this answer?
John Nash, an avid mathematician, had his room constructed such that the floor of the room was an equilateral triangle in shape instead of the usual rectangular shape. One day he brought home a bird and tied it to one end of a string and then tied the other end of the string to one of the corners of his room. The next day, he untied the other end of the string from the corner of the room and tied it to a point exactly at the center of the floor of the room. Assuming that the dimensions of the room are relatively large compared to the length of the string, find the number of times, by which the maximum possible space in which the bird can fly, increase.
a)
4
b)
5
c)
6
d)
7

|
Ashwin Chawla answered |
Consider the length of the string less than or equal to the inradius of the floor. At the comer of the floor you will find a sixth part of a hemisphere and at the centre it will be a hemisphere.
Find the number of spheres of the maximum volume that can be accommodated in the above region.- a)324
- b)323
- c)162
- d)161
Correct answer is option 'D'. Can you explain this answer?
Find the number of spheres of the maximum volume that can be accommodated in the above region.
a)
324
b)
323
c)
162
d)
161
|
|
Aarav Sharma answered |
To find the maximum number of spheres that can be accommodated in a given region, we need to consider the volume of the region and the volume of each sphere.
Given information:
- The region is not specified, but we know it can accommodate spheres.
- The volume of each sphere is also not specified.
To solve this problem, we can follow these steps:
1. Determine the volume of the region:
- The volume of the region is not given in the question.
- Without the volume of the region, it is not possible to find the maximum number of spheres that can be accommodated.
- We need more information about the region to proceed.
2. Determine the volume of each sphere:
- The volume of each sphere is not given in the question.
- Without the volume of each sphere, it is not possible to find the maximum number of spheres that can be accommodated.
- We need more information about the spheres to proceed.
Since we do not have sufficient information about the region or the spheres, we cannot determine the maximum number of spheres that can be accommodated. Therefore, none of the provided options (a, b, c, d) can be considered as the correct answer.
To solve this problem, we would need additional information such as the volume of the region and/or the volume of each sphere. Without these details, it is not possible to find the maximum number of spheres that can be accommodated.
Given information:
- The region is not specified, but we know it can accommodate spheres.
- The volume of each sphere is also not specified.
To solve this problem, we can follow these steps:
1. Determine the volume of the region:
- The volume of the region is not given in the question.
- Without the volume of the region, it is not possible to find the maximum number of spheres that can be accommodated.
- We need more information about the region to proceed.
2. Determine the volume of each sphere:
- The volume of each sphere is not given in the question.
- Without the volume of each sphere, it is not possible to find the maximum number of spheres that can be accommodated.
- We need more information about the spheres to proceed.
Since we do not have sufficient information about the region or the spheres, we cannot determine the maximum number of spheres that can be accommodated. Therefore, none of the provided options (a, b, c, d) can be considered as the correct answer.
To solve this problem, we would need additional information such as the volume of the region and/or the volume of each sphere. Without these details, it is not possible to find the maximum number of spheres that can be accommodated.
A right circular cone has height H and radius R. A small cone is cut off at the top by a plane parallel to the base. At what height above the base the section has been made?
Statement (I): H = 20 cm
Statement (II): Volume of small cone: volume of large cone : 1:15- a)If the question can be answered with statement I alone but not statement II alone, or can be answered with statement II alone but not statement I alone.
- b)If the question cannot be answered with statement I alone or with statement II alone, but can be answered if both statements are used together.
- c)If the question can be answered with either statement alone.
- d)If the question cannot be answered with the information provided.
Correct answer is option 'B'. Can you explain this answer?
A right circular cone has height H and radius R. A small cone is cut off at the top by a plane parallel to the base. At what height above the base the section has been made?
Statement (I): H = 20 cm
Statement (II): Volume of small cone: volume of large cone : 1:15
Statement (I): H = 20 cm
Statement (II): Volume of small cone: volume of large cone : 1:15
a)
If the question can be answered with statement I alone but not statement II alone, or can be answered with statement II alone but not statement I alone.
b)
If the question cannot be answered with statement I alone or with statement II alone, but can be answered if both statements are used together.
c)
If the question can be answered with either statement alone.
d)
If the question cannot be answered with the information provided.

|
Ishani Rane answered |
From statement I, we know that the height of the initial cone is 20cm. However, nothing is said about the small cone. Hence, we cannot answer the question using statement A. So, we can eliminate choices (A) and (D).
We are down to choices (A), (B) or (D).
From Statement II, we know that the ratio of the volume of the small cone to that of the large cone is 1 : 15.
i.e. *π*r2*h : *π*R2*H is 1 : 15 (r is the base radius of the smaller cone and h is the height of the smaller cone)
or r2 * h : R2 * H is 1 : 15
From this information, we will not be able to find the answer to h. Hence, we can eliminate choice (A).
Combining the information in the two statements:
When a section is made the two cones are similar triangles. so =
R =
We know H = 20
h = * r
i.e., h3 = H3. Substituting H = 20, we can get the value for h.
Choice (B) is therefore, the correct answer.
Correct Answer: If the question cannot be answered with statement I alone or with statement II alone, but can be answered if both statements are used together.
An order was placed for the supply of a carpet whose breadth was 6 m and length was 1.44 times the breadth. What be the cost of a carpet whose length and breadth are 40% more and 25% more respectively than the first carpet. Given that the ratio of carpet is Rs. 45 per sq m?- a)Rs. 4082.40
- b)Rs. 3868.80
- c)Rs. 4216.20
- d)Rs. 3642.40
Correct answer is option 'A'. Can you explain this answer?
An order was placed for the supply of a carpet whose breadth was 6 m and length was 1.44 times the breadth. What be the cost of a carpet whose length and breadth are 40% more and 25% more respectively than the first carpet. Given that the ratio of carpet is Rs. 45 per sq m?
a)
Rs. 4082.40
b)
Rs. 3868.80
c)
Rs. 4216.20
d)
Rs. 3642.40
|
|
Aarav Sharma answered |
Given:
- Breadth of the first carpet = 6 m
- Length of the first carpet = 1.44 times the breadth
To find:
- Cost of a carpet whose length and breadth are 40% more and 25% more respectively than the first carpet
Formula:
- Area of a rectangle = Length × Breadth
Calculation:
1. Length of the first carpet:
- Length = 1.44 × Breadth
- Length = 1.44 × 6
- Length = 8.64 m
2. Area of the first carpet:
- Area = Length × Breadth
- Area = 8.64 × 6
- Area = 51.84 sq m
3. Increased length and breadth of the second carpet:
- Length = 1.4 × Length of the first carpet
- Length = 1.4 × 8.64
- Length = 12.096 m
- Breadth = 1.25 × Breadth of the first carpet
- Breadth = 1.25 × 6
- Breadth = 7.5 m
4. Area of the second carpet:
- Area = Length × Breadth
- Area = 12.096 × 7.5
- Area = 90.72 sq m
5. Cost of the carpet:
- Cost per sq m = Rs. 45
- Cost of the first carpet = Area of the first carpet × Cost per sq m
- Cost of the first carpet = 51.84 × 45
- Cost of the first carpet = Rs. 2332.80
- Cost of the second carpet = Area of the second carpet × Cost per sq m
- Cost of the second carpet = 90.72 × 45
- Cost of the second carpet = Rs. 4082.40
Therefore, the cost of the carpet whose length and breadth are 40% more and 25% more respectively than the first carpet is Rs. 4082.40, which is option A.
- Breadth of the first carpet = 6 m
- Length of the first carpet = 1.44 times the breadth
To find:
- Cost of a carpet whose length and breadth are 40% more and 25% more respectively than the first carpet
Formula:
- Area of a rectangle = Length × Breadth
Calculation:
1. Length of the first carpet:
- Length = 1.44 × Breadth
- Length = 1.44 × 6
- Length = 8.64 m
2. Area of the first carpet:
- Area = Length × Breadth
- Area = 8.64 × 6
- Area = 51.84 sq m
3. Increased length and breadth of the second carpet:
- Length = 1.4 × Length of the first carpet
- Length = 1.4 × 8.64
- Length = 12.096 m
- Breadth = 1.25 × Breadth of the first carpet
- Breadth = 1.25 × 6
- Breadth = 7.5 m
4. Area of the second carpet:
- Area = Length × Breadth
- Area = 12.096 × 7.5
- Area = 90.72 sq m
5. Cost of the carpet:
- Cost per sq m = Rs. 45
- Cost of the first carpet = Area of the first carpet × Cost per sq m
- Cost of the first carpet = 51.84 × 45
- Cost of the first carpet = Rs. 2332.80
- Cost of the second carpet = Area of the second carpet × Cost per sq m
- Cost of the second carpet = 90.72 × 45
- Cost of the second carpet = Rs. 4082.40
Therefore, the cost of the carpet whose length and breadth are 40% more and 25% more respectively than the first carpet is Rs. 4082.40, which is option A.
The cost of white washing one m2 is Rs 50. What will be the maximum amount saved in painting the room in the most economical way, if the sum of the length, breadth and height is 21 m and all the sides are integers (floor is not to be white washed)?- a)Rs 1,08,050
- b)Rs 8,400
- c)Rs 9,300
- d)Rs 8,540
Correct answer is option 'C'. Can you explain this answer?
The cost of white washing one m2 is Rs 50. What will be the maximum amount saved in painting the room in the most economical way, if the sum of the length, breadth and height is 21 m and all the sides are integers (floor is not to be white washed)?
a)
Rs 1,08,050
b)
Rs 8,400
c)
Rs 9,300
d)
Rs 8,540

|
Hridoy Mehra answered |
The amount saved would be maximum only when the difference of maximum area and minimum area to be painted is maximum.
In the setup of the previous two questions, how is h related to n?- a)h = √2n
- b)h=√17n
- c)h = n
- d)h = √l3n
Correct answer is option 'C'. Can you explain this answer?
In the setup of the previous two questions, how is h related to n?
a)
h = √2n
b)
h=√17n
c)
h = n
d)
h = √l3n

|
Prateek Menon answered |
Equate the lengths of strings obtained from the above two questions.
Under the Indian Posts and Telegraph Act 1885, any package in the form of a right circular cylinder will not be accepted if the sum of its height and the diameter of its base exceeds 10 inches. The height (in inches) of a package of maximum volume that would be accepted is- a)10/3
- b)20/3
- c)10
- d)20
Correct answer is option 'A'. Can you explain this answer?
Under the Indian Posts and Telegraph Act 1885, any package in the form of a right circular cylinder will not be accepted if the sum of its height and the diameter of its base exceeds 10 inches. The height (in inches) of a package of maximum volume that would be accepted is
a)
10/3
b)
20/3
c)
10
d)
20

|
Hridoy Mehra answered |
Volume is maximum when radius is equal to height.
ABCD is a square drawn inside a square PTRS of sides 4 cm by joining midpoints of the sides PR, PT, TS, SR. Another square is drawn inside ABCD similarly. This process is repeated infinite number of times. Find the sum of all the squares.
- a)16 cm2
- b)28 cm2
- c)32 cm2
- d)Infinite
Correct answer is option 'C'. Can you explain this answer?
ABCD is a square drawn inside a square PTRS of sides 4 cm by joining midpoints of the sides PR, PT, TS, SR. Another square is drawn inside ABCD similarly. This process is repeated infinite number of times. Find the sum of all the squares.
a)
16 cm2
b)
28 cm2
c)
32 cm2
d)
Infinite

|
Rajdeep Verma answered |
If we write the infinite series of area of squares:
= 42 + (2√2)2 + 22 + ……. infinite
Since it is a decreasing series sum of infinite terms can be approximated.
= 16 + 8 + 4 +………infinite
A square PQRS has an equilateral triangle PTO inscribed as shown:What is the ratio of AΔPQT to AΔTRU?- a)1 : 3
- b)1 : √3
- c)1 : √2
- d)1 : 2
Correct answer is option 'D'. Can you explain this answer?
A square PQRS has an equilateral triangle PTO inscribed as shown:
What is the ratio of AΔPQT to AΔTRU?
a)
1 : 3
b)
1 : √3
c)
1 : √2
d)
1 : 2

|
Gowri Chakraborty answered |
Let PQ, a side of equilateral triangle be b
By symmetry QT=ST=z (say)
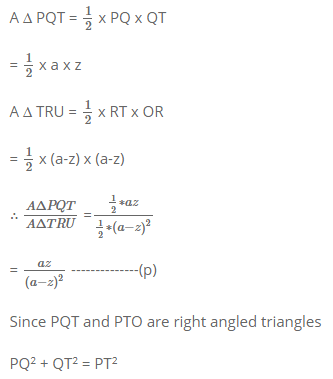
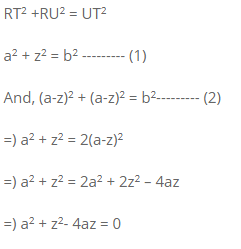
=) a^2 + z^2 – 2az = 2az (Please note how the solution is being managed here. You must always be aware of what you are looking for. Here, as equation -℗ we are looking for (a-z)2 in terms of az)
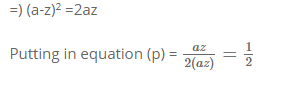
The perimeter of a triangle is 28 cm and the inradius of the triangle is 2.5 cm. What is the area of the triangle?- a)25 cm2
- b)42 cm2
- c)35 cm2
- d)70 cm2
Correct answer is option 'C'. Can you explain this answer?
The perimeter of a triangle is 28 cm and the inradius of the triangle is 2.5 cm. What is the area of the triangle?
a)
25 cm2
b)
42 cm2
c)
35 cm2
d)
70 cm2
|
|
Yash Patel answered |
Area of a triangle = r * s
Where r is the inradius and s is the semi perimeter of the triangle.
Area of triangle = 2.5 * 28/2 = 35 cm2
A cuboid of length 20 m, breadth 15 m and height 12 m is lying on a table. The cuboid is cut into two equal halves by a plane which is perpendicular to the base and passes through a pair of diagonally opposite points of that surface. Then, a second cut is made by a plane which is parallel to the surface of the table again dividing the cuboid into two equal halves. Now this cuboid is divided into four pieces. Out of these four pieces, one piece is now removed from its place. What is the total surface area of the remaining portion of the cuboid?- a)1290 m2
- b)1380 m2
- c)1440 m2
- d)Cannot be determined
Correct answer is option 'D'. Can you explain this answer?
A cuboid of length 20 m, breadth 15 m and height 12 m is lying on a table. The cuboid is cut into two equal halves by a plane which is perpendicular to the base and passes through a pair of diagonally opposite points of that surface. Then, a second cut is made by a plane which is parallel to the surface of the table again dividing the cuboid into two equal halves. Now this cuboid is divided into four pieces. Out of these four pieces, one piece is now removed from its place. What is the total surface area of the remaining portion of the cuboid?
a)
1290 m2
b)
1380 m2
c)
1440 m2
d)
Cannot be determined
|
|
Sagar Sharma answered |
Explanation:
Given Parameters:
- Length of cuboid = 20 m
- Breadth of cuboid = 15 m
- Height of cuboid = 12 m
Step 1: Cutting the Cuboid into Two Equal Halves
- When the cuboid is cut into two equal halves by a plane perpendicular to the base and passing through a pair of diagonally opposite points, we get two equal halves.
- Each half has dimensions: Length = 20 m, Breadth = 15 m, Height = 6 m
Step 2: Cutting the Halves into Two Equal Parts Again
- When the halves are cut by a plane parallel to the table, we get four equal pieces.
- Each piece has dimensions: Length = 20 m, Breadth = 7.5 m, Height = 6 m
Step 3: Removing One Piece
- One piece is removed from the four pieces obtained from the second cut.
Calculating the Total Surface Area of the Remaining Portion
- The total surface area of the remaining portion can't be determined because the exact shape and dimensions of the remaining portion after removing one piece are not specified.
Therefore, the total surface area of the remaining portion of the cuboid cannot be determined.
The square of side 1 cm are cut from four comers of a sheet of tin (having length = 1 and breadth = b) in order to form an open box. If the whole sheet of tin was rolled along its length to form a cylinder, then the volume of the cylinder is equal to (343/4) cm3. Find the volume of the box. (1 and b are integers)- a)154 cm3
- b)100 cm3
- c)126 cm3
- d)Insufficient data
Correct answer is option 'B'. Can you explain this answer?
The square of side 1 cm are cut from four comers of a sheet of tin (having length = 1 and breadth = b) in order to form an open box. If the whole sheet of tin was rolled along its length to form a cylinder, then the volume of the cylinder is equal to (343/4) cm3. Find the volume of the box. (1 and b are integers)
a)
154 cm3
b)
100 cm3
c)
126 cm3
d)
Insufficient data

|
Mrinalini Basu answered |
The length of the rectangle will be equal to the circumference of the base of the cylinder.
The maximum distance between two points of the unit cube is- a)√2 + 1
- b)√2
- c)√3
- d)√2 + √3
Correct answer is option 'C'. Can you explain this answer?
The maximum distance between two points of the unit cube is
a)
√2 + 1
b)
√2
c)
√3
d)
√2 + √3

|
Ashwin Chawla answered |
The distance from any vertex at the base of the cube to the vertex that is perpendicular along height to the diametrically opposite vertex is required.
We have to calculate DF.
We have to calculate DF.
Anil grows tomatoes in his backyard which is in the shape of a square. Each tomato takes 1 cm2 in his backyard. This year, he has been able to grow 131 more tomatoes than last year. The shape of the backyard remained a square. How many tomatoes did Anil produce this year?- a)4225
- b)4096
- c)4356
- d)Insufficient Data
Correct answer is option 'C'. Can you explain this answer?
Anil grows tomatoes in his backyard which is in the shape of a square. Each tomato takes 1 cm2 in his backyard. This year, he has been able to grow 131 more tomatoes than last year. The shape of the backyard remained a square. How many tomatoes did Anil produce this year?
a)
4225
b)
4096
c)
4356
d)
Insufficient Data

|
Naveen Jain answered |
Let the area of backyard be x2 this year and y2 last year
∴ X2- Y2 = 131
=) (X+Y) (X-Y) = 131
Now, 131 is a prime number (a unique one too. Check out its properties on Google). Also, always identify the prime number given in a question. Might be helpful in cracking the solution.
=) (X+Y) (X-Y) = 131 x 1
=) X+Y = 131
X-Y = 1
=) 2X = 132 =) X = 66
and Y = 65
∴ Number of tomatoes produced this year = 662 = 4356
Choice (C) is therefore, the correct answer.
Correct Answer: 4356
∴ X2- Y2 = 131
=) (X+Y) (X-Y) = 131
Now, 131 is a prime number (a unique one too. Check out its properties on Google). Also, always identify the prime number given in a question. Might be helpful in cracking the solution.
=) (X+Y) (X-Y) = 131 x 1
=) X+Y = 131
X-Y = 1
=) 2X = 132 =) X = 66
and Y = 65
∴ Number of tomatoes produced this year = 662 = 4356
Choice (C) is therefore, the correct answer.
Correct Answer: 4356
If the above figure is a 3-D figure and seven spherical balls of radius 6 cm each are tightly arranged in a hexagonal box in a single layer, then what will be the volume of the box unoccupied by the balls in cm3?- a)3456√3+ 5184-252π
- b)3456√3+ 5184-288π
- c)576√3 +864-288π
- d)None of these
Correct answer is option 'D'. Can you explain this answer?
If the above figure is a 3-D figure and seven spherical balls of radius 6 cm each are tightly arranged in a hexagonal box in a single layer, then what will be the volume of the box unoccupied by the balls in cm3?
a)
3456√3+ 5184-252π
b)
3456√3+ 5184-288π
c)
576√3 +864-288π
d)
None of these

|
Dipanjan Kulkarni answered |
The 3D shape of the hexagon will be a hexagonal prism.
What is the ratio of the number of unexposed smaller cubes to the total number of cubes?- a)1/27
- b)
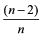
- c)
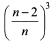
- d)
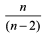
Correct answer is option 'C'. Can you explain this answer?
What is the ratio of the number of unexposed smaller cubes to the total number of cubes?
a)
1/27
b)
c)
d)

|
Hridoy Mehra answered |
Take a value of n, say 3 and then check options.
A string is wound around two circular disk as shown. If the radius of the two disk are 40 cm and 30 cm respectively. What is the total length of the string?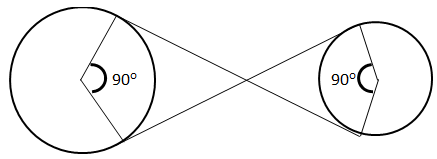
- a)70 cm
- b) 70 + 165*π
- c) 70 + 120π
- d) 70 + 165 * π/2
Correct answer is option 'D'. Can you explain this answer?
A string is wound around two circular disk as shown. If the radius of the two disk are 40 cm and 30 cm respectively. What is the total length of the string?
a)
70 cm
b)
70 + 165*π
c)
70 + 120π
d)
70 + 165 * π/2

|
Bijoy Pillai answered |
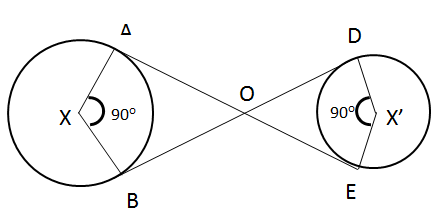
As, tangent from an exterior point makes right angle with the radius.
=) ADXB and ODX’E are squares,
∴ AO = BO = 40 cm
OD = DE = 30 cm
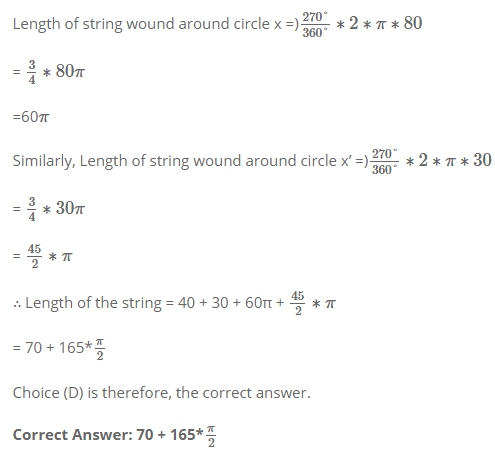
A cube is inscribed in a hemisphere of radius R, such that four of its vertices lie on the base of the hemisphere and the other four touch the hemispherical surface of the half-sphere. What is the volume of the cube?- a)0.25R3
- b)
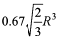
- c)
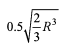
- d)
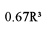
Correct answer is option 'B'. Can you explain this answer?
A cube is inscribed in a hemisphere of radius R, such that four of its vertices lie on the base of the hemisphere and the other four touch the hemispherical surface of the half-sphere. What is the volume of the cube?
a)
0.25R3
b)
c)
d)

|
Dipanjan Kulkarni answered |
Let ABCDEFGH be the cube of side a and O be the centre of the hemisphere.
AC = √2 a
OD = OC = R
Let P be the mid-point of AC
Let P be the mid-point of AC
OP = a
Now in Δ AOC
Three circles with radius 2 cm touch each other as shown :- 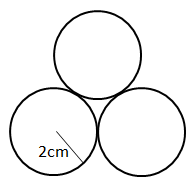
- a)3π(4+√3)2
- b)π/2(4+2√3)2
- c)π/4(4+2√3)2
- d)12π−π/2(4+2√3)2
Correct answer is option 'B'. Can you explain this answer?
Three circles with radius 2 cm touch each other as shown :-
a)
3π(4+√3)2
b)
π/2(4+2√3)2
c)
π/4(4+2√3)2
d)
12π−π/2(4+2√3)2

|
Mainak Majumdar answered |
(This can easily be derived using trigonometry. However, please remember this formula. It is useful at places)
PQRS is a square of sides 2 cm & ST = 2 cm. Also, PT=RT. What is the area of ?PST?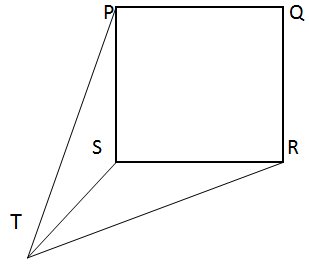
- a)2 cm2
- b)√3 cm2
- c)√2 cm2
- d)
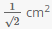
Correct answer is option 'C'. Can you explain this answer?
PQRS is a square of sides 2 cm & ST = 2 cm. Also, PT=RT. What is the area of ?PST?
a)
2 cm2
b)
√3 cm2
c)
√2 cm2
d)
|
|
Rajeev Kumar answered |
Although the figure looks like a 3D figure but on reading through the question, it is clear that the diagram is on a single plane.
Chapter doubts & questions for Mensuration: Volume, Surface Area & Solid Figures - CSAT Preparation 2025 is part of UPSC CSE exam preparation. The chapters have been prepared according to the UPSC CSE exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for UPSC CSE 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Mensuration: Volume, Surface Area & Solid Figures - CSAT Preparation in English & Hindi are available as part of UPSC CSE exam.
Download more important topics, notes, lectures and mock test series for UPSC CSE Exam by signing up for free.
CSAT Preparation
210 videos|138 docs|138 tests
|
Related UPSC CSE Content
Signup to see your scores go up within 7 days!
Study with 1000+ FREE Docs, Videos & Tests
10M+ students study on EduRev

Contact Support
Our team is online on weekdays between 10 AM - 7 PM
Typical reply within 3 hours
|
Free Exam Preparation
at your Fingertips!
Access Free Study Material - Test Series, Structured Courses, Free Videos & Study Notes and Prepare for Your Exam With Ease

 Join the 10M+ students on EduRev
Join the 10M+ students on EduRev
|

|
Forgot Password
OR
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup










