All Exams >
Civil Engineering (CE) >
Engineering Mathematics >
All Questions
All questions of Probability and Statistics for Civil Engineering (CE) Exam
A fair coin is tossed 10 times. What is the probability that ONLY the first two tosses will yield heads? - a)
- b)
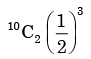
- c)
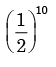
- d)
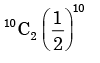
Correct answer is option 'C'. Can you explain this answer?
A fair coin is tossed 10 times. What is the probability that ONLY the first two tosses will yield heads?
a)
b)
c)
d)
|
Rhea Reddy answered |
Let A be the event that first toss is head
And B be the event that second toss is head.
By the given condition rest all 8 tosses should be tail
∴ The probability of getting head in first two cases
An examination consists of two papers, Paper 1 and Paper 2. The probability of failing in Paper 1 is 0.3 and that in Paper 2 is 0.2. Given that a student has failed in Paper 2, the probability of failing in Paper 1 is 0.6. The probability of a student failing in both the papers is- a)0.5
- b)0.18
- c) 0.12
- d) 0.06
Correct answer is option 'C'. Can you explain this answer?
An examination consists of two papers, Paper 1 and Paper 2. The probability of failing in Paper 1 is 0.3 and that in Paper 2 is 0.2. Given that a student has failed in Paper 2, the probability of failing in Paper 1 is 0.6. The probability of a student failing in both the papers is
a)
0.5
b)
0.18
c)
0.12
d)
0.06
|
Aditya Chavan answered |
Let A be the event that ‘failed in paper 1’.
B be the event that ‘failed in paper 2’.
Given P(A) = 0.3, P(B) = 0.2.
A fair dice is rolled twice. The probability that an odd number will follow an even number is - a)1/2
- b)1/6
- c)1/3
- d)1/4
Correct answer is option 'D'. Can you explain this answer?
A fair dice is rolled twice. The probability that an odd number will follow an even number is
a)
1/2
b)
1/6
c)
1/3
d)
1/4
|
Raghavendra Sharma answered |
Probability of even number =3/6 =1/2
Probability of odd number =3/6 =1/2
Both are independent so probability=1/2.1/2 =1/4
If a variable takes discrete values a + 4, a - 3.5, a - 2.5, a - 3, a - 2, a + 0.5, a + 5 and a - 0.5 where a > 0, then the median of the data set is- a)a - 2.5
- b)a - 1.25
- c)a - 1.5
- d)a - o.75
Correct answer is option 'B'. Can you explain this answer?
If a variable takes discrete values a + 4, a - 3.5, a - 2.5, a - 3, a - 2, a + 0.5, a + 5 and a - 0.5 where a > 0, then the median of the data set is
a)
a - 2.5
b)
a - 1.25
c)
a - 1.5
d)
a - o.75
|
Sanya Agarwal answered |
Given:
The given values = a + 4, a – 3.5, a – 2.5, a – 3, a – 2, a + 0.5, a + 5 and a – 0.5
The given values = a + 4, a – 3.5, a – 2.5, a – 3, a – 2, a + 0.5, a + 5 and a – 0.5
Concept used:
If n is odd
Median = [(n + 1)/2]th observations
If n is even
Median = [(n/2)th + (n/2 + 1)th observations]/2
Calculation:
a + 4, a – 3.5, a – 2.5, a – 3, a – 2, a + 0.5, a + 5 and a – 0.5
Arrange the data in ascending order
⇒ a – 3.5, a – 3, a – 2.5, a – 2, a – 0.5, a + 0.5, a + 4, a + 5
Here, the n is 8, which is even
Median = [(n/2)th + (n/2 + 1)th observations]/2
⇒ [(8/2) + (8/2 + 1)/2] term
⇒ 4th + 5th term
⇒ [(a – 2 + a – 0.5)/2]
⇒ [(2a – 2.5)/2]
⇒ a – 1.25
∴ The median of the data set is a – 1.25
If n is odd
Median = [(n + 1)/2]th observations
If n is even
Median = [(n/2)th + (n/2 + 1)th observations]/2
Calculation:
a + 4, a – 3.5, a – 2.5, a – 3, a – 2, a + 0.5, a + 5 and a – 0.5
Arrange the data in ascending order
⇒ a – 3.5, a – 3, a – 2.5, a – 2, a – 0.5, a + 0.5, a + 4, a + 5
Here, the n is 8, which is even
Median = [(n/2)th + (n/2 + 1)th observations]/2
⇒ [(8/2) + (8/2 + 1)/2] term
⇒ 4th + 5th term
⇒ [(a – 2 + a – 0.5)/2]
⇒ [(2a – 2.5)/2]
⇒ a – 1.25
∴ The median of the data set is a – 1.25
Manish has to travel from A to D changing buses at stops B and C enroute. The maximum waiting time at either stop can be 8 minutes each, but any time of waiting up to 8 minutes is equally likely at both places. He can afford up to 13 minutes of total waiting time if he is to arrive at D on time. What is the probability that Manish will arrive late at D?
- a)9/128
- b)13/64
- c)119/128
- d)8/13
Correct answer is option 'A'. Can you explain this answer?
Manish has to travel from A to D changing buses at stops B and C enroute. The maximum waiting time at either stop can be 8 minutes each, but any time of waiting up to 8 minutes is equally likely at both places. He can afford up to 13 minutes of total waiting time if he is to arrive at D on time. What is the probability that Manish will arrive late at D?
a)
9/128
b)
13/64
c)
119/128
d)
8/13
|
Machine Experts answered |
Two dice are thrown simultaneously. The probability that at least one of them will have 6 facing up is - a)1/36
- b)1/3
- c)25/36
- d)11/36
Correct answer is option 'D'. Can you explain this answer?
Two dice are thrown simultaneously. The probability that at least one of them will have 6 facing up is
a)
1/36
b)
1/3
c)
25/36
d)
11/36
|
|
Ravi Singh answered |
P(atleast one of dice will have 6 facing
= 1 - P(none of dice have 6 facing up)
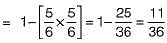
= 1 - P(none of dice have 6 facing up)
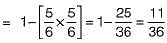
Find the no. of observations between 250 and 300 from the following data:
- a)56
- b)23
- c)15
- d)8
Correct answer is option 'B'. Can you explain this answer?
Find the no. of observations between 250 and 300 from the following data:
a)
56
b)
23
c)
15
d)
8
|
Engineers Adda answered |
Concept:
To find number of observations between 250 and 300.
first we have to draw a frequency distribution table from this data.
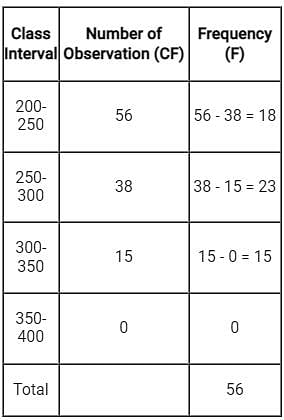
first we have to draw a frequency distribution table from this data.
∴ The Number of observation in between 250-300 = 38 - 15 = 23.
If the arithmetic mean of two numbers is 10 and their geometric mean is 8, the numbers are- a)12, 18
- b)16, 4
- c)15, 5
- d)20, 5
Correct answer is option 'B'. Can you explain this answer?
If the arithmetic mean of two numbers is 10 and their geometric mean is 8, the numbers are
a)
12, 18
b)
16, 4
c)
15, 5
d)
20, 5
|
Arjun Unni answered |
Let the numbers be a and b Then
Solving a + b = 20 and a - b = 12 we get a = 16 and b = 4.
Px(x) = M exp(–2|x|) – N exp(–3 |x|) is the probability density function for the real random variable X, over the entire x axis. M and N are both positive real numbers. The equation relating M and N is - a)
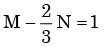
- b)
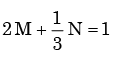
- c)
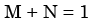
- d)
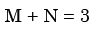
Correct answer is option 'A'. Can you explain this answer?
Px(x) = M exp(–2|x|) – N exp(–3 |x|) is the probability density function for the real random variable X, over the entire x axis. M and N are both positive real numbers. The equation relating M and N is
a)
b)
c)
d)
|
Abhishek Apoorv answered |
Given Px (x ) is the probability density function for the random variable X.
A box contains 2 washers, 3 nuts and 4 bolts. Items are drawn from the box at random one at a time without replacement. The probability of drawing 2 washers first followed by 3 nuts and subsequently the 4 bolts is- a)2/315
- b)1/630
- c)1/1260
- d)1/2520
Correct answer is option 'C'. Can you explain this answer?
A box contains 2 washers, 3 nuts and 4 bolts. Items are drawn from the box at random one at a time without replacement. The probability of drawing 2 washers first followed by 3 nuts and subsequently the 4 bolts is
a)
2/315
b)
1/630
c)
1/1260
d)
1/2520
|
Jaideep Dasgupta answered |
Here sample space = 9
The required probability of drawing 2 washers, 3 nuts and 4 bolts respectively without replac ement
Can you explain the answer of this question below:Let X and Y be two independent random variables. Which one of the relations between expectation (E), variance (Var) and covariance (Cov) given below is FALSE?
- A:
E (XY) = E (X) E (Y)
- B:
Cov (X, Y) = 0
- C:
Var (X + Y) = Var (X) + Var (Y)
- D:
E (X2 y2) = (E (X))2 (E (y))2
The answer is b.
Let X and Y be two independent random variables. Which one of the relations between expectation (E), variance (Var) and covariance (Cov) given below is FALSE?
E (XY) = E (X) E (Y)
Cov (X, Y) = 0
Var (X + Y) = Var (X) + Var (Y)
E (X2 y2) = (E (X))2 (E (y))2
|
Sooraj Krishnan answered |
Option d is the correct one as all the other options hold true
Can you explain the answer of this question below:Three companies X, Y and Z supply computers to a university. The percentage of computers supplied by them and the probability of those being defective are tabulated below
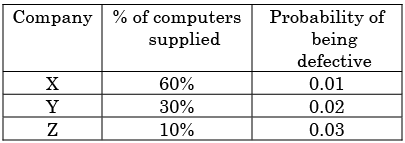
Given that a computer is defective, the probability that it was supplied by Y is
- A:
0. 1
- B:
0.2
- C:
0.3
- D:
0.4
The answer is d.
Three companies X, Y and Z supply computers to a university. The percentage of computers supplied by them and the probability of those being defective are tabulated below
Given that a computer is defective, the probability that it was supplied by Y is
0. 1
0.2
0.3
0.4
|
|
Kabir Verma answered |
Probability of defective computer supplied by Y =
(Case when Y produces defective)/(All cases of producing defective product)
Case when Y produces defective = (0.3)(0.02) = 0.006
All cases of producing defective product= (0.6x0.01)+(0.3x0.02)
(0.1x0.03)= 0.006+0.006+0.003=0.015
(Case when Y produces defective)/(All cases of producing defective product)
Case when Y produces defective = (0.3)(0.02) = 0.006
All cases of producing defective product= (0.6x0.01)+(0.3x0.02)
(0.1x0.03)= 0.006+0.006+0.003=0.015
Probability = 0.006/0.015=0.4
A probability density function is of the form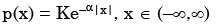 
The value of K is - a)0.5
- b)1
- c)0.5α
- d)α
Correct answer is option 'C'. Can you explain this answer?
A probability density function is of the form
The value of K is
a)
0.5
b)
1
c)
0.5α
d)
α
|
|
Ravi Singh answered |
As (x) is a probability density function
A box contains 20 defective items and 80 non-defective items. If two items are selected at random without replacement, what will be the probability that both items are defective? - a)1/5
- b)1/25
- c)20/99
- d)11/495
Correct answer is option 'D'. Can you explain this answer?
A box contains 20 defective items and 80 non-defective items. If two items are selected at random without replacement, what will be the probability that both items are defective?
a)
1/5
b)
1/25
c)
20/99
d)
11/495
|
Kaavya Sengupta answered |
Total number of items = 100
Number of defective items = 20
Number of Non-defective items = 80
Then the probability that both items are defective, when 2 items are selected at random is,
⇒ P= (20C2x80C0)/(100C2) = 19/495
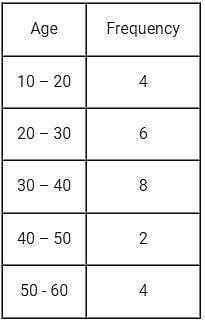
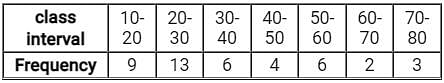
Find the mean of given data:
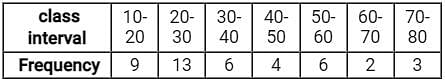
- a)39.95
- b)35.70
- c)43.95
- d)23.95
Correct answer is option 'B'. Can you explain this answer?
Find the mean of given data:
a)
39.95
b)
35.70
c)
43.95
d)
23.95
|
|
Sanya Agarwal answered |
Formula used:
The mean of grouped data is given by,
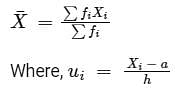
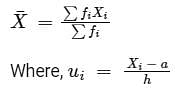
Xi = mean of ith class
fi = frequency corresponding to ith class
Given:
fi = frequency corresponding to ith class
Given:
Calculation:
Now, to calculate the mean of data will have to find ∑fiXi and ∑fi as below,
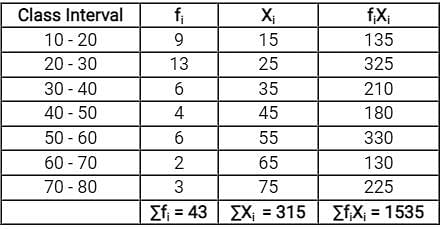
Now, to calculate the mean of data will have to find ∑fiXi and ∑fi as below,
Then,
We know that, mean of grouped data is given by
We know that, mean of grouped data is given by
Hence, the mean of the grouped data is 35.7
Given that E and F are events such that P(E) = 0.6, P(F) = 0.3 and P(E∩F) = 0.2, then P(E|F) ?- a)2/3
- b)1/3
- c)3/4
- d)1/4
Correct answer is option 'A'. Can you explain this answer?
Given that E and F are events such that P(E) = 0.6, P(F) = 0.3 and P(E∩F) = 0.2, then P(E|F) ?
a)
2/3
b)
1/3
c)
3/4
d)
1/4
|
Abhay Banerjee answered |
Solution:
Given data:
- P(E) = 0.6
- P(F) = 0.3
- P(E ∩ F) = 0.2
Calculating P(E|F):
To find the conditional probability P(E|F), we use the formula:
P(E|F) = P(E ∩ F) / P(F)
Substitute the given values into the formula:
P(E|F) = 0.2 / 0.3
P(E|F) = 2/3
Therefore, the correct answer is option 'a) 2/3'.
Given data:
- P(E) = 0.6
- P(F) = 0.3
- P(E ∩ F) = 0.2
Calculating P(E|F):
To find the conditional probability P(E|F), we use the formula:
P(E|F) = P(E ∩ F) / P(F)
Substitute the given values into the formula:
P(E|F) = 0.2 / 0.3
P(E|F) = 2/3
Therefore, the correct answer is option 'a) 2/3'.
In Regression Analysis, if a quantitative variable has 'm' categories, one can introduce- a)Only m + 1 dummy variables
- b)Only m -1 dummy variables
- c)Only m dummy variables
- d)Only 2 m variables
Correct answer is option 'B'. Can you explain this answer?
In Regression Analysis, if a quantitative variable has 'm' categories, one can introduce
a)
Only m + 1 dummy variables
b)
Only m -1 dummy variables
c)
Only m dummy variables
d)
Only 2 m variables
|
Nabanita Saha answered |
Introduction:
Regression analysis is a statistical technique used to model the relationship between a dependent variable and one or more independent variables. In regression analysis, when we have a quantitative variable with m categories, we need to introduce dummy variables to represent these categories in the regression model.
Explanation:
To introduce dummy variables for a quantitative variable with m categories, we need to follow certain rules:
1. Number of Dummy Variables:
We need to introduce m - 1 dummy variables for a quantitative variable with m categories. The reason behind this is the concept of "dummy variable trap" or "multicollinearity".
2. Dummy Variable Trap:
The dummy variable trap occurs when we include a dummy variable for each category of a qualitative variable in the regression model. Including a dummy variable for each category can lead to perfect multicollinearity, where the independent variables are highly correlated. This can result in an unstable regression model with unreliable coefficient estimates.
3. Multicollinearity:
Multicollinearity refers to the situation where two or more independent variables in a regression model are highly correlated with each other. In the case of introducing a dummy variable for each category, one category becomes the reference or baseline category, and the remaining m - 1 categories are represented by dummy variables. As a result, the dummy variables are perfectly correlated with each other, leading to multicollinearity.
4. Baseline Category:
By introducing m - 1 dummy variables, we implicitly define one category as the baseline or reference category. The baseline category is the category for which the coefficients of dummy variables are compared and interpreted. The coefficients of the dummy variables represent the difference in the mean value of the dependent variable between each category and the baseline category.
Conclusion:
In regression analysis, when a quantitative variable has m categories, we introduce m - 1 dummy variables to avoid the dummy variable trap and multicollinearity. By doing so, we can accurately model the relationship between the dependent variable and the quantitative variable with multiple categories.
Regression analysis is a statistical technique used to model the relationship between a dependent variable and one or more independent variables. In regression analysis, when we have a quantitative variable with m categories, we need to introduce dummy variables to represent these categories in the regression model.
Explanation:
To introduce dummy variables for a quantitative variable with m categories, we need to follow certain rules:
1. Number of Dummy Variables:
We need to introduce m - 1 dummy variables for a quantitative variable with m categories. The reason behind this is the concept of "dummy variable trap" or "multicollinearity".
2. Dummy Variable Trap:
The dummy variable trap occurs when we include a dummy variable for each category of a qualitative variable in the regression model. Including a dummy variable for each category can lead to perfect multicollinearity, where the independent variables are highly correlated. This can result in an unstable regression model with unreliable coefficient estimates.
3. Multicollinearity:
Multicollinearity refers to the situation where two or more independent variables in a regression model are highly correlated with each other. In the case of introducing a dummy variable for each category, one category becomes the reference or baseline category, and the remaining m - 1 categories are represented by dummy variables. As a result, the dummy variables are perfectly correlated with each other, leading to multicollinearity.
4. Baseline Category:
By introducing m - 1 dummy variables, we implicitly define one category as the baseline or reference category. The baseline category is the category for which the coefficients of dummy variables are compared and interpreted. The coefficients of the dummy variables represent the difference in the mean value of the dependent variable between each category and the baseline category.
Conclusion:
In regression analysis, when a quantitative variable has m categories, we introduce m - 1 dummy variables to avoid the dummy variable trap and multicollinearity. By doing so, we can accurately model the relationship between the dependent variable and the quantitative variable with multiple categories.
Given the regression lines X + 2Y - 5 = 0, 2X + 3Y - 8 = 0 and Var(X) = 12, the value of Var(Y) is- a)3/4
- b)4/3
- c)16
- d)4
Correct answer is option 'D'. Can you explain this answer?
Given the regression lines X + 2Y - 5 = 0, 2X + 3Y - 8 = 0 and Var(X) = 12, the value of Var(Y) is
a)
3/4
b)
4/3
c)
16
d)
4
|
|
Engineers Adda answered |
Given
Regression lines
x + 2y - 5 = 0
2x + 3y - 8 = 0
var(x)= σx = 12
Calculation
x + 2y - 5 = 0 ------(i)
Let y = - x/2 + 5/2 be the regression line of y on x [ from equation 1]
2x + 3y - 8
x = -(3/2)y + 8/2 be the regressiopn line of x on y
⇒ bxy = -1/2 and byx = -3/2
bxy = Regression line of y on x
byx = Regression line of x on y
We know that regression coefficient = r = √(byx × bxy)
⇒ r = √(-1/2 × -3/2)
∴ r = √3/2 < 1
σx = 12 = 2√3
We know that byx = r (σy/σx)
⇒ -1/2 = √3/2 (σy/2√3)
⇒ σy = - 2
∴ var(y) = variance of y =(-2)2 = 4
The probability that two friends share the same birth-month is - a)1/6
- b)1/12
- c)1/144
- d)1/24
Correct answer is option 'B'. Can you explain this answer?
The probability that two friends share the same birth-month is
a)
1/6
b)
1/12
c)
1/144
d)
1/24
|
Atharva Majumdar answered |
Let A = the event that the birth month of first friend
And B= that of second friend.
∴ P( A )= 1, as 1st friend can born in any month
and P(B) = 1/12, by the condition.
∴ Probability of two friends share same birth-month
Consider the following grouped frequency distribution:
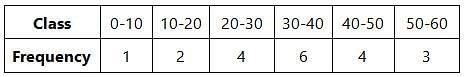What is the median of the distribution ?- a)34
- b)34.5
- c)35
- d)35.5
Correct answer is option 'C'. Can you explain this answer?
Consider the following grouped frequency distribution:
What is the median of the distribution ?
a)
34
b)
34.5
c)
35
d)
35.5
|
|
Sanvi Kapoor answered |
For a distribution, Median
where, L = lower class limit of median class
N = Sum of frequencies
CF = cumulative frequency of class preceding the median class
f = frequency of median class
h = class length of median class.
where, L = lower class limit of median class
N = Sum of frequencies
CF = cumulative frequency of class preceding the median class
f = frequency of median class
h = class length of median class.
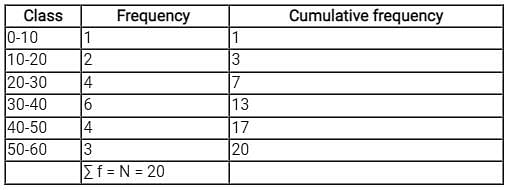
∑f = N = 20
⇒ N/2 = 10
As 7 < 10 < 13, N/2 lies in the class length 30 - 40 by comparing cumulative frequency.
Median class = 30 - 40
⇒ L = 30, N = 60, CF = 7, f = 6, h = 40 - 30 - 10
∴ The correct option is (3).
⇒ N/2 = 10
As 7 < 10 < 13, N/2 lies in the class length 30 - 40 by comparing cumulative frequency.
Median class = 30 - 40
⇒ L = 30, N = 60, CF = 7, f = 6, h = 40 - 30 - 10
∴ The correct option is (3).
If E and F are two events associated with the same sample space of a random experiment then P (E|F) is given by _________- a)P(E∩F) / P(F), provided P(F) ≠ 0
- b)P(E∩F) / P(F), provided P(F) = 0
- c)P(E∩F) / P(F)
- d)P(E∩F) / P(E)
Correct answer is option 'A'. Can you explain this answer?
If E and F are two events associated with the same sample space of a random experiment then P (E|F) is given by _________
a)
P(E∩F) / P(F), provided P(F) ≠ 0
b)
P(E∩F) / P(F), provided P(F) = 0
c)
P(E∩F) / P(F)
d)
P(E∩F) / P(E)
|
|
Engineers Adda answered |
E and F are two events associated with the same sample space of a random experiment.
The value of P (E|F) = (E∩F) / P(F), provided P(F) ≠ 0. We know that if P(F) = 0, then the value of P(E|F) will reach a value which is not defined hence it is wrong option. Also, P(E∩F) / P(F) and P(E∩F) / P(E) are wrong and do not equate to P(E|F).
The value of P (E|F) = (E∩F) / P(F), provided P(F) ≠ 0. We know that if P(F) = 0, then the value of P(E|F) will reach a value which is not defined hence it is wrong option. Also, P(E∩F) / P(F) and P(E∩F) / P(E) are wrong and do not equate to P(E|F).
If P(A) = 7/11, P(B) = 6 / 11 and P(A∪B) = 8/11, then P(A|B) = ________
- a)1
- b)2/3
- c)1/2
- d)5/6
Correct answer is option 'D'. Can you explain this answer?
If P(A) = 7/11, P(B) = 6 / 11 and P(A∪B) = 8/11, then P(A|B) = ________
a)
1
b)
2/3
c)
1/2
d)
5/6
|
|
Rounak Saini answered |
Understanding the Problem
To find P(A|B), we can use the formula for conditional probability:
P(A|B) = P(A ∩ B) / P(B)
Where:
- P(A|B) is the probability of A given B.
- P(A ∩ B) is the probability of both A and B occurring.
- P(B) is the probability of B.
Given Values
- P(A) = 7/11
- P(B) = 6/11
- P(A ∪ B) = 8/11
Finding P(A ∩ B)
Using the formula for the union of two events:
P(A ∪ B) = P(A) + P(B) - P(A ∩ B)
We can rearrange this to find P(A ∩ B):
P(A ∩ B) = P(A) + P(B) - P(A ∪ B)
Now substituting the provided values:
P(A ∩ B) = (7/11) + (6/11) - (8/11)
Calculating P(A ∩ B)
- Combine the fractions:
P(A ∩ B) = (7 + 6 - 8) / 11
P(A ∩ B) = 5/11
Calculating P(A|B)
Now, substitute P(A ∩ B) and P(B) into the conditional probability formula:
P(A|B) = P(A ∩ B) / P(B)
P(A|B) = (5/11) / (6/11)
This simplifies to:
P(A|B) = 5/6
Conclusion
The correct answer is option 'D' (5/6). This indicates that given event B has occurred, the probability of event A occurring is 5/6.
To find P(A|B), we can use the formula for conditional probability:
P(A|B) = P(A ∩ B) / P(B)
Where:
- P(A|B) is the probability of A given B.
- P(A ∩ B) is the probability of both A and B occurring.
- P(B) is the probability of B.
Given Values
- P(A) = 7/11
- P(B) = 6/11
- P(A ∪ B) = 8/11
Finding P(A ∩ B)
Using the formula for the union of two events:
P(A ∪ B) = P(A) + P(B) - P(A ∩ B)
We can rearrange this to find P(A ∩ B):
P(A ∩ B) = P(A) + P(B) - P(A ∪ B)
Now substituting the provided values:
P(A ∩ B) = (7/11) + (6/11) - (8/11)
Calculating P(A ∩ B)
- Combine the fractions:
P(A ∩ B) = (7 + 6 - 8) / 11
P(A ∩ B) = 5/11
Calculating P(A|B)
Now, substitute P(A ∩ B) and P(B) into the conditional probability formula:
P(A|B) = P(A ∩ B) / P(B)
P(A|B) = (5/11) / (6/11)
This simplifies to:
P(A|B) = 5/6
Conclusion
The correct answer is option 'D' (5/6). This indicates that given event B has occurred, the probability of event A occurring is 5/6.
In Normal distribution, the highest value of ordinate occurs at ___________- a)Mean
- b)Variance
- c)Extremes
- d)Same value occurs at all points
Correct answer is option 'A'. Can you explain this answer?
In Normal distribution, the highest value of ordinate occurs at ___________
a)
Mean
b)
Variance
c)
Extremes
d)
Same value occurs at all points
|
Rahul Sen answered |
Understanding Normal Distribution
The normal distribution, also known as the Gaussian distribution, is a fundamental concept in statistics and probability theory. It is characterized by its bell-shaped curve, which is symmetric about the mean.
Highest Ordinate Occurrence
- The highest point of the normal distribution curve is referred to as the ordinate.
- This peak occurs at the mean of the distribution.
Role of the Mean
- The mean is the central value around which data points are distributed.
- In a normal distribution, the mean, median, and mode are all equal and located at the center of the curve.
- The ordinate at the mean is the maximum because it represents the highest frequency of occurrence for the data points.
Characteristics of the Normal Distribution
- The curve is symmetric, meaning that both sides of the mean are mirror images of each other.
- As you move away from the mean towards the extremes, the frequency of occurrence decreases, resulting in lower ordinates.
- Variance, while important in determining the spread of the distribution, does not influence the height of the peak.
Conclusion
The highest ordinate in a normal distribution occurs at the mean, making it a key point in understanding the distribution of data. This characteristic helps in various applications, including civil engineering, where understanding data behavior is crucial for decision-making and analysis.
The normal distribution, also known as the Gaussian distribution, is a fundamental concept in statistics and probability theory. It is characterized by its bell-shaped curve, which is symmetric about the mean.
Highest Ordinate Occurrence
- The highest point of the normal distribution curve is referred to as the ordinate.
- This peak occurs at the mean of the distribution.
Role of the Mean
- The mean is the central value around which data points are distributed.
- In a normal distribution, the mean, median, and mode are all equal and located at the center of the curve.
- The ordinate at the mean is the maximum because it represents the highest frequency of occurrence for the data points.
Characteristics of the Normal Distribution
- The curve is symmetric, meaning that both sides of the mean are mirror images of each other.
- As you move away from the mean towards the extremes, the frequency of occurrence decreases, resulting in lower ordinates.
- Variance, while important in determining the spread of the distribution, does not influence the height of the peak.
Conclusion
The highest ordinate in a normal distribution occurs at the mean, making it a key point in understanding the distribution of data. This characteristic helps in various applications, including civil engineering, where understanding data behavior is crucial for decision-making and analysis.
Two dices are rolled simultaneously. The probability that the sum of digits on the top surface of the two dices is even, is - a)0.5
- b)0.25
- c)0.167
- d)0.125
Correct answer is option 'A'. Can you explain this answer?
Two dices are rolled simultaneously. The probability that the sum of digits on the top surface of the two dices is even, is
a)
0.5
b)
0.25
c)
0.167
d)
0.125
|
Dipika Bose answered |
Here sample space S= 6 × 6 = 36
Total no. of way in which sum of digits on the top surface of the two dice is is even is 18.
∴ The require probability = 0.5
The shape of the normal curve depends on its ___________- a)Mean deviation
- b)Standard deviation
- c)Quartile deviation
- d)Correlation
Correct answer is option 'B'. Can you explain this answer?
The shape of the normal curve depends on its ___________
a)
Mean deviation
b)
Standard deviation
c)
Quartile deviation
d)
Correlation
|
|
Engineers Adda answered |
This can be seen in the pdf of normal distribution where standard deviation is a variable.
Aishwarya studies either computer science or mathematics everyday. if the studies computer science on a day, then the probability that she studies mathematics the next day is 0.6. If she studies mathematics on a day, then the probability that she studies computer science the next day is 0.4. Given that Aishwarya studies computer science on Monday, what is the probability that she studies computer science on Wednesday? - a)0.24
- b)0.36
- c)0.4
- d)0.6
Correct answer is option 'C'. Can you explain this answer?
Aishwarya studies either computer science or mathematics everyday. if the studies computer science on a day, then the probability that she studies mathematics the next day is 0.6. If she studies mathematics on a day, then the probability that she studies computer science the next day is 0.4. Given that Aishwarya studies computer science on Monday, what is the probability that she studies computer science on Wednesday?
a)
0.24
b)
0.36
c)
0.4
d)
0.6
|
|
Bhavya Ahuja answered |
Given information:
- Aishwarya studies either computer science or mathematics every day.
- If Aishwarya studies computer science on a day, the probability that she studies mathematics the next day is 0.6.
- If Aishwarya studies mathematics on a day, the probability that she studies computer science the next day is 0.4.
We are asked to find the probability that Aishwarya studies computer science on Wednesday, given that she studies computer science on Monday.
To solve this problem, we can use conditional probability.
Conditional Probability:
Conditional probability is the probability of an event occurring given that another event has already occurred. It is denoted by P(A|B), where A and B are events.
In this case, we are interested in finding the probability of Aishwarya studying computer science on Wednesday (event A), given that she studies computer science on Monday (event B). So, we need to find P(A|B).
Applying Conditional Probability:
We know that Aishwarya studies either computer science or mathematics every day. So, we can consider these two events as the sample space.
Let's define the events:
- C: Aishwarya studies computer science
- M: Aishwarya studies mathematics
We are given that Aishwarya studies computer science on Monday (event B), so we are interested in finding the probability of Aishwarya studying computer science on Wednesday (event A).
We need to find P(C|C), which is the probability of Aishwarya studying computer science on Wednesday, given that she studied computer science on Monday.
We can use the formula for conditional probability:
P(C|C) = P(C and C) / P(C)
Finding P(C and C):
Since Aishwarya studies either computer science or mathematics every day, the events C and M are mutually exclusive. So, P(C and C) is equal to P(C).
Finding P(C):
We are given that Aishwarya studies computer science on Monday. So, P(C) = 1.
Calculating the Probability:
Using the formula for conditional probability, we have:
P(C|C) = P(C and C) / P(C)
P(C|C) = P(C) / P(C)
P(C|C) = 1 / 1
P(C|C) = 1
Therefore, the probability that Aishwarya studies computer science on Wednesday, given that she studies computer science on Monday, is 1 or 100%.
Answer:
The probability that Aishwarya studies computer science on Wednesday is 0.4 (or 40%). Therefore, the correct answer is option C) 0.4.
- Aishwarya studies either computer science or mathematics every day.
- If Aishwarya studies computer science on a day, the probability that she studies mathematics the next day is 0.6.
- If Aishwarya studies mathematics on a day, the probability that she studies computer science the next day is 0.4.
We are asked to find the probability that Aishwarya studies computer science on Wednesday, given that she studies computer science on Monday.
To solve this problem, we can use conditional probability.
Conditional Probability:
Conditional probability is the probability of an event occurring given that another event has already occurred. It is denoted by P(A|B), where A and B are events.
In this case, we are interested in finding the probability of Aishwarya studying computer science on Wednesday (event A), given that she studies computer science on Monday (event B). So, we need to find P(A|B).
Applying Conditional Probability:
We know that Aishwarya studies either computer science or mathematics every day. So, we can consider these two events as the sample space.
Let's define the events:
- C: Aishwarya studies computer science
- M: Aishwarya studies mathematics
We are given that Aishwarya studies computer science on Monday (event B), so we are interested in finding the probability of Aishwarya studying computer science on Wednesday (event A).
We need to find P(C|C), which is the probability of Aishwarya studying computer science on Wednesday, given that she studied computer science on Monday.
We can use the formula for conditional probability:
P(C|C) = P(C and C) / P(C)
Finding P(C and C):
Since Aishwarya studies either computer science or mathematics every day, the events C and M are mutually exclusive. So, P(C and C) is equal to P(C).
Finding P(C):
We are given that Aishwarya studies computer science on Monday. So, P(C) = 1.
Calculating the Probability:
Using the formula for conditional probability, we have:
P(C|C) = P(C and C) / P(C)
P(C|C) = P(C) / P(C)
P(C|C) = 1 / 1
P(C|C) = 1
Therefore, the probability that Aishwarya studies computer science on Wednesday, given that she studies computer science on Monday, is 1 or 100%.
Answer:
The probability that Aishwarya studies computer science on Wednesday is 0.4 (or 40%). Therefore, the correct answer is option C) 0.4.
If X is a Poisson variate with P(X = 0) = 0.6, then the variance of X is:- a)In (1/5)
- b)log1015
- c)0
- d)ln 15
Correct answer is option 'A'. Can you explain this answer?
If X is a Poisson variate with P(X = 0) = 0.6, then the variance of X is:
a)
In (1/5)
b)
log1015
c)
0
d)
ln 15
|
Bayshore Academy answered |
Given
In Poisson distribution
P(X = 0) = 0.6
Formula
Poisson distribution is given by
f(x) = e-λλx/x!
Calculation
P(X = 0) = e-λλ0/0!
⇒ 0.6 = e-λ
⇒ 1/eλ = 6/10 = 3/5
⇒ eλ = 5/3
Taking log on both side
⇒ logeeλ = loge(5/3)
∴ λ = Loge(5/3)
The mean of 25 observations is 36 . If the mean of the first 13 observations is 32 and that of the last 13 observations is 39 , the 13th observation is: - a)22
- b)25
- c)26
- d)23
Correct answer is option 'D'. Can you explain this answer?
The mean of 25 observations is 36 . If the mean of the first 13 observations is 32 and that of the last 13 observations is 39 , the 13th observation is:
a)
22
b)
25
c)
26
d)
23
|
|
Sanya Agarwal answered |
Given:
The mean of 25 observations is 36
The mean of the first 13 observations is 32 and that of the last 13 observations is 39
The mean of 25 observations is 36
The mean of the first 13 observations is 32 and that of the last 13 observations is 39
Concept used:
Mean = sum of all observation/total number of observation
Mean = sum of all observation/total number of observation
Calculation:
The sum of all 25 observation = 25 × 36 = 900
Sum of first 13 observations = 13 × 32 = 416
Sum of last 13 observations = 13 × 39 = 507
∴ 13th term = (416 + 507) - 900 = 923 - 900 = 23
The sum of all 25 observation = 25 × 36 = 900
Sum of first 13 observations = 13 × 32 = 416
Sum of last 13 observations = 13 × 39 = 507
∴ 13th term = (416 + 507) - 900 = 923 - 900 = 23
You have two bags of colored marbles. Bag A contains 3 red marbles and 2 green marbles, while Bag B contains 4 red marbles and 1 green marble. You choose one of the bags at random and then select a marble from that bag. What is the probability that you selected Bag A, given that you picked a red marble?
- a)3/5
- b)2/5
- c)4/7
- d)3/7
Correct answer is option 'D'. Can you explain this answer?
You have two bags of colored marbles. Bag A contains 3 red marbles and 2 green marbles, while Bag B contains 4 red marbles and 1 green marble. You choose one of the bags at random and then select a marble from that bag. What is the probability that you selected Bag A, given that you picked a red marble?
a)
3/5
b)
2/5
c)
4/7
d)
3/7
|
|
Engineers Adda answered |
Let's use conditional probability here. We want to find the probability of selecting Bag A given that a red marble was chosen.
P(A) is the probability of choosing Bag A, and P(R) is the probability of choosing a red marble.
We know that P(A) is 1/2 because we choose a bag at random, and there are two bags.
P(R|A) is the probability of choosing a red marble from Bag A, which is 3/5.
P(R|B) is the probability of choosing a red marble from Bag B, which is 4/5.
Now, we can use Bayes' theorem to find P(A|R), the probability of choosing Bag A given a red marble was picked:
P(A|R) = [P(R|A) * P(A)] / [P(R|A) * P(A) + P(R|B) * P(B)]
P(A|R) = (3/5 * 1/2) / [(3/5 * 1/2) + (4/5 * 1/2)]
P(A|R) = (3/10) / [(3/10) + (4/10)]
P(A|R) = (3/10) / (7/10)
P(A|R) = 3/7
So, the probability of selecting Bag A, given that a red marble was chosen, is 3/7, which corresponds to option D.
An examination paper has 150 multiple-choice questions of one mark each, with each question having four choices. Each incorrect answer fetches – 0.25 mark. Suppose 1000 students choose all their answers randomly with uniform probability. The sum total of the expected marks obtained all these students is - a)0
- b)2550
- c)7525
- d)9375
Correct answer is option 'D'. Can you explain this answer?
An examination paper has 150 multiple-choice questions of one mark each, with each question having four choices. Each incorrect answer fetches – 0.25 mark. Suppose 1000 students choose all their answers randomly with uniform probability. The sum total of the expected marks obtained all these students is
a)
0
b)
2550
c)
7525
d)
9375
|
Nandini Basak answered |
Ans.
Method to Solve :
Probability of choosing the correct option = 1/4
Probability of choosing a wrong option = 3/4
So, expected mark for a question for a student = 1/4�1+3/4�(−0.25)=1/16
Expected mark for a student for 150questions =1/16�150=9.375
So, sum total of the expected marks obtained by all 1000students = 9.375�1000=9375
Probability of choosing a wrong option = 3/4
So, expected mark for a question for a student = 1/4�1+3/4�(−0.25)=1/16
Expected mark for a student for 150questions =1/16�150=9.375
So, sum total of the expected marks obtained by all 1000students = 9.375�1000=9375
Let the probability density function of a random variable x be given asf(x) = ae-2|x|The value of ‘a’ is __________. Correct answer is between '0.99,1.01'. Can you explain this answer?
Let the probability density function of a random variable x be given as
f(x) = ae-2|x|
The value of ‘a’ is __________.
|
|
Engineers Adda answered |
Concept:
The probability density function for any f(x) is defined as:
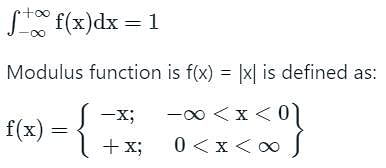
Calculation:
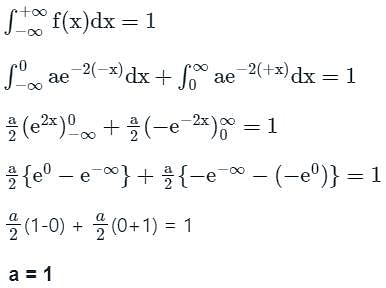
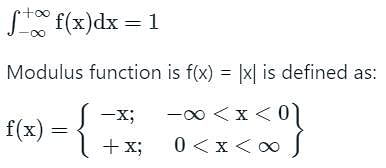
Calculation:
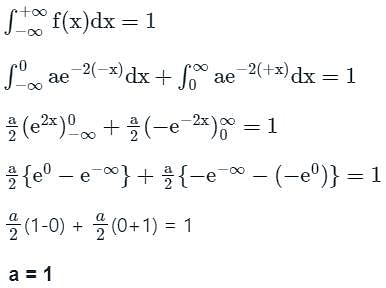
Let x be the mean of squares of first n natural numbers and y be the square of mean of first n natural numbers. If x/y = 55/42 , then what is the value of n ?- a)24
- b)25
- c)27
- d)30
Correct answer is option 'C'. Can you explain this answer?
Let x be the mean of squares of first n natural numbers and y be the square of mean of first n natural numbers. If x/y = 55/42 , then what is the value of n ?
a)
24
b)
25
c)
27
d)
30
|
|
Hiral Sharma answered |
To solve this problem, we need to find the value of 'n' when given the ratio of x/y.
Given:
x/y = 55/42
Let's break down the problem into steps to find the value of 'n':
Step 1: Understanding the problem
We are given two variables, x and y, which represent the mean of squares and the square of the mean of the first 'n' natural numbers, respectively. We need to find the value of 'n' when x/y is equal to 55/42.
Step 2: Understanding the formulas
The mean of squares of the first 'n' natural numbers can be calculated using the formula:
x = (1^2 + 2^2 + 3^2 + ... + n^2) / n
The square of the mean of the first 'n' natural numbers can be calculated using the formula:
y = [(1 + 2 + 3 + ... + n) / n]^2
Step 3: Simplifying the expressions
Let's simplify the expressions for x and y:
x = (1^2 + 2^2 + 3^2 + ... + n^2) / n
= (n(n+1)(2n+1)) / 6n
= (n+1)(2n+1) / 6
y = [(1 + 2 + 3 + ... + n) / n]^2
= [(n(n+1))/2n]^2
= (n+1)^2 / 4
Step 4: Substituting the expressions into the given ratio
Now, we substitute the expressions for x and y into the given ratio:
x/y = 55/42
[(n+1)(2n+1) / 6] / [(n+1)^2 / 4] = 55/42
Step 5: Cross-multiplying and simplifying the equation
To simplify the equation, we cross-multiply and get rid of the fractions:
[(n+1)(2n+1)] * 4 = [(n+1)^2] * 55/42
Simplifying further:
[(n+1)(2n+1)] * 4 * 42 = [(n+1)^2] * 55
Step 6: Expanding and simplifying the equation
Expanding the equation:
8(n^2) + 12n + 4 = 55n^2 + 110n + 55
Simplifying further:
47n^2 + 98n + 51 = 0
Step 7: Solving the quadratic equation
To solve the quadratic equation, we can factorize it or use the quadratic formula. In this case, the equation cannot be easily factorized, so let's use the quadratic formula:
n = [-b ± √(b^2 - 4ac)] / 2a
Plugging in the values:
n = [-98 ± √(98^2 - 4 * 47 * 51)] / (2 * 47)
n = [-98 ± √(9604 - 9596)] / 94
n = [-98 ± √8] / 94
n = [-98 ± 2√2] / 94
Step
Given:
x/y = 55/42
Let's break down the problem into steps to find the value of 'n':
Step 1: Understanding the problem
We are given two variables, x and y, which represent the mean of squares and the square of the mean of the first 'n' natural numbers, respectively. We need to find the value of 'n' when x/y is equal to 55/42.
Step 2: Understanding the formulas
The mean of squares of the first 'n' natural numbers can be calculated using the formula:
x = (1^2 + 2^2 + 3^2 + ... + n^2) / n
The square of the mean of the first 'n' natural numbers can be calculated using the formula:
y = [(1 + 2 + 3 + ... + n) / n]^2
Step 3: Simplifying the expressions
Let's simplify the expressions for x and y:
x = (1^2 + 2^2 + 3^2 + ... + n^2) / n
= (n(n+1)(2n+1)) / 6n
= (n+1)(2n+1) / 6
y = [(1 + 2 + 3 + ... + n) / n]^2
= [(n(n+1))/2n]^2
= (n+1)^2 / 4
Step 4: Substituting the expressions into the given ratio
Now, we substitute the expressions for x and y into the given ratio:
x/y = 55/42
[(n+1)(2n+1) / 6] / [(n+1)^2 / 4] = 55/42
Step 5: Cross-multiplying and simplifying the equation
To simplify the equation, we cross-multiply and get rid of the fractions:
[(n+1)(2n+1)] * 4 = [(n+1)^2] * 55/42
Simplifying further:
[(n+1)(2n+1)] * 4 * 42 = [(n+1)^2] * 55
Step 6: Expanding and simplifying the equation
Expanding the equation:
8(n^2) + 12n + 4 = 55n^2 + 110n + 55
Simplifying further:
47n^2 + 98n + 51 = 0
Step 7: Solving the quadratic equation
To solve the quadratic equation, we can factorize it or use the quadratic formula. In this case, the equation cannot be easily factorized, so let's use the quadratic formula:
n = [-b ± √(b^2 - 4ac)] / 2a
Plugging in the values:
n = [-98 ± √(98^2 - 4 * 47 * 51)] / (2 * 47)
n = [-98 ± √(9604 - 9596)] / 94
n = [-98 ± √8] / 94
n = [-98 ± 2√2] / 94
Step
If the regression line of Y on X is Y = 30 - 0.9X and the standard deviations are Sx = 2 and Sy = 9, then the value of the correlation coefficient rxy is:- a)-0.3
- b)-0.2
- c)0.2
- d)0.3
Correct answer is option 'B'. Can you explain this answer?
If the regression line of Y on X is Y = 30 - 0.9X and the standard deviations are Sx = 2 and Sy = 9, then the value of the correlation coefficient rxy is:
a)
-0.3
b)
-0.2
c)
0.2
d)
0.3
|
|
Srestha Khanna answered |
Calculation of Correlation Coefficient
Given:
- Regression line of Y on X: Y = 30 - 0.9X
- Standard deviation of X (Sx): 2
- Standard deviation of Y (Sy): 9
Formula:
rxy = -√(1 - (b^2)(Sy^2)/(Sx^2)(Sy^2))
Calculation:
- Slope (b) of the regression line = -0.9
- Substitute the values into the formula:
rxy = -√(1 - (-0.9)^2(9^2)/(2^2)(9^2))
rxy = -√(1 - 0.81*81/4*81)
rxy = -√(1 - 0.65625)
rxy = -√0.34375
rxy ≈ -0.586
Result: The value of the correlation coefficient rxy is approximately -0.586, which is closest to option B (-0.2).
Given:
- Regression line of Y on X: Y = 30 - 0.9X
- Standard deviation of X (Sx): 2
- Standard deviation of Y (Sy): 9
Formula:
rxy = -√(1 - (b^2)(Sy^2)/(Sx^2)(Sy^2))
Calculation:
- Slope (b) of the regression line = -0.9
- Substitute the values into the formula:
rxy = -√(1 - (-0.9)^2(9^2)/(2^2)(9^2))
rxy = -√(1 - 0.81*81/4*81)
rxy = -√(1 - 0.65625)
rxy = -√0.34375
rxy ≈ -0.586
Result: The value of the correlation coefficient rxy is approximately -0.586, which is closest to option B (-0.2).
A box contains 5 black and 5 red balls. Two balls are randomly picked one after another from the box, without replacement. The probability for both balls being red is - a)1/90
- b)1/2
- c)19/90
- d)2/9
Correct answer is option 'D'. Can you explain this answer?
A box contains 5 black and 5 red balls. Two balls are randomly picked one after another from the box, without replacement. The probability for both balls being red is
a)
1/90
b)
1/2
c)
19/90
d)
2/9
|
Avik Ghosh answered |
The probability of drawing two red balls without replacement
The probability of a defective piece being produced in a manufacturing process is 0.01. The probability that out of 5 successive pieces, only one is defective, is - a)(0. 99)2 (0.01)
- b)(0.99)( 0.01)4
- c)5× (0.99)(0.01)4
- d)5× (0.99)4(0.01)
Correct answer is option 'D'. Can you explain this answer?
The probability of a defective piece being produced in a manufacturing process is 0.01. The probability that out of 5 successive pieces, only one is defective, is
a)
(0. 99)2 (0.01)
b)
(0.99)( 0.01)4
c)
5× (0.99)(0.01)4
d)
5× (0.99)4(0.01)
|
|
Keerthana Joshi answered |
The required probability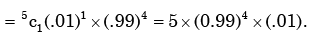 
The probability that there are 53 Sundays in a randomly chosen leap year is - a)1/7
- b)1/14
- c)1/28
- d)2/7
Correct answer is option 'D'. Can you explain this answer?
The probability that there are 53 Sundays in a randomly chosen leap year is
a)
1/7
b)
1/14
c)
1/28
d)
2/7
|
|
Gauri Roy answered |
Problem:
The probability that there are 53 Sundays in a randomly chosen leap year is:
a) 1/7
b) 1/14
c) 1/28
d) 2/7
The correct answer is option 'D'. Let's explain why it is the correct answer.
Leap Year:
A leap year is a year that is exactly divisible by 4, except for years that are divisible by 100. However, years that are divisible by 400 are also leap years. This means that every 4 years, there is an extra day, February 29th, in the calendar.
Number of Days in a Leap Year:
In a leap year, there are 366 days instead of the usual 365 days. This is because of the extra day, February 29th.
Number of Weeks in a Leap Year:
Since there are 7 days in a week, the total number of weeks in a leap year is given by:
Total number of weeks = Number of days / Number of days in a week
= 366 days / 7 days per week
= 52 weeks and 2 days
Number of Sundays in a Leap Year:
To find the probability of having 53 Sundays in a leap year, we need to determine the number of Sundays that can occur in a leap year.
In a leap year, the first day of the year can be any day of the week. Let's consider each possibility:
1) If the first day of the year is a Sunday, then there will be 53 Sundays in the year.
2) If the first day of the year is a Monday, then there will be 53 Sundays in the year.
3) If the first day of the year is a Tuesday, then there will be 52 Sundays in the year.
4) If the first day of the year is a Wednesday, then there will be 52 Sundays in the year.
5) If the first day of the year is a Thursday, then there will be 52 Sundays in the year.
6) If the first day of the year is a Friday, then there will be 52 Sundays in the year.
7) If the first day of the year is a Saturday, then there will be 52 Sundays in the year.
In options a), b), and c), the probability given is less than 1/7, which means it is incorrect. The only option left is option d), which is 2/7. This is the correct answer.
Conclusion:
The probability that there are 53 Sundays in a randomly chosen leap year is 2/7, which is the correct answer (option 'D').
The probability that there are 53 Sundays in a randomly chosen leap year is:
a) 1/7
b) 1/14
c) 1/28
d) 2/7
The correct answer is option 'D'. Let's explain why it is the correct answer.
Leap Year:
A leap year is a year that is exactly divisible by 4, except for years that are divisible by 100. However, years that are divisible by 400 are also leap years. This means that every 4 years, there is an extra day, February 29th, in the calendar.
Number of Days in a Leap Year:
In a leap year, there are 366 days instead of the usual 365 days. This is because of the extra day, February 29th.
Number of Weeks in a Leap Year:
Since there are 7 days in a week, the total number of weeks in a leap year is given by:
Total number of weeks = Number of days / Number of days in a week
= 366 days / 7 days per week
= 52 weeks and 2 days
Number of Sundays in a Leap Year:
To find the probability of having 53 Sundays in a leap year, we need to determine the number of Sundays that can occur in a leap year.
In a leap year, the first day of the year can be any day of the week. Let's consider each possibility:
1) If the first day of the year is a Sunday, then there will be 53 Sundays in the year.
2) If the first day of the year is a Monday, then there will be 53 Sundays in the year.
3) If the first day of the year is a Tuesday, then there will be 52 Sundays in the year.
4) If the first day of the year is a Wednesday, then there will be 52 Sundays in the year.
5) If the first day of the year is a Thursday, then there will be 52 Sundays in the year.
6) If the first day of the year is a Friday, then there will be 52 Sundays in the year.
7) If the first day of the year is a Saturday, then there will be 52 Sundays in the year.
In options a), b), and c), the probability given is less than 1/7, which means it is incorrect. The only option left is option d), which is 2/7. This is the correct answer.
Conclusion:
The probability that there are 53 Sundays in a randomly chosen leap year is 2/7, which is the correct answer (option 'D').
The probability that an event A occurs in one trial of an experiment is 0.4. Three independent trials of experiment are performed. The probability that A occurs at least once is- a)0.936
- b)0.784
- c)0.964
- d)None
Correct answer is option 'B'. Can you explain this answer?
The probability that an event A occurs in one trial of an experiment is 0.4. Three independent trials of experiment are performed. The probability that A occurs at least once is
a)
0.936
b)
0.784
c)
0.964
d)
None
|
Ishita Patel answered |
Here p = 0.4, q = 0.6 and n = 3.
A man draws 3 balls from a jug containing 5 white balls and 7 black balls. He gets Rs. 20 for each white ball and Rs. 10 for each black ball. What is his expectation?- a)Rs. 21.25
- b)Rs. 42.50
- c)Rs. 31.25
- d)Rs. 45.21
Correct answer is option 'B'. Can you explain this answer?
A man draws 3 balls from a jug containing 5 white balls and 7 black balls. He gets Rs. 20 for each white ball and Rs. 10 for each black ball. What is his expectation?
a)
Rs. 21.25
b)
Rs. 42.50
c)
Rs. 31.25
d)
Rs. 45.21
|
|
Bayshore Academy answered |
3 balls can be drawn in the following ways
Case (i) : Probability of drawing 3 white balls out of 12 balls
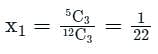
Money he gets for drawing 3 white balls
P1 = 3 × 20 = Rs. 60
Case (ii) : Probability of drawing 2 white balls and 1 black ball out of 12 balls
Money he gets for drawing 2 white balls and 1 black ball
P2 = (20 × 2) + (10 × 1) = Rs. 50
Case (iii) : Probability of drawing 1 white ball and 2 black balls out of 12 balls
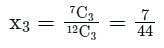
Money he gets for drawing 3 black balls
P3 = 10 × 3 = Rs. 30
Expectation = Sum of the product of probability and the money he gets for each combination.
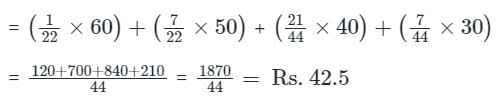
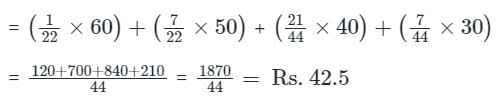
If P(A) = 5/13, P(B) = 7/13 and P(A∩B) = 3/13, evaluate P(A|B).- a)1/7
- b)3/7
- c)3/5
- d)2/7
Correct answer is option 'B'. Can you explain this answer?
If P(A) = 5/13, P(B) = 7/13 and P(A∩B) = 3/13, evaluate P(A|B).
a)
1/7
b)
3/7
c)
3/5
d)
2/7
|
Vertex Academy answered |
We know that P(A|B) = P(A∩B) / P(B). (By formula for conditional probability)
Which is equivalent to (3/13) / (7/13), hence the value of P(A|B) = 3/7.
Which is equivalent to (3/13) / (7/13), hence the value of P(A|B) = 3/7.
Let X and Y be two exponentially distributed and independent random variables with mean α and β, respectively. If Z= min (X, Y), then the mean of Z is given by- a)(1/(α + β))
- b)min (α, β)
- c)(αβ/(α + β))
- d)α + β
Correct answer is option 'C'. Can you explain this answer?
Let X and Y be two exponentially distributed and independent random variables with mean α and β, respectively. If Z= min (X, Y), then the mean of Z is given by
a)
(1/(α + β))
b)
min (α, β)
c)
(αβ/(α + β))
d)
α + β
|
|
Ashwini Ghosh answered |
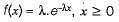
X and Y are two independent exponentially distributed random variables. Let λ1 and λ2 parameters of X and Y respectively.
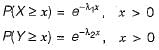
Given, Z = min (X, Y)
Since mean of exponential distribution = 1/Parameter
So,
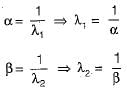
∴ Z is random variable with parameter
Mean of Z =
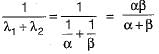
Three values of x and y are to be fitted in a straight line in the form y = a + bx by the method of least squares. GivenΣx = 6, Σy = 21, Σx2 = 14 and Σxy = 46, the values of a and b are respectively. - a)2 and 3
- b)1 and 2
- c)2 and 1
- d)3 and 2
Correct answer is option 'D'. Can you explain this answer?
Three values of x and y are to be fitted in a straight line in the form y = a + bx by the method of least squares. GivenΣx = 6, Σy = 21, Σx2 = 14 and Σxy = 46, the values of a and b are respectively.
a)
2 and 3
b)
1 and 2
c)
2 and 1
d)
3 and 2
|
Ritika Menon answered |
Chapter doubts & questions for Probability and Statistics - Engineering Mathematics 2025 is part of Civil Engineering (CE) exam preparation. The chapters have been prepared according to the Civil Engineering (CE) exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Probability and Statistics - Engineering Mathematics in English & Hindi are available as part of Civil Engineering (CE) exam.
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Engineering Mathematics
65 videos|122 docs|94 tests
|
Signup to see your scores go up within 7 days!
Study with 1000+ FREE Docs, Videos & Tests
10M+ students study on EduRev
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup