Railways Exam > Railways Questions > A beam of length L carries a uniformly distri...
Start Learning for Free
A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?
- a)Cantilever beam
- b)Simply supported beam
- c)Propped cantilever
- d)Fixed beam
Correct answer is option 'A'. Can you explain this answer?
Verified Answer
A beam of length L carries a uniformly distributed load throughout its...
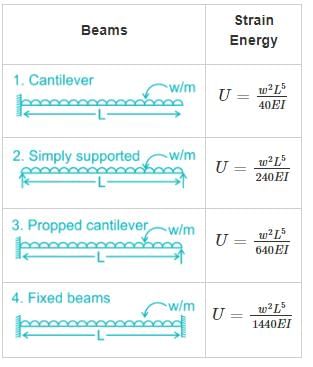
Hence, maximum strain energy is for Cantilever beam.
Most Upvoted Answer
A beam of length L carries a uniformly distributed load throughout its...
Explanation:
Strain energy is defined as the energy stored within an elastic material due to deformation. The strain energy stored in a beam is given by the equation U = (1/2)∫Mθ dx, where M is the bending moment and θ is the angle of rotation at a point on the beam. The integral is taken over the entire length of the beam.
The strain energy in a beam depends on various factors such as the type of loading, the support conditions, the material properties, and the geometry of the beam. In this case, we are given that the beam carries a uniformly distributed load throughout its length. Let us examine the four different support conditions and determine which one will result in the maximum strain energy.
A) Cantilever Beam:
- A cantilever beam is a beam that is fixed at one end and free at the other end.
- The maximum bending moment occurs at the fixed end of the beam, where the load is applied.
- The angle of rotation at the fixed end is also maximum.
- Therefore, the strain energy in a cantilever beam will be maximum.
B) Simply Supported Beam:
- A simply supported beam is a beam that is supported at two ends and free to rotate at both ends.
- The bending moment is maximum at the center of the beam.
- The angle of rotation is also maximum at the center of the beam.
- Therefore, the strain energy in a simply supported beam will not be maximum.
C) Propped Cantilever Beam:
- A propped cantilever beam is a beam that is fixed at one end and supported by a prop at some distance from the fixed end.
- The bending moment and the angle of rotation will be maximum at the fixed end of the beam, where the load is applied.
- Therefore, the strain energy in a propped cantilever beam will not be maximum.
D) Fixed Beam:
- A fixed beam is a beam that is fixed at both ends and cannot rotate.
- The bending moment is maximum at the center of the beam.
- The angle of rotation is zero at both ends of the beam.
- Therefore, the strain energy in a fixed beam will not be maximum.
Conclusion:
Based on the above analysis, we can conclude that the strain energy will be maximum in a cantilever beam.
Strain energy is defined as the energy stored within an elastic material due to deformation. The strain energy stored in a beam is given by the equation U = (1/2)∫Mθ dx, where M is the bending moment and θ is the angle of rotation at a point on the beam. The integral is taken over the entire length of the beam.
The strain energy in a beam depends on various factors such as the type of loading, the support conditions, the material properties, and the geometry of the beam. In this case, we are given that the beam carries a uniformly distributed load throughout its length. Let us examine the four different support conditions and determine which one will result in the maximum strain energy.
A) Cantilever Beam:
- A cantilever beam is a beam that is fixed at one end and free at the other end.
- The maximum bending moment occurs at the fixed end of the beam, where the load is applied.
- The angle of rotation at the fixed end is also maximum.
- Therefore, the strain energy in a cantilever beam will be maximum.
B) Simply Supported Beam:
- A simply supported beam is a beam that is supported at two ends and free to rotate at both ends.
- The bending moment is maximum at the center of the beam.
- The angle of rotation is also maximum at the center of the beam.
- Therefore, the strain energy in a simply supported beam will not be maximum.
C) Propped Cantilever Beam:
- A propped cantilever beam is a beam that is fixed at one end and supported by a prop at some distance from the fixed end.
- The bending moment and the angle of rotation will be maximum at the fixed end of the beam, where the load is applied.
- Therefore, the strain energy in a propped cantilever beam will not be maximum.
D) Fixed Beam:
- A fixed beam is a beam that is fixed at both ends and cannot rotate.
- The bending moment is maximum at the center of the beam.
- The angle of rotation is zero at both ends of the beam.
- Therefore, the strain energy in a fixed beam will not be maximum.
Conclusion:
Based on the above analysis, we can conclude that the strain energy will be maximum in a cantilever beam.

|
Explore Courses for Railways exam
|

|
Similar Railways Doubts
A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer?
Question Description
A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer? for Railways 2025 is part of Railways preparation. The Question and answers have been prepared according to the Railways exam syllabus. Information about A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for Railways 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer?.
A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer? for Railways 2025 is part of Railways preparation. The Question and answers have been prepared according to the Railways exam syllabus. Information about A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for Railways 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer?.
Solutions for A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for Railways.
Download more important topics, notes, lectures and mock test series for Railways Exam by signing up for free.
Here you can find the meaning of A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A beam of length L carries a uniformly distributed load throughout its length. In which of the following condition will the strain energy be maximum?a)Cantilever beamb)Simply supported beamc)Propped cantileverd)Fixed beamCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice Railways tests.

|
Explore Courses for Railways exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























