JEE Exam > JEE Questions > The half-life period of a radioactive element...
Start Learning for Free
The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Then
- a)X and Y have the same decay rate initially
- b)X and Y decay at the same rate always
- c)Y will decay at a faster rate than X
- d)X will decay at a faster rate than Y
Correct answer is option 'C'. Can you explain this answer?
Verified Answer
The half-life period of a radioactive element X is same as the mean-li...
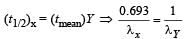
∴ λx = 0.693 λY
λx < λY. Now, rate of decay = λN
Initially, number of atoms (N) of both are equal but since λY < λx, therefore Y will decay at a faster rate than x.
λx < λY. Now, rate of decay = λN
Initially, number of atoms (N) of both are equal but since λY < λx, therefore Y will decay at a faster rate than x.
Most Upvoted Answer
The half-life period of a radioactive element X is same as the mean-li...
The half-life period of a radioactive element is the time it takes for half of the radioactive atoms in a sample to decay. The mean-life time of a radioactive element is the average time it takes for an atom to decay. In this scenario, element X has a half-life period equal to the mean-life time of element Y.
a) X and Y have the same decay rate initially:
- This statement is not correct. The decay rate of a radioactive element is determined by the number of atoms decaying per unit time. The half-life period and mean-life time do not provide information about the initial decay rate.
b) X and Y decay at the same rate always:
- This statement is not correct either. The decay rate of a radioactive element depends on the number of remaining radioactive atoms. As time passes, the number of atoms of both elements X and Y will decrease, but they will not necessarily decay at the same rate.
c) Y will decay at a faster rate than X:
- This statement is correct. Since the mean-life time of element Y is equal to the half-life period of element X, it means that on average, element Y decays faster than element X. The mean-life time represents the average time it takes for an atom to decay, so it can be inferred that Y has a faster decay rate.
d) X will decay at a faster rate than Y:
- This statement is incorrect. As mentioned above, the mean-life time of element Y is equal to the half-life period of element X, indicating that Y decays faster than X. Therefore, X will not decay at a faster rate than Y.
In summary, the correct answer is option 'C' - Y will decay at a faster rate than X because the mean-life time of Y is equal to the half-life period of X.
a) X and Y have the same decay rate initially:
- This statement is not correct. The decay rate of a radioactive element is determined by the number of atoms decaying per unit time. The half-life period and mean-life time do not provide information about the initial decay rate.
b) X and Y decay at the same rate always:
- This statement is not correct either. The decay rate of a radioactive element depends on the number of remaining radioactive atoms. As time passes, the number of atoms of both elements X and Y will decrease, but they will not necessarily decay at the same rate.
c) Y will decay at a faster rate than X:
- This statement is correct. Since the mean-life time of element Y is equal to the half-life period of element X, it means that on average, element Y decays faster than element X. The mean-life time represents the average time it takes for an atom to decay, so it can be inferred that Y has a faster decay rate.
d) X will decay at a faster rate than Y:
- This statement is incorrect. As mentioned above, the mean-life time of element Y is equal to the half-life period of element X, indicating that Y decays faster than X. Therefore, X will not decay at a faster rate than Y.
In summary, the correct answer is option 'C' - Y will decay at a faster rate than X because the mean-life time of Y is equal to the half-life period of X.

|
Explore Courses for JEE exam
|

|
The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer?
Question Description
The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer?.
The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer?.
Solutions for The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The half-life period of a radioactive element X is same as the mean-life time of another radioactive element Y. Initially both of them have the same number of atoms. Thena)X and Y have the same decay rate initiallyb)X and Y decay at the same rate alwaysc)Y will decay at a faster rate than Xd)X will decay at a faster rate than YCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice JEE tests.

|
Explore Courses for JEE exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























