Physics Exam > Physics Questions > A photon strikes an electron of massmthat is ...
Start Learning for Free
A photon strikes an electron of mass m that is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units of mc2).
Correct answer is '4'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A photon strikes an electron of massmthat is initially at rest, creati...
Let us work in the system of units in which c = 1
Now since E2 – p2 is an invariant quantity, we equate this in the initial laboratory frame and in the final frame of particles
In lab frame, the electron is at rest.
∴ Eelectron = m(c = 1) (Take m to be the mass of electron)
∴ Eelectron = m(c = 1) (Take m to be the mass of electron)
∴ Total Energy of the system is E + m = Energy of photon + Energy of electron
E, p are the total energy and momentum of the photon.
E, p are the total energy and momentum of the photon.
∴ 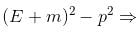 Laboratory frame ...(1)
Laboratory frame ...(1)
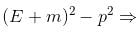 Laboratory frame ...(1)
Laboratory frame ...(1)In the final state of particles
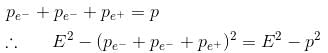
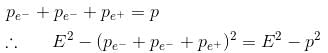
E2 – p2 = (m + m + m)2
= (3m)2
= 9m2 ...(2)
= (3m)2
= 9m2 ...(2)
Equating for the 2 frames (1) = (2)
∴ (E + m)2 – p2 = 9m2
E2 + m2 + 2Em – p2 = 9m2
since a photon is massless
∴ (E + m)2 – p2 = 9m2
E2 + m2 + 2Em – p2 = 9m2
since a photon is massless
∴ E = p
∴ m2 + 2Em = 9m2
2Em = 8m2
E = 4m
Now in SI units
E = 4mc2
∴ m2 + 2Em = 9m2
2Em = 8m2
E = 4m
Now in SI units
E = 4mc2
The correct answer is: 4
Most Upvoted Answer
A photon strikes an electron of massmthat is initially at rest, creati...
Given:
- Mass of electron, m
- Electron-positron pair is created when a photon strikes an electron
- The photon is destroyed
- The positron and 2 electrons move off at equal speeds along the initial direction of the photon
To find:
Energy of the photon (in units of mc^2)
Explanation:
When a photon strikes an electron at rest, it can undergo pair production, where an electron-positron pair is created. This process conserves both energy and momentum.
Conservation of Energy:
- Initially, the electron is at rest, so its energy is zero.
- The photon has an energy, E, which is given by E = hf, where h is the Planck's constant and f is the frequency of the photon.
- After the pair production, the positron, electron, and their kinetic energies add up to the initial energy of the photon.
- The positron and electrons move off at equal speeds, so they have equal kinetic energies.
Conservation of Momentum:
- Initially, the electron is at rest, so the total momentum is zero.
- After the pair production, the positron and electrons move off in the same direction with equal speeds, so their momenta add up to zero.
Using Conservation of Energy:
- The energy of the photon, E, is equal to the sum of the energies of the positron and electrons.
- Since the positron and electrons have equal kinetic energies, the energy of the photon is 2 times their kinetic energy.
- Let the kinetic energy of each particle be K. Therefore, the energy of the photon is 2K.
Using Conservation of Momentum:
- The momentum of the positron and electrons add up to zero.
- Since they have equal speeds, their momenta cancel each other out.
- The momentum of the photon is zero initially.
Conclusion:
- The energy of the photon is 2 times the kinetic energy of each particle.
- The momentum of the photon is zero.
- Therefore, the energy of the photon is given by E = 2K.
Answer:
The energy of the photon is 2 times the kinetic energy of each particle. Therefore, the energy of the photon is 4 times the rest mass energy of the electron (4mc^2).
- Mass of electron, m
- Electron-positron pair is created when a photon strikes an electron
- The photon is destroyed
- The positron and 2 electrons move off at equal speeds along the initial direction of the photon
To find:
Energy of the photon (in units of mc^2)
Explanation:
When a photon strikes an electron at rest, it can undergo pair production, where an electron-positron pair is created. This process conserves both energy and momentum.
Conservation of Energy:
- Initially, the electron is at rest, so its energy is zero.
- The photon has an energy, E, which is given by E = hf, where h is the Planck's constant and f is the frequency of the photon.
- After the pair production, the positron, electron, and their kinetic energies add up to the initial energy of the photon.
- The positron and electrons move off at equal speeds, so they have equal kinetic energies.
Conservation of Momentum:
- Initially, the electron is at rest, so the total momentum is zero.
- After the pair production, the positron and electrons move off in the same direction with equal speeds, so their momenta add up to zero.
Using Conservation of Energy:
- The energy of the photon, E, is equal to the sum of the energies of the positron and electrons.
- Since the positron and electrons have equal kinetic energies, the energy of the photon is 2 times their kinetic energy.
- Let the kinetic energy of each particle be K. Therefore, the energy of the photon is 2K.
Using Conservation of Momentum:
- The momentum of the positron and electrons add up to zero.
- Since they have equal speeds, their momenta cancel each other out.
- The momentum of the photon is zero initially.
Conclusion:
- The energy of the photon is 2 times the kinetic energy of each particle.
- The momentum of the photon is zero.
- Therefore, the energy of the photon is given by E = 2K.
Answer:
The energy of the photon is 2 times the kinetic energy of each particle. Therefore, the energy of the photon is 4 times the rest mass energy of the electron (4mc^2).

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer?
Question Description
A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer?.
A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer?.
Solutions for A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer?, a detailed solution for A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer? has been provided alongside types of A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A photon strikes an electron of massmthat is initially at rest, creating an electron positron pair. The photon is destroyed and the positron and 2 electrons move off at equal speeds along the initial direction of the photon. Find energy of the photon (in units ofmc2).Correct answer is '4'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















