Physics Exam > Physics Questions > When an ideal quantum gas can be treated as a...
Start Learning for Free
When an ideal quantum gas can be treated as an ideal classical gas?
Select one:
Select one:
- a)When de-Broglie wavelength be of the order of inter-particle distance
- b)When de-Broglie wavelength is much smaller than the inter-particle distance
- c)When de-Broglie wavelength is much larger than the inter particle distance
- d)A quantum gas can never be treated as classical gas
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
When an ideal quantum gas can be treated as an ideal classical gas?Sel...
Let us take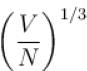 
where V is volume and N is number of particles.
Now, for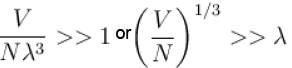
i.e. when interparticle is much larger than the thermal de-Broglie wavelength, the gas will obey Maxwell Boltzmann Statistics.
The correct answer is: When de-Broglie wavelength is much smaller than the inter-particle distance
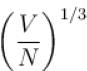where V is volume and N is number of particles.Now, for
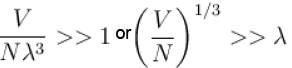
i.e. when interparticle is much larger than the thermal de-Broglie wavelength, the gas will obey Maxwell Boltzmann Statistics.
The correct answer is: When de-Broglie wavelength is much smaller than the inter-particle distance
Most Upvoted Answer
When an ideal quantum gas can be treated as an ideal classical gas?Sel...
Explanation:
In quantum mechanics, particles are described by wave functions, and their behavior is governed by wave-particle duality. The de Broglie wavelength (λ) is a fundamental concept that relates the momentum of a particle to its wavelength, given by the equation λ = h/p, where h is Planck's constant and p is the momentum of the particle.
Classical Gas:
In classical physics, particles are treated as point-like objects without any wave nature. The behavior of a classical gas is described by classical statistical mechanics, where particles are assumed to follow classical laws of motion and obey the ideal gas equation. The classical gas model is applicable when the particles are non-interacting and their individual wavelengths are negligible compared to the inter-particle distance.
Quantum Gas:
In contrast, a quantum gas consists of particles that exhibit wave-like behavior and are subject to quantum mechanical principles. The quantum gas model is necessary to describe the behavior of particles at low temperatures or high densities, where quantum effects become significant.
Conditions for Treating a Quantum Gas as a Classical Gas:
The question asks when an ideal quantum gas can be treated as an ideal classical gas. This means that the quantum effects are negligible, and the particles can be treated as classical point-like objects. The correct answer is option B - when the de Broglie wavelength is much smaller than the inter-particle distance.
Explanation of Option B:
When the de Broglie wavelength of particles in a quantum gas is much smaller than the inter-particle distance, the wave nature of the particles becomes negligible. This is because the particles are tightly packed together, and their individual wavelengths do not significantly affect their behavior. As a result, the particles can be treated as classical point-like objects, and their behavior can be described using classical statistical mechanics.
When the de Broglie wavelength is small compared to the inter-particle distance, the wave nature of the particles is effectively "squeezed" or suppressed. The particles behave more like classical particles, following classical laws of motion and exhibiting classical statistical behavior. This allows for the use of classical gas models to accurately describe the system.
In summary, when the de Broglie wavelength of particles in a quantum gas is much smaller than the inter-particle distance, the quantum effects become negligible, and the gas can be treated as a classical gas.
In quantum mechanics, particles are described by wave functions, and their behavior is governed by wave-particle duality. The de Broglie wavelength (λ) is a fundamental concept that relates the momentum of a particle to its wavelength, given by the equation λ = h/p, where h is Planck's constant and p is the momentum of the particle.
Classical Gas:
In classical physics, particles are treated as point-like objects without any wave nature. The behavior of a classical gas is described by classical statistical mechanics, where particles are assumed to follow classical laws of motion and obey the ideal gas equation. The classical gas model is applicable when the particles are non-interacting and their individual wavelengths are negligible compared to the inter-particle distance.
Quantum Gas:
In contrast, a quantum gas consists of particles that exhibit wave-like behavior and are subject to quantum mechanical principles. The quantum gas model is necessary to describe the behavior of particles at low temperatures or high densities, where quantum effects become significant.
Conditions for Treating a Quantum Gas as a Classical Gas:
The question asks when an ideal quantum gas can be treated as an ideal classical gas. This means that the quantum effects are negligible, and the particles can be treated as classical point-like objects. The correct answer is option B - when the de Broglie wavelength is much smaller than the inter-particle distance.
Explanation of Option B:
When the de Broglie wavelength of particles in a quantum gas is much smaller than the inter-particle distance, the wave nature of the particles becomes negligible. This is because the particles are tightly packed together, and their individual wavelengths do not significantly affect their behavior. As a result, the particles can be treated as classical point-like objects, and their behavior can be described using classical statistical mechanics.
When the de Broglie wavelength is small compared to the inter-particle distance, the wave nature of the particles is effectively "squeezed" or suppressed. The particles behave more like classical particles, following classical laws of motion and exhibiting classical statistical behavior. This allows for the use of classical gas models to accurately describe the system.
In summary, when the de Broglie wavelength of particles in a quantum gas is much smaller than the inter-particle distance, the quantum effects become negligible, and the gas can be treated as a classical gas.
|
Explore Courses for Physics exam
|
|
Similar Physics Doubts
When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer?
Question Description
When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer?.
When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer?.
Solutions for When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice When an ideal quantum gas can be treated as an ideal classical gas?Select one:a)When de-Broglie wavelength be of the order of inter-particle distanceb)When de-Broglie wavelength is much smaller than the inter-particle distancec)When de-Broglie wavelength is much larger than the inter particle distanced)A quantum gas can never be treated as classical gasCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Physics tests.
|
|
Explore Courses for Physics exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup