Physics Exam > Physics Questions > In a classical microcanonical ensemble for a ...
Start Learning for Free
In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.
Select one:
Select one:
- a)h3N
- b)hN
- c)h
- d)h2N
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
In a classical microcanonical ensemble for a system of N interacting p...
For the particle, volume of phase space corresponding to one microstate is
dV = (dxdydz)(dpxdpydpz)
= h3
For N interacting particles, the volume of one microstate is
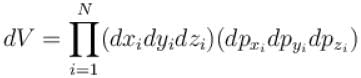
= (h3)·h3 ···N terms
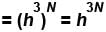
The correct answer is: h3N
dV = (dxdydz)(dpxdpydpz)
= h3
For N interacting particles, the volume of one microstate is
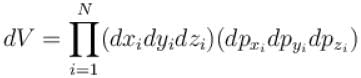
= (h3)·h3 ···N terms
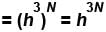
The correct answer is: h3N
Most Upvoted Answer
In a classical microcanonical ensemble for a system of N interacting p...
Fundamental volume in phase space
In the microcanonical ensemble, the system is considered to be isolated and is characterized by a fixed total energy, volume, and number of particles. Each possible state of the system is represented by a point in a high-dimensional space called phase space. The volume occupied by all possible states of the system is known as the fundamental volume in phase space.
Equivalence of microstates
The concept of microstates refers to the different ways in which the system can distribute its energy among its particles while satisfying the given constraints. In the classical microcanonical ensemble, all microstates with the same total energy, volume, and number of particles are considered equally probable.
Derivation of the answer
To find the fundamental volume in phase space equivalent to one microstate, we need to consider the number of possible momentum states and position states for each particle in the system.
- Each particle in the system can have its momentum specified by three independent variables (px, py, pz) due to the three-dimensional nature of space.
- Each particle can also have its position specified by three independent variables (x, y, z) due to the three-dimensional nature of space.
Therefore, the phase space volume for each particle is given by h^3, where h is the Planck's constant divided by 2π.
Since we have N particles in the system, the total phase space volume for all particles is given by (h^3)^N = h^(3N).
Conclusion
In the classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space equivalent to one microstate is h^3N. This means that each microstate of the system occupies a volume of h^3N in phase space.
|
Explore Courses for Physics exam
|
|
Similar Physics Doubts
In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer?
Question Description
In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer?.
In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer?.
Solutions for In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice In a classical microcanonical ensemble for a system of N interacting particles, the fundamental volume in phase space which is regarded as equivalent to one microstate is.Select one:a)h3Nb)hNc)hd)h2NCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice Physics tests.
|
|
Explore Courses for Physics exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup