All Courses
Explore Courses UPSC Exam UPSC Test Series Free Notes EduRev Infinity Get the App Login Join for Free
Sign in Open App
GMAT Exam  >  GMAT Questions  >  In a certain group of women, 70 percent of th... Start Learning for Free
SaveJoin the Discussion on the App
In a certain group of women, 70 percent of the women were employed and 25 percent did not have a graduate degree. Which of the following statements cannot be true?
  1. No woman in the group who had a graduate degree was unemployed
  2. Less than half of the women in the group were employed and had a graduate degree
  3. The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degree
  • a)
    I only
  • b)
    II only
  • c)
    III only
  • d)
    I and III only
  • e)
    II and III only
Correct answer is option 'A'. Can you explain this answer?
FREE This question is part of
Download PDF Attempt Test
Download PDF Attempt this Test
Verified Answer
In a certain group of women, 70 percent of the women were employed and...
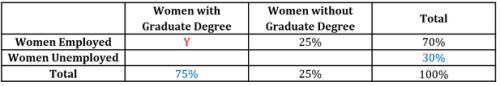
Given:
  • The Women in the group are divided into groups based on 2 parameters:
    • Employment (Employed, Unemployed)
    • Education (Have a Graduate Degree, Don’t have Graduate Degree)
  • So, the given information can be represented in a table as follows:
  • Now, % of women Unemployed = 100% - 70% = 30%
  • And, % of Women with Graduate Degree = 100% - 25% = 75%
To find: Which of the 3 statements cannot be true?
  • That is, which of the 3 statements are definitely false
Approach:
  1. We’ll evaluate the 3 statements one by one to evaluate which of them are definitely false.
Working Out:
  • Evaluating Statement I
  • No woman in the group who had a graduate degree was unemployed
    • Let the Women with Graduate Degree who are Unemployed be X, and let the Women with Graduate Degree who are Employed be Y.
    • As per this Statement, X = 0%
  • Now, the maximum possible value of Y is 70% (happens when ALL employed women have graduate degrees)
  • So, the minimum possible value of X is 5% (since the sum of Y and X is 75%)
  • Therefore, Statement I is definitely false.
  • Evaluating Statement II
  • Less than half of the women in the group were employed and had a graduate degree
    • Again, Let the women who were employed and had Graduate Degree be Y
    • As per this Statement, Y < 50%
Remember that the question is asking if a Statement is definitely false (that is, false for all possible values of Y)
  • In our analysis of Statement I above, we saw that the maximum possible value of Y is 70%
    • Therefore, for the maximum value of Y, Statement II is false
    • But we cannot say at this point if Statement II is definitely false (that is, false for all possible values of Y)
  • So, let’s now evaluate is also false for the minimum value of Y.
    • The maximum number of Employed Women without Graduate Degree is 25% (happens when ALL women without Graduate Degree are Employed)
​
  • So, the minimum value of Y is 70% - 25% = 45%
  • We observe that for the minimum value of Y, Statement II is true.
  • Thus, Statement II is true for some values of Y and false for other values of Y.
     
  • So, Statement II is not definitely false.
 
  • Evaluating Statement III
  • The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degree
    • Let the Women with Graduate Degree who are Unemployed be X, and let the Women without Graduate Degree who are Employed be Z.
    • As per Statement III, X = 1.5Z
  • Note that this Statement will be definitely false if no valid pair of (X,Z) satisfies the equation X = 1.5Z
  • We will first find the values of X and Z that will satisfy this equation as well as the table above. Then, we will check if these values are acceptable.
  • So, the equation that we can write to relate X and Z is:
    • X = 75% - (70% - Z)
      • = 5% + Z
    • Let’s now substitute X = 1.5Z in this equation:
      • 1.5Z = 5% + Z
      • So, 0.5Z = 5%
      • Therefore, Z = 10%
      • Corresponding value of X = 1.5*10% = 15%
         
  • So, X = 1.5Z is satisfied by X = 15% and Z = 10%
  • We now need to check if these values of X and Z are acceptable
    • The minimum possible value of Z is 0%.
    • In this case, the table will look as under:
The maximum possible value of Z is 25%. In this case, the table will look as under:
  • So, the values of Z range from 0% to 25% and correspondingly, the values of X range from 5% to 30%
  • So, the values X = 15% and Z = 10% do indeed fall in the acceptable range of values of X and Z.
  • So, Statement III is true for one particular pair of X and Z
  • Therefore, Statement III is not definitely false
 
  • Getting to the answer
    • We’ve seen above that out of Statements I, II and III, only Statement I is definitely false.
Looking at the answer choices, we see that the correct answer is Option A
View all questions of this test
Most Upvoted Answer
In a certain group of women, 70 percent of the women were employed and...
Cannot Be True Statements:
1. No woman in the group who had a graduate degree was unemployed.
2. Less than half of the women in the group were employed and had a graduate degree.
3. The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degree.

Explanation:
To determine which statement cannot be true, let's analyze each statement one by one.

Statement 1: No woman in the group who had a graduate degree was unemployed.
- Since 70 percent of the women in the group were employed, it is possible that some of them have graduate degrees.
- However, the statement says that no woman with a graduate degree was unemployed.
- This statement cannot be true because it is possible for some women with graduate degrees to be unemployed.

Statement 2: Less than half of the women in the group were employed and had a graduate degree.
- The statement implies that the number of women with both employment and a graduate degree is less than half of the total group.
- Since 70 percent of the women in the group were employed, it is possible that more than half of them have employment without a graduate degree.
- Therefore, this statement can be true.

Statement 3: The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degree.
- Let's assume there are 100 women in the group.
- 70 percent of them are employed, which means 70 women are employed.
- Since 25 percent of them do not have a graduate degree, 25 percent of 70 women or 17.5 women do not have a graduate degree and are employed.
- If the number of unemployed women with a graduate degree is 50 percent greater than the number of employed women without a graduate degree, it would mean there are 26.25 unemployed women with a graduate degree.
- However, since the number of women cannot be in decimals, this statement cannot be true.

Conclusion:
Based on the analysis, the statement that cannot be true is statement 1: No woman in the group who had a graduate degree was unemployed.
Attention GMAT Students!
To make sure you are not studying endlessly, EduRev has designed GMAT study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in GMAT.
< Previous Question Next Question >
Explore Courses for GMAT exam

Similar GMAT Doubts

Comparable worth, as a standard applied to eliminate inequities in pay, insists that the values of certain tasks performed in dissimilar jobs can be compared. In the last decade, this approach has become a critical social policy issue, as large numbers of private-sector firms and industries as well as federal, state, and local governmental entities have adopted comparable worth policies or begun to consider doing so.This widespread institutional awareness of comparable worth indicates increased public awareness that pay inequities—that is, situations in which pay is not "fair" because it does not reflect the true value of a job—exist in the labor market. However, the question still remains: have the gains already made in pay equity under comparable worth principles been of a precedent-setting nature or are they mostly transitory, a function of concessions made by employers to mislead female employees into believing that they have made long-term pay equity gains?Comparable worth pay adjustments are indeed precedent-setting. Because of the principles driving them, other mandates that can be applied to reduce or eliminate unjustified pay gaps between male and female workers have not remedied perceived pay inequities satisfactorily for the litigants in cases in which men and women hold different jobs. But whenever comparable worth principles are applied to pay schedules, perceived unjustified pay differences are eliminated. In this sense then, comparable worth is more comprehensive than other mandates, such as the Equal Pay Act of 1963 and Title VII of the Civil Rights Act of 1964. Neither compares tasks in dissimilar jobs (that is, jobs across occupational categories) in an effort to determine whether or not what is necessary to perform these tasks—know-how, problem-solving, and accountability—can be quantified in terms of its dollar value to the employer. Comparable worth, on the other hand, takes as its premise that certain tasks in dissimilar jobs may require a similar amount of training, effort, and skill; may carry similar responsibility; may be carried on in an environment having a similar impact upon the worker; and may have a similar dollar value to the employer.It can be inferred from the passage that application of "other mandates" (see highlighted text) would be unlikely to result in an outcome satisfactory to the female employees in which of the following situations?I. Males employed as long-distance truck drivers for a furniture company make $3.50 more per hour than do females with comparable job experience employed in the same capacity.II. Women working in the office of a cement company contend that their jobs are as demanding and valuable as those of the men working in the cement factory, but the women are paid much less per hour.III. A law firm employs both male and female paralegals with the same educational and career backgrounds, but the starting salary for male paralegals is $5,000 more than for female paralegals.

To remain financially sound, health insurance companies must charge higher rates to insure people considered a higher risk. Lacking complete information about individuals, insurers are forced to set a standard rate, based on the average risk of the group, for a particular segment of the population. Consumers in poor health are willing to pay for the insurance, knowing that it will cover their higher-than-average health-care costs. In contrast, healthy consumers often decide to forgo the insurance, reasoning that it is less expensive to pay out-of-pocket for their lower-than-average health-care costs. The result, called “adverse selection,” is that the riskier members of a group will comprise the group of insurance applicants, potentially leading to a market failure in which insurance companies cannot afford to offer insurance at any price.Among people over age sixty-five, even the wealthy can have difficulty obtaining fairly priced medical insurance, simply because of their age. However, those who blame so-called insurance company greed and discrimination against the elderly are ignoring the reality of adverse selection. Younger people generally obtain health insurance through their employers’ group insurance plan.Employer’s plans obligate all employees to enroll in the plan and effectively pre-screen for general health, as a minimum health level is required to hold a job. Insurance companies can therefore charge a lower premium, based on the lower average risk of the employee pool, without worrying that healthy employees will opt out of the plan.Consumers over sixty-five, typically not employed and thus seeking insurance individually, are necessarily more vulnerable to market failure stemming from adverse selection.Q.The passage states which of the following about the cost of health-care?

Directions: Read the Passage carefully and answer the question as follow.The annual labour of every nation is the fund which originally supplies it with all the necessaries and conveniences of life which it annually consumes, and which consist always either in the immediate produce of that labour, or in what is purchased with that produce from other nations. Accordingly, therefore, as this produce, or what is purchased with it, bears a greater or smaller proportion to the number of those who are to consume it, the nation will be better or worse supplied with all the necessaries and conveniences for which it has occasion.But this proportion must in every nation be regulated by two different circumstances: first, by the skill, dexterity, and judgment with which its labour is generally applied; and, second, by the proportion of the number of people who are employed in useful labour to that of those who are not so employed. Whatever be the soil, climate, or extent of territory of any particular nation, the abundance or scantiness of its annual supply must, in that particular situation, depend upon these two circumstances.Moreover, the abundance or scantiness of this supply seems to depend more upon the former of those two circumstances than upon the latter. Among the savage nations of hunters and fishers, every individual who is able to work is more or less employed in useful labour, and endeavours to provide, as well as he can, the necessities and conveniences of life, for himself, and such of his family or tribe as are either too old, or too young, or too infirm to go hunting and fishing. Such nations, however, are so miserably poor, that, from mere want, they are frequently reduced, or at least think themselves reduced, to the necessity sometimes of directly destroying, and sometimes of abandoning their infants, their old people, and those afflicted with lingering diseases, to perish with hunger, or to be devoured by wild beasts. Among civilized and thriving nations, on the contrary, though a great number of people do not labour at all, many of whom consume the produce of ten times, frequently of a hundred times, more labour than the greater part of those who work; yet the produce of the whole labour of the society is so great, that all are often abundantly supplied and a workman, even of the lowest and poorest order, if he is frugal and industrious, may enjoy a greater share of the necessities and conveniences of life than is possible for any savage to acquire.Q.According to the information in the passage, each of the following could be a characteristic of a savage nation EXCEPT

To remain financially sound, health insurance companies must charge higher rates to insure people considered a higher risk. Lacking complete information about individuals, insurers are forced to set a standard rate, based on the average risk of the group, for a particular segment of the population. Consumers in poor health are willing to pay for the insurance, knowing that it will cover their higher-than-average health-care costs. In contrast, healthy consumers often decide to forgo the insurance, reasoning that it is less expensive to pay out-of-pocket for their lower-than-average health-care costs. The result, called “adverse selection,” is that the riskier members of a group will comprise the group of insurance applicants, potentially leading to a market failure in which insurance companies cannot afford to offer insurance at any price.Among people over age sixty-five, even the wealthy can have difficulty obtaining fairly priced medical insurance, simply because of their age. However, those who blame so-called insurance company greed and discrimination against the elderly are ignoring the reality of adverse selection. Younger people generally obtain health insurance through their employers’ group insurance plan.Employer’s plans obligate all employees to enroll in the plan and effectively pre-screen for general health, as a minimum health level is required to hold a job. Insurance companies can therefore charge a lower premium, based on the lower average risk of the employee pool, without worrying that healthy employees will opt out of the plan.Consumers over sixty-five, typically not employed and thus seeking insurance individually, are necessarily more vulnerable to market failure stemming from adverse selection.Q.It can be inferred from the passage that unemployed people

Top Courses for GMATView all

Top Courses for GMAT

In a certain group of women, 70 percent of the women were employed and 25 percent did not have a graduate degree. Which of the following statements cannot be true? No woman in the group who had a graduate degree was unemployed Less than half of the women in the group were employed and had a graduate degree The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degreea)I onlyb)II onlyc)III onlyd)I and III onlye)II and III onlyCorrect answer is option 'A'. Can you explain this answer?
Question Description
In a certain group of women, 70 percent of the women were employed and 25 percent did not have a graduate degree. Which of the following statements cannot be true? No woman in the group who had a graduate degree was unemployed Less than half of the women in the group were employed and had a graduate degree The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degreea)I onlyb)II onlyc)III onlyd)I and III onlye)II and III onlyCorrect answer is option 'A'. Can you explain this answer? for GMAT 2024 is part of GMAT preparation. The Question and answers have been prepared according to the GMAT exam syllabus. Information about In a certain group of women, 70 percent of the women were employed and 25 percent did not have a graduate degree. Which of the following statements cannot be true? No woman in the group who had a graduate degree was unemployed Less than half of the women in the group were employed and had a graduate degree The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degreea)I onlyb)II onlyc)III onlyd)I and III onlye)II and III onlyCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for GMAT 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a certain group of women, 70 percent of the women were employed and 25 percent did not have a graduate degree. Which of the following statements cannot be true? No woman in the group who had a graduate degree was unemployed Less than half of the women in the group were employed and had a graduate degree The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degreea)I onlyb)II onlyc)III onlyd)I and III onlye)II and III onlyCorrect answer is option 'A'. Can you explain this answer?.
Solutions for In a certain group of women, 70 percent of the women were employed and 25 percent did not have a graduate degree. Which of the following statements cannot be true? No woman in the group who had a graduate degree was unemployed Less than half of the women in the group were employed and had a graduate degree The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degreea)I onlyb)II onlyc)III onlyd)I and III onlye)II and III onlyCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for GMAT. Download more important topics, notes, lectures and mock test series for GMAT Exam by signing up for free.
Here you can find the meaning of In a certain group of women, 70 percent of the women were employed and 25 percent did not have a graduate degree. Which of the following statements cannot be true? No woman in the group who had a graduate degree was unemployed Less than half of the women in the group were employed and had a graduate degree The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degreea)I onlyb)II onlyc)III onlyd)I and III onlye)II and III onlyCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of In a certain group of women, 70 percent of the women were employed and 25 percent did not have a graduate degree. Which of the following statements cannot be true? No woman in the group who had a graduate degree was unemployed Less than half of the women in the group were employed and had a graduate degree The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degreea)I onlyb)II onlyc)III onlyd)I and III onlye)II and III onlyCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for In a certain group of women, 70 percent of the women were employed and 25 percent did not have a graduate degree. Which of the following statements cannot be true? No woman in the group who had a graduate degree was unemployed Less than half of the women in the group were employed and had a graduate degree The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degreea)I onlyb)II onlyc)III onlyd)I and III onlye)II and III onlyCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of In a certain group of women, 70 percent of the women were employed and 25 percent did not have a graduate degree. Which of the following statements cannot be true? No woman in the group who had a graduate degree was unemployed Less than half of the women in the group were employed and had a graduate degree The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degreea)I onlyb)II onlyc)III onlyd)I and III onlye)II and III onlyCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an ample number of questions to practice In a certain group of women, 70 percent of the women were employed and 25 percent did not have a graduate degree. Which of the following statements cannot be true? No woman in the group who had a graduate degree was unemployed Less than half of the women in the group were employed and had a graduate degree The number of unemployed women with a graduate degree was 50 percent greater than the number of employed women without a graduate degreea)I onlyb)II onlyc)III onlyd)I and III onlye)II and III onlyCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice GMAT tests.
Explore Courses for GMAT exam

Top Courses for GMAT

Explore Courses

Suggested Free Tests

Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
10M+ students study on EduRev
Get App
© EduRev
Education Revolution
Signup to see your scores go up
within 7 days!
Takes less than 10 seconds to signup