GMAT Exam > GMAT Questions > A rectangular photograph shown by the white r...
Start Learning for Free
A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?
(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.
(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.
- a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
- b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
- c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
- d)EACH statement ALONE is sufficient.
- e)Statements (1) and (2) TOGETHER are NOT sufficient.
Correct answer is option 'C'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A rectangular photograph shown by the white region in the figure is en...
Steps 1 & 2: Understand Question and Draw Inferences
Notice that the frame has the same width throughout the border.
Let us say that the longer dimension of the photograph is L, the smaller dimension of the photograph is B and the width of the frame is x.
Then, AB = L + 2x
And, BC = B + 2x
We need to find the ratio of AB:BC. To do so, we ought to know the values of L, B, and x.
Step 3: Analyze Statement 1
The ratio of the longer dimension to the smaller dimension of the photograph is 3:2
Let’s say that for the white area the length of the longer dimension and the length of the shorter dimension are 3z and 2z where z is a positive number.
Thus,
With different values of z and x, we shall obtain different values of the required ratio.
For example, if z =1, x = 1, then AB:BC = 5:4. Whereas, if z = 1, x = 2, then AB:BC = 7:6.
INSUFFICIENT.
Step 4: Analyze Statement 2
If a frame of twice the width was used then the ratio of AB to BC would have been 5:4
In this case, AB = L + 2(2x) = L + 4x
And, BC = B + 2(2x) = B + 4x
We are given that AB:BC = 5 : 4
We need to find the ratio of 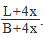
Without the values of L, B, and x, it is impossible to determine its value.
INSUFFICIENT.
Step 5: Analyze Both Statements Together (if needed)
Combining statements (1) and (2),
We know that L:B = 3:2, but we do not know the values of L and B.
Let’s assume the values of L and B to be 3z and 2z and the original width is x.
Then,
Required ratio
The ratio has been determined.
SUFFICIENT.
(C) is the correct answer.

|
Explore Courses for GMAT exam
|

|
Similar GMAT Doubts
A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer?
Question Description
A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer? for GMAT 2024 is part of GMAT preparation. The Question and answers have been prepared according to the GMAT exam syllabus. Information about A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for GMAT 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer?.
A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer? for GMAT 2024 is part of GMAT preparation. The Question and answers have been prepared according to the GMAT exam syllabus. Information about A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for GMAT 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer?.
Solutions for A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for GMAT.
Download more important topics, notes, lectures and mock test series for GMAT Exam by signing up for free.
Here you can find the meaning of A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer?, a detailed solution for A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer? has been provided alongside types of A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.d)EACH statement ALONE is sufficient.e)Statements (1) and (2) TOGETHER are NOT sufficient.Correct answer is option 'C'. Can you explain this answer? tests, examples and also practice GMAT tests.

|
Explore Courses for GMAT exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.























