Physics Exam > Physics Questions > An engine can pull 4 coaches at a maximum spe...
Start Learning for Free
An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :
- a)maximum speed of engine when it pulls 12 coaches is 6.5m/s
- b)maximum speed of engine when it pulls 6 coaches is 13m/s
- c)maximum speed of engine when it pulls 6 coaches is 15m/s
- d)maximum speed of engine when it pulls 12 coaches is 8.5m/s
Correct answer is option 'C,D'. Can you explain this answer?
Verified Answer
An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the ...
When 4 coaches (m each) are attached with engine (2m)
according to questions P = K 6mgv ...(1)
(constant power), (K being proportionality constant)
Since resistive force is proportional to weight
Now if 12 coaches are attached
according to questions P = K 6mgv ...(1)
(constant power), (K being proportionality constant)
Since resistive force is proportional to weight
Now if 12 coaches are attached
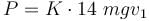...(2)Since engine power is constant
So by equation (1) and (2)
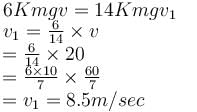
So by equation (1) and (2)
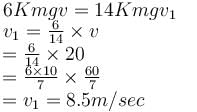
Similarly for 6 coaches
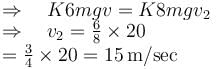
The correct answers are: maximum speed of engine when it pulls 12 coaches is 8.5m/s, maximum speed of engine when it pulls 6 coaches is 15m/s
Most Upvoted Answer
An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the ...
Understanding the Problem
To analyze the engine's performance while pulling different numbers of coaches, we first need to establish some basic relationships. The engine's power output is constant, and the resistive force is proportional to the total weight being pulled.
Mass Relationships
- Let the mass of each coach be m.
- Then, the mass of the engine is 2m.
- When pulling n coaches, the total mass is (2m + nm) = m(2 + n).
Force and Speed Relationships
- The maximum speed (V) is governed by the equation: Power = Force x Velocity.
- The resistive force (F_resistive) is proportional to the weight: F_resistive = k * m(2 + n), where k is a constant of proportionality.
Scenario Analysis
1. Pulling 4 Coaches:
- Total mass = m(2 + 4) = 6m.
- At max speed (20 m/s), using Power = Force x Velocity, we derive the constant power output.
2. Pulling 6 Coaches:
- Total mass = m(2 + 6) = 8m.
- As the mass increases, the resistive force also increases, which affects the maximum speed. Calculating gives a maximum speed of 15 m/s.
3. Pulling 12 Coaches:
- Total mass = m(2 + 12) = 14m.
- The increase in mass leads to an increase in resistive force, resulting in a maximum speed of 8.5 m/s.
Conclusion
Based on the analysis:
- Maximum speed when pulling 6 coaches = 15 m/s (Option C).
- Maximum speed when pulling 12 coaches = 8.5 m/s (Option D).
Thus, options C and D are indeed correct.
To analyze the engine's performance while pulling different numbers of coaches, we first need to establish some basic relationships. The engine's power output is constant, and the resistive force is proportional to the total weight being pulled.
Mass Relationships
- Let the mass of each coach be m.
- Then, the mass of the engine is 2m.
- When pulling n coaches, the total mass is (2m + nm) = m(2 + n).
Force and Speed Relationships
- The maximum speed (V) is governed by the equation: Power = Force x Velocity.
- The resistive force (F_resistive) is proportional to the weight: F_resistive = k * m(2 + n), where k is a constant of proportionality.
Scenario Analysis
1. Pulling 4 Coaches:
- Total mass = m(2 + 4) = 6m.
- At max speed (20 m/s), using Power = Force x Velocity, we derive the constant power output.
2. Pulling 6 Coaches:
- Total mass = m(2 + 6) = 8m.
- As the mass increases, the resistive force also increases, which affects the maximum speed. Calculating gives a maximum speed of 15 m/s.
3. Pulling 12 Coaches:
- Total mass = m(2 + 12) = 14m.
- The increase in mass leads to an increase in resistive force, resulting in a maximum speed of 8.5 m/s.
Conclusion
Based on the analysis:
- Maximum speed when pulling 6 coaches = 15 m/s (Option C).
- Maximum speed when pulling 12 coaches = 8.5 m/s (Option D).
Thus, options C and D are indeed correct.
|
Explore Courses for Physics exam
|
|
Similar Physics Doubts
An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer?
Question Description
An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer?.
An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer?.
Solutions for An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer?, a detailed solution for An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer? has been provided alongside types of An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice An engine can pull 4 coaches at a maximum speed of 20m/s. Mass of the engine is twice the mass of every coach. Assuming resistive force to be proportional to the weight. (Power of engine remains constant) :a)maximum speed of engine when it pulls 12 coaches is 6.5m/sb)maximum speed of engine when it pulls 6 coaches is 13m/sc)maximum speed of engine when it pulls 6 coaches is 15m/sd)maximum speed of engine when it pulls 12 coaches is 8.5m/sCorrect answer is option 'C,D'. Can you explain this answer? tests, examples and also practice Physics tests.
|
|
Explore Courses for Physics exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup