Mathematics Exam > Mathematics Questions > If G is a group, Z its center and if G/Z is c...
Start Learning for Free
If G is a group, Z its center and if G/Z is cyclic then G
- a)must be abelian
- b)must be non abelian
- c)must be normal subgroup
- d)must be subgroup
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
If G is a group, Z its center and if G/Z is cyclic then Ga)must be abe...
We have given that G/Z is a cyclic group, so let Zg is a generator of the cyclic group G/Z, where g ∈ G.
W e now show that G is an abelian group i.e.,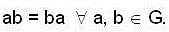
Since a ∈ G, so Za ∈ G/Z. But G/Z is a cyclic group which is gen era ted by Zg. Thus there exists an integer m such that
W e now show that G is an abelian group i.e.,
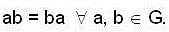
Since a ∈ G, so Za ∈ G/Z. But G/Z is a cyclic group which is gen era ted by Zg. Thus there exists an integer m such that
Za = (Zg)m = Zgm [∵ Z is a normal subgroup of G]
Again
a ∈ Za and Za = Zgm ⇒ a ∈ Z gm.
Now
a ∈ Z g m ⇒ 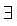 z1 ∈ Z such that a ∈ z1 gm
z1 ∈ Z such that a ∈ z1 gm
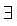 z1 ∈ Z such that a ∈ z1 gm
z1 ∈ Z such that a ∈ z1 gmSimilarly, for b ∈ G, b = z2 gn, where z2 ∈ z and n is any integer.
Now
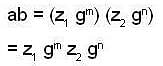
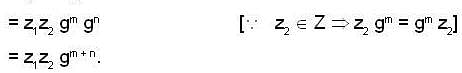 Again
Again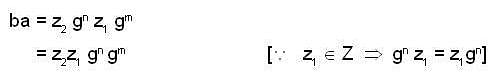
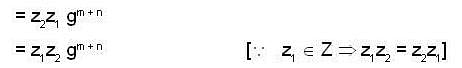
Most Upvoted Answer
If G is a group, Z its center and if G/Z is cyclic then Ga)must be abe...
Explanation:
To understand why option 'A' is the correct answer, let's break down the given information.
G is a group:
A group is a set of elements with a binary operation (usually denoted as *) that satisfies the following properties:
1. Closure: For any two elements a and b in the group G, the result of their operation a*b is also in G.
2. Associativity: For any three elements a, b, and c in G, the operation is associative, meaning (a*b)*c = a*(b*c).
3. Identity: There exists an identity element e in G such that for any element a in G, a*e = e*a = a.
4. Inverse: For every element a in G, there exists an inverse element a^(-1) in G such that a*a^(-1) = a^(-1)*a = e.
Z is the center of G:
The center of a group G, denoted as Z(G), is the set of all elements in G that commute with every element in G. In other words, for any element z in Z(G) and any element g in G, we have zg = gz.
G/Z is cyclic:
The quotient group G/Z is cyclic, which means that there exists an element in G/Z, denoted as gZ, such that every element in G/Z can be written as (gZ)^n for some integer n. In other words, the elements of G/Z can be generated by repeatedly multiplying gZ by itself.
Implications:
Given this information, we can draw the following implications:
1. G/Z being cyclic implies that every element in G/Z can be written as (gZ)^n:
This means that for any element x in G/Z, there exists an integer n such that x = (gZ)^n. Since Z is the center of G, every element in Z commutes with every element in G. Therefore, every element in G/Z commutes with every element in G.
2. Every element in G/Z commutes with every element in G:
Since every element in G/Z can be written as (gZ)^n for some integer n, and every element in G/Z commutes with every element in G, it follows that every element in G commutes with every other element in G.
Conclusion:
Based on the implications above, we can conclude that if G/Z is cyclic, then every element in G commutes with every other element in G. This implies that G is an abelian group, meaning it is commutative. Therefore, option 'A' is the correct answer.
To understand why option 'A' is the correct answer, let's break down the given information.
G is a group:
A group is a set of elements with a binary operation (usually denoted as *) that satisfies the following properties:
1. Closure: For any two elements a and b in the group G, the result of their operation a*b is also in G.
2. Associativity: For any three elements a, b, and c in G, the operation is associative, meaning (a*b)*c = a*(b*c).
3. Identity: There exists an identity element e in G such that for any element a in G, a*e = e*a = a.
4. Inverse: For every element a in G, there exists an inverse element a^(-1) in G such that a*a^(-1) = a^(-1)*a = e.
Z is the center of G:
The center of a group G, denoted as Z(G), is the set of all elements in G that commute with every element in G. In other words, for any element z in Z(G) and any element g in G, we have zg = gz.
G/Z is cyclic:
The quotient group G/Z is cyclic, which means that there exists an element in G/Z, denoted as gZ, such that every element in G/Z can be written as (gZ)^n for some integer n. In other words, the elements of G/Z can be generated by repeatedly multiplying gZ by itself.
Implications:
Given this information, we can draw the following implications:
1. G/Z being cyclic implies that every element in G/Z can be written as (gZ)^n:
This means that for any element x in G/Z, there exists an integer n such that x = (gZ)^n. Since Z is the center of G, every element in Z commutes with every element in G. Therefore, every element in G/Z commutes with every element in G.
2. Every element in G/Z commutes with every element in G:
Since every element in G/Z can be written as (gZ)^n for some integer n, and every element in G/Z commutes with every element in G, it follows that every element in G commutes with every other element in G.
Conclusion:
Based on the implications above, we can conclude that if G/Z is cyclic, then every element in G commutes with every other element in G. This implies that G is an abelian group, meaning it is commutative. Therefore, option 'A' is the correct answer.
Free Test
FREE
| Start Free Test |
Community Answer
If G is a group, Z its center and if G/Z is cyclic then Ga)must be abe...
We have given that G/Z is a cyclic group, so let Zg is a generator of the cyclic group G/Z, where g ∈ G.
W e now show that G is an abelian group i.e.,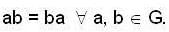
Since a ∈ G, so Za ∈ G/Z. But G/Z is a cyclic group which is gen era ted by Zg. Thus there exists an integer m such that
W e now show that G is an abelian group i.e.,
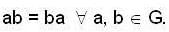
Since a ∈ G, so Za ∈ G/Z. But G/Z is a cyclic group which is gen era ted by Zg. Thus there exists an integer m such that
Za = (Zg)m = Zgm [∵ Z is a normal subgroup of G]
Again
a ∈ Za and Za = Zgm ⇒ a ∈ Z gm.
Now
a ∈ Z g m ⇒  z1 ∈ Z such that a ∈ z1 gm
z1 ∈ Z such that a ∈ z1 gm
 z1 ∈ Z such that a ∈ z1 gm
z1 ∈ Z such that a ∈ z1 gmSimilarly, for b ∈ G, b = z2 gn, where z2 ∈ z and n is any integer.
Now
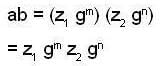
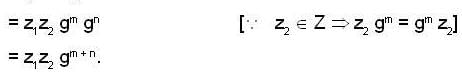 Again
Again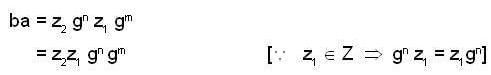
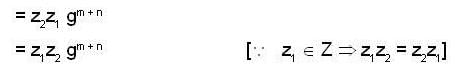

|
Explore Courses for Mathematics exam
|

|
Similar Mathematics Doubts
If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer?
Question Description
If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer? for Mathematics 2024 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for Mathematics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer?.
If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer? for Mathematics 2024 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for Mathematics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer?.
Solutions for If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mathematics.
Download more important topics, notes, lectures and mock test series for Mathematics Exam by signing up for free.
Here you can find the meaning of If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice If G is a group, Z its center and if G/Z is cyclic then Ga)must be abelianb)must be non abelianc)must be normal subgroupd)must be subgroupCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice Mathematics tests.

|
Explore Courses for Mathematics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















