Physics Exam > Physics Questions > A Michelson interferometer is illuminated wit...
Start Learning for Free
A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).
Correct answer is '495.1'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A Michelson interferometer is illuminated with monochromatic light. Wh...
In Michelson interferometer; the path differential must satisfy the condition of maxima; Let initially the no. of fringe is 'n', then
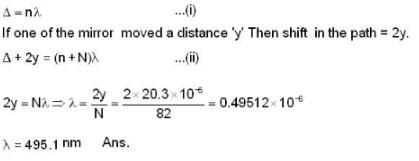

Most Upvoted Answer
A Michelson interferometer is illuminated with monochromatic light. Wh...
To analyze the behavior of a Michelson interferometer when one of the mirrors is moved, we can consider the interference pattern that is formed.
In a Michelson interferometer, a beam of monochromatic light is split into two paths by a beam splitter. One path is reflected by a mirror and the other path is transmitted through the beam splitter and reflected by another mirror. The two paths are then recombined at the beam splitter, and interference occurs between the two beams.
When one of the mirrors is moved, the optical path length of one of the paths changes, leading to a phase difference between the two beams. This phase difference results in a shift in the interference pattern observed at the output of the interferometer.
The distance that the mirror is moved, denoted as "d," determines the change in the optical path length. In this case, the mirror is moved through a distance of 20.3 units.
To calculate the resulting phase difference, we need to consider the wavelength of the monochromatic light, denoted as "λ." The phase difference is given by:
Δφ = (2π/λ) * d
where Δφ is the phase difference in radians.
Therefore, the phase difference resulting from moving the mirror through a distance of 20.3 units is:
Δφ = (2π/λ) * 20.3
It's important to note that the interference pattern observed at the output of the interferometer will depend on the specific geometry of the setup, including the position of the mirrors, the angle of the beam splitter, and the distance between the mirrors.
In a Michelson interferometer, a beam of monochromatic light is split into two paths by a beam splitter. One path is reflected by a mirror and the other path is transmitted through the beam splitter and reflected by another mirror. The two paths are then recombined at the beam splitter, and interference occurs between the two beams.
When one of the mirrors is moved, the optical path length of one of the paths changes, leading to a phase difference between the two beams. This phase difference results in a shift in the interference pattern observed at the output of the interferometer.
The distance that the mirror is moved, denoted as "d," determines the change in the optical path length. In this case, the mirror is moved through a distance of 20.3 units.
To calculate the resulting phase difference, we need to consider the wavelength of the monochromatic light, denoted as "λ." The phase difference is given by:
Δφ = (2π/λ) * d
where Δφ is the phase difference in radians.
Therefore, the phase difference resulting from moving the mirror through a distance of 20.3 units is:
Δφ = (2π/λ) * 20.3
It's important to note that the interference pattern observed at the output of the interferometer will depend on the specific geometry of the setup, including the position of the mirrors, the angle of the beam splitter, and the distance between the mirrors.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer?
Question Description
A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer?.
A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer?.
Solutions for A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer?, a detailed solution for A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer? has been provided alongside types of A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A Michelson interferometer is illuminated with monochromatic light. When one of the mirror is moved through a distance of 20.3μm, 82 fringes pass through the cross-wire. The wavelength of the monochromatic light is _______(in nm).Correct answer is '495.1'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















