Electrical Engineering (EE) Exam > Electrical Engineering (EE) Questions > A uniform plane wave travelling in air is inc...
Start Learning for Free
A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence is
- a)zero
- b)0.5 ∠180º
- c)0.33 ∠0º
- d)0.33 ∠180º
Correct answer is option 'D'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A uniform plane wave travelling in air is incident on the plane bounda...
Reflection coefficient 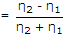
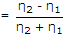
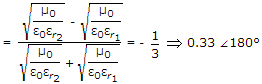 .
.Most Upvoted Answer
A uniform plane wave travelling in air is incident on the plane bounda...
A different refractive index. Upon reaching the boundary, the wave is partially reflected and partially transmitted into the second medium. The amount of reflection and transmission depends on the angle of incidence and the refractive indices of the two media.
The behavior of the wave at the boundary can be described using the Fresnel equations, which relate the amplitudes of the reflected and transmitted waves to the angle of incidence and the refractive indices of the two media.
For a wave incident from air onto a dielectric medium, the Fresnel equations are:
\begin{align*}
r_\parallel &= \frac{n_1 \cos \theta_i - n_2 \cos \theta_t}{n_1 \cos \theta_i + n_2 \cos \theta_t} \\
t_\parallel &= \frac{2 n_1 \cos \theta_i}{n_1 \cos \theta_i + n_2 \cos \theta_t} \\
r_\perp &= \frac{n_2 \cos \theta_i - n_1 \cos \theta_t}{n_2 \cos \theta_i + n_1 \cos \theta_t} \\
t_\perp &= \frac{2 n_1 \cos \theta_i}{n_2 \cos \theta_i + n_1 \cos \theta_t}
\end{align*}
where $n_1$ is the refractive index of air (approximately 1), $n_2$ is the refractive index of the second medium, $\theta_i$ is the angle of incidence (measured from the normal to the boundary), and $\theta_t$ is the angle of transmission (also measured from the normal).
$r_\parallel$ and $r_\perp$ are the amplitude reflection coefficients for parallel and perpendicular polarization, respectively, and $t_\parallel$ and $t_\perp$ are the amplitude transmission coefficients. The subscripts indicate the polarization direction relative to the plane of incidence.
The total reflection and transmission coefficients can be calculated by summing the squares of the parallel and perpendicular coefficients:
\begin{align*}
R &= |r_\parallel|^2 + |r_\perp|^2 \\
T &= |t_\parallel|^2 + |t_\perp|^2
\end{align*}
where $R$ is the reflectance (the fraction of incident power that is reflected) and $T$ is the transmittance (the fraction of incident power that is transmitted).
At normal incidence ($\theta_i = 0$), the reflection and transmission coefficients simplify to:
\begin{align*}
r_\parallel &= \frac{n_1 - n_2}{n_1 + n_2} \\
t_\parallel &= \frac{2 n_1}{n_1 + n_2} \\
r_\perp &= \frac{n_2 - n_1}{n_2 + n_1} \\
t_\perp &= \frac{2 n_1}{n_2 + n_1} \\
R &= \frac{(n_1 - n_2)^2}{(n_1 + n_2)^2} \\
T &= \frac{4 n_1 n_2}{(n_1 + n_2)^2}
\end{align*}
In general, the amount of reflection increases as the angle of incidence increases, and is maximum
The behavior of the wave at the boundary can be described using the Fresnel equations, which relate the amplitudes of the reflected and transmitted waves to the angle of incidence and the refractive indices of the two media.
For a wave incident from air onto a dielectric medium, the Fresnel equations are:
\begin{align*}
r_\parallel &= \frac{n_1 \cos \theta_i - n_2 \cos \theta_t}{n_1 \cos \theta_i + n_2 \cos \theta_t} \\
t_\parallel &= \frac{2 n_1 \cos \theta_i}{n_1 \cos \theta_i + n_2 \cos \theta_t} \\
r_\perp &= \frac{n_2 \cos \theta_i - n_1 \cos \theta_t}{n_2 \cos \theta_i + n_1 \cos \theta_t} \\
t_\perp &= \frac{2 n_1 \cos \theta_i}{n_2 \cos \theta_i + n_1 \cos \theta_t}
\end{align*}
where $n_1$ is the refractive index of air (approximately 1), $n_2$ is the refractive index of the second medium, $\theta_i$ is the angle of incidence (measured from the normal to the boundary), and $\theta_t$ is the angle of transmission (also measured from the normal).
$r_\parallel$ and $r_\perp$ are the amplitude reflection coefficients for parallel and perpendicular polarization, respectively, and $t_\parallel$ and $t_\perp$ are the amplitude transmission coefficients. The subscripts indicate the polarization direction relative to the plane of incidence.
The total reflection and transmission coefficients can be calculated by summing the squares of the parallel and perpendicular coefficients:
\begin{align*}
R &= |r_\parallel|^2 + |r_\perp|^2 \\
T &= |t_\parallel|^2 + |t_\perp|^2
\end{align*}
where $R$ is the reflectance (the fraction of incident power that is reflected) and $T$ is the transmittance (the fraction of incident power that is transmitted).
At normal incidence ($\theta_i = 0$), the reflection and transmission coefficients simplify to:
\begin{align*}
r_\parallel &= \frac{n_1 - n_2}{n_1 + n_2} \\
t_\parallel &= \frac{2 n_1}{n_1 + n_2} \\
r_\perp &= \frac{n_2 - n_1}{n_2 + n_1} \\
t_\perp &= \frac{2 n_1}{n_2 + n_1} \\
R &= \frac{(n_1 - n_2)^2}{(n_1 + n_2)^2} \\
T &= \frac{4 n_1 n_2}{(n_1 + n_2)^2}
\end{align*}
In general, the amount of reflection increases as the angle of incidence increases, and is maximum
Attention Electrical Engineering (EE) Students!
To make sure you are not studying endlessly, EduRev has designed Electrical Engineering (EE) study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Electrical Engineering (EE).

|
Explore Courses for Electrical Engineering (EE) exam
|

|
Similar Electrical Engineering (EE) Doubts
A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer?
Question Description
A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer? for Electrical Engineering (EE) 2024 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer?.
A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer? for Electrical Engineering (EE) 2024 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer?.
Solutions for A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for Electrical Engineering (EE).
Download more important topics, notes, lectures and mock test series for Electrical Engineering (EE) Exam by signing up for free.
Here you can find the meaning of A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A uniform plane wave travelling in air is incident on the plane boundary between air and another dielectric medium with εr = 4. The reflection coefficient for the normal incidence isa)zerob)0.5 ∠180ºc)0.33 ∠0ºd)0.33 ∠180ºCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice Electrical Engineering (EE) tests.

|
Explore Courses for Electrical Engineering (EE) exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























