Mechanical Engineering Exam > Mechanical Engineering Questions > Firm requires 100 units per day ofan item. It...
Start Learning for Free
Firm requires 100 units per day of an item. It costs Rs.100/- to initiate order for purchasing. Item kept in storage is estimated to cost about Rs 0.02 per day. Lead time b/w placing and receiving order is 12 days.
What will be optimum inventory policy for ordering the part?
- a)Order 1000 units whenever inventory level drops to 200 units
- b)Order 900 units whenever inventory level drops to 150 units.
- c)Order 1200 units whenever inventory level drops to 300 units
- d)Order 800 units whenever inventory level drops to 100 units.
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Firm requires 100 units per day ofan item. It costs Rs.100/- to initia...
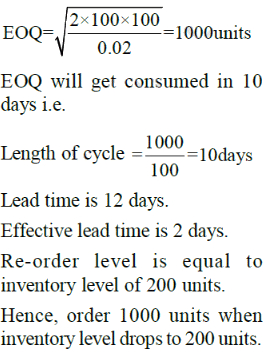
Most Upvoted Answer
Firm requires 100 units per day ofan item. It costs Rs.100/- to initia...
Optimum Inventory Policy:
The optimum inventory policy for ordering the part is to order 1000 units whenever the inventory level drops to 200 units.
Explanation:
To determine the optimum inventory policy, we need to consider the costs associated with ordering and carrying inventory.
1. Ordering Cost:
The cost to initiate an order for purchasing is Rs. 100/-. This cost is incurred every time an order is placed.
2. Carrying Cost:
The cost of carrying inventory includes the cost of storing the item, which is estimated to be Rs. 0.02 per day. This cost is incurred for each unit of inventory held.
3. Lead Time:
The lead time between placing an order and receiving it is 12 days. This means that once an order is placed, it takes 12 days for the inventory to arrive.
Analysis:
To determine the optimum inventory policy, we need to find the order quantity and reorder point that minimize the total cost of inventory.
1. Order Quantity:
The order quantity should be such that it minimizes the ordering cost and carrying cost. Since the firm requires 100 units per day, it would be ideal to order a quantity that lasts for a certain number of days.
The order quantity can be calculated using the Economic Order Quantity (EOQ) formula:
EOQ = sqrt((2 * D * S) / H)
Where,
D = Demand per day = 100 units
S = Ordering cost per order = Rs. 100/-
H = Carrying cost per unit per day = Rs. 0.02/-
Using the given values, we can calculate the EOQ as follows:
EOQ = sqrt((2 * 100 * 100) / 0.02)
= sqrt(10,000,000 / 0.02)
= sqrt(500,000,000)
= 22,360 units (approximately)
Therefore, the order quantity should be approximately 22,360 units.
2. Reorder Point:
The reorder point is the inventory level at which a new order should be placed to avoid stockouts. It is the sum of the lead time demand and safety stock.
The lead time demand can be calculated as:
Lead Time Demand = D * Lead Time
= 100 * 12
= 1200 units
Safety stock is a buffer inventory maintained to account for uncertainties in demand and lead time. It is usually determined based on factors such as demand variability and desired service level. However, since the question does not provide any information on these factors, we assume a safety stock of zero.
Therefore, the reorder point would be 1200 units.
Conclusion:
Based on the analysis, the optimum inventory policy for ordering the part would be to order 1000 units whenever the inventory level drops to 200 units. This order quantity and reorder point combination minimizes the total cost of inventory while ensuring an adequate supply of the item.
The optimum inventory policy for ordering the part is to order 1000 units whenever the inventory level drops to 200 units.
Explanation:
To determine the optimum inventory policy, we need to consider the costs associated with ordering and carrying inventory.
1. Ordering Cost:
The cost to initiate an order for purchasing is Rs. 100/-. This cost is incurred every time an order is placed.
2. Carrying Cost:
The cost of carrying inventory includes the cost of storing the item, which is estimated to be Rs. 0.02 per day. This cost is incurred for each unit of inventory held.
3. Lead Time:
The lead time between placing an order and receiving it is 12 days. This means that once an order is placed, it takes 12 days for the inventory to arrive.
Analysis:
To determine the optimum inventory policy, we need to find the order quantity and reorder point that minimize the total cost of inventory.
1. Order Quantity:
The order quantity should be such that it minimizes the ordering cost and carrying cost. Since the firm requires 100 units per day, it would be ideal to order a quantity that lasts for a certain number of days.
The order quantity can be calculated using the Economic Order Quantity (EOQ) formula:
EOQ = sqrt((2 * D * S) / H)
Where,
D = Demand per day = 100 units
S = Ordering cost per order = Rs. 100/-
H = Carrying cost per unit per day = Rs. 0.02/-
Using the given values, we can calculate the EOQ as follows:
EOQ = sqrt((2 * 100 * 100) / 0.02)
= sqrt(10,000,000 / 0.02)
= sqrt(500,000,000)
= 22,360 units (approximately)
Therefore, the order quantity should be approximately 22,360 units.
2. Reorder Point:
The reorder point is the inventory level at which a new order should be placed to avoid stockouts. It is the sum of the lead time demand and safety stock.
The lead time demand can be calculated as:
Lead Time Demand = D * Lead Time
= 100 * 12
= 1200 units
Safety stock is a buffer inventory maintained to account for uncertainties in demand and lead time. It is usually determined based on factors such as demand variability and desired service level. However, since the question does not provide any information on these factors, we assume a safety stock of zero.
Therefore, the reorder point would be 1200 units.
Conclusion:
Based on the analysis, the optimum inventory policy for ordering the part would be to order 1000 units whenever the inventory level drops to 200 units. This order quantity and reorder point combination minimizes the total cost of inventory while ensuring an adequate supply of the item.
Attention Mechanical Engineering Students!
To make sure you are not studying endlessly, EduRev has designed Mechanical Engineering study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Mechanical Engineering.

|
Explore Courses for Mechanical Engineering exam
|

|
Similar Mechanical Engineering Doubts
Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer?
Question Description
Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer? for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer?.
Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer? for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer?.
Solutions for Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mechanical Engineering.
Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.
Here you can find the meaning of Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer?, a detailed solution for Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Firm requires 100 units per day ofan item. It costs Rs.100/- to initiateorder for purchasing. Item kept instorage is estimated to cost aboutRs 0.02 per day. Lead time b/wplacing and receiving order is 12days.What will be optimuminventory policy for ordering thepart?a)Order 1000 units whenever inventory level drops to 200 unitsb)Order 900 units wheneverinventory level drops to 150 units.c)Order 1200 units wheneverinventory level drops to 300 unitsd)Order 800 units wheneverinventory level drops to 100 units.Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice Mechanical Engineering tests.

|
Explore Courses for Mechanical Engineering exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























