Physics Exam > Physics Questions > In an interference pattern with two coherent ...
Start Learning for Free
In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.
- a)1600:1
- b)40:1
- c)4:1
- d)2:1
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
In an interference pattern with two coherent sources, the amplitude of...
Let us suppose that the amplitude ratio of the two interfering sources be a:1, where a>1. If Imax and Imin be the maximum and minimum intensities, then in the interference pattern, the intensity ratio is
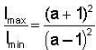
Here, if average intensity is 100 units, then the maximum is 105 minimum is 95. Therefore, the ratio is
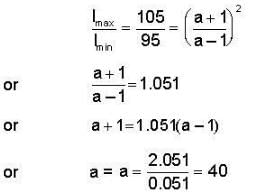
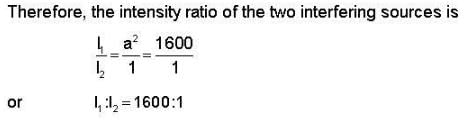
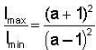
Here, if average intensity is 100 units, then the maximum is 105 minimum is 95. Therefore, the ratio is
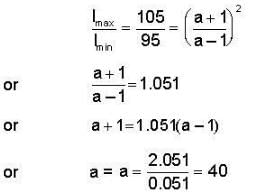
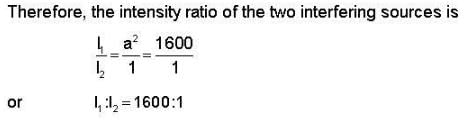
Most Upvoted Answer
In an interference pattern with two coherent sources, the amplitude of...
Interference pattern and intensity variation
Interference is a phenomenon that occurs when two or more waves overlap and combine. In the case of coherent sources, the waves have the same frequency, constant phase relationship, and are emitted from two sources with a constant phase difference between them.
When these waves interfere, they create an interference pattern characterized by regions of constructive and destructive interference. The intensity of the resulting pattern depends on the superposition of the waves.
The intensity of a wave is proportional to the square of its amplitude. In an interference pattern, the total intensity at a given point is determined by the sum of the intensities of the individual waves at that point.
Amplitude of intensity variation
In this problem, it is given that the amplitude of intensity variation is 5% of the average intensity. This means that the difference between the maximum and minimum intensities in the interference pattern is 5% of the average intensity.
The amplitude of intensity variation can be calculated using the formula:
Amplitude of intensity variation = (Imax - Imin) / (Imax + Imin)
Given that the amplitude of intensity variation is 5% of the average intensity, we can write:
0.05 = (Imax - Imin) / (Imax + Imin)
Simplifying the equation, we get:
Imax - Imin = 0.05 Imax + 0.05 Imin
0.95 Imax - 1.05 Imin = 0
Relative intensities of the interfering sources
Let's assume the intensities of the two interfering sources are I1 and I2, where I1 > I2. Using the principle of superposition, the total intensity at a given point is given by:
Itotal = I1 + I2 + 2√(I1I2)cos(θ)
In the case of constructive interference, cos(θ) = 1, and in the case of destructive interference, cos(θ) = -1.
Since the amplitude of intensity variation is 5% of the average intensity, we can write:
0.05 Iaverage = (I1 + I2) / 2
Simplifying the equation, we get:
I1 + I2 = 0.1 Iaverage
Substituting this equation into the equation for total intensity, we get:
Itotal = 0.1 Iaverage + 2√(I1I2)
From the equation 0.95 Imax - 1.05 Imin = 0, we can write:
0.95 (I1 + I2) - 1.05 √(I1I2) = 0
Substituting the equation for I1 + I2, we get:
0.095 Iaverage - 1.05 √(I1I2) = 0
Simplifying the equation, we get:
√(I1I2) = 0.091 Iaverage
Squaring both sides of the equation, we get:
I1I2 = (0.091 Iaverage)^2
Since I1 > I2, we can write:
I1 = x I2
Substituting this into the equation, we get:
x I2^2 = (0.091 Iaverage)^2
Simplifying the equation
Interference is a phenomenon that occurs when two or more waves overlap and combine. In the case of coherent sources, the waves have the same frequency, constant phase relationship, and are emitted from two sources with a constant phase difference between them.
When these waves interfere, they create an interference pattern characterized by regions of constructive and destructive interference. The intensity of the resulting pattern depends on the superposition of the waves.
The intensity of a wave is proportional to the square of its amplitude. In an interference pattern, the total intensity at a given point is determined by the sum of the intensities of the individual waves at that point.
Amplitude of intensity variation
In this problem, it is given that the amplitude of intensity variation is 5% of the average intensity. This means that the difference between the maximum and minimum intensities in the interference pattern is 5% of the average intensity.
The amplitude of intensity variation can be calculated using the formula:
Amplitude of intensity variation = (Imax - Imin) / (Imax + Imin)
Given that the amplitude of intensity variation is 5% of the average intensity, we can write:
0.05 = (Imax - Imin) / (Imax + Imin)
Simplifying the equation, we get:
Imax - Imin = 0.05 Imax + 0.05 Imin
0.95 Imax - 1.05 Imin = 0
Relative intensities of the interfering sources
Let's assume the intensities of the two interfering sources are I1 and I2, where I1 > I2. Using the principle of superposition, the total intensity at a given point is given by:
Itotal = I1 + I2 + 2√(I1I2)cos(θ)
In the case of constructive interference, cos(θ) = 1, and in the case of destructive interference, cos(θ) = -1.
Since the amplitude of intensity variation is 5% of the average intensity, we can write:
0.05 Iaverage = (I1 + I2) / 2
Simplifying the equation, we get:
I1 + I2 = 0.1 Iaverage
Substituting this equation into the equation for total intensity, we get:
Itotal = 0.1 Iaverage + 2√(I1I2)
From the equation 0.95 Imax - 1.05 Imin = 0, we can write:
0.95 (I1 + I2) - 1.05 √(I1I2) = 0
Substituting the equation for I1 + I2, we get:
0.095 Iaverage - 1.05 √(I1I2) = 0
Simplifying the equation, we get:
√(I1I2) = 0.091 Iaverage
Squaring both sides of the equation, we get:
I1I2 = (0.091 Iaverage)^2
Since I1 > I2, we can write:
I1 = x I2
Substituting this into the equation, we get:
x I2^2 = (0.091 Iaverage)^2
Simplifying the equation

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer?
Question Description
In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer?.
In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer?.
Solutions for In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer?, a detailed solution for In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice In an interference pattern with two coherent sources, the amplitude of intensity variation is found to be 5% of the average intensity. Calculate the relative intensities of the interfering sources.a)1600:1b)40:1c)4:1d)2:1Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















