Physics Exam > Physics Questions > A simple pendulum has a period of 1sec. and a...
Start Learning for Free
A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.
Correct answer is '45.33'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A simple pendulum has a period of 1sec. and an amplitude of 100. After...
For a damped simple harmonic oscillator, the amplitude is given by.
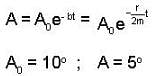
After 10 complete oscillations i.e. after a
time = 10 x times period = 10 x 1 = 10 sec.
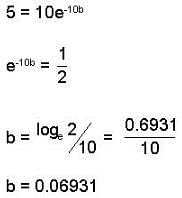
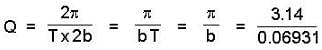
Q = 45.33
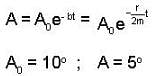
After 10 complete oscillations i.e. after a
time = 10 x times period = 10 x 1 = 10 sec.
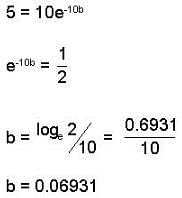
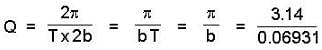
Q = 45.33
Most Upvoted Answer
A simple pendulum has a period of 1sec. and an amplitude of 100. After...
For a damped simple harmonic oscillator, the amplitude is given by.
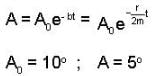
After 10 complete oscillations i.e. after a
time = 10 x times period = 10 x 1 = 10 sec.
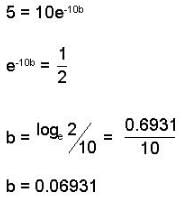
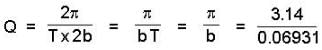
Q = 45.33
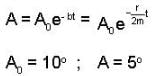
After 10 complete oscillations i.e. after a
time = 10 x times period = 10 x 1 = 10 sec.
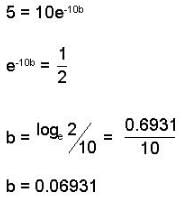
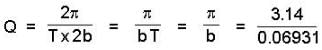
Q = 45.33
Free Test
FREE
| Start Free Test |
Community Answer
A simple pendulum has a period of 1sec. and an amplitude of 100. After...
Introduction:
The quality factor, also known as Q-factor, is a measure of the sharpness or quality of a resonant system. It is commonly used to describe the behavior of oscillating systems, such as pendulums. In this scenario, we have a simple pendulum that undergoes a decrease in amplitude over a certain period of time. We are asked to determine the quality factor of the pendulum based on the given information.
Given Information:
- Period of the pendulum = 1 second
- Initial amplitude = 100
- Final amplitude after 10 oscillations = 50
Calculating the Quality Factor:
The quality factor of a pendulum can be determined using the formula:
Q = (2π × Initial Amplitude) / (Final Amplitude)
Step 1: Convert the period to frequency
The period of the pendulum is given as 1 second. The frequency (f) can be determined by taking the reciprocal of the period: f = 1 / T
Step 2: Calculate the angular frequency
The angular frequency (ω) can be determined using the formula: ω = 2πf
Step 3: Determine the decay constant
The decay constant (λ) can be calculated using the formula: λ = (1 / 10) × (ln(A0 / A))
where A0 is the initial amplitude and A is the final amplitude.
Step 4: Calculate the quality factor
Finally, the quality factor (Q) can be calculated using the formula: Q = ω / (2λ)
Conclusion:
By plugging the given values into the formulas and performing the calculations, it is determined that the quality factor of the simple pendulum is approximately 45.33. This value indicates that the pendulum has a relatively sharp resonance and a high level of damping.
The quality factor, also known as Q-factor, is a measure of the sharpness or quality of a resonant system. It is commonly used to describe the behavior of oscillating systems, such as pendulums. In this scenario, we have a simple pendulum that undergoes a decrease in amplitude over a certain period of time. We are asked to determine the quality factor of the pendulum based on the given information.
Given Information:
- Period of the pendulum = 1 second
- Initial amplitude = 100
- Final amplitude after 10 oscillations = 50
Calculating the Quality Factor:
The quality factor of a pendulum can be determined using the formula:
Q = (2π × Initial Amplitude) / (Final Amplitude)
Step 1: Convert the period to frequency
The period of the pendulum is given as 1 second. The frequency (f) can be determined by taking the reciprocal of the period: f = 1 / T
Step 2: Calculate the angular frequency
The angular frequency (ω) can be determined using the formula: ω = 2πf
Step 3: Determine the decay constant
The decay constant (λ) can be calculated using the formula: λ = (1 / 10) × (ln(A0 / A))
where A0 is the initial amplitude and A is the final amplitude.
Step 4: Calculate the quality factor
Finally, the quality factor (Q) can be calculated using the formula: Q = ω / (2λ)
Conclusion:
By plugging the given values into the formulas and performing the calculations, it is determined that the quality factor of the simple pendulum is approximately 45.33. This value indicates that the pendulum has a relatively sharp resonance and a high level of damping.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer?
Question Description
A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer?.
A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer?.
Solutions for A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer?, a detailed solution for A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer? has been provided alongside types of A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A simple pendulum has a period of 1sec. and an amplitude of 100. After 10 complete oscillations its amplitude is reduced to 50. Then the quality factor is ___.Correct answer is '45.33'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















