Physics Exam > Physics Questions > Find the value of speed (km/sec) with which a...
Start Learning for Free
Find the value of speed (km/sec) with which a projectile should be launched from the surface of the earth so as to reach a height h equal to 1/4th of the radius r of the earth.
Correct answer is '5'. Can you explain this answer?
Verified Answer
Find the value of speed (km/sec) with which a projectile should be lau...
Let m = mass of the earth
m = mass of the projectile

Increase in potential energy =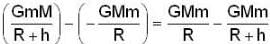
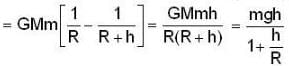
form law of conservation of energy
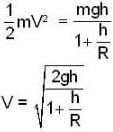
it is given h = R/4
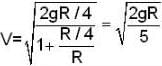
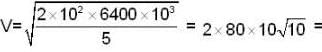 5060m /sec or ≈ 5 km/sec
5060m /sec or ≈ 5 km/sec
m = mass of the projectile

Increase in potential energy =
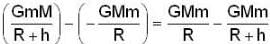
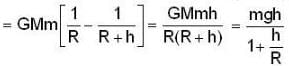
form law of conservation of energy

it is given h = R/4
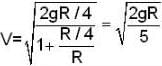
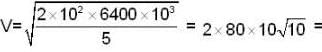 5060m /sec or ≈ 5 km/sec
5060m /sec or ≈ 5 km/secMost Upvoted Answer
Find the value of speed (km/sec) with which a projectile should be lau...
Let m = mass of the earth
m = mass of the projectile

Increase in potential energy =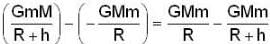
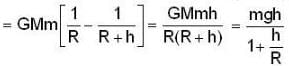
form law of conservation of energy

it is given h = R/4
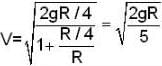
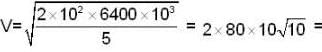 5060m /sec or ≈ 5 km/sec
5060m /sec or ≈ 5 km/sec
m = mass of the projectile

Increase in potential energy =
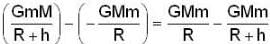
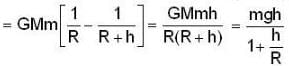
form law of conservation of energy

it is given h = R/4
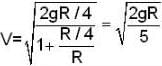
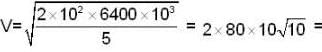 5060m /sec or ≈ 5 km/sec
5060m /sec or ≈ 5 km/secFree Test
FREE
| Start Free Test |
Community Answer
Find the value of speed (km/sec) with which a projectile should be lau...
Problem: Find the value of speed (km/hr) with which a projectile should be launched from the surface of the earth so as to reach a height h equal to 1/4th of the radius r of the earth.
Solution:
To solve this problem, we can use the principles of projectile motion and the gravitational force acting on the projectile.
1. Understanding the Problem:
- We need to find the speed at which the projectile should be launched from the surface of the earth to reach a height h.
- The height h is given as 1/4th of the radius r of the earth.
2. Analyzing the Situation:
- The projectile will follow a curved path due to the gravitational force acting on it.
- At the highest point of its trajectory, the projectile will have zero vertical velocity.
- The time taken to reach the highest point can be determined using the kinematic equation: vf = vi + at, where vf = 0 (final velocity), vi = initial velocity, a = acceleration (due to gravity), and t = time.
- The time taken to reach the highest point will be equal to the time taken to fall back to the surface of the earth.
3. Determining the Time of Flight:
- The time taken to reach the highest point can be calculated using the formula: t = (2 * vi) / g, where g is the acceleration due to gravity.
- The total time of flight will be twice the time taken to reach the highest point (as it takes the same amount of time to fall back to the surface).
- So, the total time of flight will be: T = 2 * [(2 * vi) / g] = (4 * vi) / g.
4. Finding the Required Speed:
- The required speed can be found by considering the vertical motion of the projectile.
- At the highest point, the vertical displacement will be equal to h.
- Using the equation of motion: s = vit + (1/2)at^2, where s = displacement, vi = initial velocity, t = time, and a = acceleration.
- At the highest point, the displacement is h, the initial velocity is vi, and the acceleration is -g (negative due to the direction).
- So, we can write h = vi * t - (1/2)g * t^2.
- Replacing t with (4 * vi) / g (time of flight), we get the equation: h = vi * [(4 * vi) / g] - (1/2)g * [((4 * vi) / g)^2].
- Simplifying the equation, we get: h = (4 * vi^2) / g - (8 * vi^2) / g.
- Rearranging the terms, we get: (8 * vi^2) / g = (4 * vi^2) / g - h.
- Simplifying further, we get: (4 * vi^2) / g = h.
- Rearranging the terms and substituting the value of h as (1/4)r (1/4th of the radius r of the earth), we get: vi^2 = (1/4)gr.
- Now, we can solve for vi (initial velocity) by taking
Solution:
To solve this problem, we can use the principles of projectile motion and the gravitational force acting on the projectile.
1. Understanding the Problem:
- We need to find the speed at which the projectile should be launched from the surface of the earth to reach a height h.
- The height h is given as 1/4th of the radius r of the earth.
2. Analyzing the Situation:
- The projectile will follow a curved path due to the gravitational force acting on it.
- At the highest point of its trajectory, the projectile will have zero vertical velocity.
- The time taken to reach the highest point can be determined using the kinematic equation: vf = vi + at, where vf = 0 (final velocity), vi = initial velocity, a = acceleration (due to gravity), and t = time.
- The time taken to reach the highest point will be equal to the time taken to fall back to the surface of the earth.
3. Determining the Time of Flight:
- The time taken to reach the highest point can be calculated using the formula: t = (2 * vi) / g, where g is the acceleration due to gravity.
- The total time of flight will be twice the time taken to reach the highest point (as it takes the same amount of time to fall back to the surface).
- So, the total time of flight will be: T = 2 * [(2 * vi) / g] = (4 * vi) / g.
4. Finding the Required Speed:
- The required speed can be found by considering the vertical motion of the projectile.
- At the highest point, the vertical displacement will be equal to h.
- Using the equation of motion: s = vit + (1/2)at^2, where s = displacement, vi = initial velocity, t = time, and a = acceleration.
- At the highest point, the displacement is h, the initial velocity is vi, and the acceleration is -g (negative due to the direction).
- So, we can write h = vi * t - (1/2)g * t^2.
- Replacing t with (4 * vi) / g (time of flight), we get the equation: h = vi * [(4 * vi) / g] - (1/2)g * [((4 * vi) / g)^2].
- Simplifying the equation, we get: h = (4 * vi^2) / g - (8 * vi^2) / g.
- Rearranging the terms, we get: (8 * vi^2) / g = (4 * vi^2) / g - h.
- Simplifying further, we get: (4 * vi^2) / g = h.
- Rearranging the terms and substituting the value of h as (1/4)r (1/4th of the radius r of the earth), we get: vi^2 = (1/4)gr.
- Now, we can solve for vi (initial velocity) by taking

|
Explore Courses for Physics exam
|

|
Question Description
Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer?.
Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer?.
Solutions for Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer?, a detailed solution for Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer? has been provided alongside types of Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Find the value of speed (km/sec) with which a projectile should be launched from thesurface of the earth so as to reach a height h equal to 1/4thof the radius r of theearth.Correct answer is '5'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















