Mathematics Exam > Mathematics Questions > Which of the following statements is correct?...
Start Learning for Free
Which of the following statements is correct?
- a)No two differential equations can have the same integrating factor
- b)Infinitely many differential equations can have the same integrating factor
- c)Only a finite number of differential equations can have the same integrating factor
- d)None of the above
Correct answer is option 'B'. Can you explain this answer?
Most Upvoted Answer
Which of the following statements is correct?a)No two differential equ...
The infinite number of differential equations given by
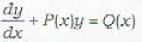
for infinitely many different choices of Q(x), possess the same integrating factor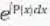
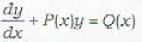
for infinitely many different choices of Q(x), possess the same integrating factor
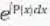
Free Test
FREE
| Start Free Test |
Community Answer
Which of the following statements is correct?a)No two differential equ...
Explanation:
In order to understand why option B is correct, we need to understand the concept of integrating factors in differential equations.
Differential Equations:
Differential equations are mathematical equations that involve an unknown function and its derivatives. They are used to model various phenomena in physics, engineering, and other fields.
Integrating Factors:
Integrating factors are used to solve certain types of differential equations. They are functions that multiply the entire equation, thereby making it easier to integrate. The choice of integrating factor depends on the type of differential equation being solved.
Option A:
The statement "No two differential equations can have the same integrating factor" is incorrect. It is possible for two different differential equations to have the same integrating factor. The integrating factor is determined by the characteristics of the equation itself, not by the specific values or parameters in the equation.
Option B:
The statement "Infinitely many differential equations can have the same integrating factor" is correct. Different differential equations can have the same integrating factor if they share certain characteristics. For example, if two differential equations have the same form or structure, they can often be solved using the same integrating factor.
Option C:
The statement "Only a finite number of differential equations can have the same integrating factor" is incorrect. There is no limit to the number of differential equations that can have the same integrating factor. As mentioned earlier, the choice of integrating factor depends on the characteristics of the equation, not on the number of equations.
Conclusion:
Based on the explanation above, we can conclude that option B is the correct statement. Infinitely many differential equations can have the same integrating factor if they share certain characteristics or form.
In order to understand why option B is correct, we need to understand the concept of integrating factors in differential equations.
Differential Equations:
Differential equations are mathematical equations that involve an unknown function and its derivatives. They are used to model various phenomena in physics, engineering, and other fields.
Integrating Factors:
Integrating factors are used to solve certain types of differential equations. They are functions that multiply the entire equation, thereby making it easier to integrate. The choice of integrating factor depends on the type of differential equation being solved.
Option A:
The statement "No two differential equations can have the same integrating factor" is incorrect. It is possible for two different differential equations to have the same integrating factor. The integrating factor is determined by the characteristics of the equation itself, not by the specific values or parameters in the equation.
Option B:
The statement "Infinitely many differential equations can have the same integrating factor" is correct. Different differential equations can have the same integrating factor if they share certain characteristics. For example, if two differential equations have the same form or structure, they can often be solved using the same integrating factor.
Option C:
The statement "Only a finite number of differential equations can have the same integrating factor" is incorrect. There is no limit to the number of differential equations that can have the same integrating factor. As mentioned earlier, the choice of integrating factor depends on the characteristics of the equation, not on the number of equations.
Conclusion:
Based on the explanation above, we can conclude that option B is the correct statement. Infinitely many differential equations can have the same integrating factor if they share certain characteristics or form.

|
Explore Courses for Mathematics exam
|

|
Similar Mathematics Doubts
Question Description
Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer? for Mathematics 2025 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Mathematics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer?.
Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer? for Mathematics 2025 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Mathematics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer?.
Solutions for Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mathematics.
Download more important topics, notes, lectures and mock test series for Mathematics Exam by signing up for free.
Here you can find the meaning of Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Which of the following statements is correct?a)No two differential equations can have the same integrating factorb)Infinitely many differential equations can have the same integrating factorc)Only a finite number of differential equations can have the same integrating factord)None of the aboveCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Mathematics tests.

|
Explore Courses for Mathematics exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















