Mathematics Exam > Mathematics Questions > The volume of the solid generated by the revo...
Start Learning for Free
The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)
Correct answer is '19.74'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The volume of the solid generated by the revolution of the cissoid abo...
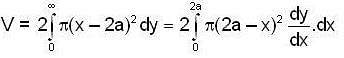
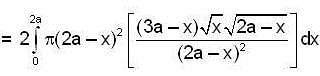
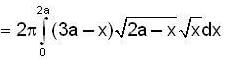
Put x = 2a sin2θ then
d x = 4 a sin θcosθdθ
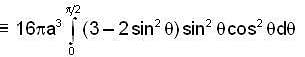
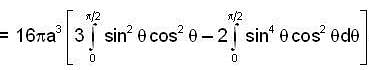
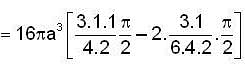
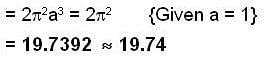
Most Upvoted Answer
The volume of the solid generated by the revolution of the cissoid abo...
The Cissoid
The given equation represents a curve known as the cissoid. The cissoid is a plane curve defined by a certain equation. In this case, the equation of the cissoid is y^2(2a - x) = x^3, where a is a given constant.
Revolution of the Cissoid
We are asked to find the volume of the solid generated by the revolution of the cissoid about the line x = 2a. To do this, we need to use the method of cylindrical shells.
Method of Cylindrical Shells
The method of cylindrical shells involves finding the volume of a solid by integrating the volumes of infinitely thin cylindrical shells.
To apply this method, we need to consider an infinitesimally small strip of width dx along the x-axis. This strip will have a corresponding height, which is given by the y-coordinate of the cissoid curve.
Setting up the Integral
To find the volume of the solid, we need to integrate the volumes of all these cylindrical shells from x = 0 to x = 2a.
The volume of an individual cylindrical shell is given by the formula: V = 2πy * h * dx, where y is the y-coordinate of the cissoid curve and h is the height of the cylindrical shell.
To find y in terms of x, we rearrange the equation of the cissoid: y^2(2a - x) = x^3. Solving for y, we get: y = (x^3) / sqrt(2a - x).
Integrating the Volume
Now we can set up the integral to find the volume of the solid:
V = ∫[0 to 2a] (2πy * h * dx) = ∫[0 to 2a] 2π * (x^3) / sqrt(2a - x) * dx.
To evaluate this integral, we can make a substitution: u = 2a - x. This gives us du = -dx, and we can rewrite the integral as:
V = ∫[2a to 0] -2π * (u^3) / sqrt(u) * (-du) = 2π * ∫[0 to 2a] (u^3) / sqrt(u) * du.
Simplifying the integrand, we get: V = 2π * ∫[0 to 2a] u^(7/2) * du.
Integrating this expression, we get: V = 2π * [2/9 * u^(9/2)] [0 to 2a] = 4π * (2/9 * (2a)^(9/2)).
Substituting the Value of a
Given that a = 1, we can substitute this value into the expression for the volume:
V = 4π * (2/9 * (2(1))^(9/2)) = 4π * (2/9 * 2^(9/2)) = 8π/9 * 2^(9/2).
Calculating this value, we get V ≈ 19.7392.
Final Answer
The given equation represents a curve known as the cissoid. The cissoid is a plane curve defined by a certain equation. In this case, the equation of the cissoid is y^2(2a - x) = x^3, where a is a given constant.
Revolution of the Cissoid
We are asked to find the volume of the solid generated by the revolution of the cissoid about the line x = 2a. To do this, we need to use the method of cylindrical shells.
Method of Cylindrical Shells
The method of cylindrical shells involves finding the volume of a solid by integrating the volumes of infinitely thin cylindrical shells.
To apply this method, we need to consider an infinitesimally small strip of width dx along the x-axis. This strip will have a corresponding height, which is given by the y-coordinate of the cissoid curve.
Setting up the Integral
To find the volume of the solid, we need to integrate the volumes of all these cylindrical shells from x = 0 to x = 2a.
The volume of an individual cylindrical shell is given by the formula: V = 2πy * h * dx, where y is the y-coordinate of the cissoid curve and h is the height of the cylindrical shell.
To find y in terms of x, we rearrange the equation of the cissoid: y^2(2a - x) = x^3. Solving for y, we get: y = (x^3) / sqrt(2a - x).
Integrating the Volume
Now we can set up the integral to find the volume of the solid:
V = ∫[0 to 2a] (2πy * h * dx) = ∫[0 to 2a] 2π * (x^3) / sqrt(2a - x) * dx.
To evaluate this integral, we can make a substitution: u = 2a - x. This gives us du = -dx, and we can rewrite the integral as:
V = ∫[2a to 0] -2π * (u^3) / sqrt(u) * (-du) = 2π * ∫[0 to 2a] (u^3) / sqrt(u) * du.
Simplifying the integrand, we get: V = 2π * ∫[0 to 2a] u^(7/2) * du.
Integrating this expression, we get: V = 2π * [2/9 * u^(9/2)] [0 to 2a] = 4π * (2/9 * (2a)^(9/2)).
Substituting the Value of a
Given that a = 1, we can substitute this value into the expression for the volume:
V = 4π * (2/9 * (2(1))^(9/2)) = 4π * (2/9 * 2^(9/2)) = 8π/9 * 2^(9/2).
Calculating this value, we get V ≈ 19.7392.
Final Answer

|
Explore Courses for Mathematics exam
|

|
Similar Mathematics Doubts
The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer?
Question Description
The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer? for Mathematics 2024 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer? covers all topics & solutions for Mathematics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer?.
The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer? for Mathematics 2024 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer? covers all topics & solutions for Mathematics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer?.
Solutions for The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mathematics.
Download more important topics, notes, lectures and mock test series for Mathematics Exam by signing up for free.
Here you can find the meaning of The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer?, a detailed solution for The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer? has been provided alongside types of The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The volume of the solid generated by the revolution of the cissoid about the line x = 2a where equation o f the cissoid is y2(2a - x) = x3 {if a = 1} i s ______ . (correct upto two decimal places)Correct answer is '19.74'. Can you explain this answer? tests, examples and also practice Mathematics tests.

|
Explore Courses for Mathematics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















