Physics Exam > Physics Questions > An amplifier of gain 1000 is made into a feed...
Start Learning for Free
An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and f
h
= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer?
Most Upvoted Answer
An amplifier of gain 1000 is made into a feedback amplifier by feeding...
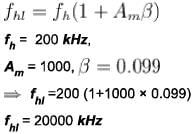
The correct answer is: 20000
Free Test
FREE
| Start Free Test |
Community Answer
An amplifier of gain 1000 is made into a feedback amplifier by feeding...
Introduction:
In a feedback amplifier, a portion of the output voltage is fed back to the input in a manner that opposes the input signal. This is done to stabilize the amplifier's gain and other characteristics. In this particular case, an amplifier with a gain of 1000 is converted into a feedback amplifier by feeding 9.9% of its output voltage in series with the input signal.
Given Information:
- Amplifier gain without feedback (A) = 1000
- Feedback fraction (β) = 9.9% = 0.099
- Lower cutoff frequency (fl) = 20Hz
- Upper cutoff frequency (fh) = 200kHz
Determination of New Frequency:
The introduction of feedback affects the overall frequency response of the amplifier. To determine the new frequency, we need to consider the effect of feedback on the amplifier's bandwidth.
Effect of Feedback on Bandwidth:
Feedback reduces the bandwidth of the amplifier by a factor of (1 + Aβ). The bandwidth with feedback (Bf) can be calculated using the formula:
Bf = B / (1 + Aβ)
Where B is the bandwidth without feedback.
Calculation of New Frequency:
In this case, the upper cutoff frequency without feedback (fh) is given as 200kHz. Let's assume the new frequency with feedback is f'.
Using the formula for bandwidth with feedback, we can write:
Bf = fh - fl
Substituting the given values:
fh - fl = (fh' - fl) / (1 + Aβ)
Since the lower cutoff frequency (fl) remains the same, we can simplify the equation:
fh - fl = fh' / (1 + Aβ)
Rearranging the equation:
fh' = (fh - fl) * (1 + Aβ)
Substituting the given values:
fh' = (200kHz - 20Hz) * (1 + 1000 * 0.099)
Calculating the expression:
fh' = 198kHz * 100.99
fh' ≈ 19800kHz
Converting kHz to Hz:
fh' ≈ 19800 * 1000Hz
fh' ≈ 19800000Hz
Therefore, the new frequency with feedback is approximately 19800000Hz, which is equivalent to 19800kHz or 20000kHz when rounded to the nearest kilohertz.
In a feedback amplifier, a portion of the output voltage is fed back to the input in a manner that opposes the input signal. This is done to stabilize the amplifier's gain and other characteristics. In this particular case, an amplifier with a gain of 1000 is converted into a feedback amplifier by feeding 9.9% of its output voltage in series with the input signal.
Given Information:
- Amplifier gain without feedback (A) = 1000
- Feedback fraction (β) = 9.9% = 0.099
- Lower cutoff frequency (fl) = 20Hz
- Upper cutoff frequency (fh) = 200kHz
Determination of New Frequency:
The introduction of feedback affects the overall frequency response of the amplifier. To determine the new frequency, we need to consider the effect of feedback on the amplifier's bandwidth.
Effect of Feedback on Bandwidth:
Feedback reduces the bandwidth of the amplifier by a factor of (1 + Aβ). The bandwidth with feedback (Bf) can be calculated using the formula:
Bf = B / (1 + Aβ)
Where B is the bandwidth without feedback.
Calculation of New Frequency:
In this case, the upper cutoff frequency without feedback (fh) is given as 200kHz. Let's assume the new frequency with feedback is f'.
Using the formula for bandwidth with feedback, we can write:
Bf = fh - fl
Substituting the given values:
fh - fl = (fh' - fl) / (1 + Aβ)
Since the lower cutoff frequency (fl) remains the same, we can simplify the equation:
fh - fl = fh' / (1 + Aβ)
Rearranging the equation:
fh' = (fh - fl) * (1 + Aβ)
Substituting the given values:
fh' = (200kHz - 20Hz) * (1 + 1000 * 0.099)
Calculating the expression:
fh' = 198kHz * 100.99
fh' ≈ 19800kHz
Converting kHz to Hz:
fh' ≈ 19800 * 1000Hz
fh' ≈ 19800000Hz
Therefore, the new frequency with feedback is approximately 19800000Hz, which is equivalent to 19800kHz or 20000kHz when rounded to the nearest kilohertz.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer?
Question Description
An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer?.
An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer? for Physics 2025 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer? covers all topics & solutions for Physics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer?.
Solutions for An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer?, a detailed solution for An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer? has been provided alongside types of An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice An amplifier of gain 1000 is made into a feedback amplifier by feeding 9.9% of its output voltage in series with the input opposing. If fl = 20Hz and fh= 200kHz for the amplifier without feedback then due to the feedback what is the new frequency (in kHz)?Correct answer is '20000'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















