Physics Exam > Physics Questions > The ratio of magnetic inductions at the centr...
Start Learning for Free
The ratio of magnetic inductions at the centre of a circular coil of radius a and on its axis at a distance equal to its radius, will be :
Correct answer is '2.828'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The ratio of magnetic inductions at the centre of a circular coil of r...
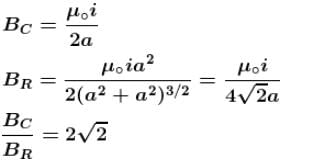
The correct answer is: 2.828
Most Upvoted Answer
The ratio of magnetic inductions at the centre of a circular coil of r...
The ratio of magnetic inductions at the center of a circular coil and on its axis at a distance equal to its radius can be determined using the Biot-Savart law and the formula for magnetic field due to a current-carrying loop.
1. Magnetic Field at the Center of a Circular Coil:
- The magnetic field at the center of a circular coil can be determined by considering the contributions from each small element of the coil.
- According to the Biot-Savart law, the magnetic field at a point due to a current element is directly proportional to the product of the current element, the sine of the angle between the current element and the line connecting the point and the current element, and inversely proportional to the square of the distance between the point and the current element.
- For a circular coil, the current elements are perpendicular to the line connecting the center of the coil and the point at its center, so the angle is 90 degrees and the sine of the angle is 1.
- The distance between the center of the coil and the point at its center is zero, so the magnetic field at the center of the coil is given by the formula: B_center = μ₀I/2R, where μ₀ is the permeability of free space, I is the current flowing through the coil, and R is the radius of the coil.
2. Magnetic Field on the Axis of the Coil at a Distance Equal to its Radius:
- The magnetic field on the axis of the coil at a distance equal to its radius can also be determined using the Biot-Savart law.
- In this case, the angle between the current element and the line connecting the point on the axis and the current element varies along the axis.
- The magnetic field contributions from each current element have both a vertical and horizontal component, but only the vertical component contributes to the magnetic field on the axis.
- By integrating the contributions from all the current elements, the magnetic field on the axis can be found to be: B_axis = μ₀IR/(2√(2)R²) = μ₀I/(2√(2)R).
3. Ratio of Magnetic Inductions:
- Now, we can calculate the ratio of magnetic inductions at the center of the coil and on its axis at a distance equal to its radius.
- B_center/B_axis = (μ₀I/2R)/(μ₀I/(2√(2)R)) = √(2)R/R = √(2) = 2.828.
- Therefore, the correct answer is 2.828.
1. Magnetic Field at the Center of a Circular Coil:
- The magnetic field at the center of a circular coil can be determined by considering the contributions from each small element of the coil.
- According to the Biot-Savart law, the magnetic field at a point due to a current element is directly proportional to the product of the current element, the sine of the angle between the current element and the line connecting the point and the current element, and inversely proportional to the square of the distance between the point and the current element.
- For a circular coil, the current elements are perpendicular to the line connecting the center of the coil and the point at its center, so the angle is 90 degrees and the sine of the angle is 1.
- The distance between the center of the coil and the point at its center is zero, so the magnetic field at the center of the coil is given by the formula: B_center = μ₀I/2R, where μ₀ is the permeability of free space, I is the current flowing through the coil, and R is the radius of the coil.
2. Magnetic Field on the Axis of the Coil at a Distance Equal to its Radius:
- The magnetic field on the axis of the coil at a distance equal to its radius can also be determined using the Biot-Savart law.
- In this case, the angle between the current element and the line connecting the point on the axis and the current element varies along the axis.
- The magnetic field contributions from each current element have both a vertical and horizontal component, but only the vertical component contributes to the magnetic field on the axis.
- By integrating the contributions from all the current elements, the magnetic field on the axis can be found to be: B_axis = μ₀IR/(2√(2)R²) = μ₀I/(2√(2)R).
3. Ratio of Magnetic Inductions:
- Now, we can calculate the ratio of magnetic inductions at the center of the coil and on its axis at a distance equal to its radius.
- B_center/B_axis = (μ₀I/2R)/(μ₀I/(2√(2)R)) = √(2)R/R = √(2) = 2.828.
- Therefore, the correct answer is 2.828.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer?
Question Description
The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer?.
The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer?.
Solutions for The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer?, a detailed solution for The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer? has been provided alongside types of The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The ratio of magnetic inductions at the centre of a circular coil of radiusa and on its axis at a distance equal to its radius, will be :Correct answer is '2.828'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















