UPSC Exam > UPSC Questions > The number of parallelograms that can be form...
Start Learning for Free
The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, is
- a)18
- b)24
- c)32
- d)36
Correct answer is option 'D'. Can you explain this answer?
Verified Answer
The number of parallelograms that can be formed from a set of four par...
In the diagram, let’s count the parallelograms one by one.
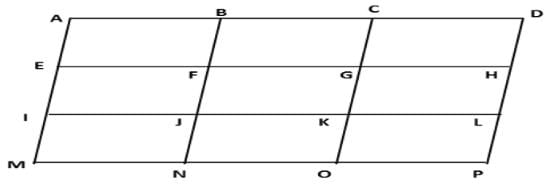
Case I - Parallelograms of 1 × 1 (ABFE type) - ABFE, BCGF, CDHG, EFJI, FGKJ, GHLK, IJNM, JKON, KLPO – total 9.
Case II - Parallelograms of 1 × 2 (ACGE type) - ACGE, BDFH, EGKI, FHLJ, IKOM, JLPN – total 6.
Case III - Parallelogram of 2 × 1 (ABJI type) - ABJI, EFNM, BCKJ, FGON, CDLK, GHPO – total 6.
Case IV - Parallelograms of 1 × 3 (ADHE type) - ADHE, EHLI, ILPM – total 3.
Case V - Parallelograms of 3 × 1 (ABNM type) - ABNM, BCON, CDPO – total 3.
Case VI - Parallelograms of 2 × 2 (ACKI type) - ACKI, BDLJ, EGOM, FHPN – total 4.
Case VII - Parallelograms of 3 × 2 (ADLI type) - ADLI, EHPM – total 2.
Case VIII - Parallelograms of 2 × 3 (ACOM type) - ACOM, BDPN – total 2.
Case IX -Parallelograms of 3 × 3 (ADPM type) – ADPM – total 1.
Total 36.
A much shorter method is by using permutations and combinations.
Select any two of the first set of 4 lines. That can be done in 4C2 ways.
Now select any two of the second set of 4 lines. That can also be done in 4C2 ways.
So the total number of ways of doing it = 4C2 x 4C2 = 6 x 6 = 36 ways.
Most Upvoted Answer
The number of parallelograms that can be formed from a set of four par...
Number of Parallelograms Formed by Intersecting Parallel Lines:
To find the number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, we can follow a step-by-step approach.
Step 1: Understand the Problem
We are given two sets of parallel lines, each containing four lines. We need to determine the number of parallelograms that can be formed by selecting four lines, two from each set, such that they form a parallelogram.
Step 2: Identify the Conditions for a Parallelogram
In order for four lines to form a parallelogram, they must satisfy the following conditions:
- Opposite sides are parallel.
- Opposite sides are equal in length.
- Opposite angles are equal in measure.
Step 3: Count the Possible Options
Let's consider the two sets of parallel lines as A and B, with four lines each. We need to select two lines from set A and two lines from set B to form a parallelogram.
Case 1: Selecting Two Adjacent Lines from Each Set
In this case, we can select two adjacent lines from set A and two adjacent lines from set B. There are four ways to select two adjacent lines from set A and four ways to select two adjacent lines from set B. Therefore, the total number of options in this case is 4 * 4 = 16.
Case 2: Selecting One Line from Each Set
In this case, we can select one line from set A and one line from set B. There are four ways to select one line from set A and four ways to select one line from set B. Therefore, the total number of options in this case is 4 * 4 = 16.
Step 4: Total Number of Parallelograms
To find the total number of parallelograms, we need to add the options from both cases. Therefore, the total number of parallelograms that can be formed is 16 + 16 = 32.
Conclusion:
The correct answer is option C) 32.
To find the number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, we can follow a step-by-step approach.
Step 1: Understand the Problem
We are given two sets of parallel lines, each containing four lines. We need to determine the number of parallelograms that can be formed by selecting four lines, two from each set, such that they form a parallelogram.
Step 2: Identify the Conditions for a Parallelogram
In order for four lines to form a parallelogram, they must satisfy the following conditions:
- Opposite sides are parallel.
- Opposite sides are equal in length.
- Opposite angles are equal in measure.
Step 3: Count the Possible Options
Let's consider the two sets of parallel lines as A and B, with four lines each. We need to select two lines from set A and two lines from set B to form a parallelogram.
Case 1: Selecting Two Adjacent Lines from Each Set
In this case, we can select two adjacent lines from set A and two adjacent lines from set B. There are four ways to select two adjacent lines from set A and four ways to select two adjacent lines from set B. Therefore, the total number of options in this case is 4 * 4 = 16.
Case 2: Selecting One Line from Each Set
In this case, we can select one line from set A and one line from set B. There are four ways to select one line from set A and four ways to select one line from set B. Therefore, the total number of options in this case is 4 * 4 = 16.
Step 4: Total Number of Parallelograms
To find the total number of parallelograms, we need to add the options from both cases. Therefore, the total number of parallelograms that can be formed is 16 + 16 = 32.
Conclusion:
The correct answer is option C) 32.

|
Explore Courses for UPSC exam
|

|
Similar UPSC Doubts
Question Description
The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer? for UPSC 2025 is part of UPSC preparation. The Question and answers have been prepared according to the UPSC exam syllabus. Information about The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer? covers all topics & solutions for UPSC 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer?.
The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer? for UPSC 2025 is part of UPSC preparation. The Question and answers have been prepared according to the UPSC exam syllabus. Information about The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer? covers all topics & solutions for UPSC 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer?.
Solutions for The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for UPSC.
Download more important topics, notes, lectures and mock test series for UPSC Exam by signing up for free.
Here you can find the meaning of The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer?, a detailed solution for The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer? has been provided alongside types of The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of four parallel lines, isa)18b)24c)32d)36Correct answer is option 'D'. Can you explain this answer? tests, examples and also practice UPSC tests.

|
Explore Courses for UPSC exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















