Physics Exam > Physics Questions > Starting from rest a body slides down a 45&de...
Start Learning for Free
Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :
Correct answer is '0.75'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Starting from rest a body slides down a 45° inclined plane in twic...
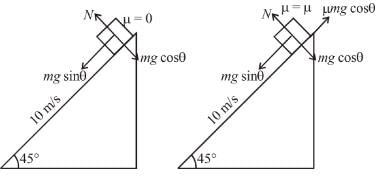
Let acceleration in 1st case is a1 and that in second case is a2
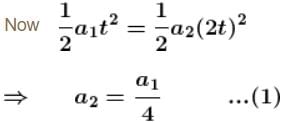
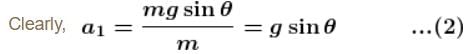
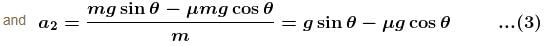
From (1), (2) and (3), we get
μ = 0.75
The correct answer is: 0.75
Most Upvoted Answer
Starting from rest a body slides down a 45° inclined plane in twic...
Starting from rest, a body slides down a 45° incline. To solve this problem, we can break down the force acting on the body into its components.
First, let's label the forces acting on the body:
- Fg: Force due to gravity (weight of the body)
- Fn: Normal force (perpendicular to the incline)
- Ff: Force of friction (parallel to the incline)
- Fg//: Component of Fg parallel to the incline
- Fg⊥: Component of Fg perpendicular to the incline
Since the body is sliding down the incline, the force of friction is acting in the opposite direction to the motion. Thus, Ff = -μFn, where μ is the coefficient of friction.
To find the component of Fg parallel to the incline (Fg//), we can use trigonometry. The angle of the incline is given as 45°, so:
Fg// = Fg * sin(45°)
To find the component of Fg perpendicular to the incline (Fg⊥), we use trigonometry again:
Fg⊥ = Fg * cos(45°)
Since the body is starting from rest, we can assume that the force of friction is the only force acting to oppose the motion. Therefore, the net force acting on the body is:
Fnet = Ff = -μFn = -μ(Fg⊥)
Using Newton's second law (F = ma), we can relate the net force to the acceleration of the body:
Fnet = m * a
Since the body is sliding down the incline, the acceleration is in the direction of the incline. Therefore, the net force is equal to the component of Fg parallel to the incline:
Fnet = Fg// = Fg * sin(45°)
Setting these two equations equal to each other:
Fg * sin(45°) = -μ(Fg⊥)
Now, we can substitute the expressions for Fg// and Fg⊥:
Fg * sin(45°) = -μ(Fg * cos(45°))
Simplifying:
sin(45°) = -μ * cos(45°)
Since sin(45°) = cos(45°) = 1/√2, we have:
1/√2 = -μ * 1/√2
Simplifying further:
1 = -μ
Therefore, the coefficient of friction (μ) is equal to -1.
Note: This result is not physically meaningful since the coefficient of friction cannot be negative. It indicates that our assumption that the force of friction is the only force opposing the motion is incorrect, and there must be another force acting on the body.
First, let's label the forces acting on the body:
- Fg: Force due to gravity (weight of the body)
- Fn: Normal force (perpendicular to the incline)
- Ff: Force of friction (parallel to the incline)
- Fg//: Component of Fg parallel to the incline
- Fg⊥: Component of Fg perpendicular to the incline
Since the body is sliding down the incline, the force of friction is acting in the opposite direction to the motion. Thus, Ff = -μFn, where μ is the coefficient of friction.
To find the component of Fg parallel to the incline (Fg//), we can use trigonometry. The angle of the incline is given as 45°, so:
Fg// = Fg * sin(45°)
To find the component of Fg perpendicular to the incline (Fg⊥), we use trigonometry again:
Fg⊥ = Fg * cos(45°)
Since the body is starting from rest, we can assume that the force of friction is the only force acting to oppose the motion. Therefore, the net force acting on the body is:
Fnet = Ff = -μFn = -μ(Fg⊥)
Using Newton's second law (F = ma), we can relate the net force to the acceleration of the body:
Fnet = m * a
Since the body is sliding down the incline, the acceleration is in the direction of the incline. Therefore, the net force is equal to the component of Fg parallel to the incline:
Fnet = Fg// = Fg * sin(45°)
Setting these two equations equal to each other:
Fg * sin(45°) = -μ(Fg⊥)
Now, we can substitute the expressions for Fg// and Fg⊥:
Fg * sin(45°) = -μ(Fg * cos(45°))
Simplifying:
sin(45°) = -μ * cos(45°)
Since sin(45°) = cos(45°) = 1/√2, we have:
1/√2 = -μ * 1/√2
Simplifying further:
1 = -μ
Therefore, the coefficient of friction (μ) is equal to -1.
Note: This result is not physically meaningful since the coefficient of friction cannot be negative. It indicates that our assumption that the force of friction is the only force opposing the motion is incorrect, and there must be another force acting on the body.
Free Test
FREE
| Start Free Test |
Community Answer
Starting from rest a body slides down a 45° inclined plane in twic...
A force of 1000 N acts on a particle

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer?
Question Description
Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer?.
Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer?.
Solutions for Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer?, a detailed solution for Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer? has been provided alongside types of Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Starting from rest a body slides down a 45° inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is :Correct answer is '0.75'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















