Class 11 Exam > Class 11 Questions > System of particles and rotational motion ( q...
Start Learning for Free
System of particles and rotational motion ( quick revision formula )?
Most Upvoted Answer
System of particles and rotational motion ( quick revision formula )?
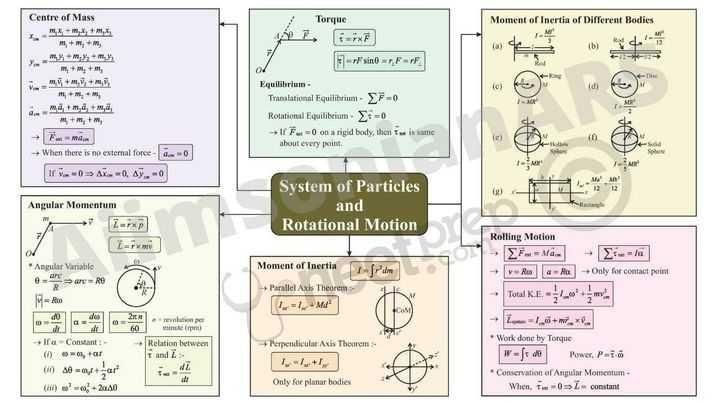
Community Answer
System of particles and rotational motion ( quick revision formula )?
**System of Particles and Rotational Motion: Quick Revision Formula**
**1. Introduction to System of Particles and Rotational Motion:**
The branch of physics that deals with the motion of a system of particles and the rotational motion of rigid bodies is known as system of particles and rotational motion. This topic is crucial in understanding the dynamics of objects in rotational motion.
**2. Center of Mass:**
- The center of mass of a system of particles is the point that represents the average position of the particles in the system.
- The center of mass can be calculated using the formula:
Xcm = (m₁x₁ + m₂x₂ + m₃x₃ + ... + mₙxₙ) / (m₁ + m₂ + m₃ + ... + mₙ),
where m₁, m₂, m₃, ..., mₙ are the masses of the particles and x₁, x₂, x₃, ..., xₙ are their respective positions.
**3. Moment of Inertia:**
- The moment of inertia is a measure of an object's resistance to rotational motion around a given axis.
- The moment of inertia of a point mass is given by the formula: I = m*r², where m is the mass and r is the distance from the axis of rotation.
- The moment of inertia of a rigid body can be calculated by integrating the moment of inertia of infinitesimally small mass elements.
- The moment of inertia depends on both the mass distribution and the axis of rotation.
**4. Torque:**
- Torque is the rotational equivalent of force. It is the measure of the tendency of a force to rotate an object about an axis.
- Torque is given by the formula: τ = r x F, where r is the position vector and F is the force applied.
- The torque can also be calculated as the product of force and perpendicular distance from the axis of rotation: τ = r * F * sin(θ).
**5. Angular Momentum:**
- Angular momentum is a vector quantity that measures the rotational motion of an object.
- Angular momentum is given by the formula: L = I * ω, where I is the moment of inertia and ω is the angular velocity.
- Angular momentum is conserved in the absence of external torques.
**6. Kinematics of Rotational Motion:**
- Angular displacement (θ) is given by the formula: θ = ω₀t + 0.5αt², where ω₀ is the initial angular velocity, α is the angular acceleration, and t is the time.
- Angular velocity (ω) is given by the formula: ω = ω₀ + αt.
- Angular acceleration (α) is given by the formula: α = (ω - ω₀) / t.
- Tangential velocity (v) is given by the formula: v = r * ω, where r is the distance from the axis of rotation.
- Centripetal acceleration (a) is given by the formula: a = r * α, where r is the distance from the axis of rotation.
These are some of the key formulas and concepts related to the system of particles and rotational motion. Understanding and applying these formulas will help in solving problems and analyzing the motion of objects undergoing rotational motion.
**1. Introduction to System of Particles and Rotational Motion:**
The branch of physics that deals with the motion of a system of particles and the rotational motion of rigid bodies is known as system of particles and rotational motion. This topic is crucial in understanding the dynamics of objects in rotational motion.
**2. Center of Mass:**
- The center of mass of a system of particles is the point that represents the average position of the particles in the system.
- The center of mass can be calculated using the formula:
Xcm = (m₁x₁ + m₂x₂ + m₃x₃ + ... + mₙxₙ) / (m₁ + m₂ + m₃ + ... + mₙ),
where m₁, m₂, m₃, ..., mₙ are the masses of the particles and x₁, x₂, x₃, ..., xₙ are their respective positions.
**3. Moment of Inertia:**
- The moment of inertia is a measure of an object's resistance to rotational motion around a given axis.
- The moment of inertia of a point mass is given by the formula: I = m*r², where m is the mass and r is the distance from the axis of rotation.
- The moment of inertia of a rigid body can be calculated by integrating the moment of inertia of infinitesimally small mass elements.
- The moment of inertia depends on both the mass distribution and the axis of rotation.
**4. Torque:**
- Torque is the rotational equivalent of force. It is the measure of the tendency of a force to rotate an object about an axis.
- Torque is given by the formula: τ = r x F, where r is the position vector and F is the force applied.
- The torque can also be calculated as the product of force and perpendicular distance from the axis of rotation: τ = r * F * sin(θ).
**5. Angular Momentum:**
- Angular momentum is a vector quantity that measures the rotational motion of an object.
- Angular momentum is given by the formula: L = I * ω, where I is the moment of inertia and ω is the angular velocity.
- Angular momentum is conserved in the absence of external torques.
**6. Kinematics of Rotational Motion:**
- Angular displacement (θ) is given by the formula: θ = ω₀t + 0.5αt², where ω₀ is the initial angular velocity, α is the angular acceleration, and t is the time.
- Angular velocity (ω) is given by the formula: ω = ω₀ + αt.
- Angular acceleration (α) is given by the formula: α = (ω - ω₀) / t.
- Tangential velocity (v) is given by the formula: v = r * ω, where r is the distance from the axis of rotation.
- Centripetal acceleration (a) is given by the formula: a = r * α, where r is the distance from the axis of rotation.
These are some of the key formulas and concepts related to the system of particles and rotational motion. Understanding and applying these formulas will help in solving problems and analyzing the motion of objects undergoing rotational motion.

|
Explore Courses for Class 11 exam
|

|
Question Description
System of particles and rotational motion ( quick revision formula )? for Class 11 2025 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about System of particles and rotational motion ( quick revision formula )? covers all topics & solutions for Class 11 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for System of particles and rotational motion ( quick revision formula )?.
System of particles and rotational motion ( quick revision formula )? for Class 11 2025 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about System of particles and rotational motion ( quick revision formula )? covers all topics & solutions for Class 11 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for System of particles and rotational motion ( quick revision formula )?.
Solutions for System of particles and rotational motion ( quick revision formula )? in English & in Hindi are available as part of our courses for Class 11.
Download more important topics, notes, lectures and mock test series for Class 11 Exam by signing up for free.
Here you can find the meaning of System of particles and rotational motion ( quick revision formula )? defined & explained in the simplest way possible. Besides giving the explanation of
System of particles and rotational motion ( quick revision formula )?, a detailed solution for System of particles and rotational motion ( quick revision formula )? has been provided alongside types of System of particles and rotational motion ( quick revision formula )? theory, EduRev gives you an
ample number of questions to practice System of particles and rotational motion ( quick revision formula )? tests, examples and also practice Class 11 tests.

|
Explore Courses for Class 11 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















