CAT Exam > CAT Questions > DIRECTIONS for questions: Select the correct...
Start Learning for Free
DIRECTIONS for questions: Select the correct alternative from the given choices.
A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?
- a)√1300
- b)30√2
- c)37.5
- d)40
Correct answer is option 'C'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
DIRECTIONS for questions: Select the correct alternative from the giv...
Consider the following figure of a rectangular paper ABCD.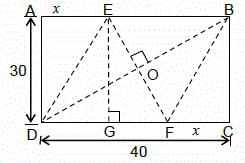
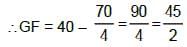
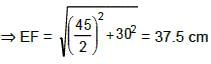
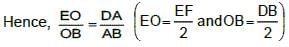
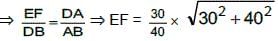
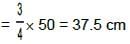
View all questions of this test

The line EF is the fold, which is made such that the corner D meets the diagonally opposite comer B. As DF coincides with FB upon making the fold DF = FB. Similarly, DE = EB. Also, as DF is the part of the length of the rectangle that is being folded so that D coincides with the opposite vertex and BE is the part of the length, that is being folded so that B coincides with D, DF = BE (from symmetry), i.e., quadrilateral DFBE is a rhombus (I) Now assume, FC = x cm
In the right angled triangle △BFC, BF =√BC2 + FC2 = √(30)2 + x2 = DF(since, EDBFis a rhombus and BF = DF)
Hence√302 + x2 = 40 - x
=> 900+x2= 1600 + x2– 80x
=> x = 700 / 80 = 35 / 4
Now, consider G on DC, such that EG ⊥DC. In △EGF, GF = 40 - 2x (as AE = FC = x), EG = 30 and EF is the length of the fold.
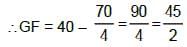
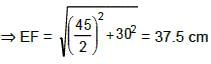
Alternative Solution
Consider the conclusion (I), i.e., that EDBF is a rhombus. Let the diagonals of the rhombus meet at O. In a rhombus, the diagonals bisect each other at right angles. Hence △EOB is similar to △DAB (both are right angled, with a common angle at B).
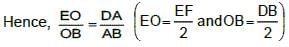
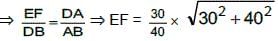
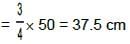
Most Upvoted Answer
DIRECTIONS for questions: Select the correct alternative from the giv...
Given:
A rectangular piece of paper with dimensions 40 cm × 30 cm.
To find:
The length of the fold when one pair of diagonally opposite vertices coincide.
Solution:
When a rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide, the fold creates a right-angled triangle.
Let's consider the dimensions of the rectangle as the base and height of the triangle. The diagonal of the rectangle will be the hypotenuse of the triangle.
Step 1: Find the length of the diagonal of the rectangle using the Pythagorean theorem.
The Pythagorean theorem states that in a right-angled triangle, the square of the hypotenuse (c) is equal to the sum of the squares of the other two sides (a and b).
Using the formula, we can calculate the length of the diagonal (c) as follows:
c² = a² + b²
where a = 40 cm and b = 30 cm
c² = 40² + 30²
c² = 1600 + 900
c² = 2500
c = √2500
c = 50 cm
Step 2: Fold the rectangle in such a way that one pair of diagonally opposite vertices coincide.
When the rectangle is folded, the length of the fold will be equal to the length of the hypotenuse of the right-angled triangle formed.
Therefore, the length of the fold = 50 cm.
Conclusion:
The length of the fold when a rectangular piece of paper with dimensions 40 cm × 30 cm is folded in such a way that one pair of diagonally opposite vertices coincide is 50 cm. Hence, option C is the correct answer.
A rectangular piece of paper with dimensions 40 cm × 30 cm.
To find:
The length of the fold when one pair of diagonally opposite vertices coincide.
Solution:
When a rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide, the fold creates a right-angled triangle.
Let's consider the dimensions of the rectangle as the base and height of the triangle. The diagonal of the rectangle will be the hypotenuse of the triangle.
Step 1: Find the length of the diagonal of the rectangle using the Pythagorean theorem.
The Pythagorean theorem states that in a right-angled triangle, the square of the hypotenuse (c) is equal to the sum of the squares of the other two sides (a and b).
Using the formula, we can calculate the length of the diagonal (c) as follows:
c² = a² + b²
where a = 40 cm and b = 30 cm
c² = 40² + 30²
c² = 1600 + 900
c² = 2500
c = √2500
c = 50 cm
Step 2: Fold the rectangle in such a way that one pair of diagonally opposite vertices coincide.
When the rectangle is folded, the length of the fold will be equal to the length of the hypotenuse of the right-angled triangle formed.
Therefore, the length of the fold = 50 cm.
Conclusion:
The length of the fold when a rectangular piece of paper with dimensions 40 cm × 30 cm is folded in such a way that one pair of diagonally opposite vertices coincide is 50 cm. Hence, option C is the correct answer.
Attention CAT Students!
To make sure you are not studying endlessly, EduRev has designed CAT study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in CAT.

|
Explore Courses for CAT exam
|

|
Similar CAT Doubts
DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer?
Question Description
DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer? for CAT 2024 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for CAT 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer?.
DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer? for CAT 2024 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for CAT 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer?.
Solutions for DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for CAT.
Download more important topics, notes, lectures and mock test series for CAT Exam by signing up for free.
Here you can find the meaning of DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer?, a detailed solution for DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer? has been provided alongside types of DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice DIRECTIONS for questions: Select the correct alternative from the given choices. A rectangular piece of paper is folded in such a way that one pair of diagonally opposite vertices coincide. If the dimensions of the rectangle are 40 cm × 30 cm, what is the length (in cm) of the fold?a) √1300b) 30√2c) 37.5d) 40Correct answer is option 'C'. Can you explain this answer? tests, examples and also practice CAT tests.

|
Explore Courses for CAT exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























