CAT Exam > CAT Questions > Directions for Questions:There are 21 employe...
Start Learning for Free
Directions for Questions:
There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.
In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.
(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.
(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.
Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team.
There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.
In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.
(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.
(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.
Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team.
(2017)
Q. The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.
- a)(2, 1)
- b)(1, 0)
- c)(0, 0)
- d)(1, 1)
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Directions for Questions:There are 21 employees working in a division,...
Given that there are 10 SE and 11 RE In the first month, since T1 has one more SE than T2, who in turn has one more SE than T3,... till T5, the number of SEs in T1, T2, T3, T4 and T5 must be 4, 3, 2, 1 and 0.
Also, the team that is assigned the challenging project has one more employees than the rest. Hence, the team that is assigned the challenging project will have 5 employees, while the other teams will have 4 employees.
Since T1 is assigned the challenging project in the first month, T1 will have 5 employees, and the other teams will have 4 employees each.
The following table provides the composition of the teams in the first month:
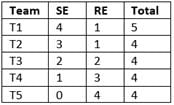
In the second month, T2 will be allotted the challenging project.
From a, two SEs will be transferred from Tl to T2. One RE is transferred from T2 to T1.
From b, one SE will be transferred from T1 to T5, one RE will be transferred from T5 to T1. Similar transfers will happen between T2 and T4.
The following table provides the number of employees in each team in the second month:
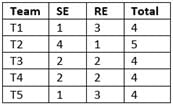
In the third month, T3 will be allotted the challenging project.
From a, two SEs will be transferred from T2 to T3. One RE is transferred from T3 to T2.
From b, one SE will be transferred from T1 to T5, one RE will be transferred from T5 to T1.
Also, one SE will be transferred from T2 to T4 and one RE will be transferred from T4 to T2.
The following table provides the number of employees in each team in the third month:
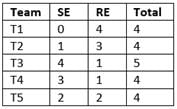
In the fourth month, T4 will be allotted the challenging project.
From a, two SEs will be transferred from T3 to T4. One RE is transferred from T4 to T3.
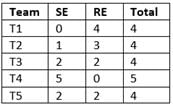
From b, one SE must be transferred from T1 to T5.
However, since there are no SEs in T1, this will not happen. Also, one SE must be transferred from T2 to T4 and one RE must be transferred from T4 to T2.
However, there are no REs in T4.
Hence, this transfer will not happen.
The following table provides the number of employees in each team in the fourth month: In the fifth month, T5 will be allotted the challenging project.
From a, two SEs will be transferred from T4 to T5. One RE is transferred trom T5 to T4.
From b, one SE must be transferred from T1 to T5.
However, since there are no SEs in T1, this will not happen.
Also, one SE will be transferred from T2 to T4 and one RE will be transferred from T4 to T2.
The following table provides the number of employees in each team in the fifth month.
The composition of T2 did not change once between the third and the fourth months.
The composition of T4 changed between any two successive months.
Hence, the answer is (1, 0).
Also, the team that is assigned the challenging project has one more employees than the rest. Hence, the team that is assigned the challenging project will have 5 employees, while the other teams will have 4 employees.
Since T1 is assigned the challenging project in the first month, T1 will have 5 employees, and the other teams will have 4 employees each.
The following table provides the composition of the teams in the first month:

In the second month, T2 will be allotted the challenging project.
From a, two SEs will be transferred from Tl to T2. One RE is transferred from T2 to T1.
From b, one SE will be transferred from T1 to T5, one RE will be transferred from T5 to T1. Similar transfers will happen between T2 and T4.
The following table provides the number of employees in each team in the second month:

In the third month, T3 will be allotted the challenging project.
From a, two SEs will be transferred from T2 to T3. One RE is transferred from T3 to T2.
From b, one SE will be transferred from T1 to T5, one RE will be transferred from T5 to T1.
Also, one SE will be transferred from T2 to T4 and one RE will be transferred from T4 to T2.
The following table provides the number of employees in each team in the third month:

In the fourth month, T4 will be allotted the challenging project.
From a, two SEs will be transferred from T3 to T4. One RE is transferred from T4 to T3.

From b, one SE must be transferred from T1 to T5.
However, since there are no SEs in T1, this will not happen. Also, one SE must be transferred from T2 to T4 and one RE must be transferred from T4 to T2.
However, there are no REs in T4.
Hence, this transfer will not happen.
The following table provides the number of employees in each team in the fourth month: In the fifth month, T5 will be allotted the challenging project.
From a, two SEs will be transferred from T4 to T5. One RE is transferred trom T5 to T4.
From b, one SE must be transferred from T1 to T5.
However, since there are no SEs in T1, this will not happen.
Also, one SE will be transferred from T2 to T4 and one RE will be transferred from T4 to T2.
The following table provides the number of employees in each team in the fifth month.

The composition of T2 did not change once between the third and the fourth months.
The composition of T4 changed between any two successive months.
Hence, the answer is (1, 0).
Most Upvoted Answer
Directions for Questions:There are 21 employees working in a division,...
Understanding Team Composition Changes
To analyze the changes in the composition of T2 and T4 over the five months, it's essential to understand the initial conditions and the rules governing team changes.
Initial Conditions
- **Team Composition**:
- Let’s assume the number of SE in T5 is x.
- Therefore, T4 has x+1 SE, T3 has x+2 SE, T2 has x+3 SE, and T1 has x+4 SE.
- **Total SE**:
- Total SE = 10, indicating that x must be a non-negative integer satisfying the conditions.
Composition Changes Rules
1. **Challenging Project Exchange**:
- Each month, the team assigned the challenging project (e.g., T1 in Month 1) receives 2 SE from the previous month’s challenging project team while transferring 1 RE back.
2. **Additional SE and RE Shifts**:
- If T1 has SE and T5 has RE, then 1 SE is shifted from T1 to T5 and 1 RE from T5 to T1.
- If T2 has SE and T4 has RE, then 1 SE is shifted from T2 to T4 and 1 RE from T4 to T2.
Analysis of T2 and T4 Changes
- **Months 1 to 2**:
- T1 becomes T2’s previous team, resulting in T2 losing SE to T4. Changes occur.
- **Months 2 to 3**:
- T2 will again change due to the transfer of SE to T4; changes occur.
- **Months 3 to 4**:
- The same logic follows; T2 changes.
- **Months 4 to 5**:
- T4 may change due to the SE from T2, but the exact SE counts will confirm this.
Conclusion
- T2's composition changes in all months = 4 times.
- T4 remains unchanged once due to the exchange constraints.
Thus, the answer is option **(1, 0)** for T2 and T4, respectively, confirming the choice is option **B**.
To analyze the changes in the composition of T2 and T4 over the five months, it's essential to understand the initial conditions and the rules governing team changes.
Initial Conditions
- **Team Composition**:
- Let’s assume the number of SE in T5 is x.
- Therefore, T4 has x+1 SE, T3 has x+2 SE, T2 has x+3 SE, and T1 has x+4 SE.
- **Total SE**:
- Total SE = 10, indicating that x must be a non-negative integer satisfying the conditions.
Composition Changes Rules
1. **Challenging Project Exchange**:
- Each month, the team assigned the challenging project (e.g., T1 in Month 1) receives 2 SE from the previous month’s challenging project team while transferring 1 RE back.
2. **Additional SE and RE Shifts**:
- If T1 has SE and T5 has RE, then 1 SE is shifted from T1 to T5 and 1 RE from T5 to T1.
- If T2 has SE and T4 has RE, then 1 SE is shifted from T2 to T4 and 1 RE from T4 to T2.
Analysis of T2 and T4 Changes
- **Months 1 to 2**:
- T1 becomes T2’s previous team, resulting in T2 losing SE to T4. Changes occur.
- **Months 2 to 3**:
- T2 will again change due to the transfer of SE to T4; changes occur.
- **Months 3 to 4**:
- The same logic follows; T2 changes.
- **Months 4 to 5**:
- T4 may change due to the SE from T2, but the exact SE counts will confirm this.
Conclusion
- T2's composition changes in all months = 4 times.
- T4 remains unchanged once due to the exchange constraints.
Thus, the answer is option **(1, 0)** for T2 and T4, respectively, confirming the choice is option **B**.
Free Test
FREE
| Start Free Test |
Community Answer
Directions for Questions:There are 21 employees working in a division,...
Given that there are 10 SE and 11 RE In the first month, since T1 has one more SE than T2, who in turn has one more SE than T3,... till T5, the number of SEs in T1, T2, T3, T4 and T5 must be 4, 3, 2, 1 and 0.
Also, the team that is assigned the challenging project has one more employees than the rest. Hence, the team that is assigned the challenging project will have 5 employees, while the other teams will have 4 employees.
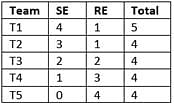
Since T1 is assigned the challenging project in the first month, T1 will have 5 employees, and the other teams will have 4 employees each.
The following table provides the composition of the teams in the first month:
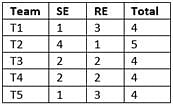
In the second month, T2 will be allotted the challenging project.
From a, two SEs will be transferred from Tl to T2. One RE is transferred from T2 to T1.
From b, one SE will be transferred from T1 to T5, one RE will be transferred from T5 to T1. Similar transfers will happen between T2 and T4.
The following table provides the number of employees in each team in the second month:
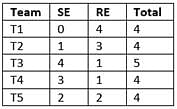
In the third month, T3 will be allotted the challenging project.
From a, two SEs will be transferred from T2 to T3. One RE is transferred from T3 to T2.
From b, one SE will be transferred from T1 to T5, one RE will be transferred from T5 to T1.
Also, one SE will be transferred from T2 to T4 and one RE will be transferred from T4 to T2.
The following table provides the number of employees in each team in the third month:
In the fourth month, T4 will be allotted the challenging project.
From a, two SEs will be transferred from T3 to T4. One RE is transferred from T4 to T3.
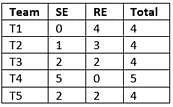
From b, one SE must be transferred from T1 to T5.
However, since there are no SEs in T1, this will not happen. Also, one SE must be transferred from T2 to T4 and one RE must be transferred from T4 to T2.
However, there are no REs in T4.
Hence, this transfer will not happen.
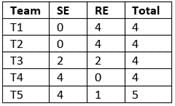
The following table provides the number of employees in each team in the fourth month: In the fifth month, T5 will be allotted the challenging project.
From a, two SEs will be transferred from T4 to T5. One RE is transferred trom T5 to T4.
From b, one SE must be transferred from T1 to T5.
However, since there are no SEs in T1, this will not happen.
Also, one SE will be transferred from T2 to T4 and one RE will be transferred from T4 to T2.
The following table provides the number of employees in each team in the fifth month.
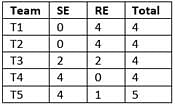
The composition of T2 did not change once between the third and the fourth months.
The composition of T4 changed between any two successive months.
Hence, the answer is (1, 0).
Also, the team that is assigned the challenging project has one more employees than the rest. Hence, the team that is assigned the challenging project will have 5 employees, while the other teams will have 4 employees.
Since T1 is assigned the challenging project in the first month, T1 will have 5 employees, and the other teams will have 4 employees each.
The following table provides the composition of the teams in the first month:

In the second month, T2 will be allotted the challenging project.
From a, two SEs will be transferred from Tl to T2. One RE is transferred from T2 to T1.
From b, one SE will be transferred from T1 to T5, one RE will be transferred from T5 to T1. Similar transfers will happen between T2 and T4.
The following table provides the number of employees in each team in the second month:

In the third month, T3 will be allotted the challenging project.
From a, two SEs will be transferred from T2 to T3. One RE is transferred from T3 to T2.
From b, one SE will be transferred from T1 to T5, one RE will be transferred from T5 to T1.
Also, one SE will be transferred from T2 to T4 and one RE will be transferred from T4 to T2.
The following table provides the number of employees in each team in the third month:

In the fourth month, T4 will be allotted the challenging project.
From a, two SEs will be transferred from T3 to T4. One RE is transferred from T4 to T3.

From b, one SE must be transferred from T1 to T5.
However, since there are no SEs in T1, this will not happen. Also, one SE must be transferred from T2 to T4 and one RE must be transferred from T4 to T2.
However, there are no REs in T4.
Hence, this transfer will not happen.
The following table provides the number of employees in each team in the fourth month: In the fifth month, T5 will be allotted the challenging project.
From a, two SEs will be transferred from T4 to T5. One RE is transferred trom T5 to T4.
From b, one SE must be transferred from T1 to T5.
However, since there are no SEs in T1, this will not happen.
Also, one SE will be transferred from T2 to T4 and one RE will be transferred from T4 to T2.
The following table provides the number of employees in each team in the fifth month.

The composition of T2 did not change once between the third and the fourth months.
The composition of T4 changed between any two successive months.
Hence, the answer is (1, 0).
Attention CAT Students!
To make sure you are not studying endlessly, EduRev has designed CAT study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in CAT.

|
Explore Courses for CAT exam
|

|
Similar CAT Doubts
Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer?
Question Description
Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer? for CAT 2024 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for CAT 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer?.
Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer? for CAT 2024 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for CAT 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer?.
Solutions for Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for CAT.
Download more important topics, notes, lectures and mock test series for CAT Exam by signing up for free.
Here you can find the meaning of Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer?, a detailed solution for Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer? has been provided alongside types of Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Directions for Questions:There are 21 employees working in a division, out of whom 10 are special-skilled employees (SE) and the remaining are regular skilled employees (RE). During the next five months, the division has to complete five projects every month. Out of the 25 projects, 5 projects are "challenging", while the remaining ones are "standard". Each of the challenging projects has to be completed in different months. Every month, five teams T1, T2, T3, T4 and T5, work on one project each. T1, T2, T3, T4 and T5 are allotted the challenging project in the first, second, third fourth and fifth month, respectively. The team assigned the challenging project has one more employee than the rest.In the first month, T1 has one more SE than T2, T2 has one more SE than T3, T3 has one more SE than T4, and T4 has one more SE than T5. Between two successive months, the composition of the teams changes as follows.(a) The team allotted the challenging project, gets two SE from the team which was allotted the challenging project in the previous month. In exchange. one RE is shifted from the former team to the latter team.(b) After the above exchange, if T1 has any SE and T5 has any RE, then one SE is shifted from T1 to T5, and one RE is shifted from T5 to T1. Also, if T2 has any SE and T4 has any RE, then one SE is shifted from T2 to T4, and one RE is shifted from T4 to T2.Each standard project has a total of 100 credit points, while each challenging project has 200 credit points. The credit points are equally shared between the employees included in that team. (2017)Q.The number of times in which the composition of team T2 and the number of times in which composition of team T4 remained unchanged in two successive months are.a)(2, 1)b)(1, 0)c)(0, 0)d)(1, 1)Correct answer is option 'B'. Can you explain this answer? tests, examples and also practice CAT tests.

|
Explore Courses for CAT exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























