Computer Science Engineering (CSE) Exam > Computer Science Engineering (CSE) Questions > What is the largest integer m such that every...
Start Learning for Free
What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?
- a)1
- b)2
- c)3
- d)n
Correct answer is option 'C'. Can you explain this answer?
Most Upvoted Answer
What is the largest integer m such that every simple connected graph w...
A graph is connected iff all nodes can be traversed from each node. For a graph with n nodes, there will be n-1 minimum number of edges.
Given that there are n edges, that means a cycle is there in the graph.
The simplex graph with these conditions may be:
Given that there are n edges, that means a cycle is there in the graph.
The simplex graph with these conditions may be:
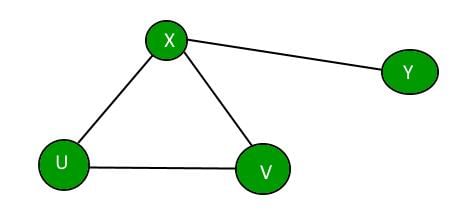
Now we can make a different spanning tree by removing one edge from the cycle, one at a time.
Minimum cycle length can be 3, So, there must be atleast 3 spanning trees in any such Graph.
Minimum cycle length can be 3, So, there must be atleast 3 spanning trees in any such Graph.
Free Test
FREE
| Start Free Test |
Community Answer
What is the largest integer m such that every simple connected graph w...
Solution:
We have to find the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees.
Spanning Tree:
A spanning tree T of an undirected graph G is a subgraph that is a tree and includes all the vertices of G. A tree is a connected graph without any cycles.
Number of Spanning Trees:
The number of spanning trees for a connected graph G with n vertices can be calculated using Kirchhoff's theorem:
The number of spanning trees is equal to any cofactor of the Laplacian matrix L(G) of G.
Laplacian Matrix:
The Laplacian matrix L(G) of a graph G is defined as the difference between the degree matrix D(G) and the adjacency matrix A(G) of G.
L(G) = D(G) - A(G)
where D(G) is a diagonal matrix with the degrees of the vertices on the diagonal and A(G) is the adjacency matrix of G.
Minimum Spanning Tree:
A minimum spanning tree (MST) of a connected weighted graph G is a spanning tree that has the minimum sum of edge weights.
Kruskal's Algorithm:
Kruskal's algorithm is a greedy algorithm that finds a minimum spanning tree for a connected weighted graph. The algorithm starts with an empty set of edges and iteratively adds the next lightest edge that does not form a cycle until all vertices are connected.
Explanation:
We have to find the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees.
Number of Spanning Trees:
From Kirchhoff's theorem, the number of spanning trees for a connected graph G with n vertices can be calculated using any cofactor of the Laplacian matrix L(G) of G.
L(G) = D(G) - A(G)
where D(G) is a diagonal matrix with the degrees of the vertices on the diagonal and A(G) is the adjacency matrix of G.
Minimum Spanning Tree:
Kruskal's algorithm is a greedy algorithm that finds a minimum spanning tree for a connected weighted graph. The algorithm starts with an empty set of edges and iteratively adds the next lightest edge that does not form a cycle until all vertices are connected.
Explanation of options:
a) 1: This option is not correct because a connected graph with n vertices and n edges can have more than one spanning tree. For example, a cycle of n vertices has n different spanning trees.
b) 2: This option is not correct because a connected graph with n vertices and n edges can have more than two spanning trees. For example, a complete graph of n vertices has n^(n-2) different spanning trees.
c) 3: This option is correct because every simple connected graph with n vertices and n edges contains at least 3 different spanning trees. This can be proved using Kruskal's algorithm. The first edge added to the minimum spanning tree can be any of the n edges. The second edge added cannot be the same as the first edge, so there are n-1 choices. The third edge added cannot form a cycle with the first two edges, so there are at least n-2 choices. Therefore, there are at least n*(n-1)*(n-2) different ordered triples of edges that form a spanning tree. However, each spanning tree has n-2 edges, so each spanning tree is counted
We have to find the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees.
Spanning Tree:
A spanning tree T of an undirected graph G is a subgraph that is a tree and includes all the vertices of G. A tree is a connected graph without any cycles.
Number of Spanning Trees:
The number of spanning trees for a connected graph G with n vertices can be calculated using Kirchhoff's theorem:
The number of spanning trees is equal to any cofactor of the Laplacian matrix L(G) of G.
Laplacian Matrix:
The Laplacian matrix L(G) of a graph G is defined as the difference between the degree matrix D(G) and the adjacency matrix A(G) of G.
L(G) = D(G) - A(G)
where D(G) is a diagonal matrix with the degrees of the vertices on the diagonal and A(G) is the adjacency matrix of G.
Minimum Spanning Tree:
A minimum spanning tree (MST) of a connected weighted graph G is a spanning tree that has the minimum sum of edge weights.
Kruskal's Algorithm:
Kruskal's algorithm is a greedy algorithm that finds a minimum spanning tree for a connected weighted graph. The algorithm starts with an empty set of edges and iteratively adds the next lightest edge that does not form a cycle until all vertices are connected.
Explanation:
We have to find the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees.
Number of Spanning Trees:
From Kirchhoff's theorem, the number of spanning trees for a connected graph G with n vertices can be calculated using any cofactor of the Laplacian matrix L(G) of G.
L(G) = D(G) - A(G)
where D(G) is a diagonal matrix with the degrees of the vertices on the diagonal and A(G) is the adjacency matrix of G.
Minimum Spanning Tree:
Kruskal's algorithm is a greedy algorithm that finds a minimum spanning tree for a connected weighted graph. The algorithm starts with an empty set of edges and iteratively adds the next lightest edge that does not form a cycle until all vertices are connected.
Explanation of options:
a) 1: This option is not correct because a connected graph with n vertices and n edges can have more than one spanning tree. For example, a cycle of n vertices has n different spanning trees.
b) 2: This option is not correct because a connected graph with n vertices and n edges can have more than two spanning trees. For example, a complete graph of n vertices has n^(n-2) different spanning trees.
c) 3: This option is correct because every simple connected graph with n vertices and n edges contains at least 3 different spanning trees. This can be proved using Kruskal's algorithm. The first edge added to the minimum spanning tree can be any of the n edges. The second edge added cannot be the same as the first edge, so there are n-1 choices. The third edge added cannot form a cycle with the first two edges, so there are at least n-2 choices. Therefore, there are at least n*(n-1)*(n-2) different ordered triples of edges that form a spanning tree. However, each spanning tree has n-2 edges, so each spanning tree is counted

|
Explore Courses for Computer Science Engineering (CSE) exam
|

|
Question Description
What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer? for Computer Science Engineering (CSE) 2025 is part of Computer Science Engineering (CSE) preparation. The Question and answers have been prepared according to the Computer Science Engineering (CSE) exam syllabus. Information about What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Computer Science Engineering (CSE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer?.
What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer? for Computer Science Engineering (CSE) 2025 is part of Computer Science Engineering (CSE) preparation. The Question and answers have been prepared according to the Computer Science Engineering (CSE) exam syllabus. Information about What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Computer Science Engineering (CSE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer?.
Solutions for What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Computer Science Engineering (CSE).
Download more important topics, notes, lectures and mock test series for Computer Science Engineering (CSE) Exam by signing up for free.
Here you can find the meaning of What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice What is the largest integer m such that every simple connected graph with n vertices and n edges contains at least m different spanning trees?a)1b)2c)3d)nCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice Computer Science Engineering (CSE) tests.

|
Explore Courses for Computer Science Engineering (CSE) exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















