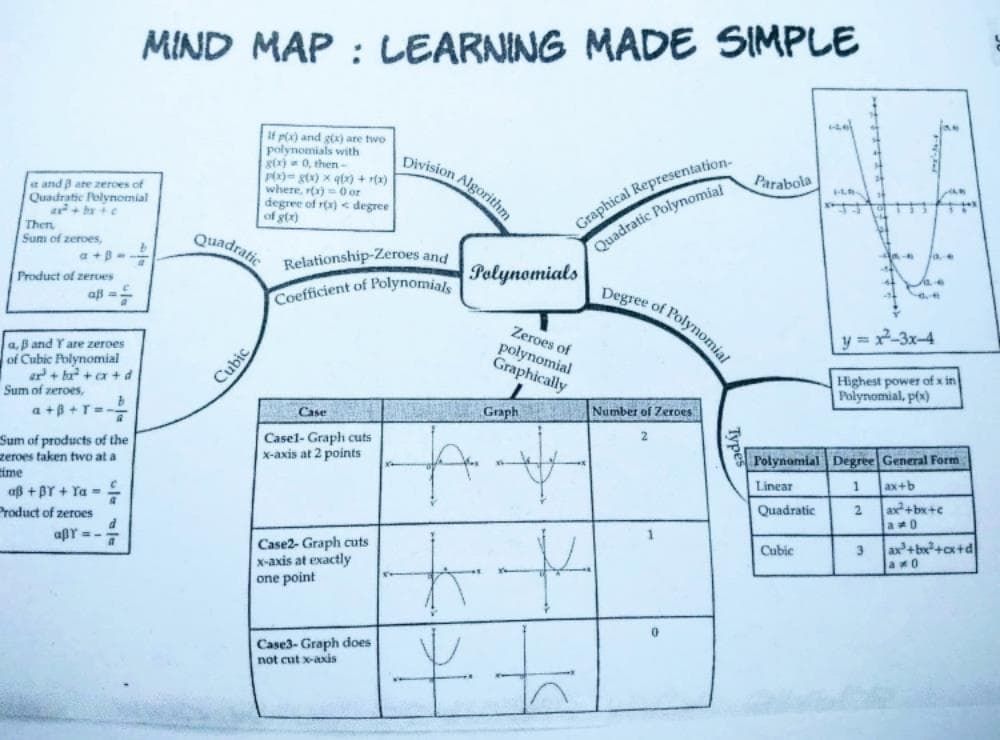
Polynomials is an important chapter in Class 10 Mathematics that deals with algebraic expressions containing one or more terms. A polynomial can be further categorized as a monomial, binomial, trinomial, or a polynomial with more than three terms. The chapter covers various topics like degree, coefficient, zeroes of a polynomial, factorization, and algebraic identities. A mind map on this chapter can greatly help in understanding these concepts in a better way.

Degree of a Polynomial:
- A polynomial is an expression containing one or more terms.
- The degree of a polynomial is the highest power of the variable in the polynomial.
- It can be further categorized as constant, linear, quadratic, cubic, or higher degree polynomial.

Coefficient of a Polynomial:
- The coefficient of a term in a polynomial is the numerical factor that multiplies the variable.
- In the polynomial 3x^2 + 5x + 7, the coefficient of x^2 is 3, the coefficient of x is 5, and the coefficient of the constant term is 7.

Zeroes of a Polynomial:
- A zero of a polynomial is a value of the variable that makes the polynomial equal to zero.
- A polynomial of degree n has at most n zeroes.
- The Factor theorem states that if f(a) = 0, then (x-a) is a factor of the polynomial f(x).

Factorization of Polynomials:
- Factorization is the process of expressing a polynomial as a product of its factors.
- The Remainder theorem states that if a polynomial f(x) is divided by (x-a), then the remainder is f(a).
- The Factor theorem and the Remainder theorem can be used to factorize polynomials.

Algebraic Identities:
- Algebraic identities are equations that are true for all values of the variables.
- Some important algebraic identities are (a+b)^2 = a^2 + 2ab + b^2, (a-b)^2 = a^2 - 2ab + b^2, and a^3 + b^3 = (a+b)(a^2 - ab + b^2).

In conclusion, mastering the concepts of Polynomials is important for a strong foundation in algebraic expressions. A mind map can help students visualize the relationships between the different concepts and improve their understanding of the chapter.