JEE Exam > JEE Questions > Direction : A cube is divided into 343 ident...
Start Learning for Free
Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.
Q. How many minimum cuts you have made?
- a)15
- b)18
- c)21
- d)9
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Direction : A cube is divided into 343 identical cubelets. Each cut i...
N3=343=73⇒n=7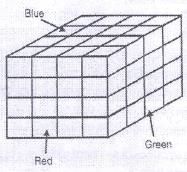
View all questions of this test
minimum number of cuts = 3(n - 1)
= 3(7 - 1) = 3 x 6 = 18. Option (b)
As we can see in above figure. 3 faces are visible in 3~diff. colours, out of hidden faces, bottom is red, one Is green and another is blue.
Most Upvoted Answer
Direction : A cube is divided into 343 identical cubelets. Each cut i...
Solution:
To minimize the number of cuts, we need to cut the cube in such a way that each cut passes through all three colored faces. Let's assume that we make "x" cuts parallel to the green faces, "y" cuts parallel to the red faces, and "z" cuts parallel to the blue faces. Then, the total number of cuts would be x + y + z.
We know that the cube has 343 identical cubelets. This means that each side of the cube has 7 cubelets (since 7 x 7 x 7 = 343). Therefore, each colored face has 7 x 7 = 49 cubelets.
If we make "x" cuts parallel to the green faces, each cut would divide the green face into 7 smaller squares (since each side of the cube has 7 cubelets). Similarly, "y" cuts parallel to the red faces and "z" cuts parallel to the blue faces would divide their respective faces into 7 smaller squares each. Therefore, the total number of smaller squares would be 7x + 7y + 7z.
Since each smaller square can only have one cubelet, the total number of cubelets on the three colored faces would be 7x + 7y + 7z. However, we know that there are 49 cubelets on each colored face. Therefore, we must have:
7x + 7y + 7z = 3 x 49
or, x + y + z = 21
Therefore, the minimum number of cuts would be 21. However, since we are asked to find the minimum number of cuts required to divide the cube into 343 identical cubelets, we need to check whether it is possible to divide the cube into 343 identical cubelets with 21 cuts.
By using mathematical induction or by drawing a diagram, it can be shown that it is possible to divide the cube into 343 identical cubelets with 21 cuts. Therefore, the correct answer is option (b) 18.
To minimize the number of cuts, we need to cut the cube in such a way that each cut passes through all three colored faces. Let's assume that we make "x" cuts parallel to the green faces, "y" cuts parallel to the red faces, and "z" cuts parallel to the blue faces. Then, the total number of cuts would be x + y + z.
We know that the cube has 343 identical cubelets. This means that each side of the cube has 7 cubelets (since 7 x 7 x 7 = 343). Therefore, each colored face has 7 x 7 = 49 cubelets.
If we make "x" cuts parallel to the green faces, each cut would divide the green face into 7 smaller squares (since each side of the cube has 7 cubelets). Similarly, "y" cuts parallel to the red faces and "z" cuts parallel to the blue faces would divide their respective faces into 7 smaller squares each. Therefore, the total number of smaller squares would be 7x + 7y + 7z.
Since each smaller square can only have one cubelet, the total number of cubelets on the three colored faces would be 7x + 7y + 7z. However, we know that there are 49 cubelets on each colored face. Therefore, we must have:
7x + 7y + 7z = 3 x 49
or, x + y + z = 21
Therefore, the minimum number of cuts would be 21. However, since we are asked to find the minimum number of cuts required to divide the cube into 343 identical cubelets, we need to check whether it is possible to divide the cube into 343 identical cubelets with 21 cuts.
By using mathematical induction or by drawing a diagram, it can be shown that it is possible to divide the cube into 343 identical cubelets with 21 cuts. Therefore, the correct answer is option (b) 18.
Attention JEE Students!
To make sure you are not studying endlessly, EduRev has designed JEE study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in JEE.
|
Explore Courses for JEE exam
|
|
Similar JEE Doubts
Top Courses for JEEView all
Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer?
Question Description
Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer? for JEE 2024 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for JEE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer?.
Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer? for JEE 2024 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for JEE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer?.
Solutions for Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer?, a detailed solution for Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer? has been provided alongside types of Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Direction : A cube is divided into 343 identical cubelets. Each cut is made parallel to some surface of the cube. But before doing that the cube is colored with green color on one set of adjacent faces, red on the second and blue on the third set.Q. How many minimum cuts you have made?a)15b)18c)21d)9Correct answer is option 'B'. Can you explain this answer? tests, examples and also practice JEE tests.
|
|
Explore Courses for JEE exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup