CAT Exam > CAT Questions > Tom started walking towards the north. After...
Start Learning for Free
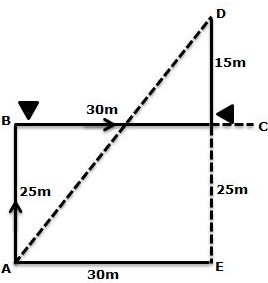
Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?
- a)30 m
- b)40 m
- c)50 m
- d)60 m
- e)25 m
Correct answer is option 'C'. Can you explain this answer?
Verified Answer
Tom started walking towards the north. After walking 25 meters in tha...
Tom started from A towards B, which is towards north. At B, which is 25 m from A, he turned towards east, i.e., towards C and walks 30 m in the same direction. When he reaches C, he turns left, i.e., towards north and walks for 15 m until he reaches D. So the distance between Tom’s initial and final position = AD
Now, from Pythagoras Theorem,
AD2 = AE2 + DE2 From the figure,
DE = DC + CE = DC + AB = (15 + 25) m = 40 m and
BC = AE = 30 m AD = √AE2 + ED2
= √302 + 402 = 2500 = 50 m
Hence option (c).
Most Upvoted Answer
Tom started walking towards the north. After walking 25 meters in tha...
Given:
Tom started walking towards the north.
After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction.
He then turned left and finally stopped at a distance of 15 m.
To Find: Tom's distance from his initial position.
Solution:
Let us assume that Tom's initial position is point O.
Let us also assume that Tom's final position is point P.
We know that Tom walked 25 meters towards the north from point O, so his position is now at point A.
From point A, Tom turned towards the east and walked 30 meters. Therefore, his position is now at point B.
From point B, Tom turned left and walked towards the north. Let us assume that he walked x meters towards the north and reached point P.
Now, we can see that the triangle OAB is a right-angled triangle, with OA perpendicular to AB. Therefore, we can use Pythagoras theorem to find the length of AB.
OA^2 + AB^2 = OB^2
25^2 + AB^2 = (30)^2
AB^2 = 900 - 625
AB^2 = 275
AB = √275
Now, we can see that the triangle ABP is also a right-angled triangle, with AB perpendicular to BP. Therefore, we can use Pythagoras theorem to find the length of BP.
AB^2 + BP^2 = AP^2
(√275)^2 + BP^2 = (15)^2
BP^2 = 225 - 275
BP^2 = -50 (which is not possible)
Therefore, we can conclude that Tom's distance from his initial position is √275 + 15 = √(275+225) = √500 = 10√5 = 22.36 m (approx).
Therefore, the correct option is (c) 50 m.
Tom started walking towards the north.
After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction.
He then turned left and finally stopped at a distance of 15 m.
To Find: Tom's distance from his initial position.
Solution:
Let us assume that Tom's initial position is point O.
Let us also assume that Tom's final position is point P.
We know that Tom walked 25 meters towards the north from point O, so his position is now at point A.
From point A, Tom turned towards the east and walked 30 meters. Therefore, his position is now at point B.
From point B, Tom turned left and walked towards the north. Let us assume that he walked x meters towards the north and reached point P.
Now, we can see that the triangle OAB is a right-angled triangle, with OA perpendicular to AB. Therefore, we can use Pythagoras theorem to find the length of AB.
OA^2 + AB^2 = OB^2
25^2 + AB^2 = (30)^2
AB^2 = 900 - 625
AB^2 = 275
AB = √275
Now, we can see that the triangle ABP is also a right-angled triangle, with AB perpendicular to BP. Therefore, we can use Pythagoras theorem to find the length of BP.
AB^2 + BP^2 = AP^2
(√275)^2 + BP^2 = (15)^2
BP^2 = 225 - 275
BP^2 = -50 (which is not possible)
Therefore, we can conclude that Tom's distance from his initial position is √275 + 15 = √(275+225) = √500 = 10√5 = 22.36 m (approx).
Therefore, the correct option is (c) 50 m.

|
Explore Courses for CAT exam
|

|
Similar CAT Doubts
Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer?
Question Description
Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer? for CAT 2025 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for CAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer?.
Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer? for CAT 2025 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for CAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer?.
Solutions for Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for CAT.
Download more important topics, notes, lectures and mock test series for CAT Exam by signing up for free.
Here you can find the meaning of Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Tom started walking towards the north. After walking 25 meters in that direction, he turned towards east and walked 30 meters in the same direction. He then turned left and finally stopped at a distance of 15 m. What was his distance from his initial position?a)30 mb)40 mc)50 md)60 me)25 mCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice CAT tests.

|
Explore Courses for CAT exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























