Computer Science Engineering (CSE) Exam > Computer Science Engineering (CSE) Questions > A square band matrix Dn,a is a n*n matrix in ...
Start Learning for Free
A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.
If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?
Correct answer is '12'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Most Upvoted Answer
A square band matrix Dn,a is a n*n matrix in which all the non-zero te...
Explanation:
To understand the location of the element D0,2 in the one-dimensional array a(0, 1, ..., 13), we need to first understand how the band matrix D4,3 is mapped onto the array.
Mapping the Band Matrix:
The band matrix D4,3 has a size of 4*4 and a band width of 3. This means that all the non-zero elements are located within a band of width 3 centered around the main diagonal. The band includes the main diagonal and 3 diagonals below and above it.
To map this band matrix onto a one-dimensional array, we start with the lowest diagonal and move upwards. The elements in each diagonal are stored in consecutive positions in the array.
Mapping the Band Matrix D4,3:
The band matrix D4,3 can be represented as follows:
| d00 d01 d02 0 |
| d10 d11 d12 d13 |
| 0 d21 d22 d23 |
| 0 0 d32 d33 |
Here, dij represents the element in the ith row and jth column of the matrix.
Mapping this matrix onto the array a(0, 1, ..., 13) by diagonals, we get:
a(0) = d00
a(1) = d10
a(2) = d01
a(3) = d21
a(4) = d11
a(5) = d02
a(6) = d32
a(7) = d12
a(8) = d22
a(9) = d13
a(10) = d23
a(11) = d33
a(12) = 0
a(13) = 0
Locating the Element D0,2:
We are interested in finding the location of the element D0,2, which is the element in the 0th row and 2nd column of the matrix.
From the mapping above, we can see that D0,2 is mapped to the 5th position in the array, which is a(5). Therefore, the element D0,2 is stored in the location 5 of the one-dimensional array a(0, 1, ..., 13).
Conclusion:
The element D0,2 is stored in the location 5 of the one-dimensional array a(0, 1, ..., 13).
To understand the location of the element D0,2 in the one-dimensional array a(0, 1, ..., 13), we need to first understand how the band matrix D4,3 is mapped onto the array.
Mapping the Band Matrix:
The band matrix D4,3 has a size of 4*4 and a band width of 3. This means that all the non-zero elements are located within a band of width 3 centered around the main diagonal. The band includes the main diagonal and 3 diagonals below and above it.
To map this band matrix onto a one-dimensional array, we start with the lowest diagonal and move upwards. The elements in each diagonal are stored in consecutive positions in the array.
Mapping the Band Matrix D4,3:
The band matrix D4,3 can be represented as follows:
| d00 d01 d02 0 |
| d10 d11 d12 d13 |
| 0 d21 d22 d23 |
| 0 0 d32 d33 |
Here, dij represents the element in the ith row and jth column of the matrix.
Mapping this matrix onto the array a(0, 1, ..., 13) by diagonals, we get:
a(0) = d00
a(1) = d10
a(2) = d01
a(3) = d21
a(4) = d11
a(5) = d02
a(6) = d32
a(7) = d12
a(8) = d22
a(9) = d13
a(10) = d23
a(11) = d33
a(12) = 0
a(13) = 0
Locating the Element D0,2:
We are interested in finding the location of the element D0,2, which is the element in the 0th row and 2nd column of the matrix.
From the mapping above, we can see that D0,2 is mapped to the 5th position in the array, which is a(5). Therefore, the element D0,2 is stored in the location 5 of the one-dimensional array a(0, 1, ..., 13).
Conclusion:
The element D0,2 is stored in the location 5 of the one-dimensional array a(0, 1, ..., 13).
Free Test
FREE
| Start Free Test |
Community Answer
A square band matrix Dn,a is a n*n matrix in which all the non-zero te...
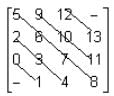
D0,2 is stored at 12.
Attention Computer Science Engineering (CSE) Students!
To make sure you are not studying endlessly, EduRev has designed Computer Science Engineering (CSE) study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Computer Science Engineering (CSE).

|
Explore Courses for Computer Science Engineering (CSE) exam
|

|
Similar Computer Science Engineering (CSE) Doubts
A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer?
Question Description
A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer? for Computer Science Engineering (CSE) 2024 is part of Computer Science Engineering (CSE) preparation. The Question and answers have been prepared according to the Computer Science Engineering (CSE) exam syllabus. Information about A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer? covers all topics & solutions for Computer Science Engineering (CSE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer?.
A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer? for Computer Science Engineering (CSE) 2024 is part of Computer Science Engineering (CSE) preparation. The Question and answers have been prepared according to the Computer Science Engineering (CSE) exam syllabus. Information about A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer? covers all topics & solutions for Computer Science Engineering (CSE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer?.
Solutions for A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer? in English & in Hindi are available as part of our courses for Computer Science Engineering (CSE).
Download more important topics, notes, lectures and mock test series for Computer Science Engineering (CSE) Exam by signing up for free.
Here you can find the meaning of A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer?, a detailed solution for A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer? has been provided alongside types of A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A square band matrix Dn,a is a n*n matrix in which all the non-zero terms lie in a band centered around the main diagonal. The band includes the main diagonal and (a - 1) diagonals below and above the main diagonal.If the band matrix D4,3 is mapped on to a one dimensional array a(0, 1, ...., 13) by diagonals, starting with the lowest diagonal, then in which location is the element D0,2 stored?Correct answer is '12'. Can you explain this answer? tests, examples and also practice Computer Science Engineering (CSE) tests.

|
Explore Courses for Computer Science Engineering (CSE) exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























